Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

394

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

We replace a, c and xi, by aqlnl, cq-lnl and ~iq-~i,

1,.

. .

respec-

tively. After some elementary manipulations we obtain

Next, we replace c by z and let ni

oo,

for

1,.

. .

(assuming

lazl

and

lbl

I), while appealing to Tannery's theorem. Finally, we

apply the simple identity

and arrive at (5.2). For establishing the conditions of convergence of the

series, see the Appendix.

Next, we provide the following multilateral generalization of (4.6).

Theorem

5.3.

Let a,

z, and

XI, . . .

xT be indeterminate. Then

provided max(lazl,

Ibl)

Proof. In (5.2), we first replace ki by -kil for

1,.

,r,

and then

simultaneously replace a,

z and xi, by bz, az, l/z and l/xi1 for

1,.

respectively.

Generalizations of Jacobi's triple product identity

395

We have given analytical convergence conditions for the identities

(5.2) and (5.4). However, as we already observed in Section 17.4 when

dealing with the respective one variable cases, these identities also hold

when regarded as identities for

formal power series

over

We complete this section with an Abel-Rothe type generalization of

the Macdonald identities for the affine root system A,, as a direct con-

sequence of Theorem 5.2.

If we multiply both sides of (5.2) by

xi/xj), extract the

l<i<j<r

coefficient of zM, using the Jacobi triple product identity (2.10) on the

left hand side and (4.7) on the right hand side, and divide the resulting

identity by (-l)~q(y), we obtain

We use the Vandermonde determinant expansion (0.2) and a little bit of

algebra and obtain

0 and

0, the terms of the sum in (5.6) are zero unless

Ikl

Specializing this further by setting

0 gives an identity

which has been shown to be equivalent to the Macdonald identities for

the affine root system

A,,

see Milne (Milne, 1985). Regarding this, we

may consider the identity (5.6)

an Abel-Rothe type generalization of

the Macdonald identities for the affine root system A,.

396

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Concluding, we want to point out that we could have given an even

more general multidimensional Abel-Rothe type generalization of Ja-

cobi's triple product identity than Theorem 5.2, by multilateralizing

Theorem 3.9 of (Schlosser, 2000) instead of Theorem

6.11

of (Schlosser,

1999) as above. However, we feel that, because of the more complicated

factors being involved, the result would be not as elegant

Theorem

5.2 which is sufficiently illustrative. We therefore decided to refrain from

giving this more general identity.

Appendix: Convergence of multiple series

Here we prove the conditions of convergence of our multiple series identity in

Theorem 5.2 (and thus also of Theorem 5.3).

We determine the condition for absolute convergence of the multilateral series in

(5.2) by splitting the entire sum into two sums,

and

and show

kl,

...,

k,=-oo

lkllo Ikl<o

the absolute convergence for each of these separately.

We first consider the sum

We obtain that for

(kl

0 the sum in (5.2)

IkllO

converges absolutely provided

We use the Vandermonde determinant expansion

where

denotes the symmetric group of order r, interchange summations in (0.1)

and obtain r! multiple sums each corresponding to a permutation

S,.

Thus for

Ikl

0 the series in (5.2) converges provided

for any

The next step is crucial and typically applies in the theory of multidimensional

basic hypergeometric series over the root system

A,-1

for a class of series. (For

instance, it applies to several of the multilateral summations and transformations in

(Milne and Schlosser, 2002).) In the summand of (0.3), we have

398

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

or equivalently, whenever

lazl

The absolute convergence of the sum

is established in a similar manner. In

lk1<0

this case we obtain that for

the sum in

(5.2)

converges absolutely provided

The further analysis is as follows. We use

(0.2)

and

(0.4)

and assume that for

Ikl

without loss of generality,

In a very similar analysis to above one easily finds

the condition

Jbl

for absolute convergence.

Remark

0.4.

In an earlier version of this article we had given a smaller region of

convergence for the series in (5.2). In particular, instead of

we had given the condition

To see that this gives a smaller region of convergence (for r

1)) assume we would

have instead max(lazl,lb1)

1. NOW take the '$roduct7' of the whole relation (0.8)

over all

. . .

r. This gives lazlT

lql(;)

Iqr(T-l)b-r

or equivalently, after

taking r-th roots, lazl

lql

lqr-lb-l

which apparently contradicts max(laz1, Ibl)

1 since r

The same argument which leads to the convergence condition in Theorem 5.2 (in

contrast to the condition (0.8)) can be used to improve some analogous results given

in the literature. In particular, this concerns the papers (Milne, 1997), (Milne and

Schlosser, 2002), (Schlosser, 1999), (Schlosser, 2000) (and possibly others).

Notes

1. The Merriam-Webster Online dictionary (http

//www

m-w

com/cgi-bin/dictionarf

gives for the entry

'-be':

". .

(1): cause to be

. . .

(2):

cause to be formed into

. .

become

. .

.usage The suffix -ize has been productive in English since the time of Thomas

Nashe (1567-1601), who claimed credit for introducing it into English to remedy the surplus

of monosyllabic words.

Almost any noun or adjective can be made into a verb by adding

-be <hospitalize> <familiarize>; many technical terms are coined this way <oxidize> as

well as verbs of ethnic derivation <Americanize> and verbs derived from proper names

<bowdlerize> <mesmerize>. Nashe noted in 1591 that his coinages in -be were being

complained about, and to this day new words in -ize <finalize> <prioritize> are sure to

draw critical fire."

References

Abel,

(1826).

Beweis eines Ausdrucks, von welchem die Binomial-Formel ein

einzelner Fall ist.

Reine Angew. Math., 1:159-160.

Generalizations of Jacobi's triple product identity

399

Andrews, G.

(1974). Applications of basic hypergeometric functions. SIAM Rev.,

16:441-484.

Bailey,

N. (1936). Series of hypergeometric type which are infinite in both direc-

tions. Quart.

Math. Oxford Series, 7:105-115.

Bailey,

N. (1950). On the basic bilateral hypergeometric series

242.

Quart.

Math. Oxford Series

1:194-198.

Bhatnagar, G. and Milne, S. C. (1997). Generalized bibasic hypergeometric series and

their

u(n)

extensions. Adv. Math., 131:188-252.

Bhatnagar, G. and Schlosser, M. (1998).

C,,

and

very-well-poised

lo(p9

transfor-

mations. Constr. Approx., 14:531-567.

Bressoud, D. M. (1999). Proofs and Confirmations. Cambridge University Press, Cam-

bridge.

Bromwich, T.

(1949). An Introduction to the Theory of Infinite Series. Macmillan,

London, second edition.

Cauchy, A.-L. (1843). MBmoire sur les fonctions dont plusieurs valeurs

. . . .

C.R. Acad.

Sci. Paris, 17:523. Reprinted in Oeuvres de Cauchy, Ser. 1, vol. 8, Gauthier-Villars,

Paris (1893), 42-50.

Euler, L. (1779). De serie Lambertiana plurimisque eius insignibus proprietatibus.

Acta Acad. Sci. Petro. I1 (1783), 29-51; reprinted in Opera Omnia, Ser.

vol. 6,

Teubner, Leibzig (1921), 350-369.

Gasper, G. and Rahman, M. (1990). Basic Hypergeometric Series, volume 35 of Ency-

clopedia of Mathematics and its Applications. Cambridge University Press, Cam-

bridge.

Gould, H.

(1956). Some generalizations of Vandermonde's convolution. Amer.

Math. Monthly, 63234-91.

Gould, H.

(1957). Final analysis of Vandermonde's convolution. Amer. Math.

Monthly, 64:409-415.

Hahn,

(1949). Beitrage zur Theorie der Heineschen Reihen. Die hypergeometrische

q-Differenzengleichung. Das q-Analogon der Laplace-Transformation. Math. Nachr.,

2:340-379.

Hardy, G. H. (1940). Ramanujan. Cambridge University Press, Cambridge. Reprinted

by: Chelsea, New York, 1978.

Ismail, M. E. H. (1977). A simple proof of Ramanujan's

141

sum. PTOC. Amer. Math.

Soc., 63:185-186.

Jackson, F. H. (1910). A q-generalization of Abel's series. Rend. Circ. Math. Palermo,

29:340-346.

Jackson, M. (1950). On Lerch's transcendant and the basic bilateral hypergeometric

series

242.

London Math. Soc., 25:189-196.

Jacobi, C.

(1829). Fundamenta Nova Theoriae hnctionum Ellipticarum. Un-

known. Reprinted in Jacobi's Gesammelte Werke, vol. 1, (Reimer, Berlin, 1881-

1891), pp. 49-239; reprinted by Chelsea (New York, 1969); now distributed by the

Amer. Math. Soc., Providence,

RI.

Johnson,

P. (1996). q-Extensions of identities of Abel-Rothe type. Discrete Math.,

159:161-177.

Krattenthaler, C. and Schlosser,

(1999).

new multidimensional matrix inverse

with applications to multiple q-series. Discrete Math., 204:249-279.

Lambert,

H. (1758). Observationes variae in mathesin puram. Acta Helvetzca, 3:128-

168.

400

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Milne, S. C.

(1985).

An elementary proof of the Macdonald identities for

A,(,,

Adv.

Math.,

57:34-70.

Milne, S. C.

(1997).

Balanced

3(pz

summation theorems for U(n) basic hypergeometric

series. Adv. Math.,

131:93-187.

Milne, S. C. and Schlosser, M.

(2002).

new A, extension of Ramanujan's

summation with applications to multilateral

series. Rocky Mount. J. Math.,

32(2):759-792.

Pdya, G. and Szegti, G.

(1925).

Aufgaben und Lehrsatze aus der Analysis, Band

Julius Springer, Berlin.

Rosengren,

(2003).

Reduction formulae for Karlsson-Minton type hypergeometric

functions. Constr. Approz. To appear.

Rothe,

(1793).

Formulae de serierum reversione demonstratio universalis signis

localibus

combinatorico-analyticorum

vicariis exhibita. Leipzig.

Schlosser, M.

(1997).

Multidimensional matrix inversions and

and

basic

hy-

pergeometric series. Ramanujan J.,

1:243-274.

Schlosser, M.

(1999).

Some new applications of matrix inversions in

A,.

Ramanujan

J.,

3:405-461.

Schlosser, M.

(2000).

A new multidimensional matrix inversion in

A,.

In q-series from

a contemporary perspective (South Hadley, MA, 1998), volume

254

of Contemp.

Math., pages

413-432.

Amer. Math. Soc., Providence,

RI.

Slater, L.

(1966).

Generalized Hypergeometric Functions. Cambridge Univ. Press,

LondonINew York.

Strehl,

(1992).

Identities of

Rothe-Abel-Schlafli-Hurwitz-type.

Discrete Math.,

99:321-

340.

Weil,

(1976).

Elliptic functions according to Eisenstein and Kronecker. Springer-

Verlag, Berlin. Ergebnisse der Mathematik und ihrer Grenzgebiete, Band

88.

SUMMABLE SUMS

HYPERGEOMETRIC SERIES*

Stanton

School of Mathematics

University of Minnesota

Minneapolis, MN

55455

stanton@rnath.urnn.edu

Abstract

New expansions for certain ~Fl's

a sum of

higher order hypergeo-

metric series are given. When specialized to the binomial theorem, these

hypergeometric series sum. The results represent cubic and higher or-

der transformations, and only Vandermonde's theorem is necessary for

the elementary proof. Some q-analogues are also given.

Introduction

hypergeometric series may always be written as a sum of two other

hypergeometric series by splitting the series into its even and odd terms,

for example

(~)2k+l z2k+1

k=O

(2k

l)!

One may also write a series as a sum of

series by reorganizing the

terms modulo

In this paper

variation of this idea is considered, where the terms

modulo

differ by a power of a linear function. We give in 52 four exam-

ples of this phenomena, Theorems 2.2, 2.5, 2.8, and 2.11, which may be

considered

rth-degree transformations. Special cases of these expan-

sions give new expansions for

x)-":

four cubic expansions are ex-

plicitly given as Corollaries 2.3, 2.6, 2.9, and 2.12 in 52. q-analogues may

also be given, we state two such in

$4:

Theorems 2.2q and 2.5q. Thus

these results are in the same spirit

those of Mizan Rahman in (Rah-

man, 1989; Rahman, 1993; Rahman, 1997), and his work with George

*Partially

supported

NSF

grant

DMS

0203282.

2005

Springer Science+Business Media, Inc.

402

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Gasper (Gasper and Rahman, 1990; Gasper and Rahman, 1990b), al-

though these are at a much lower level. They are motivated by Corollary

4.4

for

2, which was the key lemma in (Prellberg and Stanton, 2003).

Main

Results

In this section we state and prove the main results of this paper,

Theorems 2.2, 2.5, 2.8, and 2.11.

One may expand a formal power series F(x) in x as

formal power

series in

x(1

x)-'Ir

The coefficients ak may be found from the Lagrange inversion formula.

is a positive integer, then this series may be rewritten as

We shall consider variations of (2.1) where the denominator exponent

is either

instead of

ilr.

We shall find expansions for the

function

(4k

c-x

2F1

1x).

k=O

(PI

First we consider what happens if all of the denominator exponents

in (2.1) are

Proposition

2.1.

Let

F(x)

FsxS

formal power series in

s=o

and let r

be an integer. If

then

Proof.

Considering the coefficient of xrtfi, 0

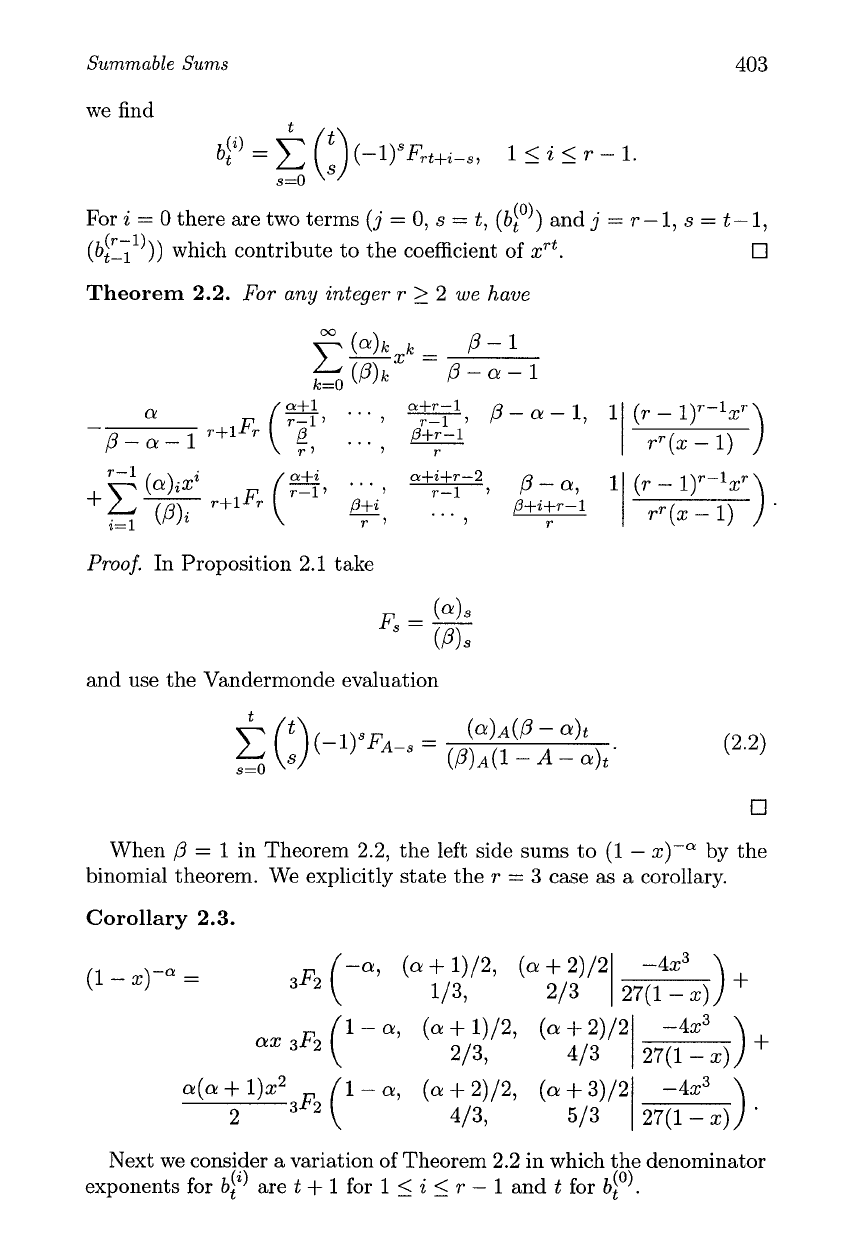
Summable Sums

we find

(0)

Fori=Otherearetwoterms(j=O,s=t,(bt

)andj=r-l,s=t-1,

(bcil))) which contribute to the coefficient of

xTt.

Theorem

2.2.

For

any integer

have

Proof.

In Proposition 2.1 take

and use the Vandermonde evaluation

When

in Theorem 2.2, the left side sums to (1

x)-" by

binomial theorem. We explicitly state the

case as a corollary.

Corollary

2.3.

the

Next we consider a variation of Theorem 2.2 in which the denominator

exponents for

bi"

are

for

and t for 6:').