Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook
Подождите немного. Документ загружается.


E
1133
¼ E
2233
¼ 2p
Z
1
0
g
2
x
2
f½ðd þ eÞð1 x
2
Þþ2f g
2
x
2
½f ð1 x
2
Þþhg
2
x
2
g
2
g
2
x
2
ð1 x
2
Þgdx
E
3311
¼ E
3322
¼ 2p
Z
1
0
ð1 x
2
Þ½dð1 x
2
Þþf g
2
x
2
½eð1 x
2
Þþf g
2
x
2
dx
E
1212
¼
p
2
Z
1
0
ð1 x
2
Þ
2
fg
2
g
2
x
2
ðd eÞ½f ð1 x
2
Þþhg
2
x
2
gdx
E
1313
¼ E
2323
¼2p
Z
1
0
gg
2
x
2
ð1 x
2
Þ½eð1 x
2
Þþf g
2
x
2
dx
where
1
¼½eð1 x
2
Þþf g
2
x
2
f½dð1 x
2
Þþf g
2
x
2
½f ð1 x
2
Þþhg
2
x
2
g
2
g
2
x
2
ð1 x
2
Þg
d ¼ C
ð0Þ
11
e ¼½C
ð0Þ
11
C
ð0Þ
12
=2
f ¼ C
ð0Þ
44
g ¼ C
ð0Þ
13
þ C
ð0Þ
44
h ¼ C
ð0Þ
33
and x is the integration variable while g ¼a is the inclusion aspect ratio.
Using these equations, the tensor E
pqrs
(and hence G
pqrs
) can be obtained by
numerical or symbolic integration. For numerical computations, instead of the more
common Voigt notation, the Kelvin notation of fourth-rank tensors in terms of 6 6
matrices is more efficient, since matrix operations can be performed on the Kelvin
6 6 matrices according to the usual matrix rules (see also Section 2.2).
In the equation for the effective elastic stiffness, the term X involving the tensor
G
d
(rs)
represents the two-point interaction between the rth set and the sth set of
inclusions. The tensor G
d
(rs)
is computed in the same way as the tensor G
ijkl
(r)
except
that the aspect ratio of the associated inclusion is set to be the aspect ratio, a
d
,of
the ellipsoidal two-point spatial correlation function, representing the symmetry of
p
(s|r)
(xx
0
), the conditional probability of finding an inclusion of type s at x
0
, given
that there is an inclusion of type r at x. The aspect ratio of the spatial correlation
function can be different from the aspect ratio of the inclusions. The effect of spatial
distribution is of higher order in the volume concentrations than the effect of
inclusion shapes. The effect of spatial distribution becomes important when the
inclusion concentration increases beyond the dilute limit. However, even the T-matrix
approximation given by equation (4.12.1) may be invalid at very high concentration,
as it neglects the higher-order interactions beyond two-point interactions (Jakobsen
et al., 2003a). According to Jakobsen et al. (2003a) it appears to be difficult to
determine the range of validity for the various T-matrix approximations. For the case
207 4.12 T-matrix inclusion models for effective moduli

of a matrix phase with just one type of inclusion, Ponte-Castaneda and Willis (1995)
found that the T-matrix approximation is consistent only for certain combinations of
a
d
, (aspect ratio of the correlation function), a (inclusion aspect ratio), and n (inclu-
sion concentration). When a
d
> a, the minimum possible value for inclusion aspect
ratio is a
min
¼a
d
n.
Using a series expansion of the reciprocal, the equation for the effective stiffness
may be written as
C
T
¼ C
ð0Þ
þ
X
r
v
ðrÞ
t
ðr Þ
X
r
X
s
n
ðr Þ
t
ðrÞ
G
ðrsÞ
d
t
ðsÞ
n
ðsÞ
þ Ov
ðrÞ
3
which agrees with Eshelby’s (1957) dilute estimate to first order in concentration.
When the spatial distribution is assumed to be the same for all pairs of inter-
acting inclusions, G
ðrsÞ
d
¼ G
d
for all r and s. Under this assumption the equation for
C
T
becomes
C
T
¼ C
ð0Þ
þ
X
r
v
ðr Þ
t
ðrÞ
"#
I þ G
d
X
s
t
ðsÞ
n
ðsÞ
"#()
1
or
C
T
¼ C
ð0Þ
þ
X
r
v
ðrÞ
t
ðr Þ
!
X
r
v
ðrÞ
t
ðrÞ
!
G
d
X
s
v
ðsÞ
t
ðsÞ
!
þ O n
ðrÞ
3
These non-self-consistent, second-order T-matrix approximations with a well-defined
C
(0)
are sometimes termed the generalized optical potential approximation (OPA)
(Jakobsen et al., 2003a). The generalized OPA equations reduce to Nishizawa’s
(1982) differential effective medium formulation when the second-order correction
terms are dropped, and the calculations are done incrementally, increasing the inclusion
concentration in small steps, and taking C
(0)
to be the effective stiffness from the
previous incremental step. This formulation can be written as a system of ordinary
differential equations for the effective stiffness C
DEM
(Jakobsen et al., 2000):
1 n
ðrÞ
hi
dC
DEM
dn
ðrÞ
¼ C
ðrÞ
C
DEM
hi
I G
ðDEMÞ
ðC
ðr Þ
C
DEM
Þ
hi
1
with initial conditions
C
DEM
ðn
ðrÞ
¼ 0Þ¼C
ð0Þ
The superscript (DEM) on the tensor G indicates that it is computed for a medium
having elastic stiffness C
DEM
.
When the multiphase aggregate does not have a clearly defined matrix material and
each phase is in the form of inclusions embedded in a sea of inclusions, one can set
208 Effective elastic media: bounds and mixing laws

C
ð0Þ
¼ C
T
to give the self-consistent T-matrix approximation, also called the gener-
alized coherent potential approximation (CPA):
X
r
v
ðr Þ
t
ðrÞ
"#
I þ
X
s
v
ðsÞ
t
ðsÞ
"#
1
X
p
X
q
n
ðpÞ
t
ðpÞ
G
ðpqÞ
d
n
ðqÞ
t
ðqÞ
8
<
:
9
=
;
1
¼ 0
or
X
r
v
ðr Þ
t
ðrÞ
"#
X
p
X
q
n
ðpÞ
t
ðpÞ
G
ðpqÞ
d
n
ðqÞ
t
ðqÞ
0
where the subscript
*
on the tensor quantities t and G indicates that they are
computed for a reference medium having the (as yet unknown) elastic stiffness C
T
.
The generalized CPA equations are an implicit set of equations for the effective
stiffness, and must be solved iteratively. The generalized CPA reduces to the first-
order CPA, ignoring higher-order terms:
X
r
v
ðrÞ
t
ðr Þ
0
which is equivalent to Berryman’s symmetric self-consistent approximation (see
Section 4.8).
When the rth set of inclusions have a spatial distribution described by an orienta-
tion distribution function O
ðr Þ
ðy; ;Þ, the T-matrix for the individual inclusion, t
ðr Þ
,
in the above equations for the first- and second-order corrections have to be replaced
by the orientation averaged tensor
t
ðrÞ
given by
t
ðrÞ
¼
Z
p
0
dy sin y
Z
2p
0
d
Z
2p
0
d O
ðrÞ
ðy; ;Þt
ðr Þ
ðy; ;Þ
The three Euler angles ðy; ;Þ define the orientation of the ellipsoid with principal
axes X
1
X
2
X
3
with respect to the fixed global coordinates x
1
x
2
x
3
, where y is the angle
between the short axis of the ellipsoid and the x
3
-axis. Computing the orientation
average T-matrix involves coordinate transformation of the inclusion stiffness tensor
C
ðr Þ
from the local coordinate system of the inclusion to the global coordinates using
the usual transformation laws for the stiffness tensor.
Jakobsen et al. (2003b) have extended the T-matrix formulation to take into
account fluid effects in porous rocks. For a single fully saturated ellipsoidal inclusion
of the rth set that is allowed to communicate and exchange fluid mass with other
cavities, the T-matrix, t
ðr Þ
sat
is given as the dry T-matrix t
ðr Þ
dry
plus an extra term
accounting for fluid effects. The T-matrix for dry cavities is obtained by setting
C
ðr Þ
to zero in the above equations. The saturated T-matrix is expressed as
t
ðrÞ
sat
¼ t
ðrÞ
dry
þ
Z
ðrÞ
þ iok
f
X
ðrÞ
1 þ i og
ðr Þ
209 4.12 T-matrix inclusion models for effective moduli

X
ðrÞ
¼ t
ðrÞ
dry
S
ð0Þ
ðI
2
I
2
ÞS
ð0Þ
t
ðrÞ
dry
Z
ðrÞ
¼ t
ðrÞ
dry
S
ð0Þ
ðI
2
I
2
ÞS
ð0Þ
X
r
n
ðrÞ
t
ðrÞ
dry
1 þ iog
ðrÞ
!
¼ k
f
1 k
f
S
ð0Þ
uuvv
X
r
n
ðrÞ
1 þ iog
ðrÞ
!
þ k
f
X
r
n
ðrÞ
K
ðrÞ
d
uuvv
1 þ iog
ðrÞ
0
@
1
A
ik
u
k
v
uv
k
f
o
f
8
<
:
9
=
;
1
K
ðrÞ
d
¼ I
4
þ G
ðrÞ
C
ð0Þ
1
S
ð0Þ
g
ðrÞ
¼ 1 þ k
f
K
ðrÞ
d
S
ð0Þ
uuvv
In the above equations, S
ð0Þ
¼ C
ð0Þ
1
is the elastic compliance of the background
medium, k
f
and
f
are the bulk modulus and viscosity of the fluid, o is the frequency,
t is a flow-related relaxation-time constant, k
u
, k
n
are components of the wave vector,
and G
uv
is the (possibly anisotropic) permeability tensor of the rock. Repeated
subscripts u and v imply summation over u, v ¼1, 2, 3. The tensors I
2
and I
4
are
second- and fourth-rank identity tensors, respectively. The dyadic product is denoted
by and I
2
I
2
ðÞ
ijkl
¼ d
ij
d
kl
. Usually the relaxation-time constant t is poorly
defined and has to be calibrated from experimental data. Jakobsen et al. (2003b)
show that the first-order approximations of these expressions reduce to the Brown and
Korringa results (see Section 6.5) in the low-frequency limit.
Uses
The results described in this section can be used to model effective-medium elastic
properties of composites made up of anisotropic ellipsoidal inclusions in an aniso-
tropic background. The formulation for saturated, communicating cavities can be
used to model fluid-related velocity dispersion and attenuation in porous rocks.
Assumptions and limitations
The results described in this section assume the following:
idealized ellipsoidal inclusion shape;
linear elasticity and a Newtonian fluid.
4.13 Elastic constants in finely layered media: Backus average
Synopsis
A transversely isotropic medium with the symmetry axis in the x
3
-direction has
an elastic stiffness tensor that can be written in the condensed Voigt matrix form
(see Section 2.2 on anisotropy):
210 Effective elastic media: bounds and mixing laws

abf 000
baf 000
ffc000
000d 00
0000d 0
00000m
2
6
6
6
6
6
6
4
3
7
7
7
7
7
7
5
; m ¼
1
2
ða bÞ
where a, b, c, d, and f are five independent elastic constants. Backus (1962) showed
that in the long-wavelength limit a stratified medium composed of layers of trans-
versely isotropic materials (each with its symmetry axis normal to the strata) is also
effectively anisotropic, with effective stiffness as follows:
ABF 000
BAF 000
FFC000
000D 00
0000D 0
00000M
2
6
6
6
6
6
6
4
3
7
7
7
7
7
7
5
; M ¼
1
2
ðA BÞ
where
A ¼ a f
2
c
1
þ c
1
1
fc
1
2
B ¼ b f
2
c
1
þ c
1
1
fc
1
2
C ¼ c
1
1
F ¼ c
1
1
fc
1
D ¼ d
1
1
M ¼ m
hi
The brackets
hi
indicate averages of the enclosed properties weighted by their
volumetric proportions. This is often called the Backus average.
If the individual layers are isotropic, the effective medium is still transversely
isotropic, but the number of independent constants needed to describe each individual
layer is reduced to 2:
a ¼ c ¼ l þ 2m; b ¼ f ¼ l; d ¼ m ¼ m
giving for the effective medium
A ¼
4mðl þ m Þ
l þ 2m
þ
1
l þ 2m
1
l
l þ 2m
2
B ¼
2ml
l þ 2m
þ
1
l þ 2m
1
l
l þ 2m
2
211 4.13 Backus average
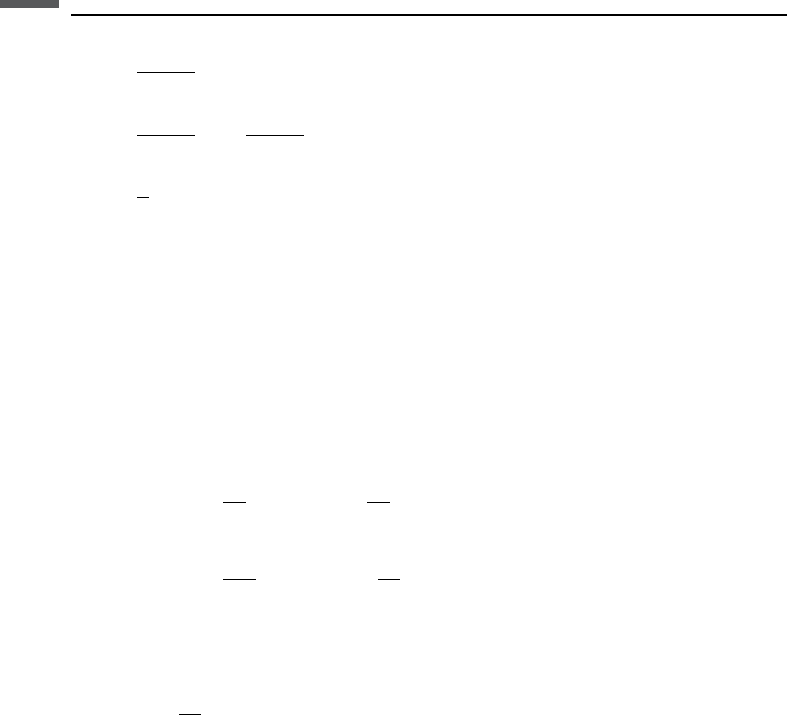
C ¼
1
l þ 2m
1
F ¼
1
l þ 2m
1
l
l þ 2m
D ¼
1
m
1
M ¼ m
hi
In terms of the P- and S-wave velocities and densities in the isotropic layers (Levin, 1979),
a ¼ V
2
P
d ¼ V
2
S
f ¼ ðV
2
P
2V
2
S
Þ
the effective parameters can be rewritten as
A ¼ 4V
2
S
1
V
2
S
V
2
P
þ 1 2
V
2
S
V
2
P
2
V
2
P
1
DE
1
B ¼ 2V
2
S
1
2V
2
S
V
2
P
þ 1 2
V
2
S
V
2
P
2
V
2
P
1
DE
1
C ¼ V
2
P
1
DE
1
F ¼ 1 2
V
2
S
V
2
P
ðV
2
P
Þ
1
DE
1
D ¼ V
2
S
1
DE
1
M ¼ V
2
S
The P- and S-wave velocities in the effective anisotropic medium can be written as
V
SH;h
¼
ffiffiffiffiffiffiffiffiffi
M=
p
V
SH;v
¼ V
SV;h
¼ V
SV;v
¼
ffiffiffiffiffiffiffiffiffi
D=
p
V
P;h
¼
ffiffiffiffiffiffiffiffi
A=
p
V
P;v
¼
ffiffiffiffiffiffiffiffiffi
C=
p
where r is the average density; V
P,v
is for the vertically propagating P-wave; V
P,h
is
for the horizontally propagating P-wave; V
SH,h
is for the horizontally propagating,
horizontally polarized S-wave; V
SV,h
is for the horizontally propagating, vertically
polarized S-wave; and V
SV,v
and V
SH,v
are for the vertically propagating S-waves of
any polarization (vertical is defined as being normal to the layering).
212 Effective elastic media: bounds and mixing laws
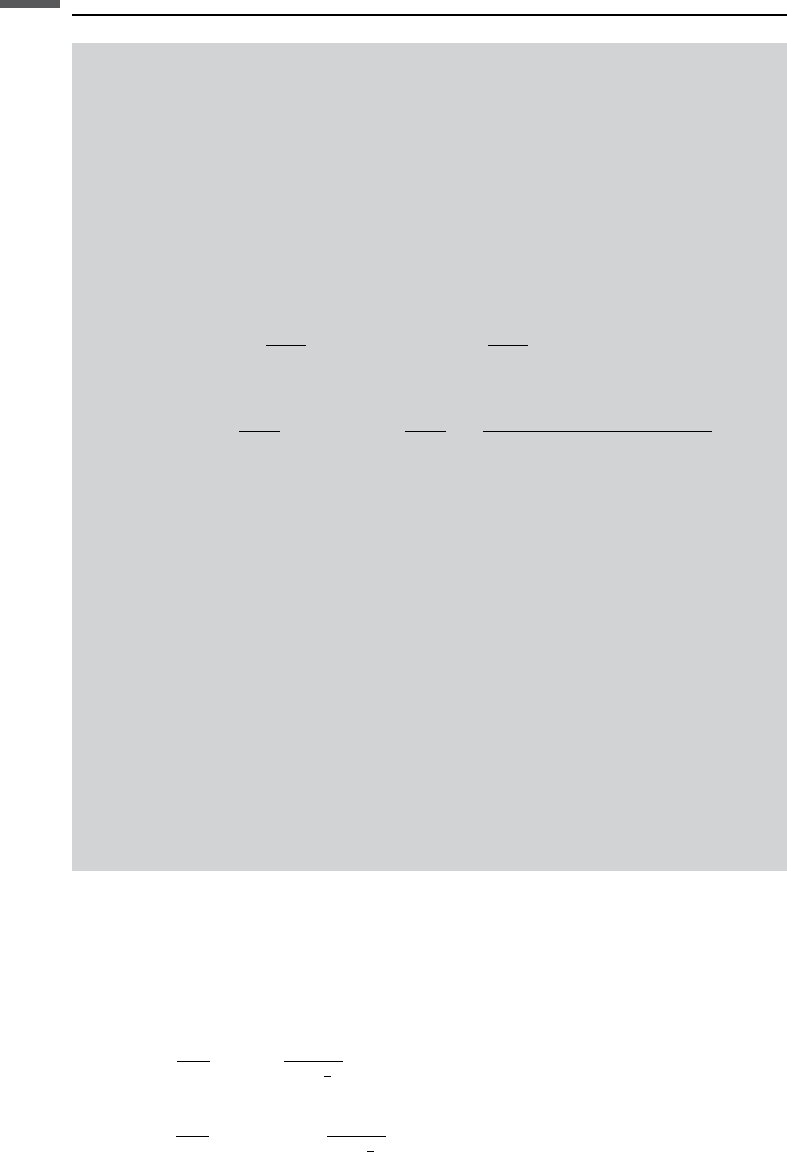
Calculate the effective anisotropic elastic constants and the velocity anisotropy for
a thinly layered sequence of dolomite and shale with the following layer properties:
V
Pð1Þ
¼ 5200 m=s; V
Sð1Þ
¼ 2700 m=s;
1
¼ 2450 kg=m
3
; d
1
¼ 0:75 m
V
Pð2Þ
¼ 2900 m=s; V
Sð2Þ
¼ 1400 m=s;
2
¼ 2340 kg=m
3
; d
2
¼ 0:5m
The volumetric fractions are
f
1
¼ d
1
=ðd
1
þ d
2
Þ¼0:6; f
2
¼ d
2
=ðd
1
þ d
2
Þ¼0:4
If one takes the volumetric weighted averages of the appropriate properties,
A ¼ f
1
4
1
V
2
Sð1Þ
1
V
2
Sð1Þ
V
2
Pð1Þ
!
þ f
2
4
2
V
2
Sð2Þ
1
V
2
Sð2Þ
V
2
Pð2Þ
!
þ f
1
1 2
V
2
Sð1Þ
V
2
Pð1Þ
!
þ f
2
1 2
V
2
Sð2Þ
V
2
Pð2Þ
!"#
2
1
f
1
=ð
1
V
2
Pð1Þ
Þþf
2
=ð
2
V
2
Pð2Þ
Þ
A ¼ 45:1 GPa
Similarly, computing the other averages we obtain C ¼34.03 GPa, D ¼8.28 GPa,
M ¼12.55 GPa, F ¼16.7 GPa, and B ¼A –2M ¼20 GPa.
The average density r ¼ f
1
r
1
þf
2
r
2
¼ 2406 kg/m
3
. The anisotropic velocities are:
V
SH;h
¼
ffiffiffiffiffiffiffiffiffi
M=
p
¼ 2284:0m=s
V
SH;v
¼ V
SV;h
¼ V
SV;v
¼
ffiffiffiffiffiffiffiffiffi
D=
p
¼ 1854:8m=s
V
P;h
¼
ffiffiffiffiffiffiffiffi
A=
p
¼ 4329:5m=s
V
P;v
¼
ffiffiffiffiffiffiffiffiffi
C=
p
¼ 3761:0m=s
P-wave anisotropy ¼(4329.5 – 3761.0)/3761.0 15%
S-wave anisotropy ¼(2284.0 – 1854.8)/1854.8 23%
Finally, consider the case in which each layer is isotropic with the same shear
modulus but with a different bulk modulus. This might be the situation, for example,
for a massive, homogeneous rock with fine layers of different fluids or saturations.
Then, the elastic constants of the medium become
A ¼ C ¼
1
V
2
P
1
¼
1
K þ
4
3
m
*+
1
B ¼ F ¼
1
V
2
P
1
2m ¼
1
K þ
4
3
m
*+
1
2m ¼ A 2m
D ¼ M ¼ m
213 4.13 Backus average

A finely layered medium of isotropic layers, all having the same shear modulus, is
isotropic.
Bakulin and Grechka (2003) show that any effective property, m
eff
, of a finely
layered medium (in fact, any heterogeneous medium) can be written as
Fm
eff
¼
1
V
Z
V
Fm xðÞ½dx
where F is the appropriate averaging operator, m is the parameter (tensor, vector, or
scalar) of interest (e.g., the elastic stiffnesses, c
ij
, or Thomsen parameters, e, d, g), V is
the representative volume, and x denotes the Cartesian coordinates over which
m varies. For example, from the Backus average one can write
c
eff
66
¼
1
V
Z
V
c
66
xðÞdx
where F is the scalar identity function,
c
eff
44
¼
1
V
Z
V
c
44
xðÞ½
1
dx
1
where Fm½¼m
1
, and so on. An important consequence is that
m
eff
¼ m þ O
~
m
2
where
m ¼
1
V
Z
V
mxðÞdx
~
m ¼ m
m
In other words, the effective property of a finely layered medium is, to first order, just
the arithmetic average of the individual layer properties. The effect of the layer
contrasts is second order in the deviations from the mean.
A composite of fine isotropic layers with weak layer contrasts is, to first order,
isotropic (Bakulin, 2003). The well-known anisotropy resulting from the Backus
average is second order. When the layers are intrinsically anisotropic, as with shales,
then the first-order anisotropy is the average of the layer intrinsic anisotropies, and
the anisotropy due to the layer contrasts is often less important, especially when the
layer contrasts are small.
Uses
The Backus average is used to model a finely stratified medium as a single homo-
geneous medium.
214 Effective elastic media: bounds and mixing laws
Assumptions and limitations
The following presuppositions and conditions apply to the Backus average:
all materials are linearly elastic;
there are no sources of intrinsic energy dissipation, such as friction or viscosity; and
the layer thickness must be much smaller than the seismic wavelength. How small
is still a subject of disagreement and research, but a rule of thumb is that the
wavelength must be at least ten times the layer thickness.
4.14 Elastic constants in finely layered media:
general layer anisotropy
Techniques for calculation of the effective elastic moduli of a finely layered one-
dimensional medium were first developed by Riznichenko (1949) and Postma (1955).
Backus (1962) further developed the method and introduced the well-known Backus
average of isotropic or VTI layers (Section 4.13). Helbig and Schoenberg (1987) and
Schoenberg and Muir (1989) presented the results for layers with arbitrary anisotropy
in convenient matrix form, shown here. Assuming that the x
3
-axis is normal to the
layering, one can define the matrices
C
ðiÞ
NN
¼
c
ðiÞ
33
c
ðiÞ
34
c
ðiÞ
35
c
ðiÞ
34
c
ðiÞ
44
c
ðiÞ
45
c
ðiÞ
35
c
ðiÞ
45
c
ðiÞ
55
2
6
6
6
4
3
7
7
7
5
C
ðiÞ
TN
¼
c
ðiÞ
13
c
ðiÞ
14
c
ðiÞ
15
c
ðiÞ
23
c
ðiÞ
24
c
ðiÞ
25
c
ðiÞ
36
c
ðiÞ
46
c
ðiÞ
56
2
6
6
6
4
3
7
7
7
5
C
ðiÞ
TT
¼
c
ðiÞ
11
c
ðiÞ
12
c
ðiÞ
16
c
ðiÞ
12
c
ðiÞ
22
c
ðiÞ
26
c
ðiÞ
16
c
ðiÞ
26
c
ðiÞ
66
2
6
6
6
4
3
7
7
7
5
where the c
ðiÞ
kl
are the Voigt-notation elastic constants of the ith layer. Then the
effective medium having the same stress–strain behavior of the composite has the
corresponding matrices
C
NN
¼
c
33
c
34
c
35
c
34
c
44
c
45
c
35
c
45
c
55
2
6
4
3
7
5
¼ C
1
NN
1
215 4.14 General layer anisotropy

C
TN
¼
c
13
c
14
c
15
c
23
c
24
c
25
c
36
c
46
c
56
2
6
4
3
7
5
¼ C
TN
C
1
NN
C
NN
C
TT
¼
c
11
c
12
c
16
c
12
c
22
c
26
c
16
c
26
c
66
2
6
4
3
7
5
¼ C
TT
hi
C
TN
C
1
NN
C
NT
þ C
TN
C
1
NN
C
NN
C
1
NN
C
NT
where the
c
kl
terms are the effective elastic constants. In the above equations the
operator hidenotes a thickness-weighted average over the layers.
4.15 Poroelastic Backus average
Synopsis
For a medium consisting of thin poroelastic layers with different saturating fluids,
Gelinsky and Shapiro (1997a) give the expressions for the poroelastic constants of the
effective homogeneous anisotropic medium. This is equivalent to the Backus average
for thin elastic layers (see Section 4.13). At very low frequencies, (quasi-static limit)
seismic waves cause interlayer flow across the layer boundaries. At higher frequen-
cies, layers behave as if they are sealed with no flow across the boundaries, and this
scenario is equivalent to elastic Backus averaging with saturated rock moduli
obtained from Gassmann’s relations for individual layers.
When the individual poroelastic layers are themselves transversely anisotropic
with a vertical axis of symmetry, x
3
, normal to the layers, each layer is described
by five independent stiffness constants, b
d
, c
d
, f
d
, d
d
, and m
d
, and three additional
poroelastic constants, p, q, and r, describing the coupling between fluid flow and
elastic deformation. The five stiffness constants correspond to the components of the
dry-frame stiffness tensor (see Section 4.13). In the usual two-index Voigt notation they
are: b
d
¼ C
d
12
, c
d
¼ C
d
33
, f
d
¼ C
d
13
, d
d
¼ C
d
44
¼ C
d
55
, where the sub- and superscript “d”
indicates dry frame. The constants p, q,andr for the individual layers can be expressed
in terms of the dry anisotropic frame moduli and fluid properties as follows:
p ¼ a
1
rq¼ a
2
r
r ¼ K
0
1
K
d
K
0
1
K
0
K
f
1
a
1
¼ 1 C
d
11
þ C
d
12
þ C
d
13
= 3K
0
ðÞ
a
2
¼ 1 2C
d
13
þ C
d
33
= 3K
0
ðÞ
K
d
¼
1
9
C
iijj
¼ dry bulk modulus
K
0
¼mineral bulk modulus
K
f
¼pore fluid bulk modulus
f ¼porosity
216 Effective elastic media: bounds and mixing laws
