Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook

Подождите немного. Документ загружается.

obtain the overall correction. Thus, for three crack sets with crack densities e

, e

, and

with crack normals aligned along the 1-, 2-, and 3-axes, respectively, the overall

first-order corrections to c

, c

1ð3setsÞ

may be given in terms of linear combinations of

the corrections for a single set, with the appropriate crack densities as follows (where

we have taken into account the symmetry properties of c

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

1ð3setsÞ

¼ c

ðe

Þþc

ðe

Note that c

¼ 0 and c

¼ c

 2c

¼ c

Hudson (1981) also gives the attenuation coefficient (g ¼oQ

–1

/2V) for elastic

waves in cracked media. For aligned cracks with normals along the 3-axis, the

attenuation coefficients for P-, SV-, and SH-waves are



30p

sin

2y þ BU

 2 sin



30p

ðAU

cos

2y þ BU

sin

2yÞ



30p

ðAU

cos

yÞ

A ¼

B ¼ 2 þ

 10

þ 8

In the preceding expressions, V

and V

are the P and S velocities in the uncracked

isotropic background matrix, o is the angular frequency, and y is the angle between

the direction of propagation and the 3-axis (axis of symmetry).

197 4.10 Hudson’s model for cracked media

For randomly oriented cracks (isotropic distribution), the P and S attenuation

coefficients are given as



BðB  2ÞU





75p



The fourth-power dependence on o is characteristic of Rayleigh scattering.

Hudson (1990) gives results for overall elastic moduli of material with various

distributions of penny-shaped cracks. If conditions at the cracks are taken to be

uniform, so that U

and U

do not depend on the polar and azimuthal angles y and f,

the first-order correction is given as

ijpq

¼

½l

þ 2lmðd

þ d

Þþ4m

ijpq



 AmU

ðd

þ d

 4

ijpq

where

A ¼

p=2

eðy;Þsin y dy d

p=2

eðy;Þn

sin y dy d

ijpq

p=2

eðy;Þn

sin y dy d

and n

are the components of the unit vector along the crack normal, n ¼(sin y cos f,

sin y sin f , cos y), whereas e(y, f) is the crack density distribution function, so that

e(y, f) sin y dy df is the density of cracks with normals lying in the solid angle

between (y, y þdy) and (f, f þdf).

Special cases of crack distributions

(a) Cracks with total crack density e

, which have all their normals aligned along

y ¼y

, f ¼f

eðy;Þ¼e

dðy  y

sin y

dð  

A ¼ e

¼ n

ijpq

¼ n

where n

¼ sin y

cos 

; n

¼ sin y

sin 

; and n

¼ cos y

198 Effective elastic media: bounds and mixing laws

(b) Rotationally symmetric crack distributions with normals symmetrically distributed

about y ¼0, that is, where e is a function of y only:

A ¼ 2p

p=2

eðyÞsin y dy

¼ 0

p=2

eðyÞsin

y dy ¼

ð1 

1111

2222

p=2

eðyÞsin

y dy ¼ 3

1122

¼ 3

1212

; etc:

3333

1111

 4

þ 1

1133



1111

2233

1313

2323

; etc:

Elements other than those related to the preceding elements by symmetry are zero.

A particular rotationally symmetric distribution is the Fisher distribution, for which

e(y)is

eðyÞ¼

ðcos yÞ=



ðe

1=

 1Þ

For small s

, this is approximately a model for a Gaussian distribution on the sphere

eðyÞe

y

=2

2p

The proportion of crack normals outside the range 0  y  2s is approximately 1/e

For this distribution,

A ¼ e

1 þ 2

1=

 2

ðe

1=

 1Þ

2ðe

1=

 1Þ

 

1111

1 þ 4

ð2e

1=

þ 1Þ24

1=

þ 24

ðe

1=

 1Þ

ðe

1=

 1Þ

 3

This distribution is suitable when crack normals are oriented randomly with a small

variance about a mean direction along the 3-axis.

forming a cone. In this case e is independent of f and is zero unless y ¼y

0  f  2p.

eðyÞ¼e

dðy  y

2p sin y

199 4.10 Hudson’s model for cracked media

which gives

A ¼ e

sin

1111

sin

and the first-order corrections are

1111

¼

½U

ð2l

þ 4lm sin

þ 3m

sin

ÞþU

sin

ð4  3 sin

Þ

¼ c

2222

3333

¼

½U

ðl þ 2m cos

þ U

4 cos

sin



1122

¼

½U

ð2l

þ 4lm sin

þ m

sin

ÞU

sin



1133

¼

½U

ðl þ m sin

Þðl þ 2m cos

ÞU

2 sin

cos



¼ c

2233

2323

¼

m½U

4 sin

cos

þ U

ðsin

þ 2 cos

 4 sin

cos

Þ

¼ c

1313

1212

¼

m½U

sin

þ U

sin

ð2  sin

Þ

Heavily faulted structures

Hudson and Liu (1999) provide a model for the effective elastic properties of rocks

with an array of parallel faults based on the averaging process of Schoenberg and

Douma (1988). The individual faults themselves are modeled using two different

approaches: model 1 as a planar distribution of small circular cracks; model 2 as a

planar distribution of small circular welded contacts. Hudson and Liu also give

expressions where both models 1 and 2 are replaced by an equivalent layer of

constant thickness and appropriate infill material, which can then be averaged using

the Backus average. Model 2 (circular contacts) provides expressions for effective

elastic properties of heavily cracked media with cracks aligned and confined within

the fault planes.

Assuming the fault normals to be aligned along the 3-axis, the elements of the

effective stiffness tensor are (Hudson and Liu, 1999)

200 Effective elastic media: bounds and mixing laws

1111

¼ c

2222

¼ l þ 2m

3333

l þ 2m

1 þ E

1122

¼ l

1133

¼ c

2233

1 þ E

2323

¼ c

1313

1 þ E

1212

¼ m

The quantities E

and E

depend on the fault model. For model 1, where the fault

plane consists of an array of circular cracks,



l þ 2m



1 þ p U



3=2

1 

l þ 2m





1 þ



3=2

3  2

l þ 2m



where a is the crack radius, n

is the number density of cracks on the fault surface,

H is the spacing between parallel faults, and l and m are the Lame

parameters of the

uncracked background material. The overall number density of cracks, n ¼ n

=H and

the overall crack density e ¼ na

¼ n

=H. The relative area of cracking on the fault

is given by r ¼ n

Alternatively, the faults may be replaced by an elastically equivalent layer with

bulk and shear moduli given by



¼ K



pam

l þ 2m



1 



1=2



¼ m

3pam

3l þ 4m

l þ 2m



1 



1=2

In the above expressions K

and m

are, respectively, the bulk and shear moduli of

the material filling the cracks, of aspect ratio a, within the faults. Viscous fluid may be

modeled by setting m

to be imaginary. Setting both moduli to zero represents dry

cracks. The equivalent layers can be used in Backus averaging to estimate the overall

stiffness. These give results very close (but not completely identical, because of the

approximations involved) to the results obtained from the equations for c

ijkl

given above.

For model 2, which assumes a planar distribution of small, circular, welded

contacts:

l þ 2mðÞ

4mlþ mðÞ

HbðÞ

1 þ 2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ



1

201 4.10 Hudson’s model for cracked media

3l þ 4mðÞ

8 l þ mðÞ

HbðÞ

1 þ 2

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ



1

where b is the radius of the welded contact region and n

is the number density of

welded contacts on the fault surface.

In this case, the relative area of cracking (noncontact) on the fault is given by

r ¼ 1  n

Again, as an alternate representation, the faults in model 2 may be replaced by an

elastically equivalent layer with bulk and shear moduli given by



¼ K



4m d=bðÞr 1  rðÞ

l þ mðÞ4m  lðÞ

3l þ 4mðÞl þ 2mðÞ

1 þ 2

1  r



1=2



¼ m

8m d=bðÞr 1  rðÞ

l þ m

3l þ 4m



1 þ 2

1  r



1=2

where d is the aperture of the cracked (noncontact) area. Note that d/b is not the

aspect ratio of cracks since b is the radius of the welded regions. The above equations

are valid for small d/b. The equivalent layers can be used in Backus averaging to

estimate the overall stiffness.

The equations for model 1 are valid for r 1 (dilute cracks, predominantly

welded) while the equations for model 2 are valid for (1 r) 1 (dilute contacts,

heavily cracked). Hudson and Liu (1999) show that, to first order, the elastic response

of heavily cracked faults (model 2) is the same as that of a cubic packing of identical

spheres with the same number density and size of contact areas. To the first order, the

actual distribution of contact regions and the shape of cavities between the contact

regions do not affect the overall elastic moduli.

Uses

Hudson’s model is used to estimate the effective elastic moduli and attenuation of a

rock in terms of its constituents and pore space.

Assumptions and limitations

The use of Hudson’s model requires the following considerations:



an idealized crack shape (penny-shaped) with small aspect ratios and either small

crack density or small contact density are assumed. The crack radius and the

distance between cracks are much smaller than a wavelength. The formal limit

quoted by Hudson for both first- and second-order terms is e less than 0.1;



the second-order expansion is not a uniformly converging series and predicts increas-

ing moduli with crack density beyond the formal limit (Cheng, 1993). Better results

will be obtained by using just the first-order correction rather than inappropriately

202 Effective elastic media: bounds and mixing laws

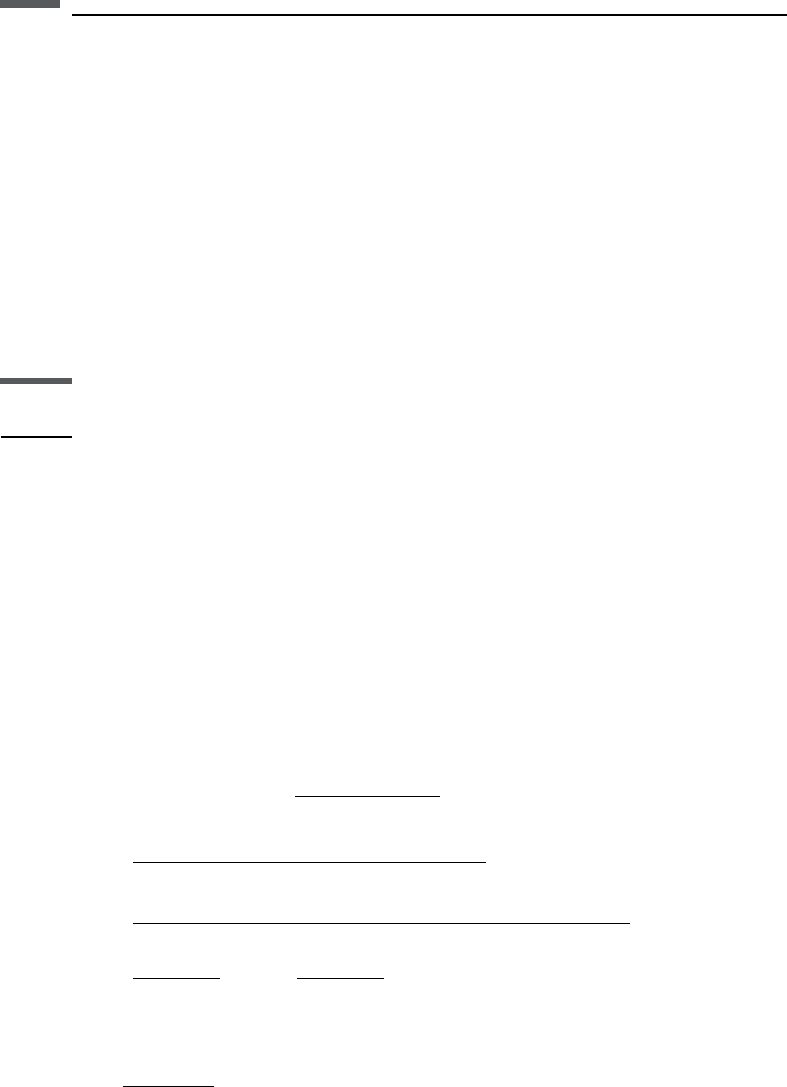
using the second-order correction. Cheng gives a new expansion based on the Pade

approximation, which avoids this problem;



cracks are isolated with respect to fluid flow. Pore pressures are unequilibrated and

adiabatic. The model is appropriate for high-frequency laboratory conditions. For

low-frequency field situations, use Hudson’s dry equations and then saturate by

using the Brown and Korringa relations (Section 6.5). This should not be confused

with the tendency to think of this approach as a low-frequency theory, because

crack dimensions are assumed to be much smaller than a wavelength; and



sometimes a single crack set may not be an adequate representation of crack-

induced anisotropy. In this case we need to superpose several crack sets with

angular distributions.

4.11 Eshelby–Cheng model for cracked anisotropic media

Synopsis

Cheng (1978, 1993) has given a model for the effective moduli of cracked, trans-

versely isotropic rocks based on Eshelby’s (1957) static solution for the strain inside

an ellipsoidal inclusion in an isotropic matrix. The effective moduli c

eff

for a rock

containing fluid-filled ellipsoidal cracks with their normals aligned along the 3-axis

are given as

eff

¼ c

 c

where f is the porosity and c

are the moduli of the uncracked isotropic rock.

The corrections c

are

¼ lðS

 S

þ 1Þþ

2mE

DðS

 S

þ 1Þ

ðl þ 2mÞðS

 S

þ 1Þþ2lS

þ 4mC

ðl þ 2mÞðS

þ S

Þ4mC þ lðS

 S

þ 2Þ

1  2S

1313

1  2S

1212

with

C ¼

3ðK  K

D ¼ S

þ S

 2S

ðS

þ S

 1  3CÞ

 C½S

þ S

þ 2ðS

 S

Þ

203 4.11 Eshelby–Cheng model for cracked anisotropic media

E ¼ S

 S

ðS

þ S

 2C 1ÞþCðS

þ S

 S

¼ QI

þ RI

¼ Q

 2I



þ I

¼ QI

 RI

¼ QI

 RI

¼ QI

 RI

1212

¼ QI

þ RI

1313

Qð1 þa

ÞI

RðI

þ I

2paðcos

1

a  aS

¼ 4p  2I

 I

¼ p 

 ¼

3K  2m

6K þ 2m

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1  a

R ¼

1  2

8pð1  Þ

Q ¼

1  2

In the preceding equations, K and m are the bulk and shear moduli of the isotropic

matrix, respectively; K

is the bulk modulus of the fluid; and a is the crack aspect

ratio. Dry cavities can be modeled by setting the inclusion moduli to zero. Do not

confuse S with the anisotropic compliance tensor. This model is valid for arbitrary

aspect ratios, unlike the Hudson model, which assumes very small aspect ratio cracks

(see Section 4.10 on Hudson’s model). The results of the two models are essentially

the same for small aspect ratios and low crack densities (< 0.1), as long as the “weak

inclusion” form of Hudson’s theory is used (Cheng, 1993).

Uses

The Eshelby–Cheng model is used to obtain the effective anisotropic stiffness tensor

for transversely isotropic, cracked rocks.

Assumptions and limitations

The following presuppositions and limitations apply to the Eshelby–Cheng model:



the model assumes an isotropic, homogeneous, elastic background matrix and an

idealized ellipsoidal crack shape;



the model assumes low crack concentrations but can handle all aspect ratios; and



because the cavities are isolated with respect to flow, this approach simulates

very-high-frequency behavior appropriate to ultrasonic laboratory conditions.

204 Effective elastic media: bounds and mixing laws

At low frequencies, when there is time for wave-induced pore-pressure increments

to flow and equilibrate, it is better to find the effective moduli for dry cavities and

then saturate them with the Brown and Korringa low-frequency relations. This

should not be confused with the tendency to term this approach a low-frequency

theory, for crack dimensions are assumed to be much smaller than a wavelength.

Extensions

The model has been extended to a transversely isotropic background by Nishizawa

(1982).

4.12 T-matrix inclusion models for effective moduli

Synopsis

One approach for estimating effective elastic constants for composites is based on the

integral equation or T-matrix approach of quantum scattering theory. This approach

takes into account interactions between inclusions based on multiple-point correlation

functions. The integral equation for effective elastic constants of macroscopically

homogeneous materials with statistical fluctuation of properties at the microscopic

level is very similar to the Lippmann–Schwinger–Dyson equation of multiple scat-

tering in quantum mechanics. The theory in the context of elastic composites was

developed by Eimer (1967, 1968), Kro

ner (1967, 1977, 1986), Zeller and Dederichs

(1973), Korringa (1973), and Gubernatis and Krumhansl (1975). Willis (1977) used

a T-matrix approach to obtain bounds and estimates for anisotropic composites.

Middya and Basu ( 1986) applied the T-matrix formalism for polycrystalline aggre-

gates. Jakobsen et al. (2003a) synthesized many of the existing effective medium

approximations and placed them on a common footing using the T-matrix language.

They also applied the formalism to model elastic properties of anisotropic shales.

A homogeneous anisotropic matrix with elastic stiffness tensor C

ð0Þ

has embedded

inclusions, divided into families r ¼1,2, . . . ,N having concentrations n

ðrÞ

and shapes

ðrÞ

. For ellipsoidal inclusions a

ðr Þ

denotes the aspect ratio of the rth family of

inclusions. While the general theory is not limited to ellipsoidal inclusions, most

practical calculations are carried out assuming idealized ellipsoidal shapes. The

effective elastic stiffness is given by Jakobsen et al. (2003a)as



¼ C

ð0Þ

þ T

I  T

1



1

ð4:12:1Þ

where

ðrÞ

ðr Þ

205 4.12 T-matrix inclusion models for effective moduli

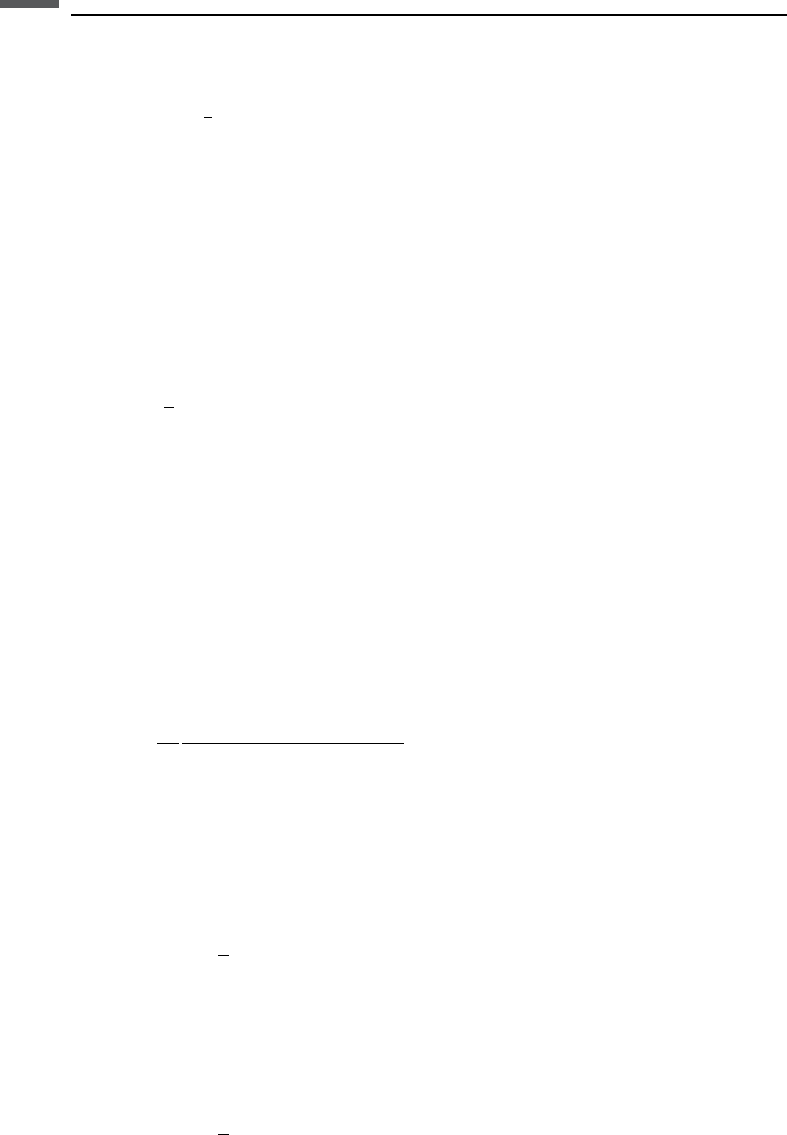
X ¼

ðr Þ

ðrÞ

ðrsÞ

ðsÞ

and I ¼ I

jklm

ðd

þ d

Þ is the fourth-rank identity tensor. The T-matrix t

(r)

for a single inclusion of elastic stiffness C

ðrÞ

is given by

ðrÞ

¼ dC

ðr Þ

I  G

ðrÞ

ðr Þ



1

where

ðr Þ

¼ C

ðr Þ

 C

ð0Þ

The fourth-rank tensor G

(r)

(not the same as the tensor G

(rs)

) is a function of only

(0)

and the inclusion shape. It is computed using the following equations:

ðrÞ

pqrs

¼

ðrÞ

pqrs

þ E

ðr Þ

pqsr

þ E

ðr Þ

qprs

þ E

ðrÞ

qpsr

ðrÞ

pqrs

dy sin y

d D

1

ðkÞk

ðr Þ

ðy;Þ

¼ C

ð0Þ

qmsn

where k, y,andf are spherical coordinates in k-space (Fourier space), and the

components of the unit k-vector are given as usual by ½sin y cos ; sin y sin ; cos y.

The term A

(r)

(y,f) is a shape factor for the rth inclusion and is independent of elastic

constants. For an oblate spheroidal inclusion with short axis b

aligned along the x

-axis,

long axes b

¼b

, and aspect ratio a

(r)

¼b

, A

(r)

is independent of f and is given by

ðrÞ

ðyÞ¼

ðrÞ

sin

y þða

ðr Þ

cos

3=2

For a spherical inclusion A

(r)

¼1/(4p). For the case when C

(0)

has hexagonal sym-

metry and the principal axes of the oblate spheroidal inclusions are aligned along the

symmetry axes of C

(0)

, the nonzero elements of E

pqrs

are given by definite integrals of

polynomial functions (Mura, 1982):

1111

¼ E

2222

ð1  x

Þf½f ð1  x

Þþhg



½ð3e þ dÞð1  x

Þþ4f g

g

ð1  x

Þgdx

3333

¼ 4p

g

½dð1  x

Þþf g

½eð1  x

Þþf g

dx

1122

¼ E

2211

ð1  x

Þf½f ð1  x

Þþhg



½ðe þ 3dÞð1  x

Þþ4f g

3g

ð1  x

Þgdx

206 Effective elastic media: bounds and mixing laws