Stewart J. Calculus
Подождите немного. Документ загружается.

EXAMPLE 3 At the beginning of Section 15.3 we discussed the heat index (perceived
temperature) as a function of the actual temperature and the relative humidity and
gave the following table of values from the National Weather Service.
Find a linear approximation for the heat index when is near and is
near 70%. Use it to estimate the heat index when the temperature is and the relative
humidity is 72%.
SOLUTION We read from the table that . In Section 15.3 we used the tabu-
lar values to estimate that and . (See pages 914–15.)
So the linear approximation is
In particular,
Therefore, when and H 苷 72%, the heat index is
M
DIFFERENTIALS
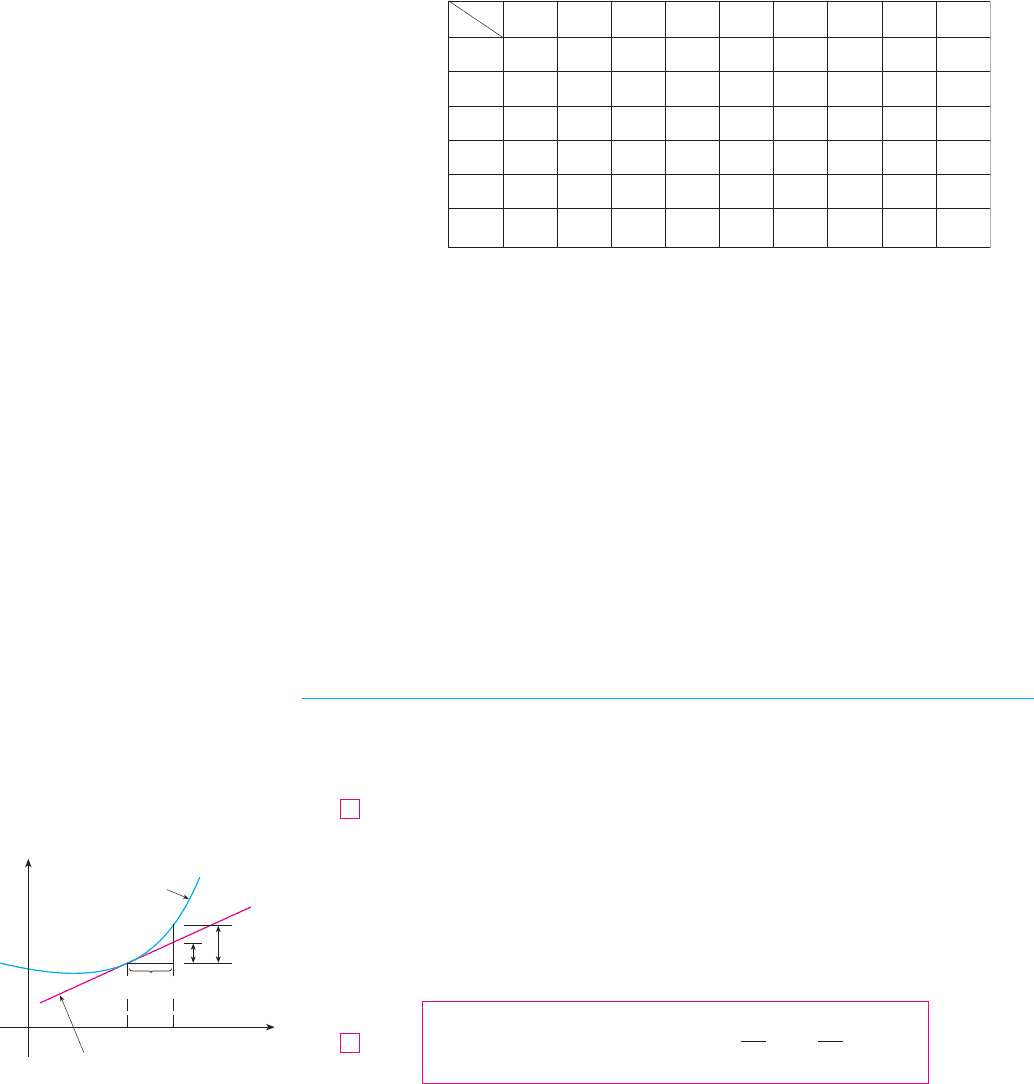
For a differentiable function of one variable, , we define the differential dx to be
an independent variable; that is, dx can be given the value of any real number. The differ-
ential of is then defined as
(See Section 3.9.) Figure 6 shows the relationship between the increment and the dif-
ferential : represents the change in height of the curve and represents the
change in height of the tangent line when changes by an amount
For a differentiable function of two variables, , we define the differentials
and to be independent variables; that is, they can be given any values. Then the dif-
ferential , also called the total differential, is defined by
(Compare with Equation 9.) Sometimes the notation is used in place of .dzdf
dz 苷 f
x
共x, y兲 dx ⫹ f
y
共x, y兲 dy 苷
⭸z
⭸x
dx ⫹
⭸z
⭸y
dy
10
dz
dydx
z 苷 f 共x, y兲
dx 苷 ⌬x.x
dyy 苷 f 共x兲⌬ydy
⌬y
dy 苷 f ⬘共x兲 dx
9
y
y 苷 f 共x兲
I ⬇ 131⬚F
T 苷 97⬚F
f 共97, 72兲⬇125 ⫹ 3.75共1兲 ⫹ 0.9共2兲 苷 130.55
⬇ 125 ⫹ 3.75共T ⫺ 96兲 ⫹ 0.9共H ⫺ 70兲
f 共T, H兲⬇f 共96, 70兲 ⫹ f
T
共96, 70兲共T ⫺ 96兲 ⫹ f
H
共96, 70兲共H ⫺ 70兲
f
H
共96, 70兲⬇0.9f
T
共96, 70兲⬇3.75
f 共96, 70兲 苷 125
97⬚F
H96⬚FTI 苷 f 共T, H 兲
96
100
104
109
114
119
98
103
107
113
118
124
100
105
111
116
123
129
103
108
114
121
127
135
106
112
118
125
133
141
109
115
122
130
138
147
112
119
127
135
144
154
115
123
132
141
150
161
119
128
137
146
157
168
T
H
Relative humidity (%)
Actual
temperature
(°F)
90
92
94
96
98
100
50 55 60 65 70 75 80 85 90
HTI
932
||||
CHAPTER 15 PARTIAL DERIVATIVES
x
a a+Îx
y
0
dx=Îx
y=ƒ
dy
Îy
y=f(a)+fª(a)(x-a)
tangent line
FIGURE 6
If we take and in Equation 10, then the differen-
tial of is
So, in the notation of differentials, the linear approximation (4) can be written as
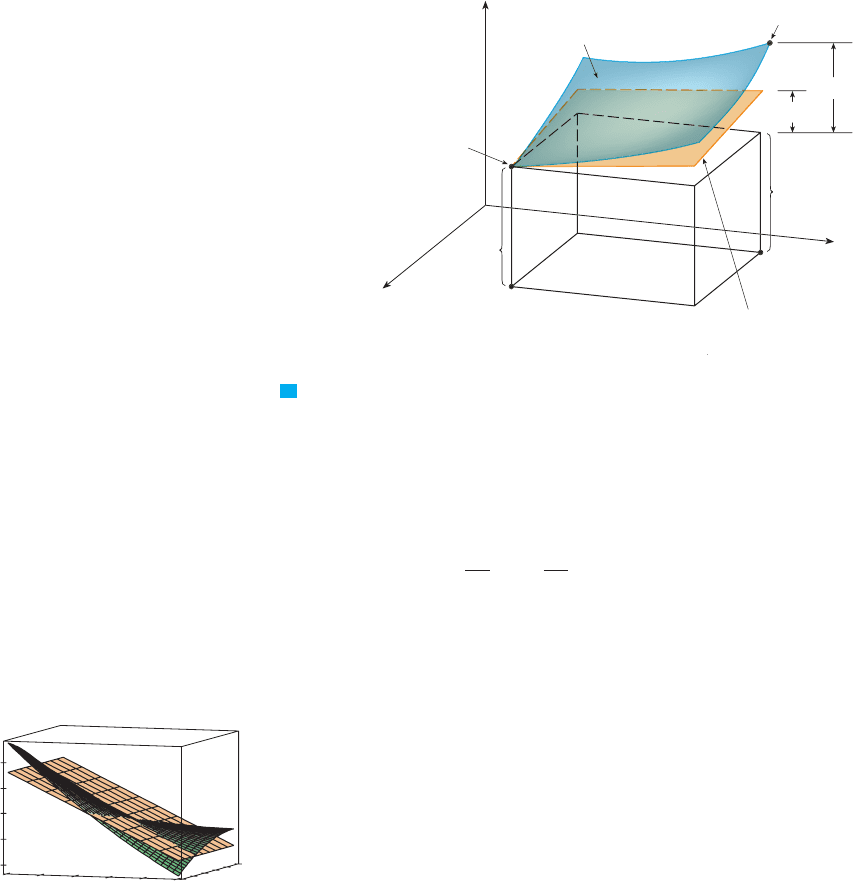
Figure 7 is the three-dimensional counterpart of Figure 6 and shows the geometric inter-
pretation of the differential and the increment : represents the change in height of
the tangent plane, whereas represents the change in height of the surface
when changes from to .
EXAMPLE 4
(a) If , find the differential .
(b) If changes from 2 to and changes from 3 to , compare the values
of and .
SOLUTION
(a) Definition 10 gives
(b) Putting , , , and , we get
The increment of is
Notice that but is easier to compute. M
EXAMPLE 5 The base radius and height of a right circular cone are measured as 10 cm
and 25 cm, respectively, with a possible error in measurement of as much as cm in 0.1
dz⌬z ⬇ dz
苷 0.6449
苷 关共2.05兲
2
⫹ 3共2.05兲共2.96兲 ⫺ 共2.96兲
2
兴 ⫺ 关2
2
⫹ 3共2兲共3兲 ⫺ 3
2
兴
⌬z 苷 f 共2.05, 2.96兲 ⫺ f 共2, 3兲
z
苷 0.65 dz 苷 关2共2兲 ⫹ 3共3兲兴0.05 ⫹ 关3共2兲 ⫺ 2共3兲兴共⫺0.04兲
dy 苷 ⌬y 苷 ⫺0.04y 苷 3dx 苷 ⌬x 苷 0.05x 苷 2
dz 苷
⭸z
⭸x
dx ⫹
⭸z
⭸y
dy 苷 共2x ⫹ 3y兲 dx ⫹ 共3x ⫺ 2y兲 dy
dz⌬z
2.96y2.05x
dzz 苷 f 共x, y兲 苷 x
2
⫹ 3xy ⫺ y
2
V
y
x
z
Î
x
=
d
x
0
{
a,
{
b,
f
(a
,
b
)
}
(
a,
b
,
0)
(
a
+
Î
x
,
b
+
Î
y
,
0)
{
a
+
Î
x,
b+
Î
y
,
f
(
a+
Î
x,
b
+
Î
y)
}
f
(a
,
b)
f
(
a
,
b)
Îy=
d
y
tan
g
ent plan
e
z-
f
(
a
,
b)=f
x
f
f
(
a
,
b)(x-a)+f
y
ff
(
a
,
b
)
(
y
-
b)
su
r
face
z
=
f
(
x
,
y)
dz
Î
z
FI
G
URE
7
共a ⫹⌬x, b ⫹⌬y兲共a, b兲共x, y兲
z 苷 f 共x, y兲⌬z
dz⌬zdz
f 共x, y兲⬇f 共a, b兲 ⫹ dz
dz 苷 f
x
共a, b兲共x ⫺ a兲 ⫹ f
y
共a, b兲共y ⫺ b兲
z
dy 苷 ⌬y 苷 y ⫺ bdx 苷 ⌬x 苷 x ⫺ a
SECTION 15.4 TANGENT PLANES AND LINEAR APPROXIMATIONS
||||
933
FIGURE 8
60
0
5
3
1
2
x
y
z
20
4
40
2
4
_20
0
0
N In Example 4, is close to because the
tangent plane is a good approximation to the
surface near .
(See Figure 8.)
共2, 3, 13兲z 苷 x
2
⫹ 3xy ⫺ y
2
⌬zdz

each. Use differentials to estimate the maximum error in the calculated volume of the
cone.
SOLUTION The volume of a cone with base radius and height is . So the
differential of is
Since each error is at most cm, we have , . To find the largest
error in the volume we take the largest error in the measurement of and of . Therefore
we take and along with , . This gives
Thus the maximum error in the calculated volume is about cm cm .
M
FUNCTIONS OF THREE OR MORE VARIABLES
Linear approximations, differentiability, and differentials can be defined in a similar man-
ner for functions of more than two variables. A differentiable function is defined by an
expression similar to the one in Definition 7. For such functions the linear approximation
is
and the linearization is the right side of this expression.
If , then the increment of is
The differential is defined in terms of the differentials , , and of the independ-
ent variables by
EXAMPLE 6 The dimensions of a rectangular box are measured to be 75 cm, 60 cm,
and 40 cm, and each measurement is correct to within cm. Use differentials to esti-
mate the largest possible error when the volume of the box is calculated from these
measurements.
SOLUTION If the dimensions of the box are , , and , its volume is and so
We are given that , , and . To find the largest error in
the volume, we therefore use , , and together with ,
, and :
Thus an error of only cm in measuring each dimension could lead to an error of as
much as 1980 cm in the calculated volume! This may seem like a large error, but it’s
only about 1% of the volume of the box. M
3
0.2
⌬V ⬇ dV 苷 共60兲共40兲共0.2兲 ⫹ 共75兲共40兲共0.2兲 ⫹ 共75兲共60兲共0.2兲 苷 1980
z 苷 40y 苷 60
x 苷 75dz 苷 0.2dy 苷 0.2dx 苷 0.2
ⱍ
⌬z
ⱍ
艋 0.2
ⱍ
⌬y
ⱍ
艋 0.2
ⱍ
⌬x
ⱍ
艋 0.2
dV 苷
⭸V
⭸x
dx ⫹
⭸V
⭸y
dy ⫹
⭸V
⭸z
dz 苷 yz dx ⫹ xz dy ⫹ xy dz
V 苷 xyzzyx
0.2
dw 苷
⭸
w
⭸x
dx ⫹
⭸
w
⭸y
dy ⫹
⭸
w
⭸z
dz
dzdydxdw
⌬w 苷 f 共x ⫹⌬x, y ⫹⌬y, z ⫹⌬z兲 ⫺ f 共x, y, z兲
ww 苷 f 共x, y, z兲
L共x, y, z兲
f 共x, y, z兲⬇f 共a, b, c兲 ⫹ f
x
共a, b, c兲共x ⫺ a兲 ⫹ f
y
共a, b, c兲共y ⫺ b兲 ⫹ f
z
共a, b, c兲共z ⫺ c兲
33
⬇ 6320
dV 苷
500
3
共0.1兲 ⫹
100
3
共0.1兲 苷 20
h 苷 25r 苷 10dh 苷 0.1dr 苷 0.1
hr
ⱍ
⌬h
ⱍ
艋 0.1
ⱍ
⌬r
ⱍ
艋 0.10.1
dV 苷
⭸V
⭸r
dr ⫹
⭸V
⭸h
dh 苷
2
rh
3
dr ⫹
r
2
3
dh
V
V 苷
r
2
h兾3hrV
934
||||
CHAPTER 15 PARTIAL DERIVATIVES
SECTION 15.4 TANGENT PLANES AND LINEAR APPROXIMATIONS
||||
935
19.
Find the linear approximation of the function
at and use it to
approximate .
;
20. Find the linear approximation of the function
at and use it to approximate
. Illustrate by graphing and the tangent plane.
Find the linear approximation of the function
at and use it to
approximate the number .
22. The wave heights in the open sea depend on the speed
of the wind and the length of time that the wind has been
blowing at that speed. Values of the function are
recorded in feet in the following table.
Use the table to find a linear approximation to the wave
height function when is near 40 knots and is near
20 hours. Then estimate the wave heights when the wind has
been blowing for 24 hours at 43 knots.
23. Use the table in Example 3 to find a linear approximation to
the heat index function when the temperature is near
and the relative humidity is near 80%. Then estimate the heat
index when the temperature is and the relative humidity
is 78%.
24. The wind-chill index is the perceived temperature when the
actual temperature is and the wind speed is , so we can
write . The following table of values is an excerpt
from Table 1 in Section 15.1.
Use the table to find a linear approximation to the wind-chill
⫺18
⫺24
⫺30
⫺37
⫺20
⫺26
⫺33
⫺39
⫺21
⫺27
⫺34
⫺41
⫺22
⫺29
⫺35
⫺42
⫺23
⫺30
⫺36
⫺43
T
v
20 30 40 50 60
⫺10
⫺15
⫺20
⫺25
Actual temperature (°C)
70
⫺23
⫺30
⫺37
⫺44
Wind speed (km/h)
W 苷 f 共T, v兲
vT
W
95⬚F
94⬚F
t
v
5
9
14
19
24
7
13
21
29
37
8
16
25
36
47
8
17
28
40
54
9
18
31
45
62
9
19
33
48
67
9
19
33
50
69
v
t
5 101520304050
20
30
40
50
60
Duration (hours)
Wind speed (knots)
h 苷 f 共v, t兲
t
vh
s
共3.02兲
2
⫹ 共1.97兲
2
⫹ 共5.99兲
2
共3, 2, 6兲
f 共x, y, z兲 苷
s
x
2
⫹ y
2
⫹ z
2
21.
ff 共6.9, 2.06兲
共7, 2兲f 共x, y兲 苷 ln共x ⫺ 3y兲
f 共1.95, 1.08兲
共2, 1兲
f 共x, y兲 苷
s
20 ⫺ x
2
⫺ 7y
2
1–6 Find an equation of the tangent plane to the given surface at
the specified point.
1. ,
2. ,
3. ,
4. ,
5. ,
6. ,
;
7–8 Graph the surface and the tangent plane at the given point.
(Choose the domain and viewpoint so that you get a good view of
both the surface and the tangent plane.) Then zoom in until the
surface and the tangent plane become indistinguishable.
7. ,
8. ,
9–10 Draw the graph of and its tangent plane at the given
point. (Use your computer algebra system both to compute the
partial derivatives and to graph the surface and its tangent plane.)
Then zoom in until the surface and the tangent plane become
indistinguishable.
9.
10.
11–16 Explain why the function is differentiable at the given
point. Then find the linearization of the function at
that point.
,
12. ,
13. ,
14. ,
15. ,
16. ,
17–18 Verify the linear approximation at .
17. 18.
s
y ⫹ cos
2
x
⬇ 1 ⫹
1
2
y
2x ⫹ 3
4y ⫹ 1
⬇ 3 ⫹ 2x ⫺ 12y
共0, 0兲
共⫺3, 2兲f 共x, y兲 苷 sin共2x ⫹ 3y兲
共
, 0兲f 共x, y兲 苷 e
⫺xy
cos y
共3, 0兲f 共x, y兲 苷
s
x ⫹ e
4y
共2, 1兲f 共x, y兲 苷
x
x ⫹ y
共1, 1兲f 共x, y兲 苷 x
3
y
4
共1, 4兲
f 共x, y兲 苷 x
s
y
11.
L共x, y兲
f 共x, y兲 苷 e
⫺xy兾10
(
s
x
⫹
s
y
⫹
s
xy
)
, 共1, 1, 3e
⫺0.1
兲
f 共x, y兲 苷
xy sin共x ⫺ y兲
1 ⫹ x
2
⫹ y
2
, 共1, 1, 0兲
f
CAS
共1, 1,
兾4兲z 苷 arctan共xy
2
兲
共1, 1, 5兲z 苷 x
2
⫹ xy ⫹ 3y
2
共1, ⫺1, 1兲z 苷 e
x
2
⫺y
2
共2, 2, 2兲z 苷 y cos共x ⫺ y兲
共1, 4, 0兲z 苷 y ln x
共1, 1, 1兲z 苷
s
xy
共2, ⫺2, 12兲z 苷 3共x ⫺ 1兲
2
⫹ 2共y ⫹ 3兲
2
⫹ 7
共⫺1, 2, 4兲z 苷 4x
2
⫺ y
2
⫹ 2y
EXERCISES
15.4
39. If is the total resistance of three resistors, connected in par-
allel, with resistances , , , then
If the resistances are measured in ohms as ,
, and , with a possible error of in
each case, estimate the maximum error in the calculated value
of .
40. Four positive numbers, each less than 50, are rounded to the
first decimal place and then multiplied together. Use differen-
tials to estimate the maximum possible error in the computed
product that might result from the rounding.
41. A model for the surface area of a human body is given by
, where is the weight (in pounds), is
the height (in inches), and is measured in square feet. If the
errors in measurement of and are at most 2%, use differ-
entials to estimate the maximum percentage error in the
calculated surface area.
42. Suppose you need to know an equation of the tangent plane to
a surface at the point . You don’t have an equation
for but you know that the curves
both lie on . Find an equation of the tangent plane at .
43– 44 Show that the function is differentiable by finding values
of and that satisfy Definition 7.
44.
Prove that if is a function of two variables that is differen-
tiable at , then is continuous at .
Hint: Show that
46. (a) The function
was graphed in Figure 4. Show that and
both exist but is not differentiable at . [Hint: Use
the result of Exercise 45.]
(b) Explain why and are not continuous at .共0, 0兲f
y
f
x
共0, 0兲f
f
y
共0, 0兲f
x
共0, 0兲
f 共x, y兲 苷
再
0
xy
x
2
⫹ y
2
if
if
共x, y兲 苷 共0, 0兲
共x, y兲 苷 共0, 0兲
lim
共⌬x, ⌬y兲
l
共0, 0兲
f 共a ⫹⌬x, b ⫹⌬y兲 苷 f 共a, b兲
共a, b兲f共a, b兲
f
45.
f 共x, y兲 苷 xy ⫺ 5y
2
f 共x, y兲 苷 x
2
⫹ y
2
43.
2
1
PS
r
2
共u兲 苷 具1 ⫹ u
2
, 2u
3
⫺ 1, 2u ⫹ 1典
r
1
共t兲 苷 具2 ⫹ 3t, 1 ⫺ t
2
, 3 ⫺ 4t ⫹ t
2
典
S
P共2, 1, 3兲S
h
w
S
h
wS 苷 0.1091w
0.425
h
0.725
R
0.5%R
3
苷 50 ⍀R
2
苷 40 ⍀
R
1
苷 25 ⍀
1
R
苷
1
R
1
⫹
1
R
2
⫹
1
R
3
R
3
R
2
R
1
Rindex function when is near and is near .
Then estimate the wind-chill index when the temperature is
and the wind speed is 55 km兾h.
25–30 Find the differential of the function.
25. 26.
27. 28.
29. 30.
If and changes from to
compare the values of and .
32. If and changes from to
, compare the values of and .
33. The length and width of a rectangle are measured as 30 cm and
24 cm, respectively, with an error in measurement of at most
cm in each. Use differentials to estimate the maximum
error in the calculated area of the rectangle.
34. The dimensions of a closed rectangular box are measured as
80 cm, 60 cm, and 50 cm, respectively, with a possible error
of cm in each dimension. Use differentials to estimate the
maximum error in calculating the surface area of the box.
Use differentials to estimate the amount of tin in a closed tin
can with diameter 8 cm and height 12 cm if the tin is cm
thick.
36. Use differentials to estimate the amount of metal in a closed
cylindrical can that is 10 cm high and 4 cm in diameter if the
metal in the top and bottom is cm thick and the metal in the
sides is cm thick.
A boundary stripe 3 in. wide is painted around a rectangle
whose dimensions are 100 ft by 200 ft. Use differentials to
approximate the number of square feet of paint in the stripe.
38. The pressure, volume, and temperature of a mole of an ideal
gas are related by the equation , where is mea-
sured in kilopascals, in liters, and in kelvins. Use differen-
tials to find the approximate change in the pressure if the
volume increases from 12 L to 12.3 L and the temperature
decreases from 310 K to 305 K.
TV
PPV 苷 8.31T
37.
0.05
0.1
0.04
35.
0.2
0.1
dz⌬z共2.96, ⫺0.95兲
共3, ⫺1兲共x, y兲z 苷 x
2
⫺ xy ⫹ 3y
2
dz⌬z
共1.05, 2.1兲,共1, 2兲共x, y兲z 苷 5x
2
⫹ y
2
31.
w 苷 xye
xz
R 苷
␣

2
cos
␥
T 苷
v
1 ⫹ uvw
m 苷 p
5
q
3
v 苷 y cos xyz 苷 x
3
ln共y
2
兲
⫺17⬚C
50 km兾h
v⫺15⬚CT
936
||||
CHAPTER 15 PARTIAL DERIVATIVES

THE CHAIN RULE
Recall that the Chain Rule for functions of a single variable gives the rule for differenti-
ating a composite function: If and , where and are differentiable func-
tions, then is indirectly a differentiable function of and
For functions of more than one variable, the Chain Rule has several versions, each of
them giving a rule for differentiating a composite function. The first version (Theorem 2)
deals with the case where and each of the variables and is, in turn, a func-
tion of a variable . This means that is indirectly a function of , , and the
Chain Rule gives a formula for differentiating as a function of . We assume that is dif-
ferentiable (Definition 15.4.7). Recall that this is the case when and are continuous
(Theorem 15.4.8).
THE CHAIN RULE (CASE 1) Suppose that is a differentiable func-
tion of and , where and are both differentiable functions of .
Then is a differentiable function of and
PROOF A change of in produces changes of in and in . These, in turn, pro-
duce a change of in , and from Definition 15.4.7 we have
where and as . [If the functions and are not
defined at , we can define them to be 0 there.] Dividing both sides of this equation
by , we have
If we now let , then because is differentiable and
therefore continuous. Similarly, . This, in turn, means that and , so
M 苷
⭸f
⭸x
dx
dt
⫹
⭸f
⭸y
dy
dt
苷
⭸f
⭸x
dx
dt
⫹
⭸f
⭸y
dy
dt
⫹ 0 ⴢ
dx
dt
⫹ 0 ⴢ
dy
dt
苷
⭸f
⭸x
lim
⌬t
l
0
⌬x
⌬t
⫹
⭸f
⭸y
lim
⌬t
l
0
⌬y
⌬t
⫹
冢
lim
⌬t
l
0
1
冣
lim
⌬t
l
0
⌬x
⌬t
⫹
冢
lim
⌬t
l
0
2
冣
lim
⌬t
l
0
⌬y
⌬t
dz
dt
苷 lim
⌬t
l
0
⌬z
⌬t
2
l 0
1
l 0⌬y l 0
t⌬x 苷 t共t ⫹⌬t兲 ⫺ t共t兲 l 0⌬t l 0
⌬z
⌬t
苷
⭸f
⭸x
⌬x
⌬t
⫹
⭸f
⭸y
⌬y
⌬t
⫹
1
⌬x
⌬t
⫹
2
⌬y
⌬t
⌬t
共0, 0兲
2
1
共⌬x, ⌬y兲 l 共0, 0兲
2
l 0
1
l 0
⌬z 苷
⭸f
⭸x
⌬x ⫹
⭸f
⭸y
⌬y ⫹
1
⌬x ⫹
2
⌬y
z⌬z
y⌬yx⌬xt⌬t
dz
dt
苷
⭸f
⭸x
dx
dt
⫹
⭸f
⭸y
dy
dt
tz
ty 苷 h共t兲x 苷 t共t兲yx
z 苷 f 共x, y兲
2
f
y
f
x
ftz
z 苷 f 共t共t兲, h共t兲兲tzt
yxz 苷 f 共x, y兲
dy
dt
苷
dy
dx
dx
dt
1
ty
tfx 苷 t共t兲y 苷 f 共x兲
15.5
SECTION 15.5 THE CHAIN RULE
||||
937
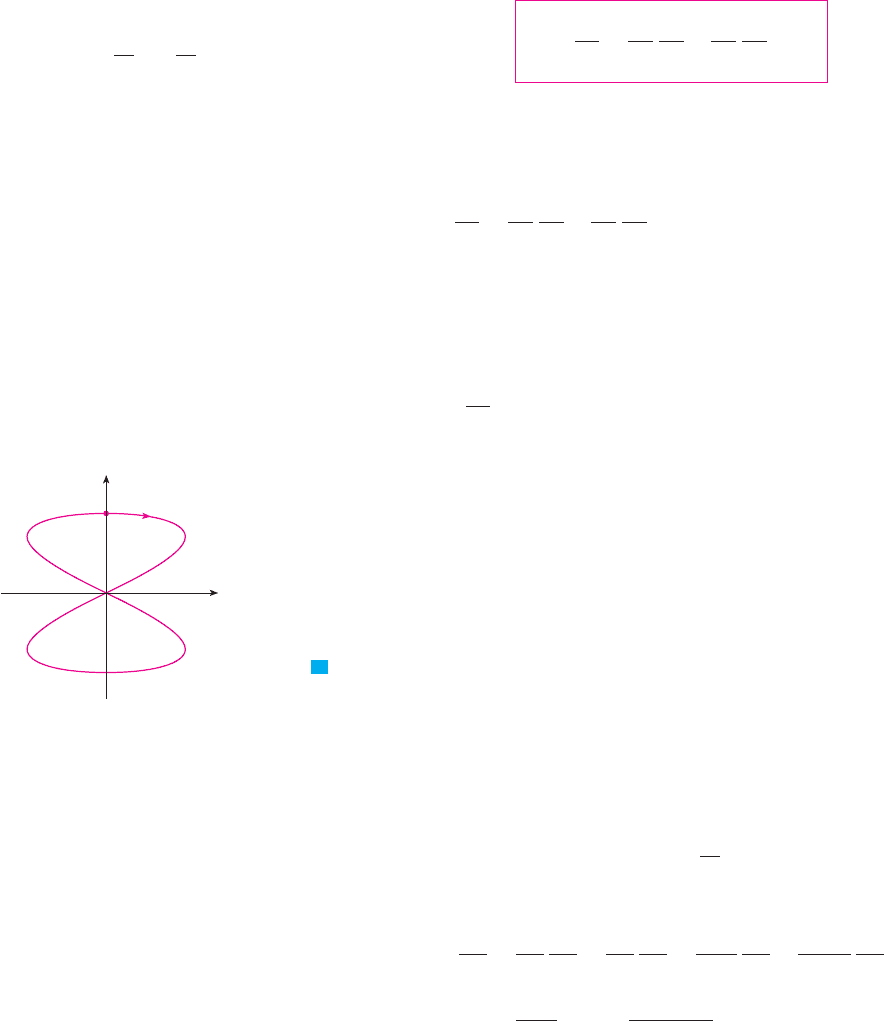
Since we often write in place of , we can rewrite the Chain Rule in the form
EXAMPLE 1 If , where and , find when .
SOLUTION The Chain Rule gives
It’s not necessary to substitute the expressions for and in terms of . We simply
observe that when , we have x 苷 sin 0 苷 0 and y 苷 cos 0 苷 1. Therefore
M
The derivative in Example 1 can be interpreted as the rate of change of with respect
to as the point moves along the curve with parametric equations ,
. (See Figure 1.) In particular, when , the point is and
is the rate of increase as we move along the curve through . If, for instance,
represents the temperature at the point , then the compos-
ite function represents the temperature at points on and the deriva-
tive represents the rate at which the temperature changes along .
EXAMPLE 2 The pressure (in kilopascals), volume (in liters), and temperature
(in kelvins) of a mole of an ideal gas are related by the equation . Find the
rate at which the pressure is changing when the temperature is and increasing at a
rate of and the volume is 100 L and increasing at a rate of .
SOLUTION If represents the time elapsed in seconds, then at the given instant we have
, , , . Since
the Chain Rule gives
The pressure is decreasing at a rate of about kPa兾s. M
We now consider the situation where but each of and is a function of two
variables and : , . Then is indirectly a function of and and we tszy 苷 h共s, t兲x 苷 t共s, t兲ts
yxz 苷 f 共x, y兲
0.042
苷
8.31
100
共0.1兲 ⫺
8.31共300兲
100
2
共0.2兲 苷 ⫺0.04155
dP
dt
苷
⭸P
⭸T
dT
dt
⫹
⭸P
⭸V
dV
dt
苷
8.31
V
dT
dt
⫺
8.31T
V
2
dV
dt
P 苷 8.31
T
V
dV兾dt 苷 0.2V 苷 100dT兾dt 苷 0.1T 苷 300
t
0.2 L兾s0.1 K兾s
300 K
PV 苷 8.31T
TVP
V
Cdz兾dt
Cz 苷 T共sin 2t, cos t兲
共x, y兲z 苷 T共x, y兲 苷 x
2
y ⫹ 3xy
4
共0, 1兲C
dz兾dt 苷 6共0, 1兲共x, y兲t 苷 0y 苷 cos t
x 苷 sin 2tC共x, y兲t
z
dz
dt
冟
t苷0
苷 共0 ⫹ 3兲共2 cos 0兲 ⫹ 共0 ⫹ 0兲共⫺sin 0兲 苷 6
t 苷 0
tyx
苷 共2xy ⫹ 3y
4
兲共2 cos 2t兲 ⫹ 共x
2
⫹ 12xy
3
兲共⫺sin t兲
dz
dt
苷
⭸z
⭸x
dx
dt
⫹
⭸z
⭸y
dy
dt
t 苷 0dz兾dty 苷 cos tx 苷 sin 2tz 苷 x
2
y ⫹ 3xy
4
dz
dt
苷
⭸z
⭸x
dx
dt
⫹
⭸z
⭸y
dy
dt
⭸f兾⭸x⭸z兾⭸x
938
||||
CHAPTER 15 PARTIAL DERIVATIVES
N Notice the similarity to the definition of the
differential:
dz 苷
⭸z
⭸x
dx ⫹
⭸z
⭸y
dy
FIGURE 1
The curve x=sin2t, y=cost
x
(0,1)
y
C
wish to find and . Recall that in computing we hold fixed and compute
the ordinary derivative of with respect to . Therefore we can apply Theorem 2 to obtain
A similar argument holds for and so we have proved the following version of the
Chain Rule.
THE CHAIN RULE (CASE 2) Suppose that is a differentiable func-
tion of and , where and are differentiable functions of s
and t. Then
EXAMPLE 3 If , where and , find and .
SOLUTION Applying Case 2 of the Chain Rule, we get
M
Case 2 of the Chain Rule contains three types of variables: and are independent
variables, and are called intermediate variables, and is the dependent variable.
Notice that Theorem 3 has one term for each intermediate variable and each of these terms
resembles the one-dimensional Chain Rule in Equation 1.
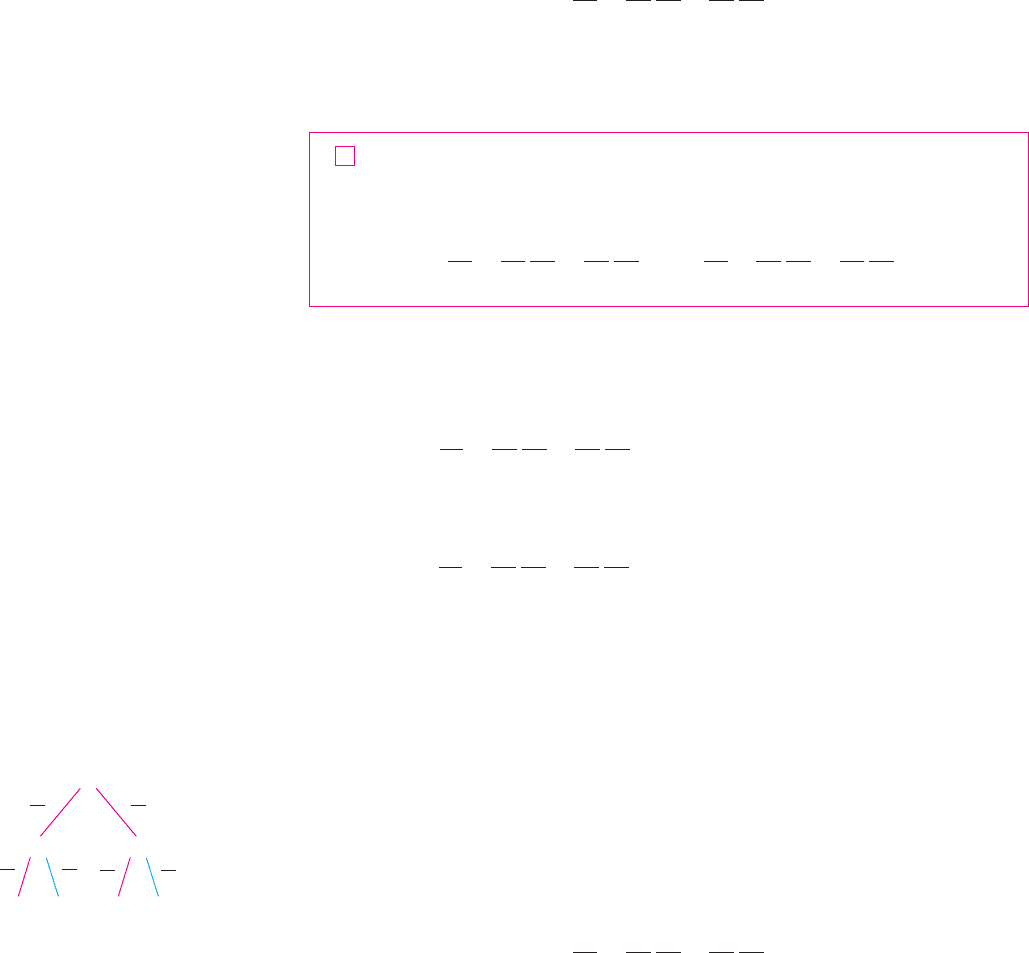
To remember the Chain Rule, it’s helpful to draw the tree diagram in Figure 2. We draw
branches from the dependent variable to the intermediate variables and to indicate
that is a function of and . Then we draw branches from and to the independent
variables and . On each branch we write the corresponding partial derivative. To find
, we find the product of the partial derivatives along each path from to and then
add these products:
Similarly, we find by using the paths from to .
Now we consider the general situation in which a dependent variable is a function of
intermediate variables , , each of which is, in turn, a function of independent
variables , . Notice that there are terms, one for each intermediate variable. The
proof is similar to that of Case 1.
nt
m
...,t
1
mx
n
...,x
1
n
u
tz⭸z兾⭸t
⭸z
⭸s
苷
⭸z
⭸x
⭸x
⭸s
⫹
⭸z
⭸y
⭸y
⭸s
sz⭸z兾⭸s
ts
yxyxz
yxz
zyx
ts
苷 2ste
st
2
sin 共s
2
t兲 ⫹ s
2
e
st
2
cos共s
2
t兲
⭸z
⭸t
苷
⭸z
⭸x
⭸x
⭸t
⫹
⭸z
⭸y
⭸y
⭸t
苷 共e
x
sin y兲共2st兲 ⫹ 共e
x
cos y兲共s
2
兲
苷 t
2
e
st
2
sin 共s
2
t兲 ⫹ 2ste
st
2
cos共s
2
t兲
⭸z
⭸s
苷
⭸z
⭸x
⭸x
⭸s
⫹
⭸z
⭸y
⭸y
⭸s
苷 共e
x
sin y兲共t
2
兲 ⫹ 共e
x
cos y兲共2st兲
⭸z兾⭸t⭸z兾⭸sy 苷 s
2
tx 苷 st
2
z 苷 e
x
sin y
⭸z
⭸t
苷
⭸z
⭸x
⭸x
⭸t
⫹
⭸z
⭸y
⭸y
⭸t
⭸z
⭸s
苷
⭸z
⭸x
⭸x
⭸s
⫹
⭸z
⭸y
⭸y
⭸s
y 苷 h共s, t兲x 苷 t共s, t兲yx
z 苷 f 共x, y兲
3
⭸z兾⭸s
⭸z
⭸t
苷
⭸z
⭸x
⭸x
⭸t
⫹
⭸z
⭸y
⭸y
⭸t
tz
s⭸z兾⭸t⭸z兾⭸t⭸z兾⭸s
SECTION 15.5 THE CHAIN RULE
||||
939
FIGURE 2
z
yx
st st
x
s
x
t
y
t
y
s
z
x
z
y

THE CHAIN RULE (GENERAL VERSION) Suppose that is a differentiable func-
tion of the variables , , and each is a differentiable function of the
variables , , . Then is a function of , , and
for each , , .
EXAMPLE 4 Write out the Chain Rule for the case where and
, , , and .
SOLUTION We apply Theorem 4 with and . Figure 3 shows the tree diagram.
Although we haven’t written the derivatives on the branches, it’s understood that if a
branch leads from to , then the partial derivative for that branch is . With the aid
of the tree diagram, we can now write the required expressions:
M
EXAMPLE 5 If , where , , and , find the
value of when , , .
SOLUTION With the help of the tree diagram in Figure 4, we have
When , , and , we have , , and , so
M
EXAMPLE 6 If and is differentiable, show that satisfies
the equation
SOLUTION Let and . Then and the Chain Rule
gives
Therefore
Mt
t
s
s
t
t
苷
冉
2st
f
x
2st
f
y
冊
冉
2st
f
x
2st
f
y
冊
苷 0
t
t
苷
f
x
x
t
f
y
y
t
苷
f
x
共2t兲
f
y
共2t兲
t
s
苷
f
x
x
s
f
y
y
s
苷
f
x
共2s兲
f
y
共2s兲
t共s, t兲 苷 f 共x, y兲y 苷 t
2
s
2
x 苷 s
2
t
2
t
t
s
s
t
t
苷 0
tft共s, t兲 苷 f 共s
2
t
2
, t
2
s
2
兲
u
s
苷 共64兲共2兲 共16兲共4兲 共0兲共0兲 苷 192
z 苷 0y 苷 2x 苷 2t 苷 0s 苷 1r 苷 2
苷 共4x
3
y兲共re
t
兲 共x
4
2yz
3
兲共2rse
t
兲 共3y
2
z
2
兲共r
2
sin t兲
u
s
苷
u
x
x
s
u
y
y
s
u
z
z
s
t 苷 0s 苷 1r 苷 2u兾s
z 苷 r
2
s sin ty 苷 rs
2
e
t
x 苷 rse
t
u 苷 x
4
y y
2
z
3
V
w
v
苷
w
x
x
v
w
y
y
v
w
z
z
v
w
t
t
v
w
u
苷
w
x
x
u
w
y
y
u
w
z
z
u
w
t
t
u
y兾uuy
m 苷 2n 苷 4
t 苷 t共u, v兲z 苷 z共u, v兲y 苷 y共u, v兲x 苷 x共u, v兲
w 苷 f 共x, y, z, t兲
V
m...,2i 苷 1
u
t
i
苷
u
x
1
x
1
t
i
u
x
2
x
2
t
i
u
x
n
x
n
t
i
t
m
...,t
2
t
1
ut
m
...,t
2
t
1
m
x
j
x
n
...,x
2
x
1
n
u
4
940
||||
CHAPTER 15 PARTIAL DERIVATIVES
FIGURE 3
w
ytx
u
v
u
v
u
v
z
u
v
FIGURE 4
u
yzx
srt srt srt

EXAMPLE 7 If has continuous second-order partial derivatives and
and , find (a) and (b) .
SOLUTION
(a) The Chain Rule gives
(b) Applying the Product Rule to the expression in part (a), we get
But, using the Chain Rule again (see Figure 5), we have
Putting these expressions into Equation 5 and using the equality of the mixed second-
order derivatives, we obtain
M
IMPLICIT DIFFERENTIATION
The Chain Rule can be used to give a more complete description of the process of implicit
differentiation that was introduced in Sections 3.6 and 15.3. We suppose that an equa-
tion of the form defines implicitly as a differentiable function of , that is,
, where for all in the domain of . If is differentiable, we can
apply Case 1 of the Chain Rule to differentiate both sides of the equation with
respect to . Since both and are functions of , we obtain
But , so if we solve for and obtain
dy
dx
苷
F
x
F
y
苷
F
x
F
y
6
dy兾dxF兾y 苷 0dx兾dx 苷 1
F
x
dx
dx
F
y
dy
dx
苷 0
xyxx
F共x, y兲 苷 0
FfxF共x, f 共x兲兲 苷 0y 苷 f 共x兲
xyF共x, y兲 苷 0
苷 2
z
x
4r
2
2
z
x
2
8rs
2
z
x y
4s
2
2
z
y
2
2
z
r
2
苷 2
z
x
2r
冉
2r
2
z
x
2
2s
2
z
y x
冊
2s
冉
2r
2
z
x y
2s
2
z
y
2
冊
苷
2
z
x y
共2r兲
2
z
y
2
共2s兲
r
冉
z
y
冊
苷
x
冉
z
y
冊
x
r
y
冉
z
y
冊
y
r
苷
2
z
x
2
共2r兲
2
z
y x
共2s兲
r
冉
z
x
冊
苷
x
冉
z
x
冊
x
r
y
冉
z
x
冊
y
r
苷 2
z
x
2r
r
冉
z
x
冊
2s
r
冉
z
y
冊
2
z
r
2
苷
r
冉
2r
z
x
2s
z
y
冊
5
z
r
苷
z
x
x
r
z
y
y
r
苷
z
x
共2r兲
z
y
共2s兲
2
z兾r
2
z兾ry 苷 2rsx 苷 r
2
s
2
z 苷 f 共x, y兲
SECTION 15.5 THE CHAIN RULE
||||
941
FIGURE 5
z
x
yx
rsrs
