Alligood K., Sauer T., Yorke J.A. Chaos: An Introduction to Dynamical Systems
Подождите немного. Документ загружается.

7.4 NONLINEAR S YSTEMS
d
de
L(t
1
-t
0
)
R
n
t
0
t
1
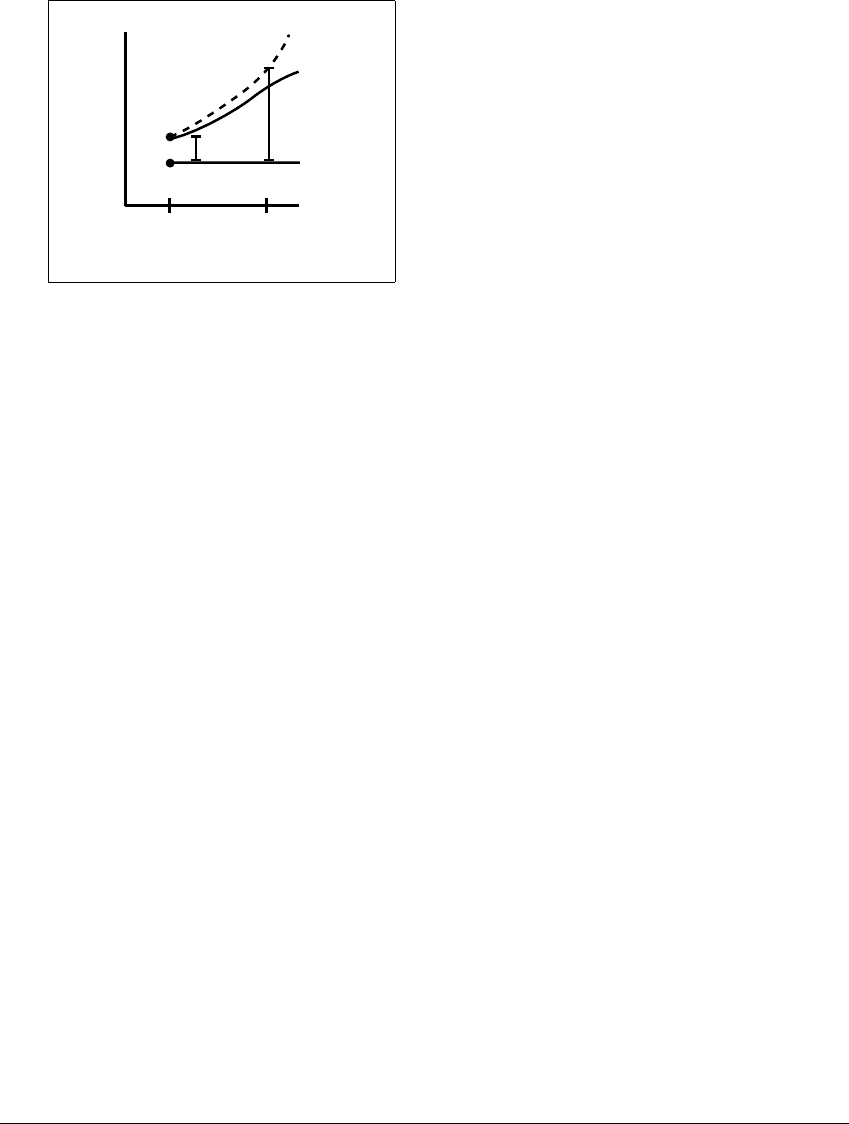
Figure 7.13 The Gronwall inequality.
Nearby solutions can diverge no faster than an exponential rate determined by the
Lipschitz constant of the differential equation.
Higher-order equations often can be transformed into a first-order system
of form (7.28). We illustrate the process with the nth-order differential equation
x
(n)
⫽ f(x,
˙
x,
¨
x,...,x
(n⫺1)
),
where x
(n)
denotes the nth derivative of the function x(t) with respect to t.Define
a new set of variables by
x
1
⫽ x
x
2
⫽
˙
x
x
3
⫽
¨
x
.
.
.
x
n
⫽ x
(n⫺1)
These new variables satisfy the first-order autonomous system
˙
x
1
⫽ x
2
˙
x
2
⫽ x
3
.
.
.
˙
x
n⫺1
⫽ x
n
˙
x
n
⫽ f(x
1
,x
2
,...,x
n
)
which is of form (7.28).
297
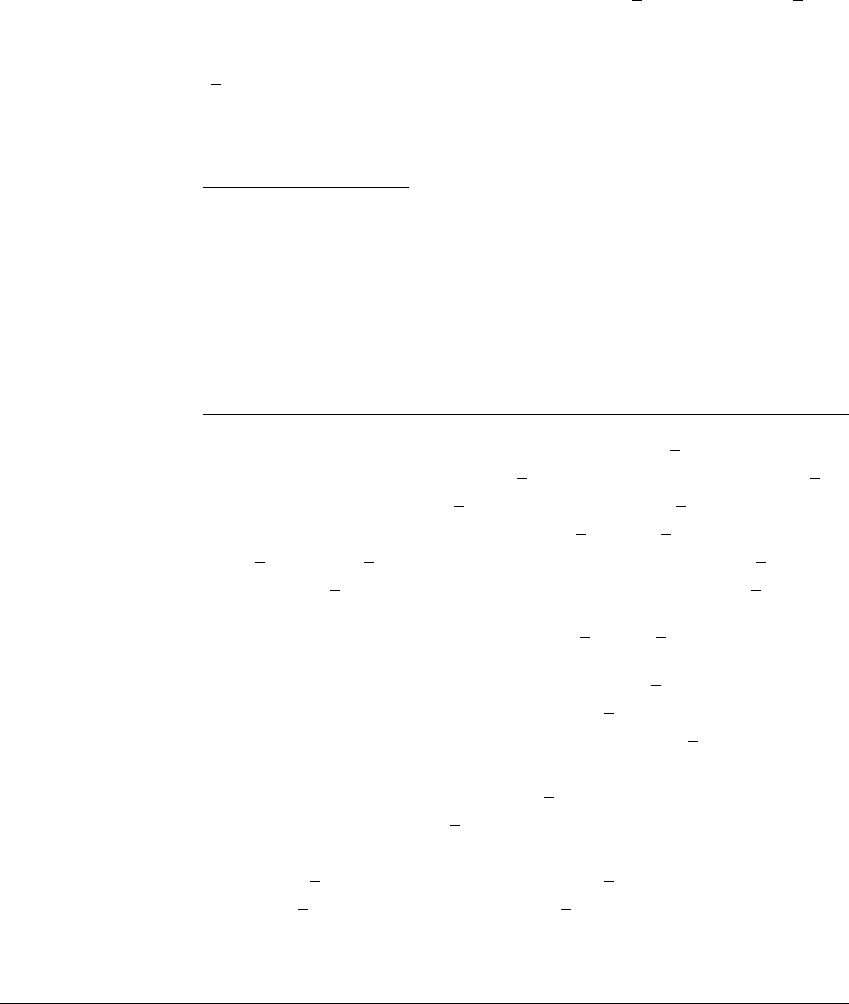
D IFFERENTIAL E QUATIONS
Unlike linear systems, most nonlinear systems of ordinary differential equa-
tions cannot be solved explicitly, meaning that the solutions cannot be found
through an analytic calculation. Fortunately, much of the stability analysis for
linear systems carries over to the study of equilibria of nonlinear systems.
Recall that an equilibrium of (7.29) is a vector
v in ⺢
n
such that f
i
(v) ⫽ 0
for i ⫽ 1,...,n. Again, for a given initial value v
0
, we denote by F(t, v
0
)the
solution of (7.29) at time t. While a linear system either has only one equilibrium
(
v ⫽ 0) or has an entire line (or higher-dimensional subspace) of equilibria (see
Exercise T7.6), a nonlinear system can have many isolated equilibria, as the
following exercise illustrates.
✎ E XERCISE T7.8
Verify that the equilibria of the system
˙
x ⫽ y
˙
y ⫽ sin x ⫺ y (7.30)
are (n
, 0) for all integers n.
In order to determine the stability of an equilibrium v of (7.29), we use the
linear map that best approximates f at
v—namely, the Jacobian matrix Df(v)of
partial derivatives evaluated at
v. The Jacobian matrix Df(v) can be expected to
approximate f only in a small neighborhood of
v. Since f(v) ⫽ 0, the approxima-
tion f(
v ⫹
⑀
) ⬇ Df(v)
⑀
holds for small
⑀
. Start a solution w(t) ⫽ F(t, v ⫹
⑀
)from
an initial value
v ⫹
⑀
close to the equilibrium. Then u(t) ⫽ w(t) ⫺ v satisfies
˙u ⫽ ˙w ⫽ f(w(t)) ⫽ f(u(t) ⫹
v) ⬇ Df(v)u(t),
at least for short times. The solutions of (7.29) near
v move toward or away
from the equilibrium like the solutions of ˙u ⫽ Df(
v)u. The behavior of the latter
equation, which is linear, depends on the eigenvalues of Df(
v).
Definition 7.17 An equilibrium
v of ˙v ⫽ f(v) is called hyperbolic if
none of the eigenvalues of Df(
v) has real part 0.
When
v is hyperbolic, the linear part Df(v) completely determines the
stability of
v. When all eigenvalues of Df(v) have nonpositive real parts and
there is at least one eigenvalue with zero real part, then higher-order terms must
be taken into account to determine the stability; that is, it is not sufficient to know
298

7.4 NONLINEAR S YSTEMS
Df(v). Hyperbolic or not, v is unstable if the real part of at least one eigenvalue
of Df(
v) is strictly positive.
Theorem 7.18 Let
v be an equilibrium of ˙v ⫽ f(v). If the real part of each
eigenvalue of Df(
v) is strictly negative, then v is asymptotically stable. If the real part
of at least one eigenvalue is strictly positive, then
v is unstable.
E XAMPLE 7.19
We apply Theorem 7.18 to the following system:
˙
x ⫽ x
2
⫺ y
2
˙
y ⫽ xy ⫺ 4. (7.31)
There are two equilibria, (2, 2) and (⫺2, ⫺2). The Jacobian matrix is
Df ⫽
2x ⫺2y
yx
.
Evaluated at (2, 2), Df has eigenvalues 3 ⫾
7i. Since the real part of the
eigenvalues is ⫹3, (2, 2) is unstable. Evaluated at (⫺2, ⫺2), Df has eigenvalues
⫺3 ⫾
7i. Therefore, (⫺2, ⫺2) is asymptotically stable. A solution with initial
condition (x
0
,y
0
) sufficiently close to the equilibrium (⫺2, ⫺2) will tend to
(⫺2, ⫺2) as t →
⬁
. Note that Theorem 7.18 does not tell us how close (x
0
,y
0
)
must be to (⫺2, ⫺2) for the solution to converge to this equilibrium.
E XAMPLE 7.20
The one-dimensional equation
˙
x ⫽⫺x
3
(7.32)
has an equilibrium at x ⫽ 0. Since f(x) ⫽⫺x
3
is a function of one variable, Df is
the derivative f
(x). Since f
(0) ⫽ 0, we cannot use Theorem 7.18 to determine
the stability of x ⫽ 0.
✎ E XERCISE T7.9
Decide whether x ⫽ 0 is an asymptotically stable equilibrium of (7.32).
Solve by separating variables, as in Example 7.4. Does this equation have
unique solutions? Find all solutions that satisfy x(0) ⫽ 1.
299

D IFFERENTIAL E QUATIONS
7.5 MOTION IN A POTENTIAL FIELD
Perhaps the most familiar system that illustrates the concepts of kinetic and
potential energy is the pendulum equation
¨
x ⫹ k sin x ⫽ 0, (7.33)
where x is the angle of the pendulum rod from the vertical and k is the posi-
tive constant k ⫽ g l,wherel is the length of the pendulum rod and g is the
acceleration of gravity. We will set k ⫽ 1 to simplify our analysis.
We are assuming no damping and no external forces aside from gravity. This
equation can be rewritten as a first-order system with dependent variables x and
y by setting y ⫽
˙
x. Then
˙
y ⫽
¨
x ⫽⫺sin x, and (7.33) becomes
˙
x ⫽ y
˙
y ⫽⫺sin x. (7.34)
✎ E XERCISE T7.10
(a) Show that the equilibria of (7.34) are 兵(n
, 0) : n ⫽ 0, ⫾1, ⫾2,...其.
(b) Show that Theorem 7.18 identifies (n
, 0) as an unstable (saddle) equi-
librium if n is odd, but tells us nothing if n is even.
Taking a cue from mechanics, we use the principle of Conservation of
Energy: In the absence of damping or any external forces, the system neither
gains nor loses energy. Given an initial condition (x
0
,y
0
), the energy function E
remains constant on the orbit F(t, (x
0
,y
0
)) for all time t:
dE
dt
(F(t, (x
0
,y
0
))) ⫽ 0. (7.35)
Total energy is the sum of kinetic plus potential energies, which for the pendulum
is given by
E(x, y) ⫽ (1 2)y
2
⫹ 1 ⫺ cos x. (7.36)
The potential energy is minus the integral of the force,
x
0
sin udu(the work
required to raise the pendulum from angle 0 to angle x; we have set the mass
of the pendulum bob to 1). To verify (7.35), write (x(t),y(t)) for a solution and
300
7.5 MOTIONINAP OTENTIAL F IELD
compute
d
dt
E(x(t),y(t)) as
dE
dt
⫽
E
x
dx
dt
⫹
E
y
dy
dt
⫽ (sin x)
˙
x ⫹ y
˙
y
⫽ (sin x)y ⫹ y(⫺ sin x)
⫽ 0. (7.37)
Notice an interesting aspect of this analysis: We have completed this cal-
culation without knowing the solutions (x(t),y(t)). Equation (7.37) says that
d
dt
E(x(t),y(t)) ⫽ 0. We conclude in this case that for each solution E(x(t),y(t))
remains constant as t varies. The function E provides a useful partition of the
points (x, y) in the phase plane into individual solution trajectories.
Definition 7.21 Given a real number c and a function E : ⺢
2
−→ ⺢, the
set E
c
⫽ 兵(x, y):E(x, y) ⫽ c其 is called a level curve of the function E.
Notice that the minimum value of the energy function E is 0; hence, E
c
is
empty for c ⬍ 0. Some of the level curves of E are sketched in Figure 7.14(a).
x
(a)
(b)
x
x
x
Figure 7.14 Solution curves of the undamped pendulum.
(a) Level curves of the energy function. (b) The phase plane of the pendulum. The
solutions move along level curves; equilibria are denoted by dots. The variable x is
an angle, so what happens at x also happens at x ⫹ 2
. As a results, (a) and (b) are
periodic in x with period 2
.
301
D IFFERENTIAL E QUATIONS
Keeping in mind that a solution of (7.34) is constrained to one energy level,
we can turn the sketch of level curves into a phase plane of (7.34) merely by
putting arrows on the level curves to indicate the direction of motion, as in
Figure 7.14(b). Notice that solutions above the x-axis move from left to right,
and solutions below the x-axis move from right to left. Of particular interest are
the points in E
0
. These are precisely the equilibria whose stability could not be
determined by Theorem 7.18: (n
, 0), where n is even. In this case, the phase
plane shows us that these are stable equilibria, since the trajectories of nearby
points lie on closed level curves around the equilibria.
The pendulum equation (7.33) is a special case of the more general equation
¨
x ⫹
P
x
⫽ 0 (7.38)
governing motion in a potential field. This is another way of viewing Newton’s
second law of motion—acceleration is proportional to the force, which is the
negative of the gradient of the potential field. The potential energy field of the
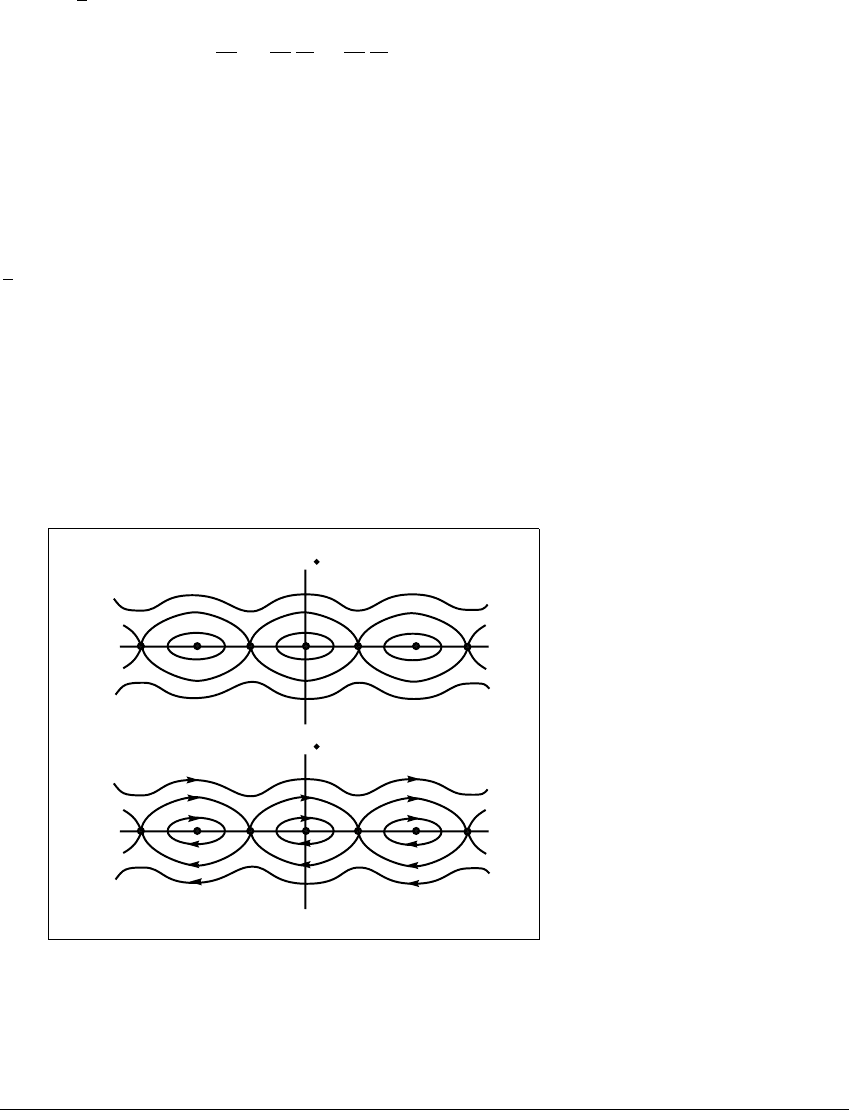
pendulum equation with k ⫽ 1 is a series of potential wells whose minima are
spaced at 2n
for all integers n, shown in Figure 7.15(a).
In the general case we multiply (7.38) by
˙
x and integrate both sides:
¨
x
˙
x ⫹
P
x
dx
dt
⫽ 0
1
2
˙
x
2
⫹ P(x) ⫽ E
1
(7.39)
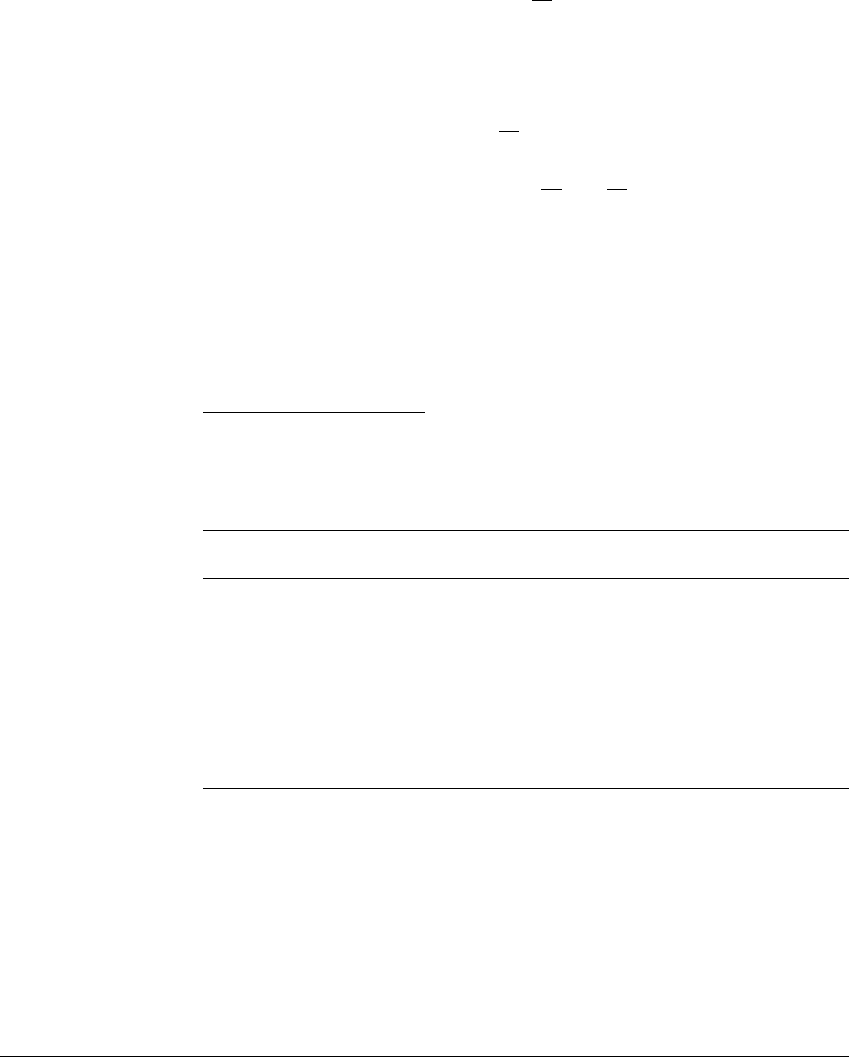
2-2
x
P(x)
-1
1
x
P(x)
(a) (b)
Figure 7.15 Potential energy functions.
(a) The potential function for the pendulum is P(x) ⫽ 1 ⫺ cos x. There are infinitely
many wells. (b) The double-well potential P(x) ⫽ x
4
4 ⫺ x
2
2.
302
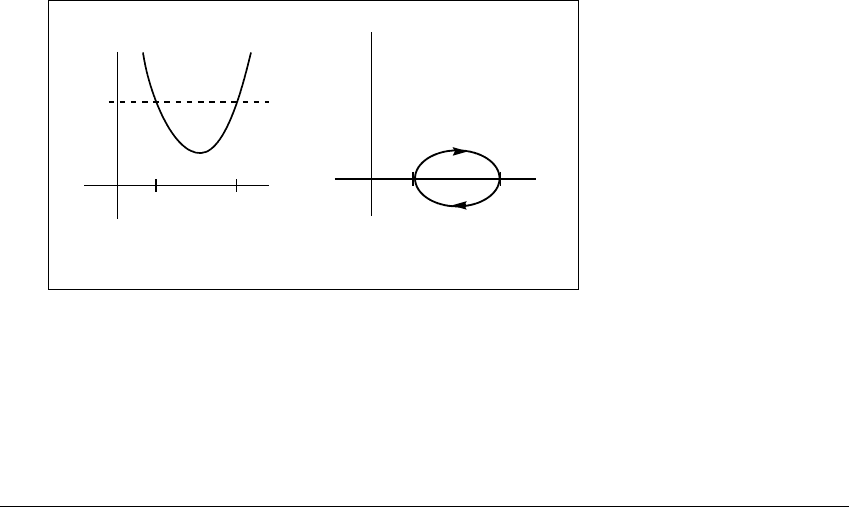
7.5 MOTIONINAP OTENTIAL F IELD
where E
1
is a constant of integration. This leads to a simple technique for drawing
phase plane solutions of motion in a potential field (7.38). The key is to follow
the difference between total and potential energy as x varies; this difference is the
kinetic energy
˙
x
2
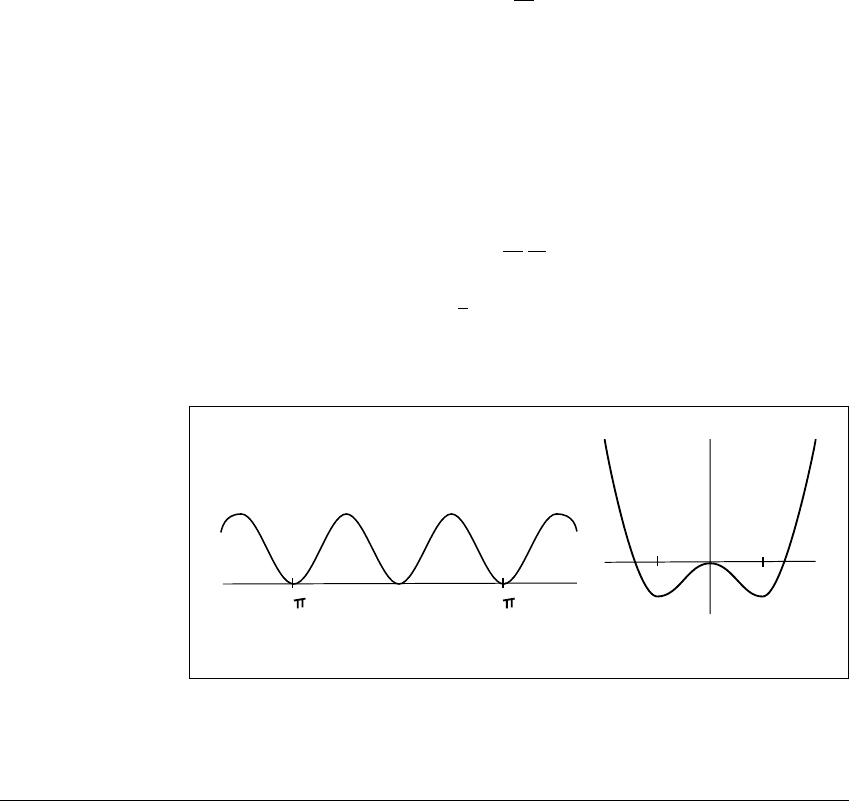
2. In Figure 7.16(a) we illustrate a typical single-well potential
energy function. According to (7.39), for a fixed energy level E
1
, the difference
E
1
⫺ P(x) is proportional to the square of the velocity
˙
x. As x increases from x
1
(where P ⫽ E
1
)tox
2
, the derivative
˙
x increases from 0 to some maximum value
(at the minimum of P) and then decreases to 0 at x
2
. The phase plane solution
at energy level E
1
is depicted in Figure 7.16(b). Solutions to single-well potential
problems in the absence of damping are periodic orbits.
Figure 7.15(b) shows the double-well potential P(x) ⫽ x
4
4 ⫺ x
2
2, which
by substitution in (7.38) leads to the double-well Duffing equation
¨
x ⫺ x ⫹ x
3
⫽ 0. (7.40)
Most of the solutions are periodic orbits. If the initial conditions (x,
˙
x) are set so
that the total energy E
1
⫽ P(x) ⫹
˙
x
2
2 is less than zero, then the orbit is trapped
in one of the two potential wells. If E
1
⬎ 0, orbits will move periodically through
both wells, reaching a maximum of P(x) ⫽ E
1
on the far sides of the wells.
As might be expected from our experiences with the pendulum equation
throughout this book, the Duffing equation becomes even more interesting if
P(x)
x
x
1
x
2
E
1
x
x
2
x
1
x
.
(a) (b)
Figure 7.16 Drawing phase plane curves from the potential.
(a) Graph of the potential energy function P(x). Each trajectory of the system is
trapped in a potential energy well. The total energy
˙
x
2
2 ⫹ P(x) is constant for
trajectories. As a trajectory with fixed total energy E
1
tries to climb out near x
1
or x
2
, the kinetic energy
˙
x
2
2 ⫽ E
1
⫺ P(x) goes to zero, as the energy E converts
completely into potential energy. (b) A periodic orbit results: The system oscillates
between positions x
1
and x
2
.
303
D IFFERENTIAL E QUATIONS
damping and periodic forcing are added. With damping, (7.38) becomes
¨
x ⫹ c
˙
x ⫹
P
x
⫽ 0. (7.41)
Energy is no longer conserved, for the time derivative of total energy E ⫽
˙
x
2
2 ⫹
P(x)is
˙
E ⫽
˙
x
¨
x ⫹
P
x
˙
x
⫽
˙
x
⫺c
˙
x ⫺
P
x
⫹
P
x
˙
x
⫽⫺c
˙
x
2
. (7.42)
Total energy decreases along orbits. Typical orbits will move progressively slower
and stop at the bottom of one of the two energy wells. See the Computer Experi-
ment 7.3 and Section 9.5 for the results of periodically forcing the Duffing double
well oscillator.
✎ E XERCISE T7.11
Not all orbits of (7.41) are attracted to the bottom of one of the wells.
Describe as many as you can that end up elsewhere.
➮ COMPUTER EXPERIMENT 7.3
Write a computer program to plot numerical solutions of the forced damped
double-well Duffing oscillator
¨
x ⫹ 0.1
˙
x ⫺ x ⫹ x
3
⫽ 2sint in the (x,
˙
x)-plane. In
particular, locate and plot the attracting periodic orbit of period 2
and the two
attracting periodic orbits of period 6
that lie in the region ⫺5 ⱕ x,
˙
x ⱕ 5.
7.6 LYAPUNOV FUNCTIONS
The basic idea of using energy-like functions to investigate the dynamics of
solutions can be applied to equations more general than (7.38). The theory
of Lyapunov functions, a generalization of potential energy functions, gives us
a global approach toward determining asymptotic behavior of solutions. The
main stability result of this chapter, Theorem 7.18, tells about local stability. In
304
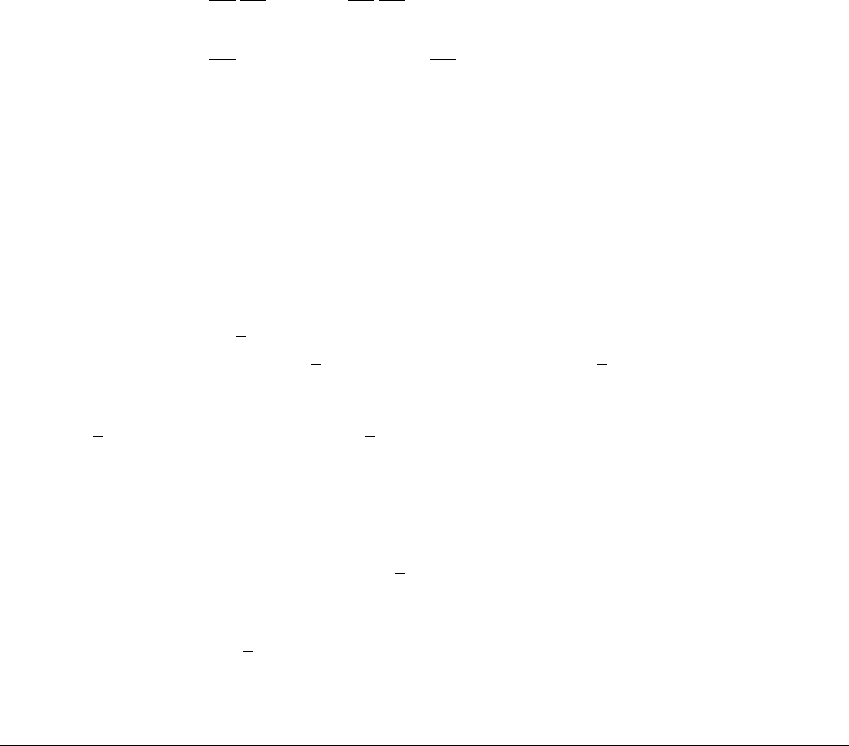
7.6 LYAP U N OV F UNCTIONS
the neighborhood of an equilibrium, solution trajectories are attracted to the
equilbrium if the eigenvalues of the linear part of the equation have negative
real part. Lyapunov functions can tell us that initial values from a large region
converge to an equilibrium. In addition, they can sometimes be used to determine
stability of equilibria where the eigenvalues of Df have real part 0, as in Example
7.20.
Let v(t) ⫽ (x
1
(t),...,x
n
(t)) be a solution of the n-dimensional system
(7.29) of differential equations. Suppose we pick a real-valued function of the
state, E(v), which we would like to consider to be the energy of the system when
it is in the state v. To measure the time rate of change of E along a solution
trajectory, we need to take the derivative of E with respect to t. Using the chain
rule and the differential equation, we find:
˙
E(x
1
,...,x
n
) ⫽
E
x
1
dx
1
dt
⫹⭈⭈⭈⫹
E
x
n
dx
n
dt
⫽
E
x
1
f
1
(x
1
,...,x
n
) ⫹⭈⭈⭈⫹
E
x
n
f
n
(x
1
,...,x
n
). (7.43)
That is, the derivative of E with respect to time can be expressed in terms of the
differential equation itself—the solutions do not explicitly appear in this formula.
The derivative
˙
E(v) measures the rate of change of E along a solution
trajectory of (7.29) as it passes through the point v. In the example of the
pendulum, we found
˙
E ⫽ 0 along trajectories. Using the total energy function
to determine the stability of equilibria for a conservative system (one in which
energy is conserved) is an example of the technique of Lyapunov functions.
Definition 7.22 Let
v be an equilibrium of (7.29). A function E : ⺢
n
→
⺢ is called a Lyapunov function for
v if for some neighborhood W of v,the
following conditions are satisfied:
1. E(
v) ⫽ 0, and E(v) ⬎ 0 for all v ⫽ v in W,and
2.
˙
E(v) ⱕ 0 for all v in W.
If the stronger inequality
2
.
˙
E(v) ⬍ 0 for all v ⫽ v in W
holds, then E is called a strict Lyapunov function.
Condition (1) says that
v is at the bottom of the well formed by the graph
of the Lyapunov function E, as shown in Figures 7.17(a) and (b). Condition (2)
says that solutions can’t move up, but can only move down the side of the well
305
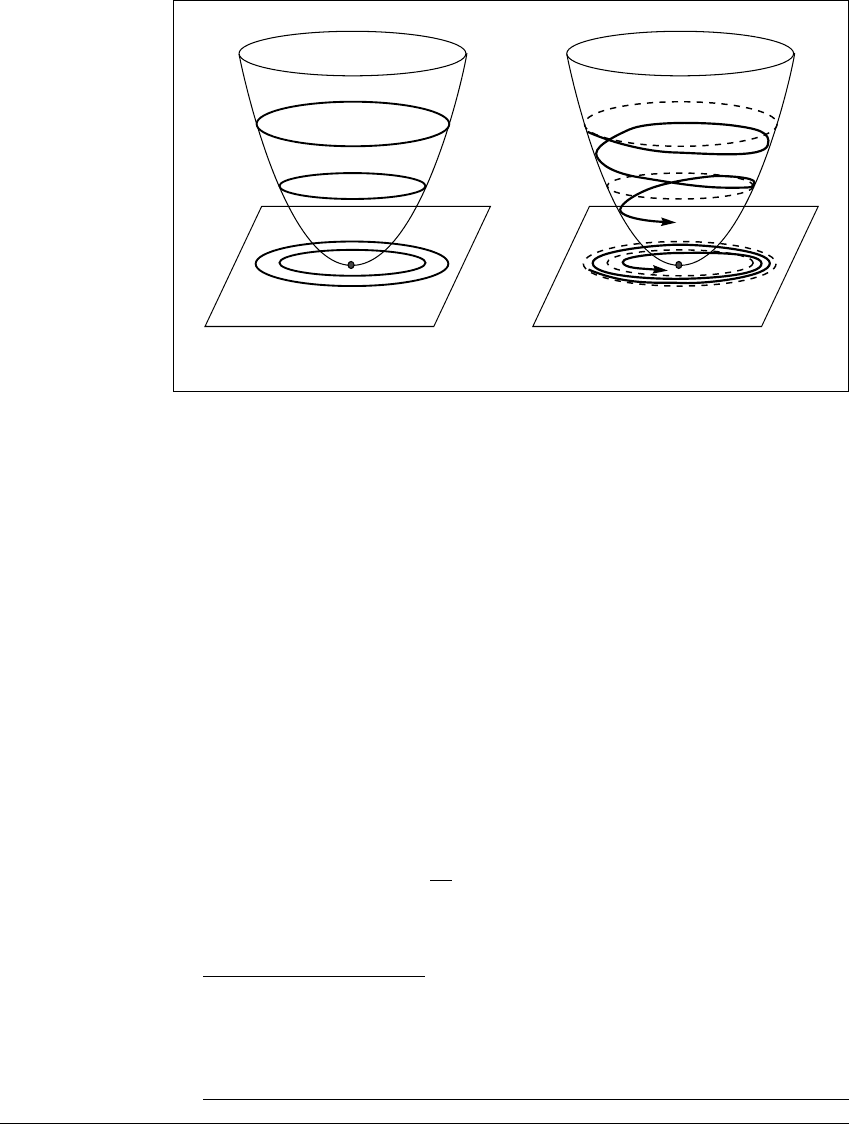
D IFFERENTIAL E QUATIONS
(a) (b)
Figure 7.17 Behavior of solution trajectories with a Lyapunov function.
The bowl in the figure is the graph of E. In both parts, we plot E (v(t)) versus the
solution trajectory v(t), which lies in the horizontal plane. (a) An equilibrium is
at the critical point of the graph of the Lyapunov function E. The equilibrium is
(Lyapunov) stable, since any nearby solution cannot go uphill, and can move away
only a bounded distance dictated by its original energy level. (b) For a strict Lya-
punov function, energy of solutions must continually decrease toward zero, cutting
through energy level sets. The equilibrium is asymptotically stable.
or stay level. Figure 7.17(b) shows a strict Lyapunov function, where energy must
keep decreasing toward the equilibrium.
In order to verify the conditions for a Lyapunov function, it is helpful to
use (7.43). For example, the one-dimensional equation
˙
x ⫽ ax,wherea ⬍ 0, has
the Lyapunov function E(x) ⫽ x
2
. It is clear that condition (1) is satisfied for the
equilibrium x ⫽ 0. Moreover, (7.43) shows
˙
E(x) ⫽
E
x
f(x) ⫽ (2x)(ax) ⫽ 2ax
2
⬍ 0,
which verifies (2’). Therefore E(x) ⫽ x
2
is a strict Lyapunov function.
✎ E XERCISE T7.12
Show that for each even integer n, total energy E(x, y) ⫽ (1 2)y
2
⫹ 1 ⫺ cos x
is a Lyapunov function for the equilibria (n
, 0) of (7.34).
306
