Alligood K., Sauer T., Yorke J.A. Chaos: An Introduction to Dynamical Systems
Подождите немного. Документ загружается.

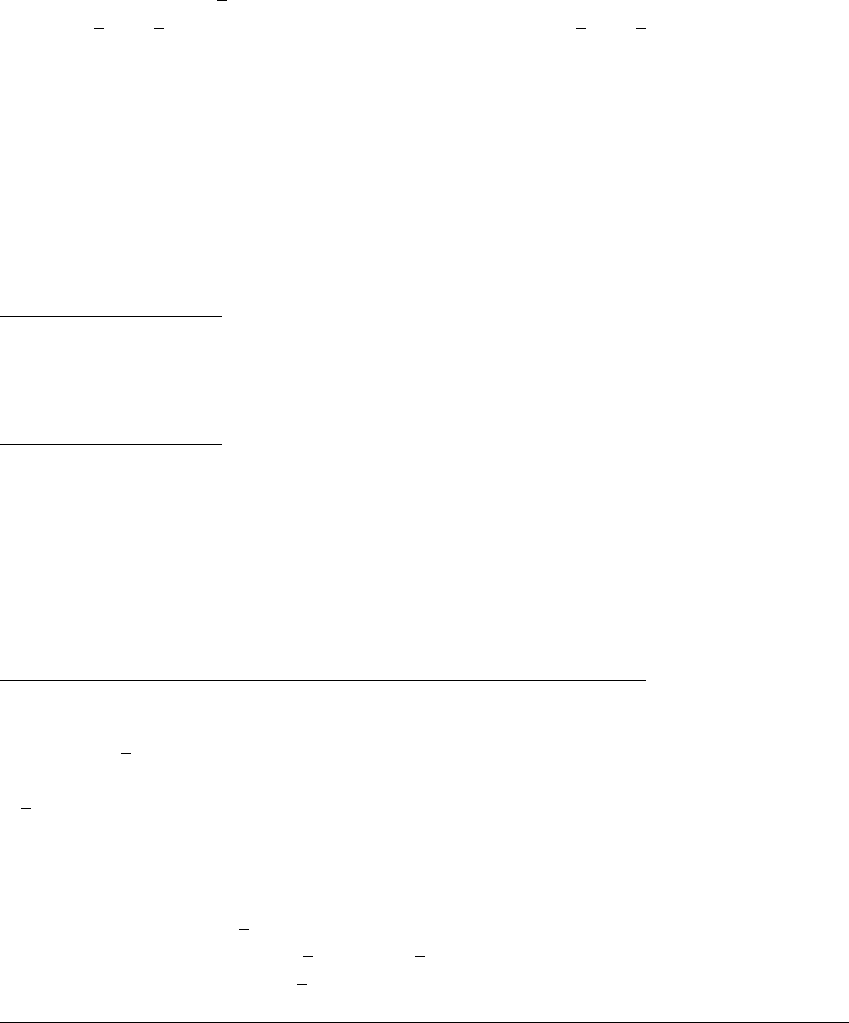
7.6 LYAP U N OV F UNCTIONS
The stability analysis illustrated above for the pendulum relies on Theorem
7.23, due to the Russian mathematician Alexander Mikhailovich Lyapunov.
Theorem 7.23 Let
v be an equilibrium of ˙v ⫽ f(v). If there exists a Lyapunov
function for
v, then v is stable. If there exists a strict Lyapunov function for v, then v
is asymptotically stable.
We refer the reader to (Hirsch and Smale, 1974) for a proof of the theorem.
Note that the energy function of Exercise T7.12 is not a strict Lyapunov function.
It cannot be, since the equilibria are not attracting.
We can now return to the one-dimensional Example 7.20:
˙
x ⫽⫺x
3
. Linear
analysis is inconclusive in determining the stability of the equilibrium x ⫽ 0. The
next exercise implies that x ⫽ 0 is asymptotically stable.
✎ E XERCISE T7.13
Show that E(x) ⫽ x
2
is a strict Lyapunov function for the equilibrium x ⫽ 0
in
˙
x ⫽⫺x
3
.
✎ E XERCISE T7.14
Let
˙
x ⫽⫺x
3
⫹ xy
˙
y ⫽⫺y
3
⫺ x
2
. (7.44)
Prove that (0,0) is an asymptotically stable equilibium of (7.44).
Figure 7.17(b) depicts bounded level curves of a strict Lyapunov function for
an equilibrium
v. Since E is strictly decreasing (as a function of t) along solutions,
the solutions (shown with arrows) cut through the level curves of E and converge
to
v. The sets W
c
⫽ 兵v 僆 W : E(v) ⱕ c其 can help us understand the extent of the
set of initial conditions whose trajectories converge to an asymptotically stable
equilibrium.
Definition 7.24 Let
v be an asymptotically stable equilibrium of ˙v ⫽
f(v). Then the basin of attraction of
v, denoted B(v), is the set of initial condi-
tions v
0
such that lim
t→
⬁
F(t, v
0
) ⫽ v.
307

D IFFERENTIAL E QUATIONS
Note that any set W on which V is a strict Lyapunov function for v (as in
Definition 7.22) will be a subset of the basin B(
v).
✎ E XERCISE T7.15
Show that the basin of attraction of the equilibrium (0, 0) for the system
(7.44) is ⺢
2
.
A somewhat weaker notion of containment than a basin is that of a “trap-
ping region”—a set in ⺢
n
where solutions, once they enter, cannot leave as time
increases.
Definition 7.25 AsetU 傺 ⺢
n
is called a forward invariant set for (7.29)
if for each v
0
僆 U, the forward orbit 兵F(t, v
0
):t ⱖ 0其 is contained in U. A forward
invariant set that is bounded is called a trapping region. We also require that a
trapping region be an n-dimensional set.
✎ E XERCISE T7.16
Let E be a Lyapunov function on W,letc be a positive real number, and let
W
c
⫽ 兵v 僆 W : E(v) ⱕ c其.
(a) Show that, for each c, W
c
is a forward invariant set. (b) Show that if in
addition E(v) →
⬁
as |v| →
⬁
,thenW
c
is a trapping region.
Returning to the pendulum example, we reconsider the system under the
effects of a damping force, such as air resistance. Once the pendulum is set into
motion, it will lose energy and eventually come to rest. We assume that the
damping force is proportional to, but opposite in direction from, the velocity of
the pendulum, so that the motion of the pendulum is governed by the equation
¨
x ⫹ b
˙
x ⫹ sin x ⫽ 0, (7.45)
for a constant b ⬎ 0.
From our observations, we would like to be able to conclude that what
were stable equilibria for the undamped pendulum are now asymptotically stable
and attract nearby initial conditions. The fact these equilibria are asymptotically
stable follows from evaluating the Jacobian (check this); however, we are not
able to conclude from the local analysis that all trajectories converge to an
equilibrium. Understanding the technique of Lyapunov functions for conservative
308
7.7 LOTKA-VOLTERRA M ODELS
systems, such as the undamped pendulum, enabled us to draw the phase plane
for this system and view it globally. In that case we were able to understand
the motions for all initial conditions. Unfortunately, total energy is not a strict
Lyapunov function for any equilibria of the damped system. Instead, we compute
˙
E(v) ⫽⫺by
2
, so that the inequality
˙
E(v) ⬍ 0 fails to be strict for points arbitrarily
near the equilibrium.
Notice that trajectories (other than equilibrium points) do not stay on
the x-axis, the set on which
˙
E ⫽ 0. Therefore we might expect trajectories to
behave as if E were a strict Lyapunov function. The following corollary, often
called “LaSalle’s Corollary” to the Lyapunov Theorem 7.23, not only provides
another means of deducing asymptotic stability for equilibria of the damped
system, but also gives information as to the extent of the basin of attraction for
each asymptotically stable equilibrium. We postpone the proof of the theorem
until Chapter 8 when we study limit sets of trajectories.
Corollary 7.26 (Barbashin-LaSalle) Let E be a Lyapunov function for
v
on the neighborhood W, as in Definition 7.22. Let Q ⫽ 兵v 僆 W :
˙
E(v) ⫽ 0其.
Assume that W is forward invariant. If the only forward-invariant set contained
completely in Q is
v, then v is asymptotically stable. Furthermore, W is contained
in the basin of
v; that is, for each v
0
僆 W, lim
t→
⬁
(F(t, v
0
)) ⫽ v.
✎ E XERCISE T7.17
Let
¨
x ⫹ b
˙
x ⫹ sin x ⫽ 0, for a constant b ⬎ 0. (a) Convert the differential
equation to a first-order system. (b) Use Corollary 7.26 to show that the
equilibria (n
, 0), for even integers n are asymptotically stable. (c) Sketch
the phase plane for the associated first-order system.
7.7 LOTKA-VOLTERRA MODELS
A family of models called the Lotka-Volterra equations are often used to simulate
interactions between two or more populations. Interactions are of two types.
Competition refers to the possibility that an increase in one population is bad for
the other populations: an example would be competition for food or habitat. On
the other hand, sometimes an increase in one population is good for the other.
Owls are happy when the mouse population increases. We will consider two cases
of Lotka-Volterra equations, called competing species models and predator-prey
models.
309
D IFFERENTIAL E QUATIONS
We begin with two competing species. Because of the finiteness of resources,
the reproduction rate per individual is adversely affected by high levels of its own
species and the other species with which it is in competition. Denoting the two
populations by x and y, the reproduction rate per individual is
˙
x
x
⫽ a(1 ⫺ x) ⫺ by, (7.46)
where the carrying capacity of population x is chosen to be 1 (say, by adjusting
our units). A similar equation holds for the second population y,sothatwehave
the competing species system of ordinary differential equations
˙
x ⫽ ax(1 ⫺ x) ⫺ bxy
˙
y ⫽ cy(1 ⫺ y) ⫺ dxy (7.47)
where a, b, c, and d are positive constants. The first equation says the population
of species x grows according to a logistic law in the absence of species y (i.e.,
when y ⫽ 0). In addition, the rate of growth of x is negatively proportional to xy,
representing competition between members of x and members of y. The larger
the population y, the smaller the growth rate of x. The second equation similarly
describes the rate of growth for population y.
The method of nullclines is a technique for determining the global behavior
of solutions of competing species models. This method provides an effective means
of finding trapping regions for some differential equations. In a competition model,
if a species population x is above a certain level, the fact of limited resources will
cause x to decrease. The nullcline, a line or curve where
˙
x ⫽ 0, marks the boundary
between increase and decrease in x. The same characteristic is true of the second
species y, and it has its own curve where
˙
y ⫽ 0. The next two examples show that
the relative orientation of the x and y nullclines determines which of the species
survives.
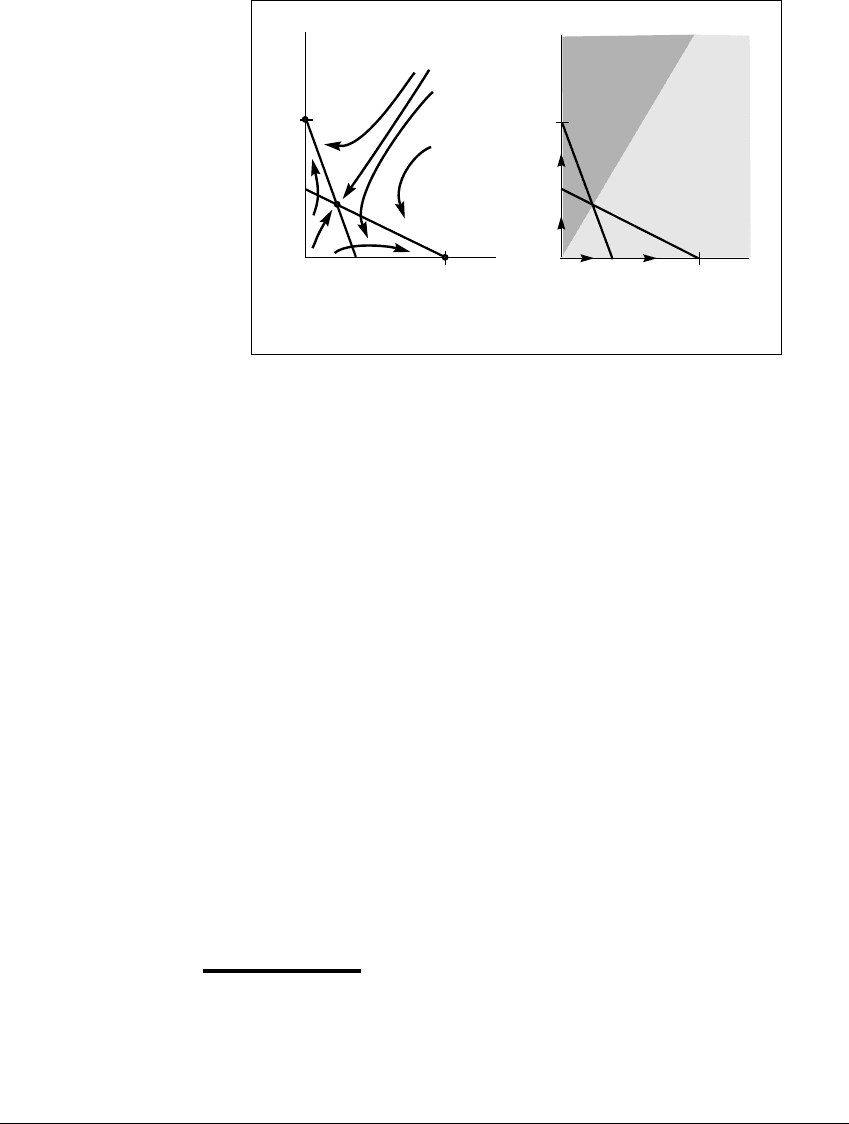
E XAMPLE 7.27
(Species extinction) Set the parameters of (7.47) to be a ⫽ 1, b ⫽ 2, c ⫽ 1,
and d ⫽ 3. To construct a phase plane for (7.47), we will determine four regions
in the first quadrant: sets for which (I)
˙
x ⬎ 0and
˙
y ⬎ 0; (II)
˙
x ⬎ 0and
˙
y ⬍ 0;
(III)
˙
x ⬍ 0and
˙
y ⬎ 0; and (IV)
˙
x ⬍ 0and
˙
y ⬍ 0. Other assumptions on a, b, c,
and d lead to different outcomes, but can be analyzed similarly.
In Figure 7.18(a) we show the line along which
˙
x ⫽ 0, dividing the plane
into two regions: points where
˙
x ⬎ 0 and points where
˙
x ⬍ 0. Analogously,
Figure 7.18(b) shows regions where
˙
y ⬎ 0and
˙
y ⬍ 0, respectively. Combining
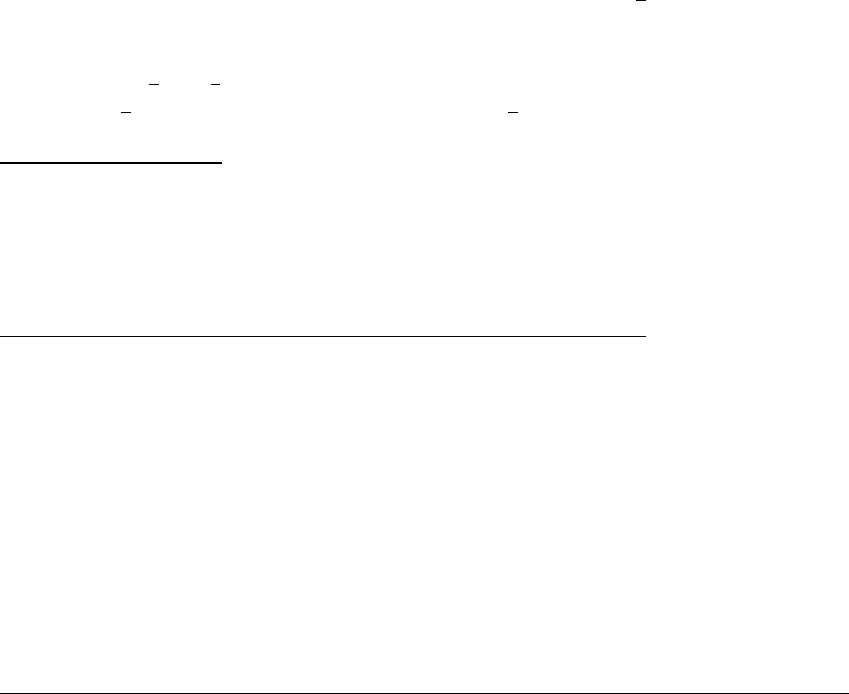
the information from these two figures, we indicate regions (I)–(IV) (as described
above) in Figure 7.19(a). Along the nullclines (lines on which either
˙
x ⫽ 0or
310
7.7 LOTKA-VOLTERRA M ODELS
x
1
1
y
x = 0
x < 0
x > 0
.
.
.
x
1
1
y
y < 0
y > 0
y = 0
.
.
.
(a) (b)
Figure 7.18 Method of nullclines for competing species.
The straight line in (a) shows where
˙
x ⫽ 0, and in (b) it shows where
˙
y ⫽ 0for
a ⫽ 1, b ⫽ 2, c ⫽ 1, and d ⫽ 3 in (7.47). The y-axis in (a) is also an x-nullcline,
and the x-axis in (b) is a y-nullcline.
x
1
1
y
I
II
III
IV
Figure 7.19 Competing species: Nullclines.
The vectors show the direction that trajectories move. The nullclines are the lines
along which either
˙
x ⫽ 0or
˙
y ⫽ 0. In this figure, the x-axis, the y-axis, and the two
crossed lines are nullclines. Triangular regions II and III are trapping regions.
311
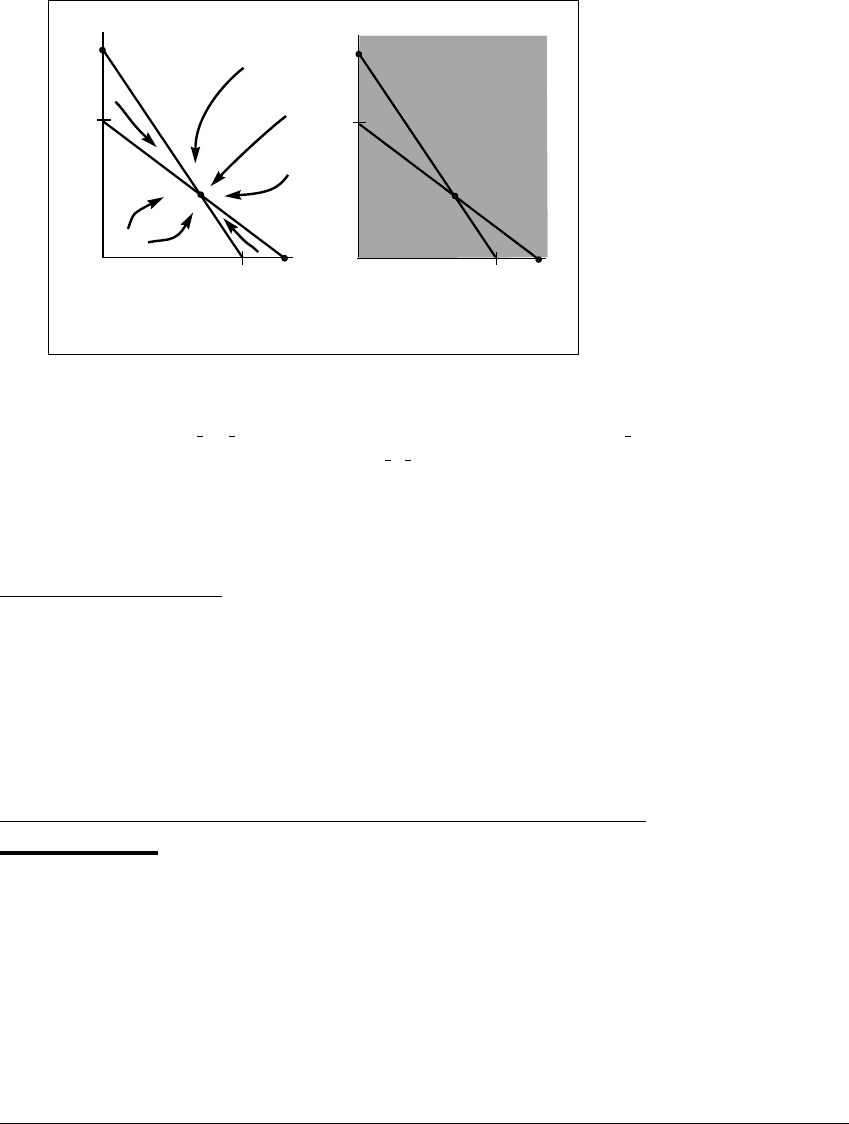
D IFFERENTIAL E QUATIONS
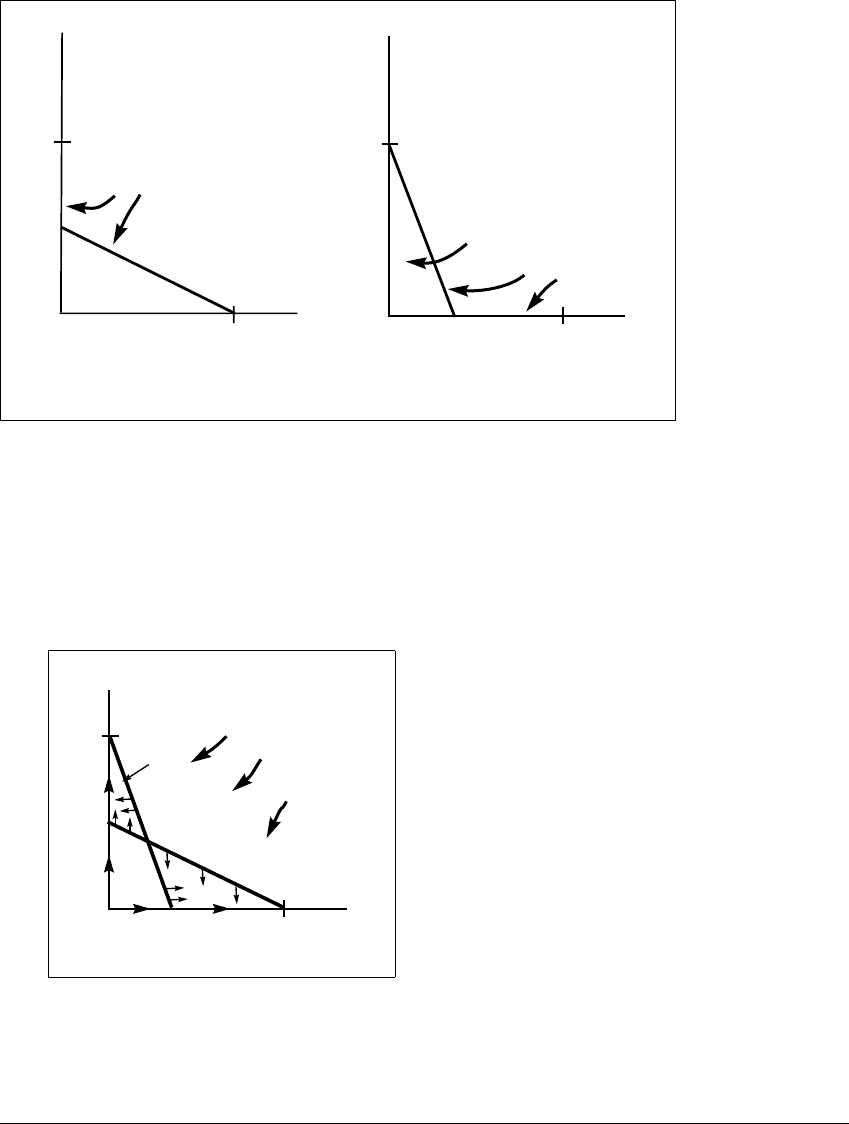
x
1
1
y
x
1
1
y
(a) (b)
Figure 7.20 Competing species, extinction.
(a) The phase plane shows attracting equilibria at (1, 0) and (0, 1), and a third,
unstable equilibrium at which the species coexist. (b) The basin of (0, 1) is shaded,
while the basin of (1, 0) is the unshaded region. One or the other species will die
out.
˙
y ⫽ 0), arrows indicate the direction of the flow: left/right where
˙
y ⫽ 0 or up/down
where
˙
x ⫽ 0. Notice that points where the two different types of nullclines cross
are equilibria. There are four of these points. The equilibrium (0, 0) is a repellor;
(1 5, 2 5) is a saddle; and (1, 0) and (0, 1) are attractors.
The entire phase plane is sketched in Figure 7.20(a). Almost all orbits
starting in regions (I) and (IV) move into regions (II) and (III). (The only
exceptions are the orbits that come in tangent to the stable eigenspace of the
saddle (1 5, 2 5). These one-dimensional curves form the “stable manifold” of
the saddle and are discussed more fully in Chapter 10.) Once orbits enter regions
(II) and (III), they never leave. Within these trapping regions, orbits follow the
direction indicated by the derivative toward one or the other asymptotically stable
equilibrium. The basins of attraction of the two possibilities are shown in Figure
7.20(b). The stable manifold of the saddle forms the boundary between the basin
shaded gray and the unshaded basin. We conclude that for almost every choice
of initial populations, one or the other species eventually dies out.
E XAMPLE 7.28
(Coexistence) Set the parameters to be a ⫽ 3, b ⫽ 2, c ⫽ 4, and d ⫽ 3.
The nullclines are shown in Figure 7.21(a). In this case there is a steady state at
(x, y) ⫽ (2 3, 1 2) which is attracting. The basin of this steady state includes
312
7.7 LOTKA-VOLTERRA M ODELS
x
1
1
y
x
1
1
y
(a) (b)
Figure 7.21 Competing species, coexistence.
(a) The phase plane shows an attracting equilibrium in which both species survive.
The x-nullcline y ⫽
3
2
⫺
3
2
x has smaller x-intercept than the y-nullcline y ⫽ 1 ⫺
3
4
x.
According to Exercise T7.18, the equilibrium (
2
3
,
1
2
) is asymptotically stable. (b) All
initial conditions with x ⬎ 0andy ⬎ 0 are in the basin of this equilibrium.
the entire first quadrant, as shown in Figure 7.21(b). Every set of nonzero starting
populations moves toward this equilibrium of coexisting populations.
✎ E XERCISE T7.18
Consider the general competing species equation (7.47) with positive pa-
rameters a, b, c, d. (a) Show that there is an equilibrium with both popu-
lations positive if and only if either (i) both a b and c d are greater than
one, or (ii) both a b and c d are less than one. (b) Show that a positive
equilibrium in (a) is asymptotically stable if and only if the x-intercept of the
x-nullcline is less than the x-intercept of the y-nullcline.
E XAMPLE 7.29
(Predator-Prey) We examine a different interaction between species in this
example in which one population is prey to the other. A simple model of this
interaction is given by the following equations:
˙
x ⫽ ax ⫺ bxy
˙
y ⫽⫺cy ⫹ dxy (7.48)
where a, b, c,andd are positive constants.
313
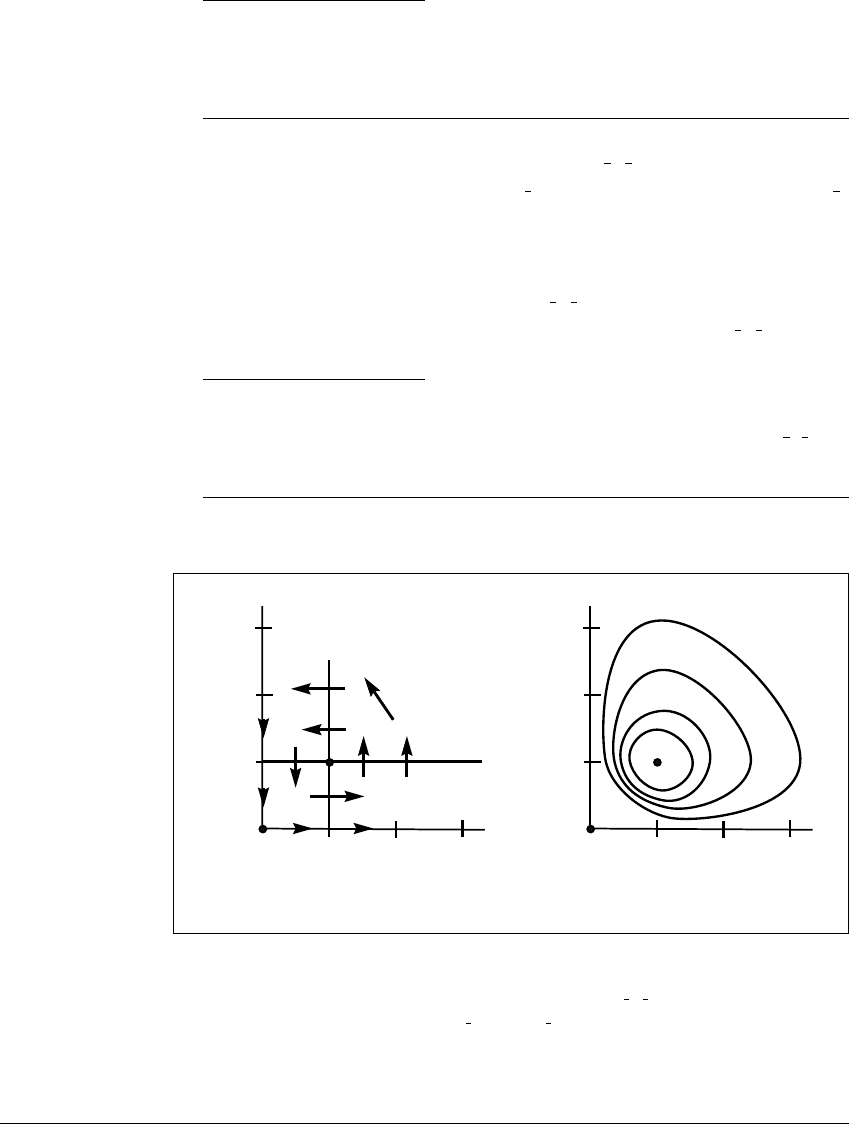
D IFFERENTIAL E QUATIONS
✎ E XERCISE T7.19
Explain the contribution of each term, positive or negative, to the predator-
prey model (7.48).
System (7.48) has two equilibria, (0, 0) and (
c
d
,
a
b
). There are also nullclines;
namely,
˙
x ⫽ 0whenx ⫽ 0orwheny ⫽
a
b
, and
˙
y ⫽ 0wheny ⫽ 0orwhenx ⫽
c
d
.
Figure 7.22(a) shows these nullclines together with an indication of the flow
directions in the phase plane.
Unlike previous examples, there are no trapping regions. Solutions appear
to cycle about the nontrivial equilibrium (
c
d
,
a
b
). Do they spiral in, spiral out, or
are they periodic? First, check the eigenvalues of the Jacobian at (
c
d
,
a
b
).
✎ E XERCISE T7.20
Find the Jacobian Df for (7.48). Verify that the eigenvalues of Df(
c
d
,
a
b
)are
pure imaginary.
a/b
c/d
y
x
a/b
c/d
y
x
(a) (b)
Figure 7.22 Predator-prey vector field and phase plane.
(a) The vector field shows equilibria at (0, 0) and (
c
d
,
a
b
). Nullclines are the x-axis,
the y-axis, and the lines x ⫽
c
d
and y ⫽
a
b
. There are no trapping regions. (b) The
curves shown are level sets of the Lyapunov function E.Since
˙
E ⫽ 0, solutions
starting on a level set must stay on that set. The solutions travel periodically around
the level sets in the counterclockwise direction.
314

7.7 LOTKA-VOLTERRA M ODELS
Since the system is nonlinear and the eigenvalues are pure imaginary, we can
conclude nothing about the stability of (
c
d
,
a
b
). Fortunately, we have a Lyapunov
function.
✎ E XERCISE T7.21
Let
E(x, y) ⫽ dx ⫺ c ln x ⫹ by ⫺ a ln y ⫹ K,
where a, b, c, and d are the parameters in (7.48) and K is a constant. Ver-
ify that
˙
E ⫽ 0 along solutions and that E is a Lyapunov function for the
equilibrium (
c
d
,
a
b
).
We can conclude that (
c
d
,
a
b
) is stable. Solutions of (7.48) lie on level
curves of E. Since (
c
d
,
a
b
) is a relative minimum, these level curves are closed
curves encircling the equilibrium. See Figure 7.22(b). Therefore, solutions of this
predator-prey system are periodic for initial conditions near (
c
d
,
a
b
). In fact, every
initial condition with x and y both positive lies on a periodic orbit.
315

D IFFERENTIAL E QUATIONS
☞ C HALLENGE 7
A Limit Cycle in the Van der Pol System
T
HE LIMITING BEHAVIOR we have seen in this chapter has consisted largely
of equilibrium states. However, it is common for solutions of nonlinear equations
to approach periodic behavior, converging to attracting periodic orbits, or limit
cycles. The Van der Pol equation
¨
x ⫹ (x
2
⫺ 1)
˙
x ⫹ x ⫽ 0 (7.49)
is a model of a nonlinear electrical circuit that has a limit cycle.
Defining y ⫽
˙
x, the second-order equation is transformed to
˙
x ⫽ y
˙
y ⫽⫺x ⫹ (1 ⫺ x
2
)y. (7.50)
The origin (0, 0) is the only equilibrium of (7.50), and it is unstable. In this
Challenge, you will show that all other trajectories of the system approach a
single attracting periodic orbit that encircles the origin. This type of limiting
behavior for orbits is a phenomenon of nonlinear equations. Although linear
systems may have periodic orbits, they do not attract initial values from outside
the periodic orbit.
We begin by introducing a change of coordinates. Let z ⫽ y ⫹ F(x), where
F(x) ⫽
x
3
3
⫺ x.
Step 1 Show that the correspondence (x, y) → (x, z) is one-to-one (and
therefore a change of coordinates), and that the system (7.50) is transformed to
the following system:
˙
x ⫽ z ⫺
x
3
3
⫺ x
˙
z ⫽⫺x. (7.51)
Step 2 Draw a phase plane for the system (7.51), indicating the approx-
imate direction of the flow. (Hint: Begin with Figure 7.23.) Argue that starting
from a point on the positive z-axis, denoted z
⫹
, a solution v(t) ⫽ (x(t),z(t)) will
go into region I until it intersects the branch F
⫹
(the graph of F(x) for positive x),
316
