Baumgarte T., Shapiro S. Numerical Relativity. Solving Einstein’s Equations on the Computer

Подождите немного. Документ загружается.

Gravitational waves

Spherically symmetric spacetimes, which we discussed in Chapter 8, do not admit gravi-

tational radiation. Once we relax this symmetry restriction, as we shall do in the following

chapters, we will encounter spacetimes that do contain gravitational radiation. In fact,

simulating promising sources of gravitational radiation and predicting their gravitational

wave signals are among the most important goals of numerical relativity. These goals are

especially urgent in light of the new generation of gravitational wave laser interferometers

which are now operational. A book on numerical relativity therefore would not be complete

without a discussion of gravitational waves.

In this chapter we review several topics related to gravitational waves. We start in

Section 9.1 with a discussion of linearized waves propagating in nearly Minkowski space-

times and the role that these waves play even in the case of nonlinear sources of gravitational

radiation. In Section 9.2 we survey plausible sources of gravitational waves, highlighting

those that seem most promising from the perspective of gravitational wave detection. We

brieﬂy describe some of the existing and planned gravitational wave detectors in Sec-

tion 9.3. Finally, in Section 9.4 we make contact with numerical relativity, and review

different strategies that have been employed to extract gravitational radiation data from

numerical relativity simulations.

9.1 Linearized waves

Most of this book deals with strong-ﬁeld solutions of Einstein’s equations, including black

holes, neutron stars, and binaries containing these objects. As long as these solutions

are dynamical and nonspherical, they will emit gravitational radiation. In the near-ﬁeld

region of such sources, the gravitational ﬁelds consist of a combination of longitudinal and

transverse (i.e., radiative) components that cannot be disentangled unambigiously. As the

transverse ﬁelds propagate away from their sources, however, they will reach an asymptotic

region in which they can be modeled as a linear perturbation of a nearly Minkowski

spacetime. These linearized gravitational waves carry information about the nature of the

nonlinear sources that generated them. It is these linearized waves that are measured by

gravitational wave detectors. The goal in simulating astrophysically promising sources

of gravitational radiation is therefore to predict the emitted gravitational waveforms that

reach this asymptotic regime. We will discuss this numerical “extraction” of gravitational

311

312 Chapter 9 Gravitational waves

waveforms in Section 9.4. Before doing so, however, we begin with a review of some of

the basic properties of linearized gravitational waves.

9.1.1 Perturbation theory and the weak-ﬁeld, slow-velocity regime

A detailed discussion and derivation of the generation and propagation of gravitational

waves in the weak-ﬁeld, slow-velocity regime of general relativity can be found in any of

the standard textbooks on general relativity. We have already provided a brief summary in

Chapter 1.1; here we will review the main results and use them in several applications.

Consider a small perturbation h

of a known “background” solution to Einstein’s

equations. In principle the background could be any solution, but here we are interested in

waves propagating in a nearly Minkowski spacetime, for which the metric becomes

= η

+ h

, |h

|1. (9.1)

It is convenient to introduce the “trace-reversed” perturbation

≡ h

−

. (9.2)

We can now exploit our coordinate freedom to impose the “Lorentz gauge” condition,

∇

= 0, (9.3)

in which case Einstein’s equations in vacuum reduce to the wave equation



≡∇

∇

= 0 (vacuum). (9.4)

As it turns out, the Lorentz-gauge condition (9.3) does not determine

uniquely,

since we can introduce further inﬁnitesimal gauge transformations that leave this condi-

tion unchanged. We can therefore use this remaining gauge freedom to impose further

conditions on the perturbations

. Particularly useful is the transverse-traceless or “TT”

gauge, in which

= 0,

TTa

= 0. (9.5)

The ﬁrst condition implies that the only nonzero components of

are purely spatial.

The second condition implies that h

= 0, so that, according to equation (9.2), the trace-

reversed metric perturbations

are identical to the original perturbations h

, and we are

entitled to drop the bars whenever we write down results in the TT gauge. In the TT gauge

the time-space components of the 4-dimensional Riemann tensor are simply expressed in

terms of the metric perturbations:

(4)

i0 j 0

=−

. (9.6)

Departing from the notation in most of the rest of the book we shall adopt Cartesian coordinates here, whereby

= diag(−1, 1, 1, 1).

9.1 Linearized waves 313

× Polarization

+ Polarization

Figure 9.1 Lines of force associated with the two polarization states h

and h

of a linear plane gravitational

wave traveling in vacuum in the z-direction. [From Abramovici et al. (1992).]

Here the double dot denotes the second time derivative. Equation (9.6) exhibits the special

role that the metric perturbations in the TT gauge play in providing a direct measure of the

Riemann tensor, which determines the gravitational tidal ﬁeld. Together, the conditions

(9.3) and (9.5) provide eight constraints on the originally ten independent components of

The remaining two degrees of freedom correspond to the two possible polarization

states of gravitational radiation. It is often convenient to express these two polarization

states in terms of the two polarization tensors e

and e

. For a linear plane wave propa-

gating in vacuum in the z-direction, for example, we have

=−e

= 1, e

= e

= 1, all other components zero. (9.7)

A general gravitational wave is then speciﬁed by two dimensionless amplitudes h

and h

= h

+ h

. (9.8)

The effect of a passing gravitational wave on two nearby, freely-falling test particles at

a spatial separation ξ

is to change their separation according to

. (9.9)

Equation (9.9) is a direct consequence of the equation of geodesic deviation applied to the

two particles, combined with equation (9.6). The relative strain δξ/ξ between these two

particles is therefore proportional to the gravitational wave amplitude, which explains why

is sometimes called the gravitational wave strain. In Figure 9.1 we show the lines of

force associated with the two polarization states h

and h

. For the h

polarization, for

example, particles separated along the x-direction (with coordinate axes chosen as in the

Superﬁcially, it appears as if the conditions (9.3)and(9.5) provided nine conditions, not eight. However, one of the

four equations

= 0in(9.5) is redundant with one of the conditions in (9.3), reducing the number of conditions

to eight. See, for example, Section 35.4 in Misner et al. (1973) for a more detailed discussion.

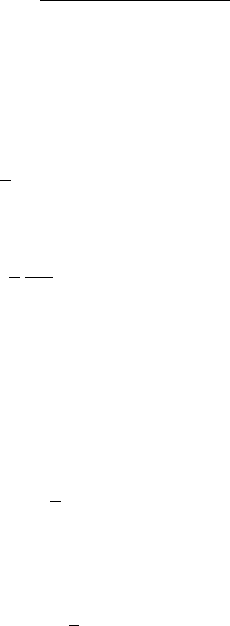
314 Chapter 9 Gravitational waves

ﬁgure), will be pulled apart while particles along the y-direction are pushed together, and

vice versa a half-cycle later.

Consider now the generation of gravitational radiation from a weak-ﬁeld, slow-velocity

source.

For such a source, Einstein’s equations reduce to



=∇

∇

=−16π T

(weak-ﬁeld, slow-velocity). (9.10)

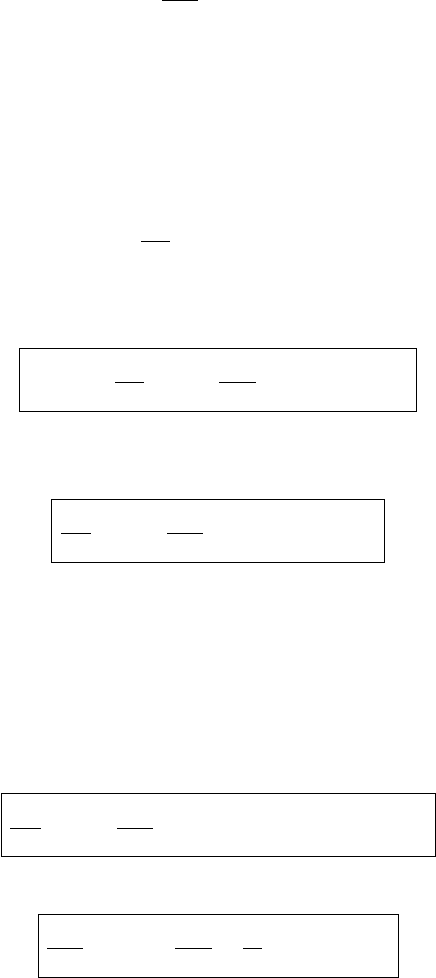
Imposing an outgoing-wave boundary condition, we can solve equation (9.10) with the

help of a Green function to obtain the integral equation,

(t, x

) = 4





(t −|x

− x

i

|, x

i

)

− x

i

. (9.11)

Here t −|x

− x

i

| is the retarded time; it implies that

at the event (t, x

) depends on

the stress-energy tensor T

on the event’s past light cone.

In the wave zone at large distances r from the source we may expand the retarded integral

in equation (9.11)inpowersofx

i

/r to ﬁnd

(t, x

) =





(t − r, x

k

). (9.12)

We now use the tensor virial theorem









i

j

, (9.13)

together with the expression T

≈ ρ

, valid for Newtonian sources, and the deﬁnition of

the second moment of the mass distribution,

(t) ≡





(t, x

k

i

j

, (9.14)

to rewrite (9.12)as

(t, x

) =

(t − r ). (9.15)

It is then convenient to deﬁne the reduced quadrupole moment as the traceless part of the

second moment of the mass distribution,

≡ I

−

I, (9.16)

where I = I

. The reduced quadrupole moment has a more transparent physical meaning

than the second moment of the mass distribution, since it appears in the near-zone expansion

Weak-ﬁeld means   1, where  is the Newtonian potential of the source; slow-velocity means v  1, where v is

the characteristic speed of the source and its internal components.

In what follows, adopting the weak-ﬁeld, slow-velocity limit allows us to neglect the stress-energy pseudotensor

that must be added to T

on the right hand side of equation (9.10) in the general case; see Misner et al. (1973),

Section 36.10.

This can be proven by using ∂

=−∂

∂

= ∂

∂

(a consequence of successive application of the conservation

of energy-momentum in the weak-ﬁeld limit, ∂

= 0) and repeated integration by parts.

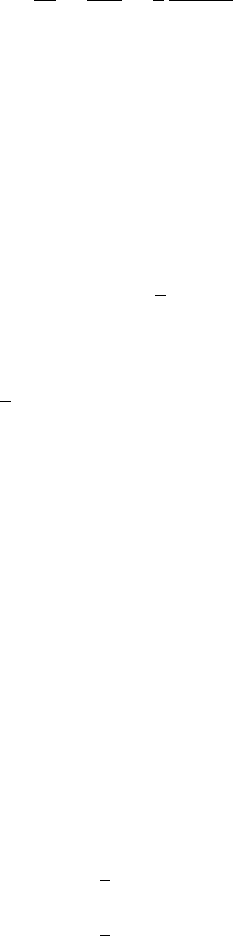
9.1 Linearized waves 315

of the Newtonian gravitational potential,

 =−



+ ...



. (9.17)

Here M is the total mass of the system, and d

is its dipole moment.

Finally, to bring equation (9.15) into the TT gauge, we need to project out its transverse-

traceless part. Using the projection operator

≡ η

− n

, (9.18)

where n

= x

/r is a unit vector pointing in the wave’s local direction of propagation, this

can be accomplished by deﬁning

≡



−



. (9.19)

We t hen h ave

(t, x

) =

(t − r ) (weak-ﬁeld, slow-velocity). (9.20)

since the TT parts of I

and I

are identical. We emphasize that equation (9.20), referred to

as the “quadrupole approximation”, holds only for a weak-ﬁeld, slow-velocity source. But a

majority of the most promising sources of detectable gravitational waves are characterized

by strong ﬁelds and high velocities, for which numerical relativity is required to determine

the waveforms. Nevertheless, equation (9.20) often provides a useful ﬁrst approximation

even in these cases. It also serves as a good check on numerical calculations in the

appropriate limit.

Exercise 9.1 A distant observer at (r,θ,φ) in the wave zone measures a gravitational

wave from a radiating source at the origin. Decompose the waveform into its two

polarization modes as in equation (9.8), whereby the 3-metric in the wave zone may

be written

= dr

+ (1 + h

dθ

+ (1 − h

)sin

θdφ

+ 2h

sinθdθ dφ. (9.21)

(a) Show that the two polarization modes are related to



according to

(

−

), (9.22)

, (9.23)

where



are the components of the reduced quadrupole moment in an orthonormal

basis (e

, e

(b) Show that if we relate spherical polar coordinates to Cartesian coordinates

(x, y, z) in the usual way, the two polarization modes can be expressed in terms of

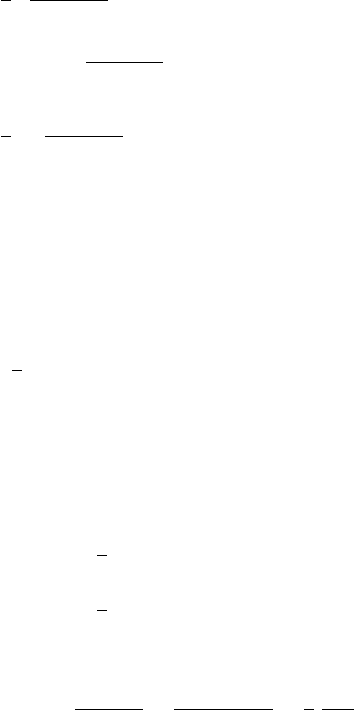
316 Chapter 9 Gravitational waves

the components of I

in a Cartesian basis according to



−

(1 + cos

θ)cos(2φ) +

(1 + cos

θ)sin(2φ)



−



sin



, (9.24)



−

cosθ sin(2φ) +

cosθ cos(2φ)



. (9.25)

Exercise 9.2 Consider a Newtonian binary consisting of two point masses m

and

in circular orbit about each other. Choose coordinates so that the binary orbits in

the xy plane and is aligned with the x-axis at t = 0.

(a) Compute the reduced quadrupole moment in the center-of-mass frame to show

that it can be written as

µR





cos 2t + 1/3 sin 2t 0

sin 2t −cos 2t + 1/30

00−2/3





, (9.26)

where  is the orbital angular velocity, µ = m

/(m

+ m

) the reduced mass,

and R the binary separation.

(b) Show that a distant observer situated at (r,θ,φ), where, without loss of generality,

φ = 0, measures the waveforms to be

=−



µR

(1 + cos

θ)cos2(t − r),

=−



µR

cos θ sin 2(t −r) .

Thus the magnitude h of the waveform amplitude is of order

h 

4µ

4µM

2/3



2/3

µM

, (9.27)

where the total mass is given by M = m

+ m

and where we have used Kepler’s

law 

= M/R

. Note that the frequency of the wave is twice the frequency of the

orbit in the quadrupole approximation.

Gravitational radiation carries energy, momentum, and angular momentum. To see this

formally

we need to expand Einstein’s equations to second order. In vacuum, the resulting

second-order equations contain two different kinds of terms – terms that are linear in the

second-order perturbations of the metric, and terms that are quadratic in the ﬁrst-order

perturbations h

. Upon suitable averaging, the latter constitute a source term for the

background curvature. This source term thus deﬁnes an “effective” stress-energy tensor

The proceedure is know as the “shortwave approximation”; see Misner et al. (1973), Sections 35.13–35.15.

9.1 Linearized waves 317

for the gravitational waves, T

. In a (nearly) Minkowski frame of linearized theory, this

effective stress-energy tensor reduces to

32π

∂

∂

TTij

, (9.28)

where denotes an average over several wavelengths. We can now use this stress-energy

tensor to compute the energy, momentum and angular momentum carried off by the

gravitational waves. The outgoing energy ﬂux in the radial direction, for example, is given

by the T

component of equation (9.28). To ﬁnd the gravitational wave luminosity, which

is equal and opposite the energy change of the source, we integrate the energy ﬂux passing

through a large sphere surrounding the source,

≡−

=−lim

r→∞



d. (9.29)

We can express the radiated energy in terms of the wave amplitudes h

and h

of the

outgoing gravitational radiation according to

=−

= lim

r→∞

16π





d, (9.30)

where we have used the fact that ∂

=−∂

for radially outgoing radiation. Similarly,

we can ﬁnd the loss of angular momentum due to radiation,

= lim

r→∞

16π



Re

∗

Hd, (9.31)

where H ≡ h

− ih

and where the operator

is deﬁned as

=−sin φ∂

− cos φ(cot θ∂

+ 2i csc θ ) (9.32)

= cos φ∂

− sin φ(cot θ∂

+ 2i csc θ ) (9.33)

= ∂

. (9.34)

In particular, this relation gives

= lim

r→∞

16π



∂

∂

+ ∂

∂

d. (9.35)

Finally, the loss of linear momentum due to radiation is

= lim

r→∞

−

16π





d. (9.36)

See, e.g., Ruiz et al. (2008a).

318 Chapter 9 Gravitational waves

In all the above expressions the quantities E, J and P

refer to the source, and their changes

are equal and opposite to the corresponding quantities carried off by the waves. We note

that equations (9.30)–(9.36) apply even in the case of strong-ﬁeld sources, since the waves

they generate constitute small perturbations to the nearly ﬂat background spacetime once

they reach large distances from the source, where these ﬂuxes are evaluated.

In the case of a weak-ﬁeld, slow-velocity source, we can express the radiated energy in

terms of the source’s reduced quadrupole moment. Inserting equation (9.20)into(9.29)

and integrating yields

=−



...

. (9.37)

This energy loss can also be modeled with a corresponding radiation-reaction force F

react

that can be written as the gradient of a radiation-reaction potential 

react

=−mD



react

,

react

(5)

. (9.38)

Here the superscript (5) denotes a ﬁfth time derivative. To show that equations (9.37)and

(9.38) are consistent with each other we compute the energy loss of a swarm of particles,

labeledbyanindexA,as



react

=−





react

=−

(5)



=−

(5)



=−

(5)

ij(1)

. (9.39)

In the last step we have been able to convert an I

into an I

since I

= 0. Taking

an average over several wave cycles we can integrate by parts twice to convert

(5)

ij(1)

into I

(3)

ij(3)

, which yields equation (9.37). We can perform a very similar calculation to

compute the loss of angular momentum, obtaining

=−



ijk



...

. (9.40)

The so-called “quadrupole formulae” (9.37) and (9.40) can be used to calculate the loss of

energy and angular momentum from any weak-ﬁeld, slow-velocity source. As an example,

we return in exercise 9.3 to the point-mass binary system of exercise 9.2.

Exercise 9.3 Revisit the point-mass binary of exercise 9.2 and evaluate (9.37)and

(9.40)toﬁnd

=−



(9.41)

Before the integration can be carried out, the transverse-traceless components of I

in equation (9.20)haveto

be expanded in terms of I

, whereby the normal vector n

= x

/r in the projection operator (9.18) introduces a

(quadrupolar) angular dependence. See, e.g., Carroll (2004), page 313, for details.

See, e.g., Burke (1971) as well as the discussion in Shapiro and Teukolsky (1983).

9.1 Linearized waves 319

and

=−



. (9.42)

The loss of energy and angular momentum due to gravitational radiation causes the

binary orbit to shrink, i.e., gravitational radiation drives binary inspiral. The inspiral and

merger of binaries containing compact stars is a very promising source of gravitational

waves for detection by laser interferometers (see Section 9.2). Consequently, compact

binary inspiral and merger is a central theme in this book, and we shall return to this topic

on several occasions.

Exercise 9.3 provides an example of the remarkable relationship

dE =  dJ, (9.43)

which holds under quite general conditions involving relativistic systems in quasistationary

equilibrium undergoing secular changes. We will encounter this relation several times later

in this book. For example, in exercise 12.2, we will see that equation (9.43) guarantees that

a circular binary undergoing slow inspiral due to gravitational wave emission will remain

in a (nearly) circular orbit.

9.1.2 Vacuum solutions

Return now to Einstein’s linearized equations (9.4) in vacuum,



= 0. (9.44)

Just as in electrodynamics, this type of equation admits simple plane wave solutions of the

form

= Re





. (9.45)

Here k

= (ω, k

) is a 4-dimensional wave vector, x

= (t, x

) denote the inertial coordi-

nates of a point in spacetime and A

is a constant tensor representing the wave amplitude.

Einstein’s equations (9.44) then demand that k

be a null vector,

= 0, (9.46)

whereby ω =|k

|. This dispersion relation implies that gravitational waves propagate at

the speed of light. The Lorentz condition (9.3) requires

= 0, (9.47)

implying that gravitational waves are transverse. The above results are quite general, since

we can always decompose an arbitrary, linear gravitational wave propagating in a nearly

ﬂat, vacuum spacetime into a superposition of the plane wave solutions (9.45).

See Chapter 12.1 for a more detailed overview of binary inspiral.

320 Chapter 9 Gravitational waves

From a numerical point of view the plane wave solutions found above are not the

most useful. Most numerical simulations treat spacetimes with ﬁnite, bounded sources, for

which the waves propagate radially outward at large distance. Moreover such spacetimes

approach asymptotic ﬂatness at least as fast as r

−1

. Clearly spacetimes containing with

plane waves (9.45) do not share these properties. More useful for simulation purposes are

multipole expansions of linear, vacuum solutions to equation (9.45) expressed in terms of

tensor spherical harmonics. Tensor spherical objects are deﬁned in Appendix D.

When working in spherical coordinates, it is more convenient to express the two polar-

ization states of gravitational radiation in terms of even-andodd-parity modes rather than

the + and × modes of equation (9.7) An even-parity mode (l, m) has parity (−1)

under

space inversion (θ,φ) → (π − θ,φ + π), while an odd-parity mode has parity (−1)

l+1

Evidently, depending on the index l, both odd- and even-parity modes can have odd or even

parity. To choose a less confusing name, some authors therefore refer to the even-parity

modes as polar and the the odd-parity modes as axial.

Of particular interest is the quadrupole l = 2 wave, which often is the dominant mode

for many sources of gravitational radiation. For even-parity or polar quadrupole modes,

the metric takes the form

=−dt

+ (1 + Af

) dr

+ (2Bf

rθ

) rdrdθ + (2Bf

rφ

) r sin θ drdφ

+(1 + Cf

(1)

θθ

+ Af

(2)

θθ

) r

dθ

+ [2(A − 2C) f

θφ

] r

sin θdθdφ

+(1 + Cf

(1)

φφ

+ Af

(2)

φφ

) r

sin

θdφ

. (9.48)

Here the coefﬁcients A, B and C can be constructed from an arbitrary function F(x),

wherewehavex = t − r for an outgoing solution or x = t + r for an ingoing solution. In

the general case, we may take

F = F

(t − r ) + F

(t + r ) (9.49)

and deﬁne

(n)

≡

(x)

x=t−r

+ (−1)

(x)

x=t+r

. (9.50)

In terms of F(x) and its derivatives we then have

A = 3



(2)

(1)



, (9.51)

B =−



(3)

(2)

(1)



, (9.52)

C =



(4)

(3)

(2)

21F

(1)

21F



. (9.53)

Teukolsky (1982); linear, vacuum quadrupole waves in this representation are sometimes referred to as “Teukolsky

waves”. For generalization to all multipoles, see Rinne (2008b).