Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.


THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Writing out explicitly the terms between square brackets for
$9
gives,
for p
=
a,
sin
(~(4
+
it
+
ip))
sin (T($
-
it
+
ip))
-
Ir(4
-it+iP)I2 1r($+it+ip)l2
From Euler's reflection formula it follows that this is equal to zero. So
$3
has a removable singularity at the point p
=
a.
From the Riemann-Lebesgue lemma, see, e.g., (Whittaker and Wat-
son, 1963, §9.41), it follows that for
f
qi
E
L1(O, CO),
i
=
1,2,3, the terms
with
$i,
i
=
1,2,3, vanish. This leaves us with a Dirichlet integral, for
which we have the property (see, e.g., (Whittaker and Watson, 1963,
S9.7))
co
lim
1
Jg(x)
sin[t (x
-
y)]
t+CO
T
dx
=
dy), (5.11)
2-Y
0
for a continuous function
g
E
L1 (0, CO). This gives for a continuous
function
f
that satisfies
f
$:
E
L1
(0,
CO),
sin (n
(4
+
it
+
ia))
sin (n
(4
-
it
+
ia))
Ir
(4
-
it
+
ia)
I
2
+
Ir
(4
+
it
+
ia)
l2
- -
et(2cp-.rr)
=
wr1(a)
f
(a).
r
(2k2)
I?
(k2
-
kl
+
it
+
1) r(2ia)
r
(k2
-
kl
+
4
+
ia)
I'
(k1+
k2
-
+
ia)
2

Continuous Hahn functions
263
In the last step Euler's reflection formula is used. The conditions kl,
k2
>
1
can be removed by analytic continuation. Since
we find that the proposition is valid for the conditions off as stated.
Next we consider the truncated inner product
(~p;,
and the
corresponding Wronskians. We need to find the analogues of Lemma
5.11 and Proposition 5.12.
Lemma
5.13.
Let kl
>
1
and p,a
2
0.
For
x
+
f
oo
Proof.
The proof is similar to the proof of Lemma 5.11.
0
Proposition
5.14.
Let
a
2
0,
and let
f
be an even continuous function
satisfying
then
00
where
1
I?
(kl
-
k2
+
it)
I?
(a
-
it
-
ia)
I?
(i
-
it
+
ia)
w1(a)
=
-e-t(2~-r)
2~
I?
(k2
-
kl
-
it
+
1)
I?
(2l~2)~
r
(k2
-
kl
+
a
+
ia)
r
(k1+ k2
-
a
+
ia)
F(2ia)
Proof.
The proof runs along the same lines as the proof of Proposition
5.12, therefore we leave out the details.

264
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
As in the proof of Proposition 5.12 we find from Lemma 5.13
where
For
p
=
a
the term between the square brackets for
$3
zero, so
$3
has a removable singularity at the point
p
=
is equal to
a.
So, for
f$i
E
L1(O,
w),
i
=
1,2,3, the terms with
$i,
i
=
1,2,3, vanish by
the Riemann-Lebesgue lemma. This leaves us with a Dirichlet integral.
Then, after applying (5.1 I), we find for
f
$4
E
L1(O,
oo)
Writing out
$4
explicitly gives the result.
0
Remark
5.15.
In Remark
5.8
we observed that the i-periodic function
p(x)
cancels the poles of
@,(x).
Other obvious choices with the same

Continuous Hahn functions
265
property would be e2kTxp(x), for
k
E
Z.
However for
k
#
0,
the method
we used here to find an integral transform pair would fail, since the
method depends on the use of the Riemann-Lebesgue lemma and the
Dirichlet kernel, which can no longer be used in case
k
#
0.
This can,
e.g., be seen from Lemma
5.11,
where the terms in front of
~xl~(~-p)
would contain a factor e2ICTx. So this gives a heuristic argument for the
choice
(5.3)
of the i-periodic function.
Let
f
be a continuous function satisfying,
and let
f
be the vector
f(d
=
@)
We define, for x
E
R,
an operator
F
by
To verify that this is a well-defined expression, we determine the be-
haviour of cpp(x) and Wo(p) for p
+
m
and p
0.
From Thomae's
transformation (Andrews et al., 1999, Cor. 3.3.6) we find
And from (5.7) we obtain
Then we see that the integral in (5.13) converges absolutely for
f
satis-
fying (5.12).
For a continuous function g satisfying
we define, for p
2
0,
an operator
6
by
cp*
(4
W(P)
=
J
g(x)
(v;(x))
WW~X.
R
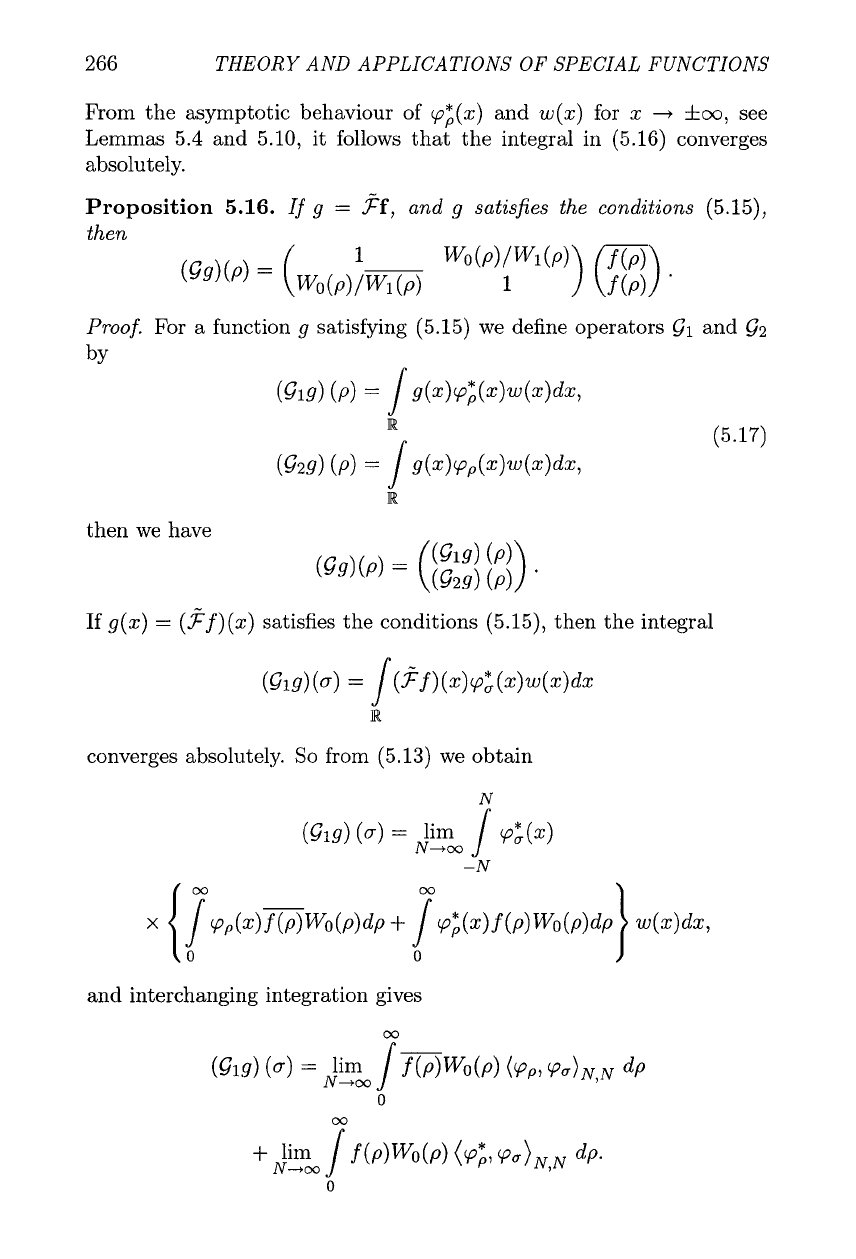
266
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
From the asymptotic behaviour of
cp;(x)
and
w(x)
for
x
+
f
oo,
see
Lemmas
5.4
and
5.10,
it follows that the integral in
(5.16)
converges
absolutely.
Proposition
5.16.
If
g
=
Ff,
and
g
satisfies the conditions
(5.15),
then
1
1
Proof.
For a function
g
satisfying
(5.15)
we define operators
G1
and
82
by
then we have
(W(P)
=
([:$
[zi)
.
If
g(x)
=
(F
f) (x)
satisfies the conditions
(5.15))
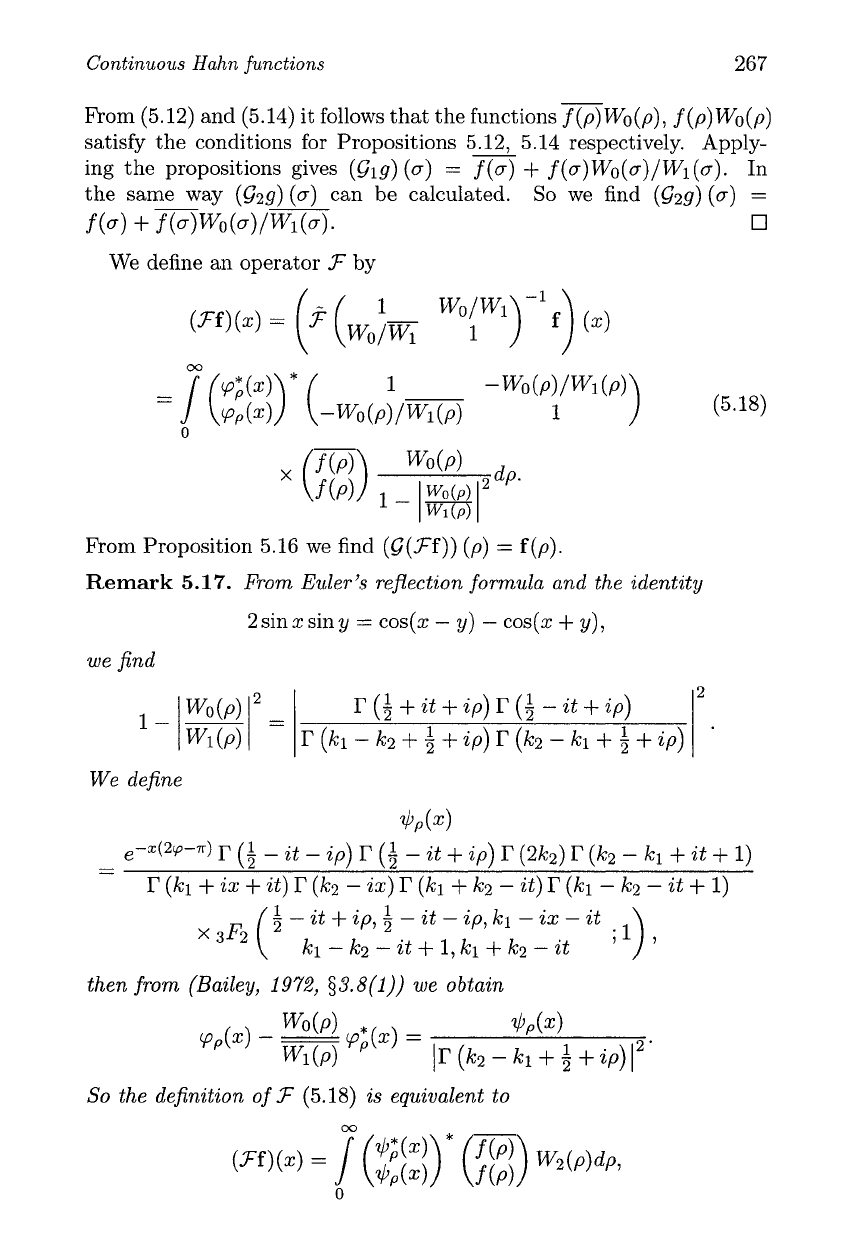
then the integral
converges absolutely. So from
(5.13)
we obtain
and interchanging integration gives
Continuous Hahn functions 267
-
From
(5.12)
and
(5.14)
it follows that the functions
f
(p) Wo(p),
f
(p) Wo(p)
satisfy the conditions for Propositions
5.12, 5.14
respectively. Apply-
ing the propositions gives
(Glg)
(a)
=
fo
+
f(a)Wo(a)/Wl(a).
In
the same way
(G2g)
(a)
can be calculated. So we find
(~2~)
(a)
=
f
(4
+
f(a)~o(a)/m.
0
We define an operator
F
by
1
wo/wl)-l
f)
(x)
(")(XI
=
1
From Proposition
5.16
we find
(G(Ff))
(p)
=
f
(p).
Remark
5.17.
From Euler's reflection formula and the identity
2
sin
x
sin
y
=
cos(x
-
y)
-
cos(x
+
y),
we find
We define
+P(4
e-~(~v-")
r
(4
-
it
-
ip)
I?
(4
-
it
+
ip)
I?
(2k2)
I'
(k2
-
kl
+
it
+
1)
-
I?
(kl
+
ix
+
it)
I?
(k2
-
ix)
(kl
+
k2
-
it)
I?
(kl
-
k2
-
it
+
1)
1
3
-
it
+
ip,
5
-
it
-
ip, kl
-
ix
-
it
x
r~2
kl
-
k2
-
it
+
1, kl
+
k2
-
it
;
1)
,
then from (Bailey,
1972,
§3.8(1))
we obtain
So the definition
of
F
(5.18) is equivalent to

268
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
where
Note that W2(p)dp is the orthogonality measure for the continuous dual
Hahn polynomials.
The function
Ff
exists for all functions
f
for which the integral
(5.18)
converges. We want to find a domain on which
F
is injective and isomet-
ric. We look for a set
S
of functions for which
FS
is a dense subspace
of
L2 (R, w(x)dx).
Recall that the set of polynomials is a dense subspace
of
L2 (R, w(x)dx).
0)
Lemma
5.18.
Let pn
(.;
9)
denote a Meixner-Pollazcek polynomial as
defined by (2.2), then
with qn given by
Proof.
Let
G1
and
G2
be
as
defined by
(5.17).
Since
-
the Meixner-
Pollaczek polynomial
pn
is real, we have
G1pn
=
G2pn.
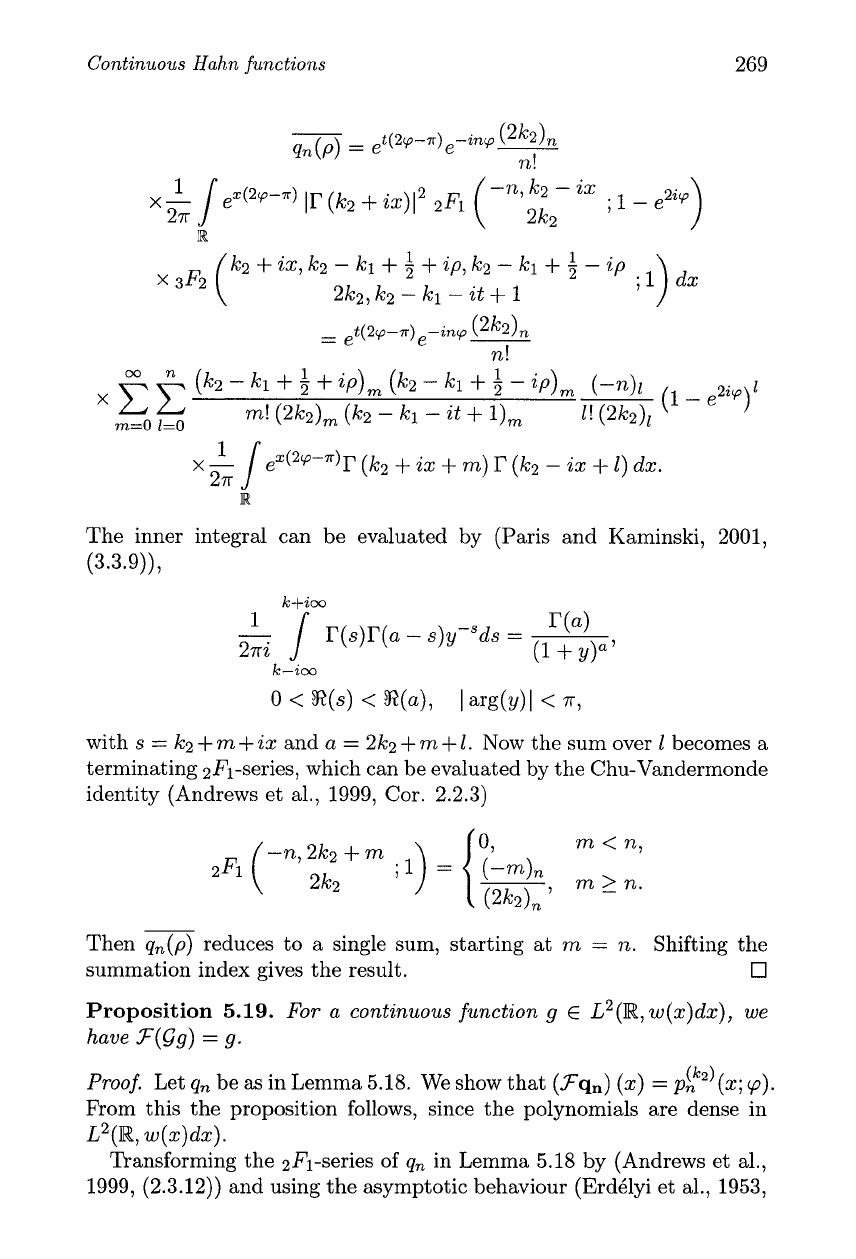
Writing out
qn (p)
=
(91pLk2)
(.;
p))
(p)
explicitl~, gives
Continuous
Hahn
functions
269
k2
+
ix, k2
-
k1
+
f
+
ip, k2
-
k1
+
f
-
ip
2k2, k2
-
kl
-
it
+
1
ca
n
(k2
-
kt
+
f
+
ip)_ (k2
-
kt
+
f
-
ip)_ (-n)r
(1
-
e2'~)z
x
x
m! (2k2),
(h
-
kl
-
it
+
1),
m=O 1=0
11
(2k2)l
x
1
ex(2q-")I' (k2
+
ix
+
m)
r
(k2
-
ix
+
1) dx.
27r
R
The inner integral can be evaluated by (Paris and Kaminski, 2001,
(3.3.911,
k-ico
with
s
=
k2
+
m
+
ix and
a
=
2k2
+
m
+
I. Now the sum over
I
becomes a
terminating 2Fl-series, which can be evaluated by the Chu-Vandermonde
identity (Andrews et al., 1999, Cor. 2.2.3)
Then qn(p) reduces to a single sum, starting at m
=
n. Shifting the
summation index gives the result.
0
Proposition
5.19.
For
a
continuous function g
E
L2(R, w(x)dx),
we
have F(6g)
=
g.
Proof. Let qn be as in Lemma 5.18. We show that
(Fq.)
(2)
=
pik2)
(x;
p).
From this the proposition follows, since the polynomials are dense in
L2 (R, w (x)dx).
Transforming the 2Fl-series of qn in Lemma 5.18 by (Andrews et al.,
1999, (2.3.12)) and using the asymptotic behaviour (ErdBlyi et al., 1953,
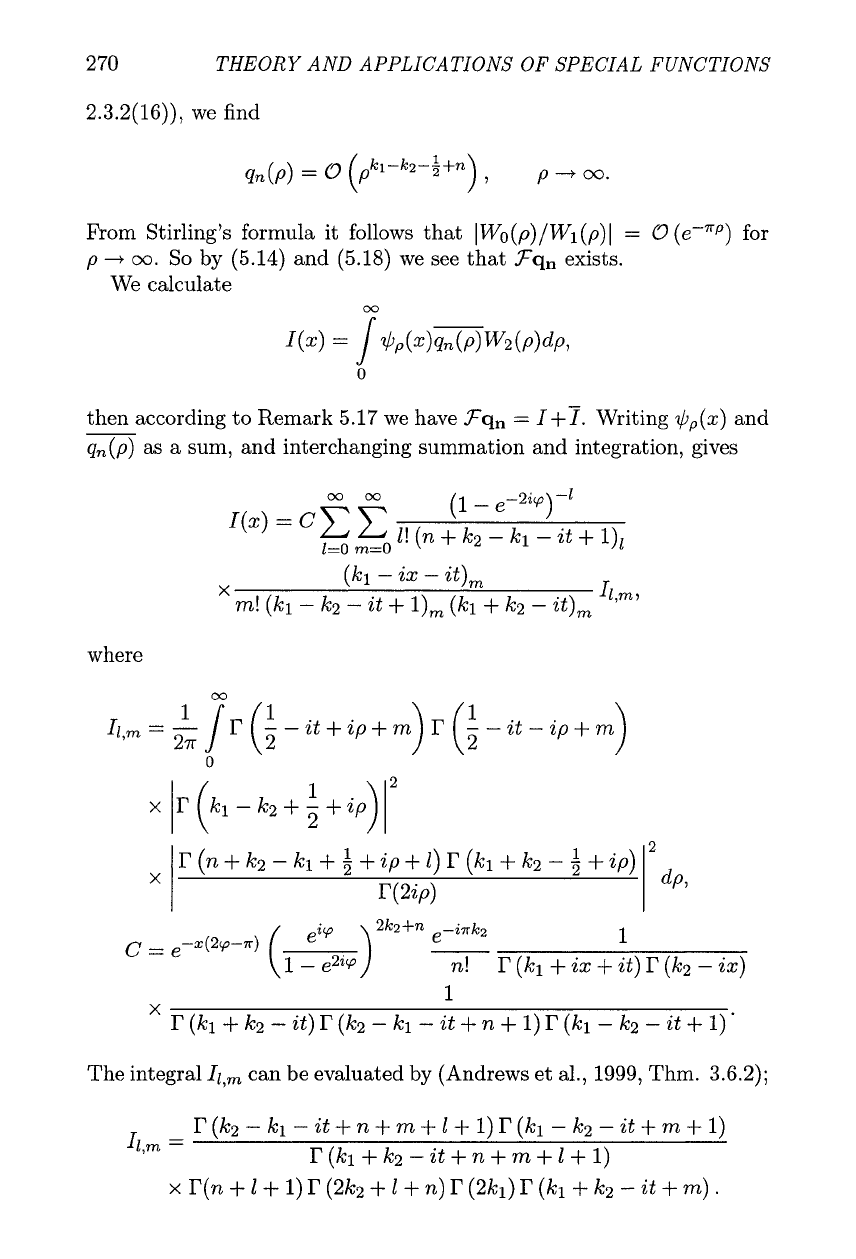
270
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
2.3.2(16)), we find
From Stirling's formula it follows that
I
Wo(p)/ Wl (p)
1
=
O
(e-"p) for
p
+
oo.
So by (5.14) and (5.18) we see that
Fq,
exists.
We calculate
then according to Remark 5.17 we have
Fq,
=
I
+q.
Writing Gp(x) and
q,(p)
as
a sum, and interchanging summation and integration, gives
where
(
:i~~~~
)
2k'+n
e-irb
C
=
e-"(2~-")
1
n!
I?
(kl
+
ix
+
it)
r
(k2
-
ix)
X
1
r
(kl
+
k2
-
it)
r
(k2
-
k1
-
it
+
n
+
1)
F
(k1
-
k2
-
it
+
1)
'
The integral
IZ,,
can be evaluated by (Andrews et al., 1999, Thm. 3.6.2);
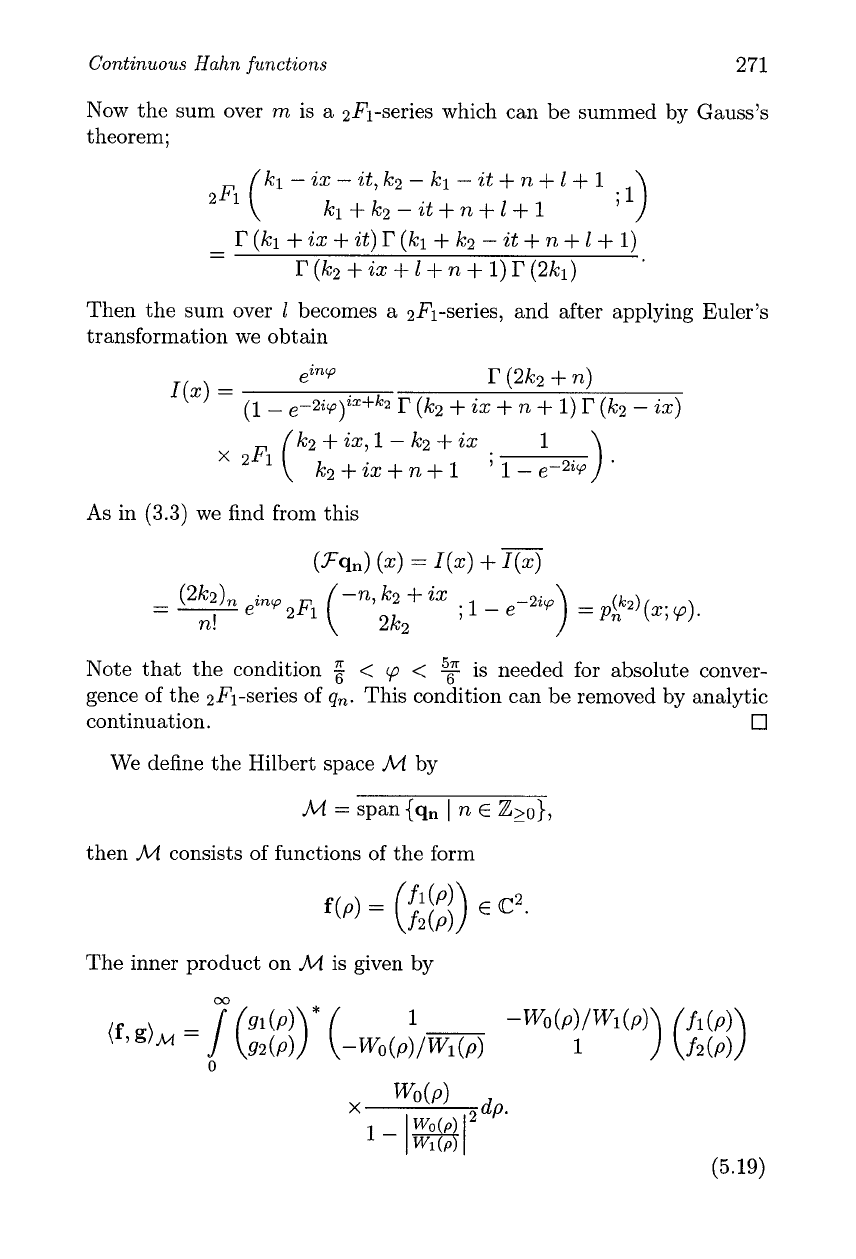
Continuous Hahn functions
271
Now the sum over
m
is a 2F1-series which can be summed by Gauss's
theorem;
Then the sum over
1
becomes a 2Fl-series7 and after applying Euler's
transformation we obtain
eincp
I(x)
=
r
(2k2
+
n)
(1
-
e-2i9)ix+k2
r
(k2
+
ix
+
n
+
1)
I?
(k2
-
ix)
k2
+
ix,
1
-
k2
+
ix
k2
+ix
+
n
+
1
As in
(3.3)
we find from this
Note that the condition
2
<
p
<
is needed for absolute conver-
gence of the 2F1-series of qn. This condition can be removed
by
analytic
continuation.
0
We define the Hilbert space
M
by
M
=
span
{qn
1
n
E
Z~O)'
then
M
consists of functions of the form
The inner product on
M
is
given by
