Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

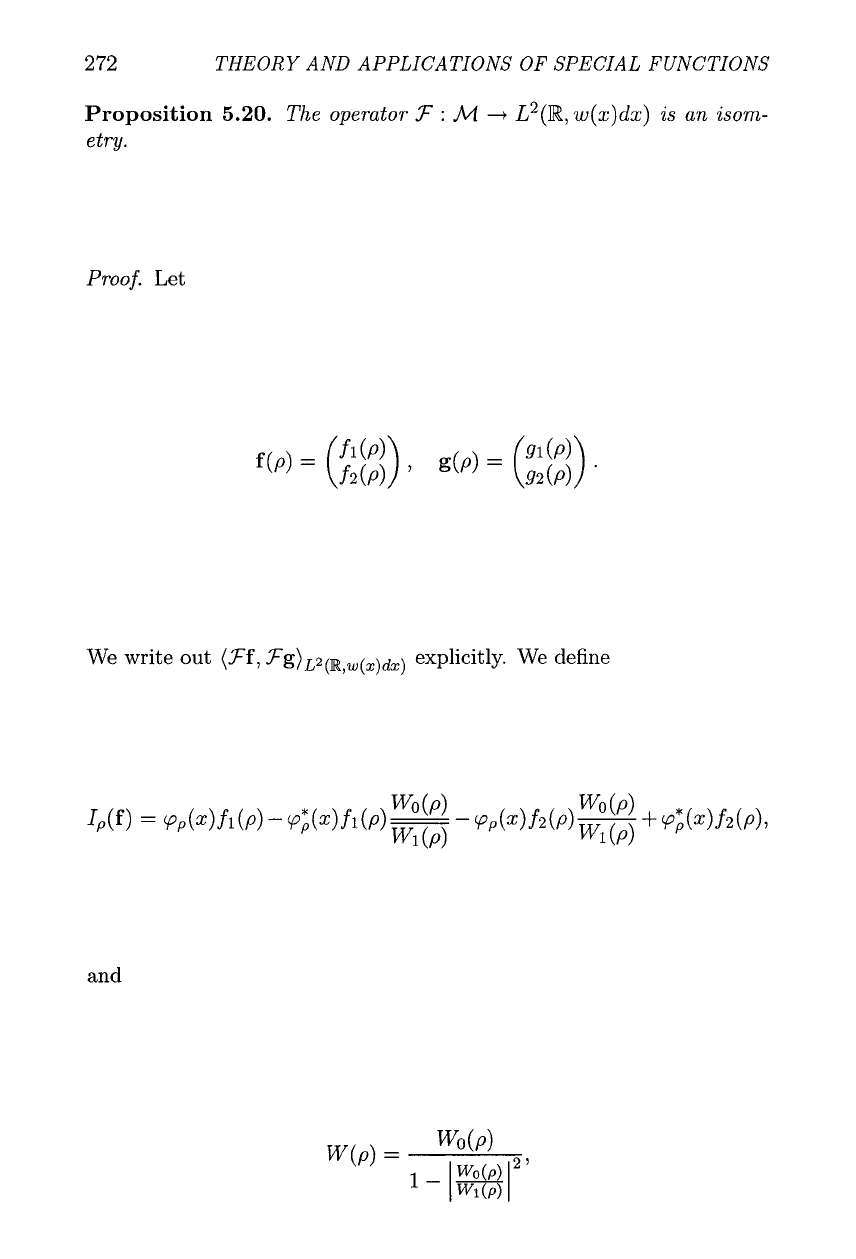
272
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Proposition
5.20.
The
operator
F
:
M
-+
L2(R, w(x)dx)
is
an
isom-
etry.
Proof.
Let
and
Continuous
Hahn
functions
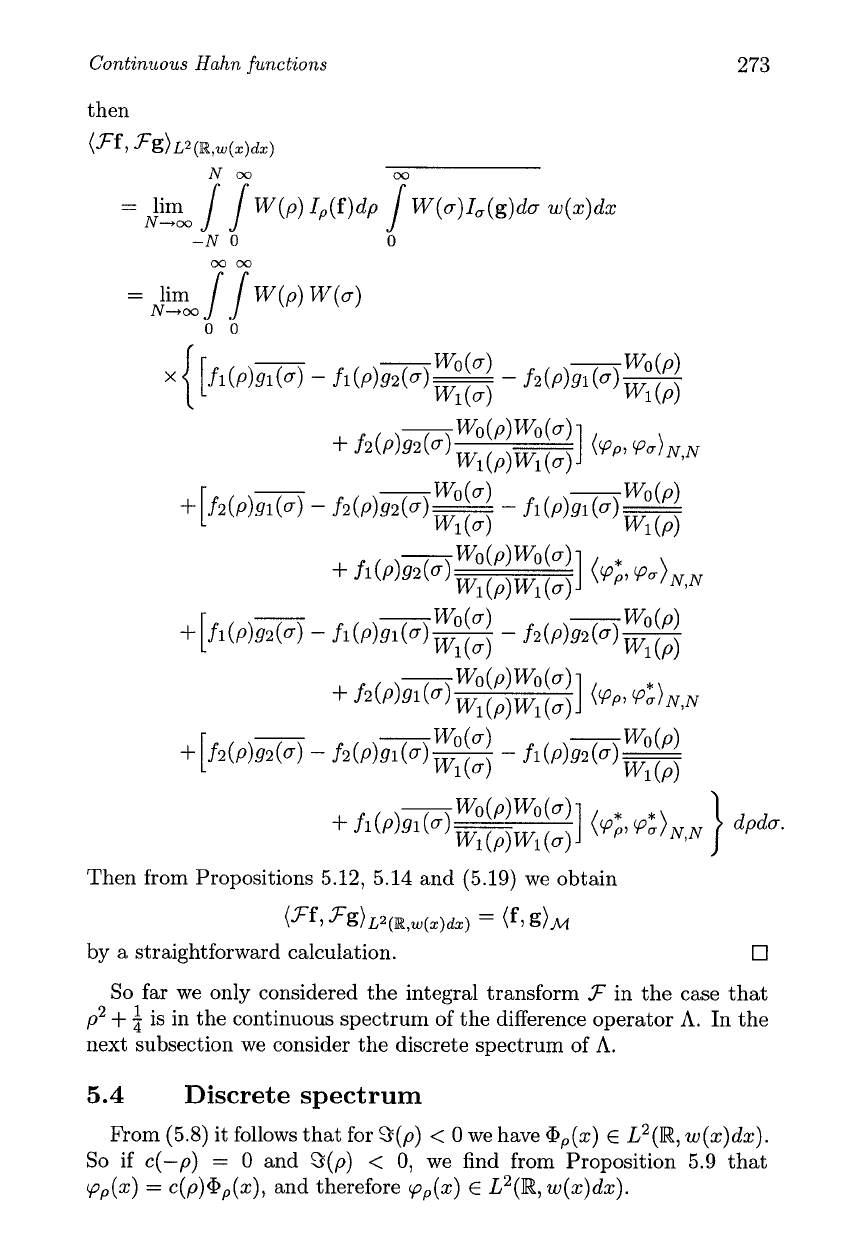
Then from Propositions
5.12,
5.14
and
(5.19)
we obtain
(Ff
7
Fg)
~y~,~(~)d~)
=
(f
7
g)~
by a straightforward calculation.
I7
So far we only considered the integral transform
F
in the case that
p2
+
a
is in the continuous spectrum of the difference operator
A.
In the
next subsection we consider the discrete spectrum of
A.
5.4
Discrete spectrum
From
(5.8)
it follows that for
Q(p)
<
0
we have
ap(x)
E
L2(R, w(x)dx).
So if
c(-p)
=
0
and
3(p)
<
0,
we find from Proposition
5.9
that
vp(x)
=
c(p)<Pp(x),
and therefore
cpp(x)
E
L2(R, w(x)dx).

274
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
There are two possible cases for c(-p)
=
0, cf. Theorem 4.1:
1.
k2
-
k1
+
<
0, then p
=
i
(k2
-
kl
+
4
+
n), n
=
0,.
.
.
,
no, where
no is the largest nonnegative integer such that k2
-
kl
+
4
+
no
<
0,
2. k1 +k2
-
8
<
0, then p=i(kl
+
k2- 8).
Case (ii) does not occur for k1
>
1,
which is needed for convergence
of the 3F2-series of yp(x
+
i).
However for kl
5
1
we use expression
(5.6) for ap(x) (which still converges if kl
5
1) and vp(x)
=
c(p)ap(x).
We see that the 3F2-series becomes a 2F1-series of unit argument, and
then, with Gauss's summation formula, we find that in case (ii) we have
@,(x)
=
e-2"xp(x).
Observe that case (i) and case (ii) exclude each
other.
First we consider case (i). For pn
=
i
(k2
-
k1
+
8
+
n), 0
5
n
5
no,
we denote yp(x) by
vpn
(2). We show that
cppn
(x) is orthogonal to
cp,,
(x)
and cpEm(x) for n
#
m. Note that
vpn
is given by a terminating series,
cf. (5.4).
Proposition
5.21.
For m, n
=
0,.
. .
,
no
e2t('f'-z)
F
(2k2)
F
(2kl
-
2k2
-
1)
=
Jnm
(kl
-
k2
+
it)
F
(kl
-
k2
-
it)
I'
(2kl
-
1)
n! (2k2
-
2kl
+
n
+
I), (2
-
2kl),
X
(2k2), (2k2
-
2kl+ 2)2,
Continuous Hahn functions
275
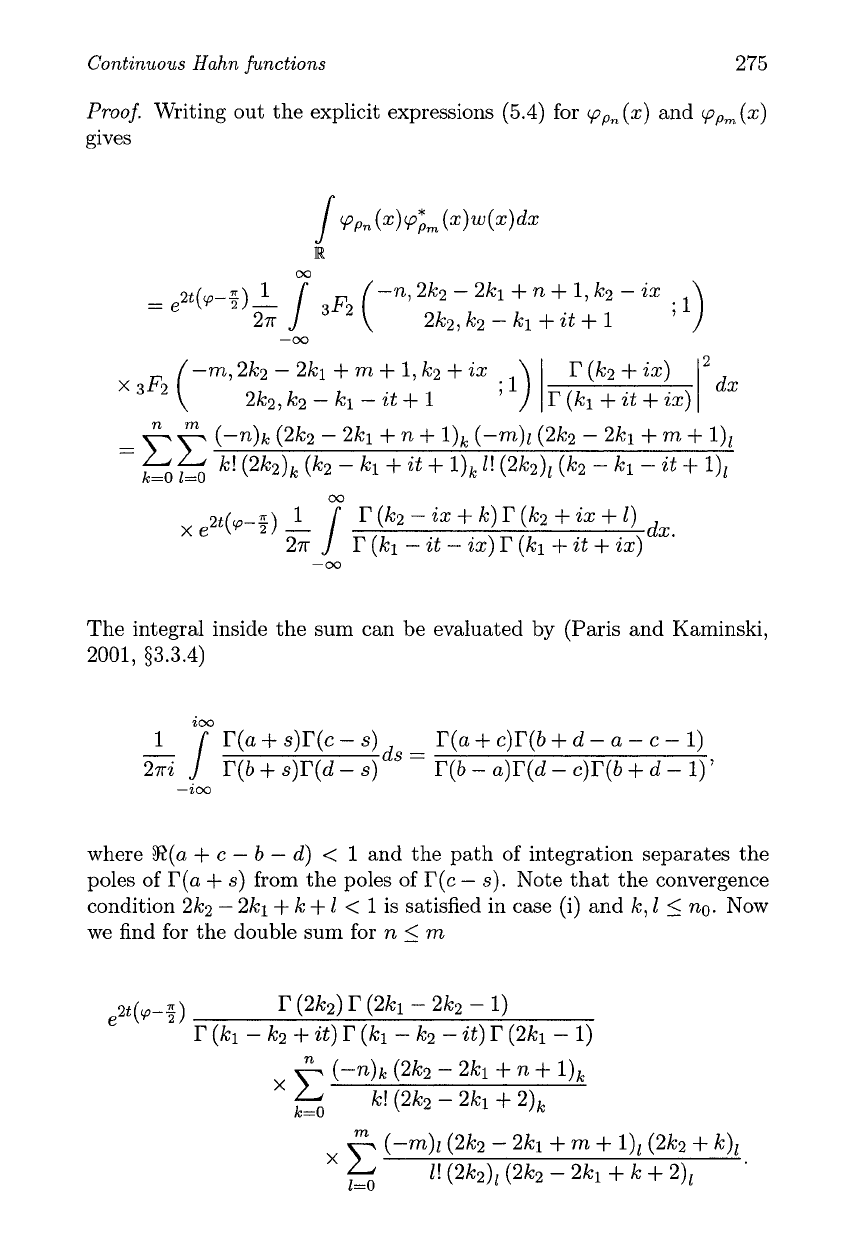
Proof.
Writing out the explicit expressions
(5.4)
for
vpn
(x)
and
ppm
(x)
gives
I'
(k2
-
ix
+
k)
I'
(k2
+
ix
+
1)
dx.
r
(kl
-
it
-
ix)
I?
(kl
+
it
+
is)
The integral inside the sum can be evaluated by (Paris and Kaminski,
2001, 93.3.4)
where
%(a
+
c
-
b
-
d)
<
1
and the path of integration separates the
poles of
,(a
+
s)
from the poles of
r(c
-
s)
.
Note that the convergence
condition
2k2
-
2kl
+
k
+
1
<
1
is satisfied in case (i) and
k,
1
2
no.
Now
we find for the double sum for
n
<
m
e2t(~-i.)
r
(21~~)
r
(2k1
-
21c2
-
1)
r
(kl
-
k2
+
it)
I?
(kl
-
k2
-
it)
(2kl
-
1)

276
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
The sum over
1
is a terminating 3F2-series, which can be evaluated by
the Pfaff-Saalschiitz theorem
So we find for
n
5
m
I?
(2k2)
I?
(2kl
-
2k2
-
1)
=
dnm
I?
(kl
-
k2
+
it)
I?
(kl
-
k2
-
it)
I?
(2kl
-
1)
Note that from the condition kg
-
kl
+
4
+
n
<
0
follows that this
expression is positive in case n
=
m.
For
n
2
m
we find the same
result by interchanging the summations over k and
1.
A straightforward
calculation shows that the expression found is equal to
Proposition
5.22.
For
m,
n
=
0,.
. .
,
no
(-l)"n! e2t(v-I)
I'
(2k2)
I?
(2kl
-
2k2
-
1)
=
Snm
I?
(kl
-
k2
+
it)
I?
(kl
-
k2
-
it)
I?
(2kl
-
1)
Proof.
From the first formula on page 142 in (Andrews et al., 1999) we
find
Continuous Hahn functions
277
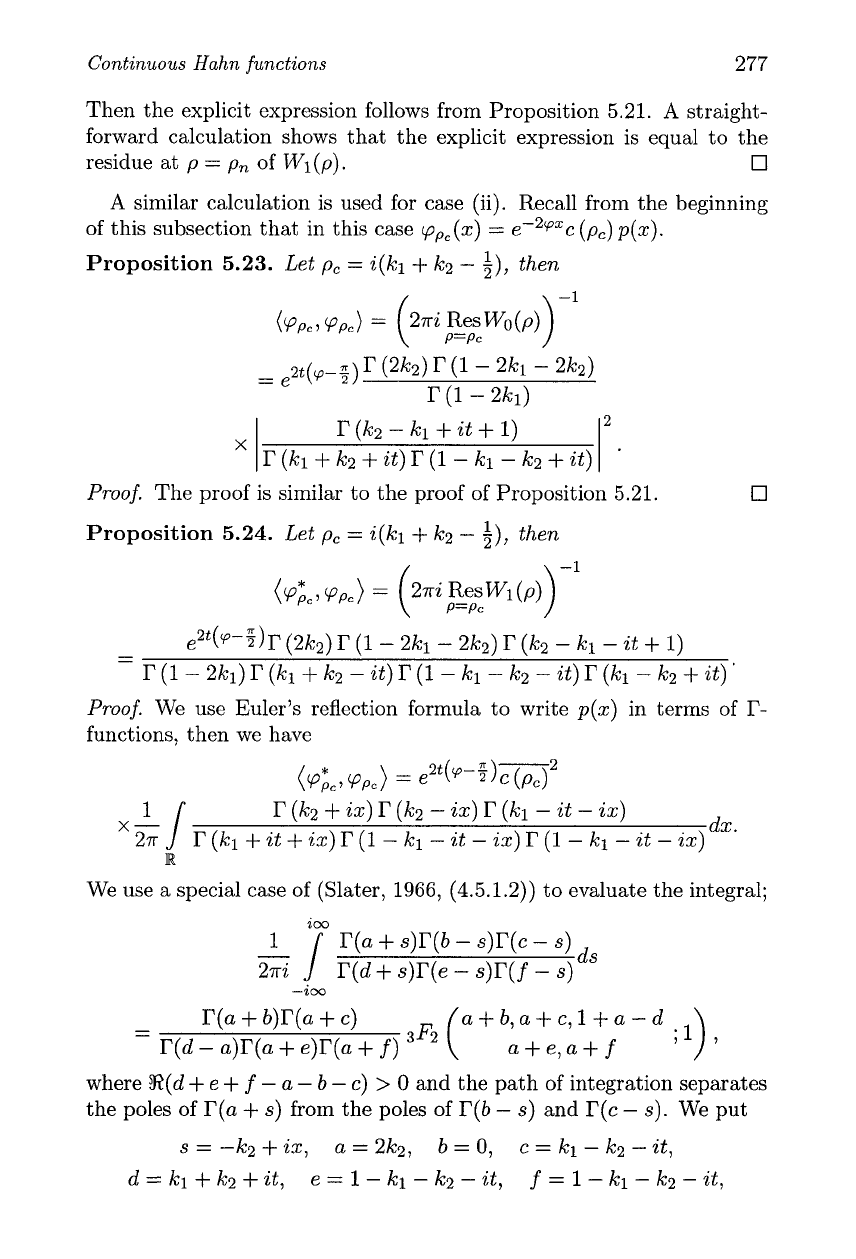
Then the explicit expression follows from Proposition
5.21.
A
straight-
forward calculation shows that the explicit expression is equal to the
residue at
p
=
p,
of
Wl
(p).
0
A
similar calculation is used for case (ii). Recall from the beginning
of this subsection that in this case
ypc(x)
=
e-2qxc
(p,)
p(x).
Proposition
5.23.
Let
p,
=
i(kl
+
k2
-
$),
then
Proof.
The proof is similar to the proof of Proposition
5.21.
Proposition
5.24.
Let
p,
=
i(kl
+
k2
-
i),
then
- -
e2'('-$)I' (2k2) I? (1
-
2kl
-
2k2) I? (k2
-
kl
-
it
+
1)
I'
(1
-
2kl)
I'
(kl
+
k2
-
it)
r
(1
-
kl
-
k2
-
it)
I? (kl
-
k2
+
it)
'
Proof.
We use Euler's reflection formula to write
p(x)
in terms of
r-
functions, then we have
(CP;~,
~p,)
=
e2t(q-;)m2
X'J
I'
(k2
+
is)
I'
(k2
-
ix) I? (kl
-
it
-
ix)
dx.
2
r(kl+it+ix)I'(l-kl-it-ix)r(l-kl-it-ix)
IW
We use a special case of (Slater,
1966, (4.5.1.2))
to evaluate the integral;
where
%(d
+
e
+
f
-
a
-
b
-
c)
>
0
and
the path of integration separates
the poles of
r(a
+
s)
from the poles of
r(b
-
s)
and
I'(c
-
s).
We put
s
=
-k2
+
ix, a
=
2k2,
b
=
0, c
=
kl
-
k2
-
it,
d
=
kl
+
k2
+
it,
e
=
1
-
kl
-
kg
-
it,
f
=
1
-
k1
-
k2
-
it,

278
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
then we find that the sF2-series reduces to a 2F1-series, which can be
evaluated by Gauss's summation formula. F'rom this the result follows.
0
Next we show that if p2
+
is in the discrete spectrum of
A,
cpp(x)
is
orthogonal to
cp,(x)
and
cp:(x),
if
a
is real.
Proposition
5.25.
For
a
E
[0,
m)
and
p
=
p,,
or
p
=
p,,
we have
Proof.
By
Propositions
5.3
and
5.7
We show that the limit of each Wronskian is zero. Let
First we consider the asymptotic behaviour of
f
(-M)
and
f (N).
For p
=
pn and
0
5
y
5
1
we find from Lemmas
5.4, 5.10
and
Proposition
5.9
For p
=
p, we find
Here the implied constants do not depend on
y.
Then dominated con-
vergence gives the result.
The proof for
(yp,
cp;)
=
0
runs along the same lines.
0
Remark
5.26.
The explicit calculations
in
this subsection can be carried
out because of the choice (5.3) of the i-periodic function p(x). It is not
likely that with another choice for the function p(x) all the calculations
can be done explicitly. This gives another (heuristic) argument for the
choice of p(x)
.
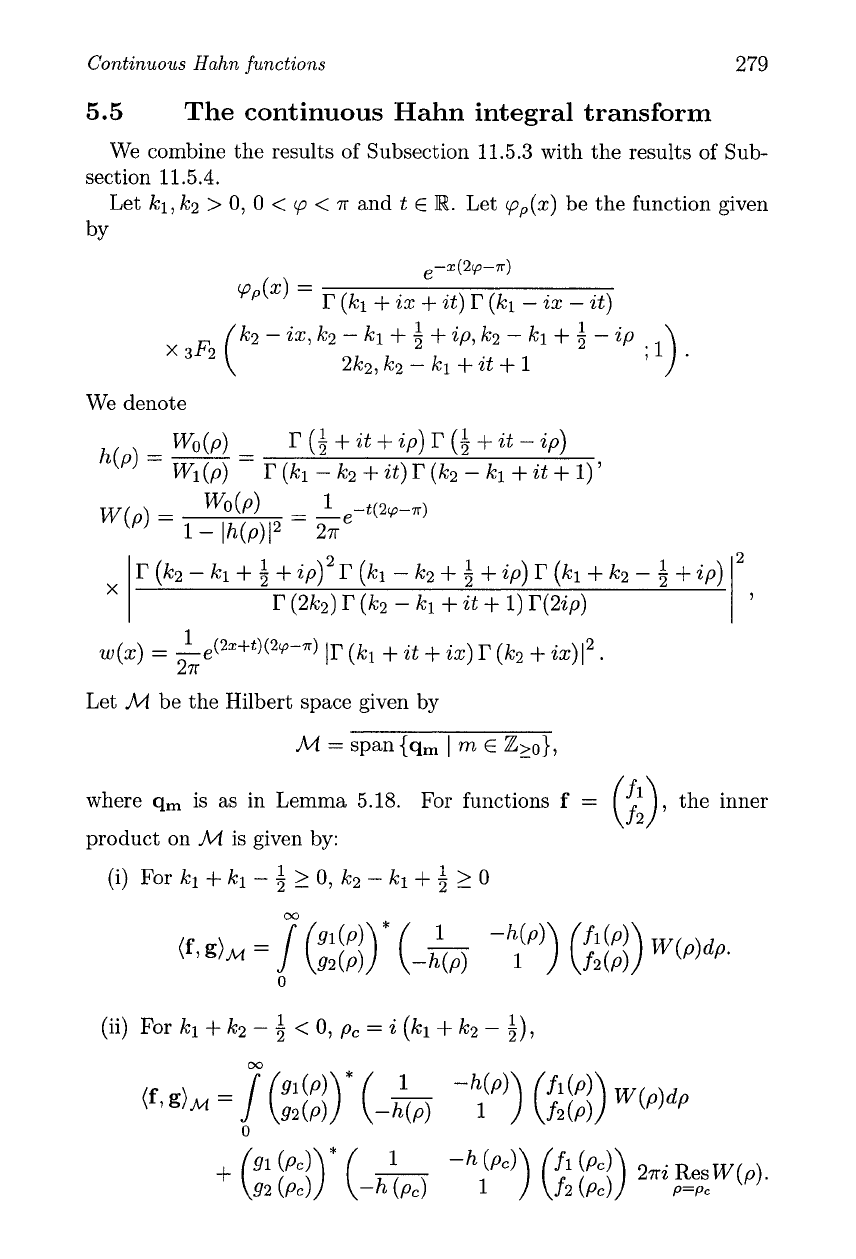
Continuous Hahn functions
279
5.5
The continuous Hahn integral transform
We combine the results of Subsection
11.5.3
with the results of Sub-
section
11.5.4.
Let
kl, k2
>
0,
0
<
cp
<
7r
and
t
E
W.
Let
cpp(x)
be the function given
by
k2
-
ix, k2
-
kl
+
+
ip, k2
-
kl
+
i
-
ip
2k2,k2
-
k1 +it
+
1
We denote
WO(P>
-
r
(i
+
it
+
ip)
I?
(i
+
it
-
ip)
h(p)
=
-
-
Wl
(p)
r
(kl
-
k2
+
it) (k2
-
k1+ it
+
1)
'
Let
M
be the Hilbert space given by
where
q,
is as in Lemma
5.18.
For functions
f
=
(2)
,
the inner
product on
M
is given by:
(i) For
kl
+
kl
-
i
2
0, k2
-
kl
+
i
1
0
(ii) For
k~
+
k2
-
4
<
0, p,
=
i
(kl
+
k2
-
i),
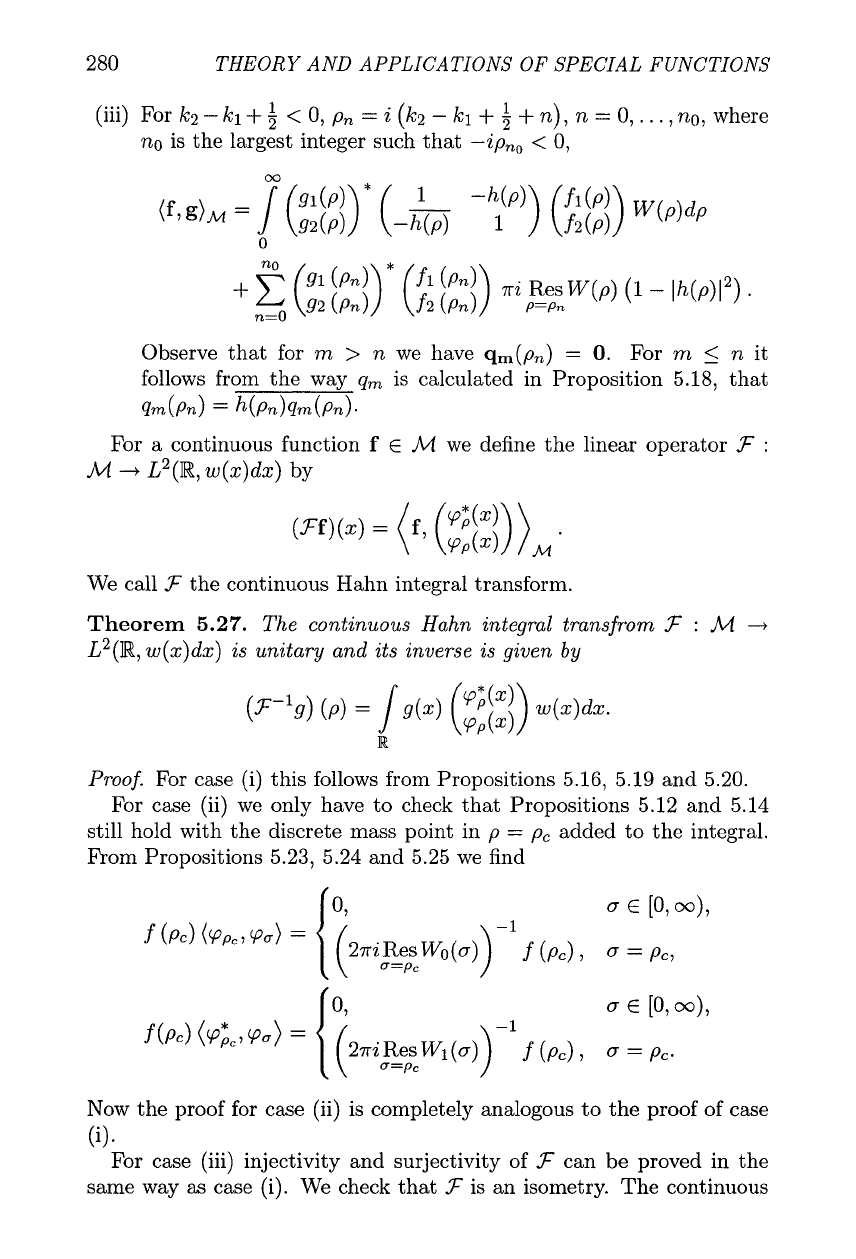
280
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
(iii) ~orkz-kl+; <O,pn =i(k2-kl+;+n),n=O,
...,
no, where
no is the largest integer such that -ipn,
<
0,
Observe that for
m
>
n we have q,(pn)
=
0.
For
m
5
n
it
follows from the way qm is calculated in Proposition 5.18, that
qm(~n)
=
h(~n)qm(~n).
For a continuous function
f
E
M
we define the linear operator
F
:
M
-t
L2
(R,
w (x)dx) by
We call
3:
the continuous Hahn integral transform.
Theorem
5.27.
The continuous Hahn integral transfrom
F
:
M
-+
L2(R, w(x)dx) is unitary and its inverse is given
by
Proof. For case (i) this follows from Propositions 5.16, 5.19 and 5.20.
For case (ii) we only have to check that Propositions 5.12 and 5.14
still hold with the discrete mass point in p
=
p, added to the integral.
From Propositions 5.23, 5.24 and 5.25 we find
Now the proof for case (ii) is completely analogous to the proof of case
6)
For case (iii) injectivity and surjectivity of
F
can be proved in the
same way
as
case (i). We check that
F
is an isometry. The continuous

Continuous Hahn functions 281
part follows from Proposition
5.20,
so we only have to check for the
discrete part. We write out
(Fqk,
Fql)LZ(a,w(x)dx),
k,
1
E
Z>o,
-
for the
discrete part of
Fqk
and
Fql.
From Propositions
5.21, 5.22
and
qk(pn)
=
h (pn)qk (pn)
we find, for
n,
m
=
0,.
. .
,
no,
no
/
C
(pPn
(Pn)
+
(P;.
(X)PI
(pn))
?ii
Res
Wo(p)
B
n=O
P'Pn
no
C
((Pprn
(XIPI
(Pm)
+
(x)
P
(Pm))
~i
Res
Wo
(p)
w
(x)
dx
m=O
P'Pm
no
--
=
C
(qk
(Pn)
41
(Pn)
+
Q
(~n)ql( pn))
ri
Res
Wo
(p)
.
n=O
P=Pn
Here we recognize the discrete part of the inner product
(qk,
ql)M.
Com-
bined with Propositions
5.20
and
5.25
this shows that
F
acts isometric
on the basis elements
qk.
By linearity
F
extends to an isometry.
The continuous Hahn integral transform in case
(i)
corresponds ex-
actly to the integral transform we found in
s11.4.3
by formal computa-
tions.
Remark
5.28.
Let us denote the operator
A
by
A
(kl, k2,
t),
let w(x)
=
w
(x; kl, k2,
t),
and let
Tt
denote the shzft operator. Observe that
It is clear that L2
(W,
w (x; kl, k2,
t)
dx) is invariant under the action of
Tt
.
A
short calculation shows that
T-t
o
A
(kl, k2,
t)
0
Tt
=
A
(k2, kl,
-t),
so
cpp
(x
+
t;
-t,
k2, kl,
(P)
is an eigenfunction of
A
(kl, k2,
t)
for eigen-
value p2
+
i.
Going through the whole machinery of this section again,
then gives another spectral measure of
A
(kl, k2,
t),
namely the one we
found with kl
H
k2, k2
H
k1 and
t
I-+
-t.
Finally we compare the spectrum of the difference operator
A
with
the tensor product decomposition in Theorem
4.1.
The discrete term
in case (ii) in this section corresponds to one complementary series rep-
resentation in the tensor product decomposition in Theorem
4.1.
Case
