Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

404
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
00
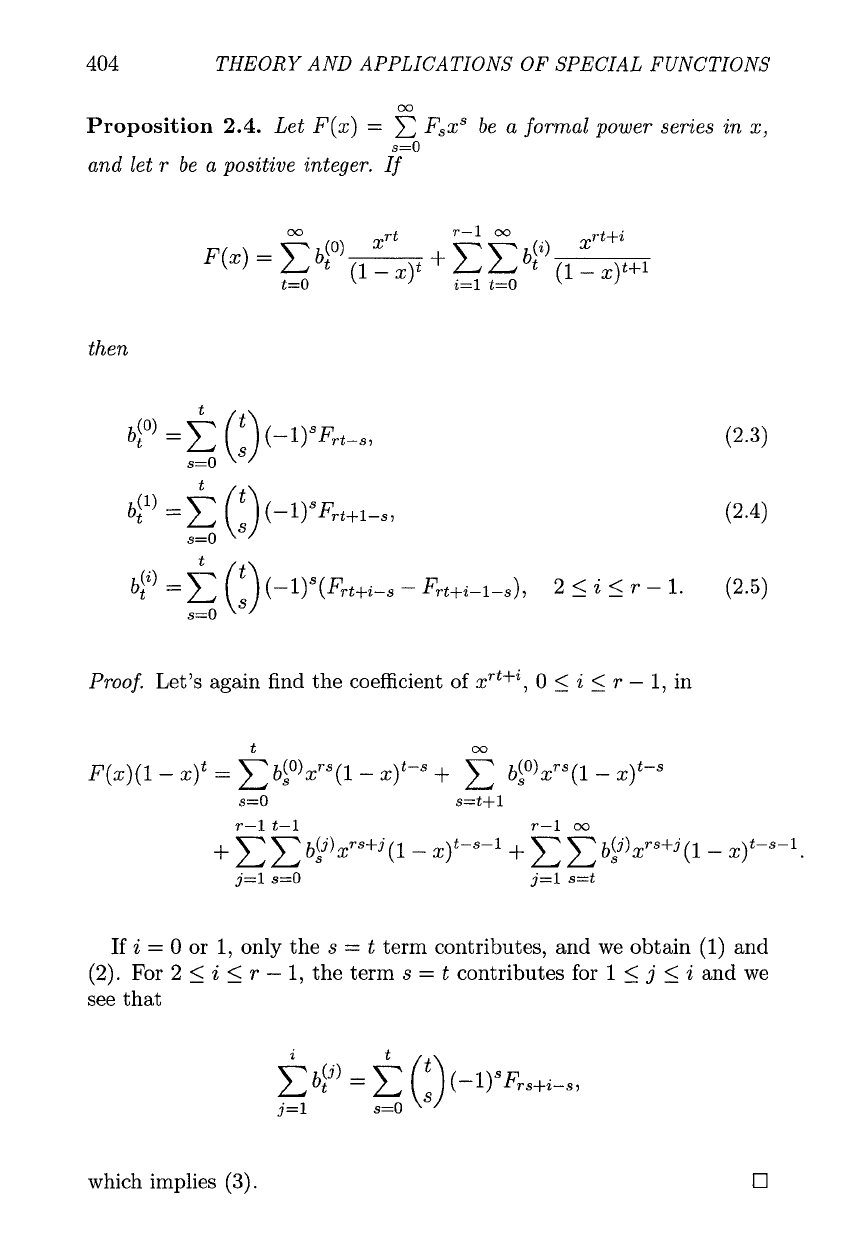
Proposition
2.4.
Let
F(x)
=
C
FSxS
be
a formal power series
in
x,
s=o
and let
r
be a positive integer. If
00
x
7-1
00
xrt+i
F
(z)
=
x
by)
(1
-
x)t
+
CCbl')
t=O
i=l
t=O
(1
-
x)t+l
then
Proof.
Let's again find the coefficient of xrt+i, 0
5
i
5
T
-
1,
in
If
i
=
0 or
1,
only the
s
=
t
term contributes, and we obtain
(1)
and
(2).
For
2
5
i
5
r
-
1,
the term
s
=
t
contributes for
1
5
j
5
i
and we
see that
which implies
(3).
Summable Sums
405
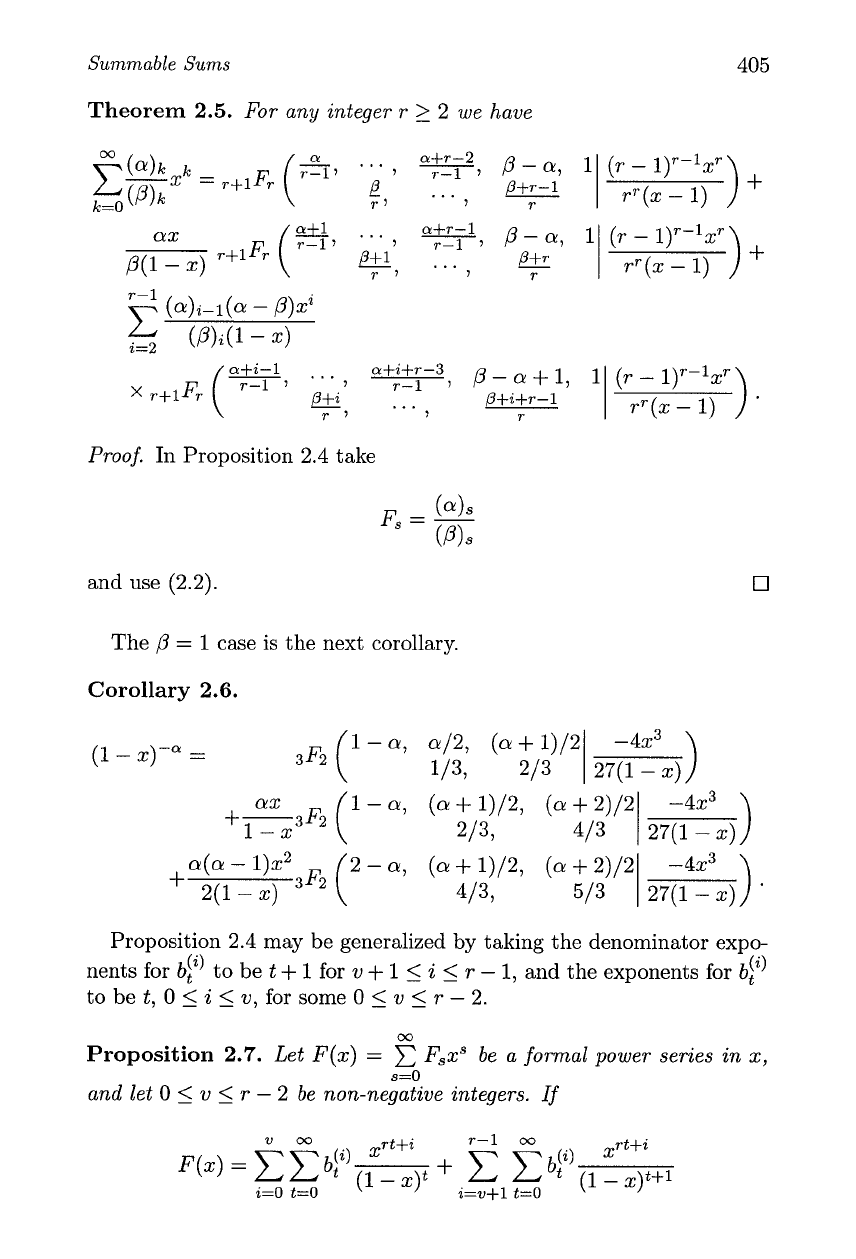
Theorem
2.5.
For any integer r
2
2
we have
Proof.
In Proposition
2.4
take
and use
(2.2).
The
/3
=
1
case is the next corollary.
Corollary
2.6.
Proposition
2.4
may be generalized by taking the denominator expo-
nents for
bf)
to be
t
+
1
for
v
+
1
<
i
<
r
-
1,
and the exponents for
bf)
to be
t,
0
<
i
<
v,
for some
0
<
v
<
r
-
2.
00
Proposition
2.7.
Let
F(x)
=
C
FsxS
be a formal power series
in
x,
s=o
and let
0
<
v
<
r
-
2
be non-negative integers.
If
2)
03
x~t+i
T-1
a3
x~t+i
F (x)
=
6:)
(1
-
x)t
+
x
bf)
i=O t=O i=v+l t=O
(1
-
x)t+l
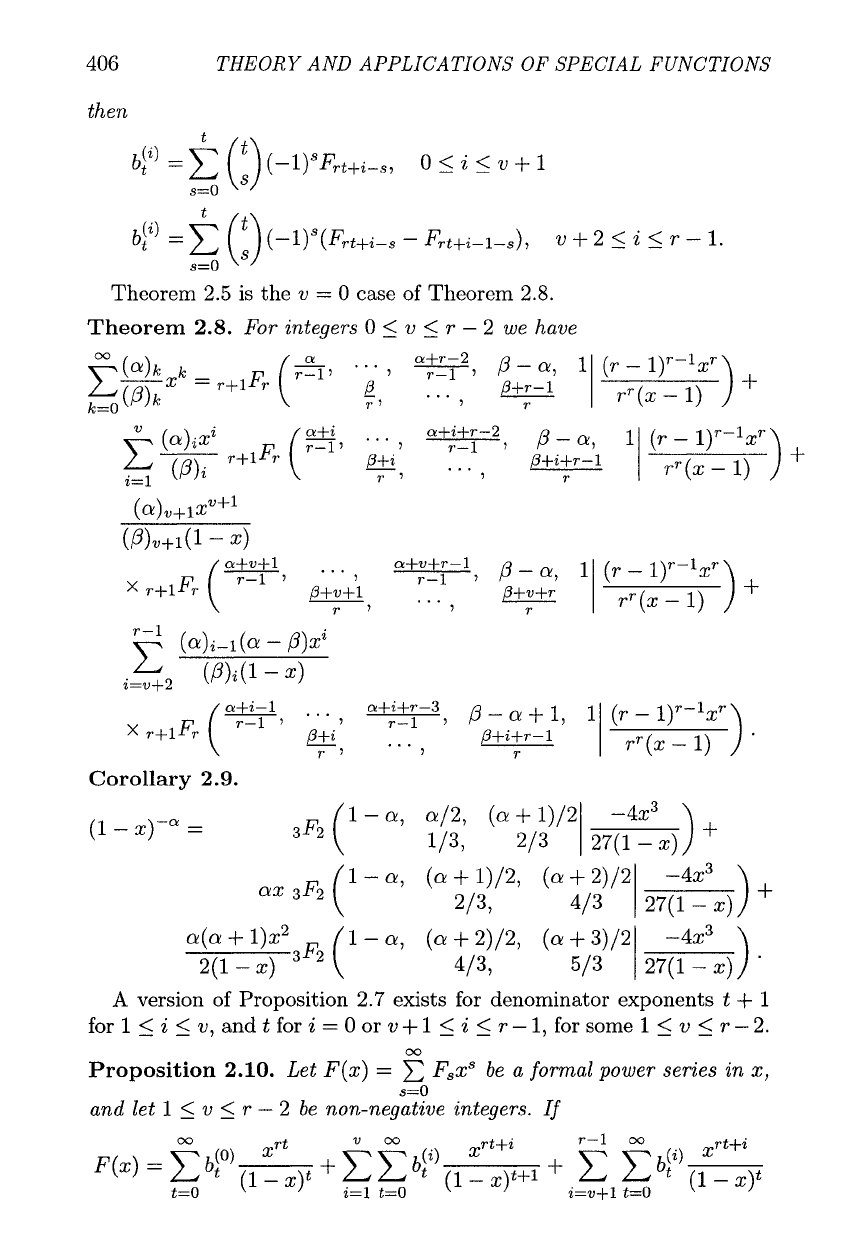
406
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
then
Theorem
2.5
is the
v
=
0
case of Theorem
2.8.
Theorem
2.8.
For integers
0
<
v
<
r
-
2
we have
Corollary
2.9.
A
version of Proposition
2.7
exists for denominator exponents
t
+
1
for1~i<v,andtfori=Oorv+1<i<r-1,forsome1<v<r-2.
00
Proposition
2.10.
Let
F(x)
=
C
FsxS
be a formal power series
in
x,
s=o
and let
1
5
v
<
r
-
2
be non-negative integers. If
00
xrt
v
00
xrt+i
r-1
00
xrt+i
F
(x)
=
bp)
(1
-
x)t
+
y
7;
bp
(1
-
x)t+l
+
C
C
bp
t=O i=l t=O i=v+l t=0
(1
-
x)~
Summable Sums
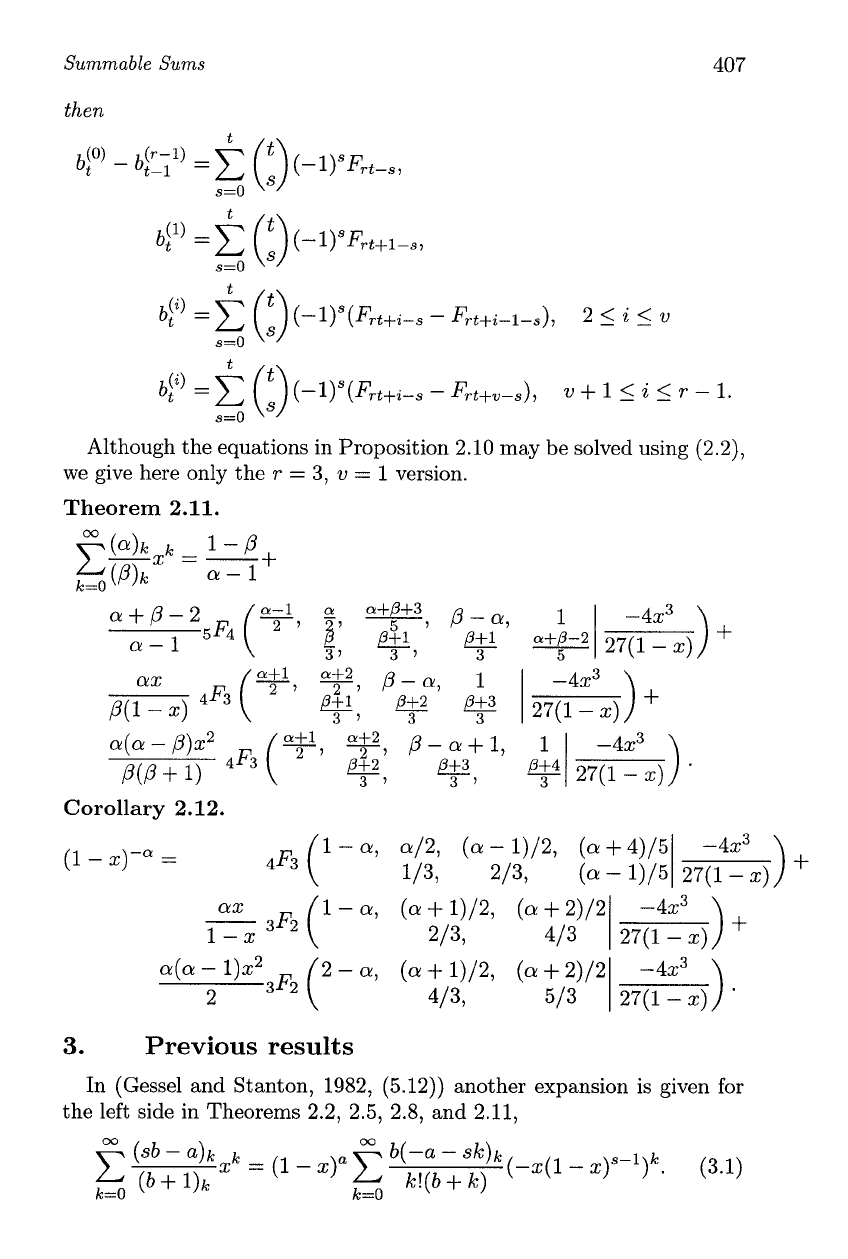
then
Although the equations in Proposition 2.10 may be solved using (2.2),
we give here only the
r
=
3,
v
=
1
version.
Theorem
2.11.
Corollary
2.12.
3.
Previous results
In (Gessel and Stanton, 1982, (5.12)) another expansion is given for
the left side in Theorems 2.2, 2.5, 2.8, and 2.11,
408
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
If
s
=
r,
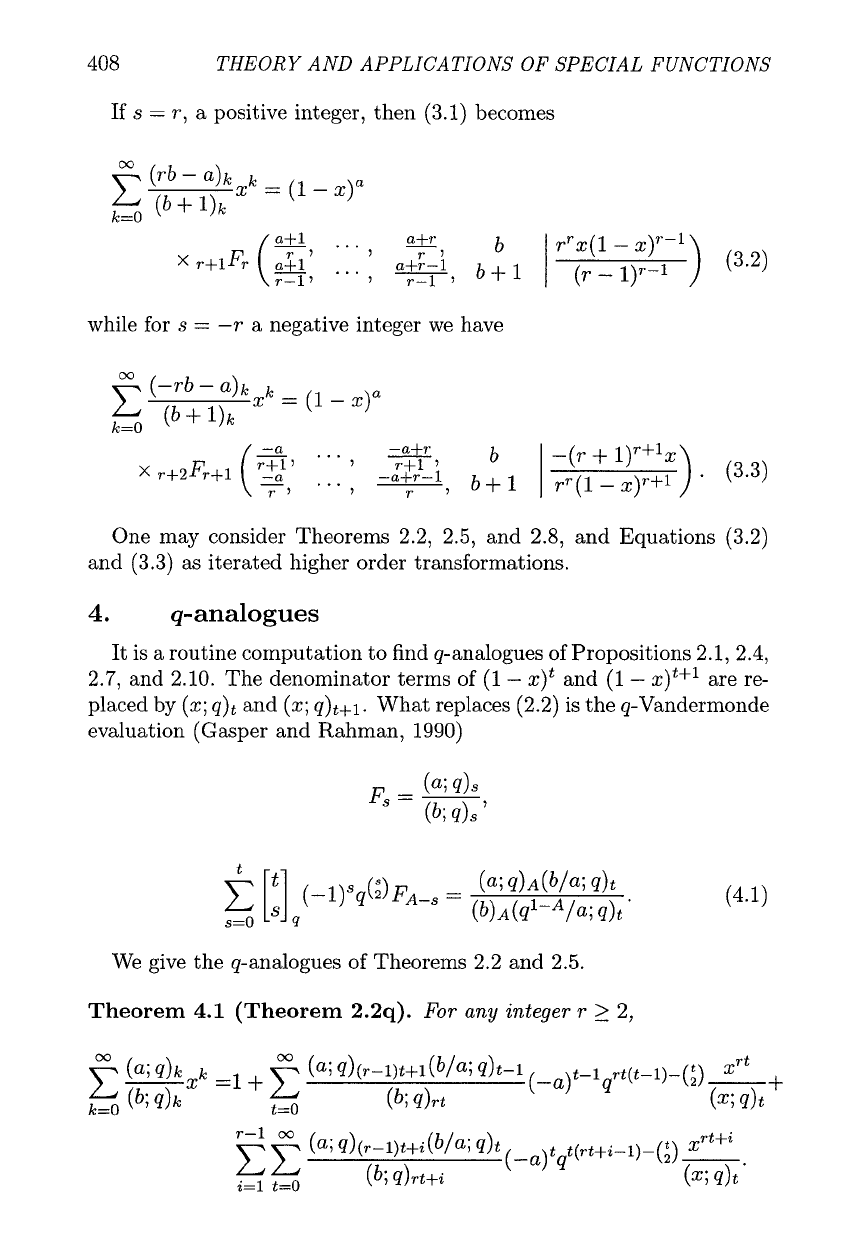
a positive integer, then (3.1) becomes
while for
s
=
-r
a negative integer we have
One may consider Theorems 2.2, 2.5, and 2.8, and Equations (3.2)
and (3.3)
as
iterated higher order transformations.
4.
q-analogues
It is a routine computation to find q-analogues of Propositions 2.1, 2.4,
2.7, and 2.10. The denominator terms of (1
-
x)~ and (1
-
x)~+~ are re-
placed by (x; q)t and (x; q)t+l. What replaces (2.2) is the q-Vandermonde
evaluation (Gasper and Rahman, 1990)
We give the q-analogues of Theorems 2.2 and 2.5.
Theorem
4.1
(Theorem
2.2q).
For
any integer
r
2
2,
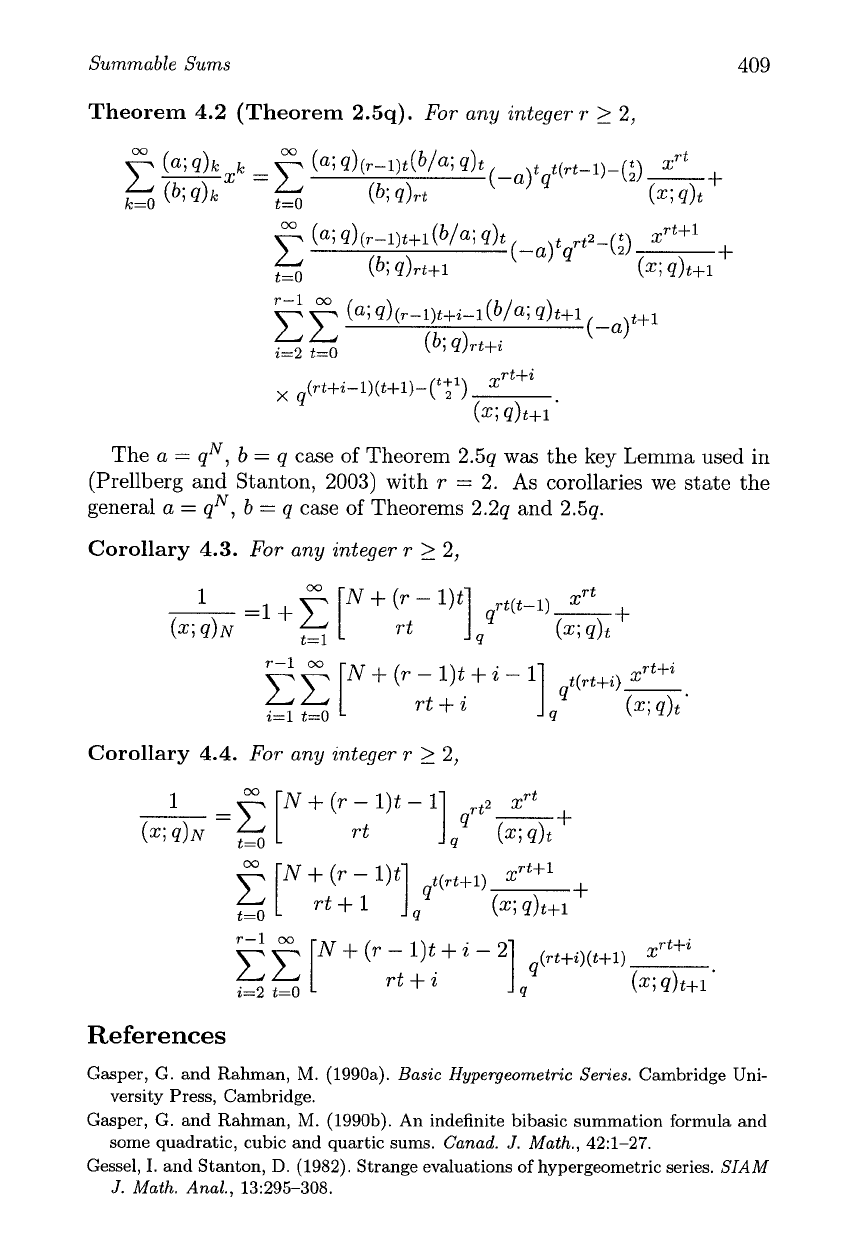
Summable Sums
409
Theorem
4.2
(Theorem
2.5q).
For any integer r
2
2,
r-l
00
CC
(a;
q)(r-l)t+i-l
(b/a;
q)t+l
i=2
t=~
(b;
q)rt+i
The
a
=
qN,
b
=
q
case of Theorem
2.5q
was the key Lemma used in
(Prellberg and Stanton,
2003)
with r
=
2.
As corollaries we state the
general
a
=
qN,
b
=
q
case of Theorems
2.2q
and
2.5q.
Corollary
4.3.
For any integer r
2
2,
Corollary
4.4.
For any integer r
>
2,
References
Gasper, G. and Rahman,
M.
(1990a). Basic Hypergeometric Series. Cambridge Uni-
versity Press, Cambridge.
Gasper, G. and Rahman,
M.
(1990b). An indefinite bibasic summation formula and
some quadratic, cubic and quartic sums. Canad.
J.
Math., 42:l-27.
Gessel,
I.
and Stanton,
D.
(1982). Strange evaluations of hypergeometric series. SIAM
J.
Math. Anal., 13:295-308.

410
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
Prellberg,
T.
and Stanton,
D.
(2003). Proof of a monotonicity conjecture.
J.
Comb.
Th.
A,
103:377-381.
Rahman,
M.
(1989). Some cubic summation formulas for basic hypergeometric series.
Utilztas Math., 36:161-172.
Rahman,
M.
(1993). Some quadratic and cubic summation formulas for basic hyper-
geometric series. Canad.
J.
Math., 45:394-411.
Rahman,
M.
(1997). Some cubic summation and transformation formulas. Ramanujan
J.,
1 :299-308.
ASKEY-WILSON FUNCTIONS AND
QUANTUM GROUPS
Jasper
V.
Stokman*
KdV Institute for Mathematics
Universiteit van Amsterdam
Plantage Muidergracht
24
1018
TV Amsterdam
THE NETHERLANDS
Abstract
Eigenfunctions of the Askey-Wilson second order q-difference operator
for
0
<
q
<
1
and
lql
=
1
are constructed
as
formal matrix coefficients of
the principal series representation of the quantized universal enveloping
algebra
Uq(s1(2,
C)).
The eigenfunctions are given in integral form. We
show that for
0
<
q
<
1
the resulting eigenfunction can be rewritten as
a very-well-poised *p7-series, and reduces for special parameter values
to a natural elliptic analogue of the cosine kernel.
1.
Introduction
The aim of this paper is to simplify the quantum group construction
of explicit eigenfunctions of the second order Askey-Wilson q-difference
operator, and to extend the results to the interesting and less well studied
Iq1
=
1
case.
The approach is based on the known fact from (Koornwinder, 1993),
(Noumi and Mimachi, 1992) and (Koelink, 1996) that the second order
Askey-Wilson difference operator arises
as
radial part of the quantum
Casimir element of Uq(51(2, C)) when the radial part is computed with
respect to Koornwinder's twisted primitive elements. Using this result,
we construct nonpolynomial eigenfunctions of the Askey-Wilson second
order difference operator as matrix coefficients of the principal series
representation of Uq(5[(2, C)). The two cases
0
<
q
<
1
and Iql
=
1
will be treated seperately. The theory for
0
<
q
<
1
is related to the
*Supported
by
the Royal Netherlands Academy
of
Arts and Sciences
(KNAW).
O
2005
Springer Science+Business Media, Inc.

412
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
noncompact quantum group Uq(su(l,
I)),
while for Iql
=
1
it is related
to the noncompact quantum group Uq(sI(2,
R)).
This approach was considered in (Koelink and Stokman, 2001b) for
0
<
q
<
1
using an explicit realization of the principal series rep-
resentation on
12(2).
The resulting eigenfunction then appears as a
non-symmetric Poisson type kernel involving nonterminating
acpl
series.
With the help of a highly nontrivial summation formula, proved by Rah-
man in the appendix of (Koelink and Stokman, 2001b) (see (Koelink
and Rosengren, 2002) for extensions), this eigenfunction was expressed
as one of the explicit 8cp7-solutions of the Askey-Wilson second order dif-
ference operator from (Ismail and Rahman, 1991). This eigenfunction
was called the Askey-Wilson function in (Koelink and Stokman, 2001a),
since it is a meromorphic continuation of the Askey-Wilson polynomial
in its degree. In this paper we start by reproving this result, now using an
explicit realization of the principal series representation of Uq(sI(2,
(C))
as
difference operators acting on analytic functions on the complex plane.
Koornwinder's twisted primitive element then acts as a first order differ-
ence operator, hence eigenvectors are easily constructed (for the positive
discrete series, this was observed in (Van der Jeugt and Jagannathan,
1998) and in (Rosengren, 2000)). The corresponding matrix coefficients
lead to explicit integral representations for eigenfunctions of the Askey-
Wilson second order difference operator. These matrix coefficients can
be rewritten as the explicit 8cp7-series representation of the Askey-Wilson
function by a residue computation.
We also show that for a special choice of parameter values, the Askey-
Wilson function reduces to an elliptic analogue of the cosine kernel. This
is the analogue of the classical fact that the Jacobi function reduces to
the cosine kernel for special parameter values, see e.g., (Koornwinder,
1984). We give two proofs, one proof uses an explicit expansion formula
of the Askey-Wilson function in Askey-Wilson polynomials from (Stok-
man, 2002), the other proof uses Cherednik's Hecke algebra techniques
from (Cherednik, 1997) and (Stokman, 2001).
In the second part of the paper we consider the quantum group tech-
niques for 141
=
1.
In this case, the approach is similar to the con-
struction of quantum analogues of Whittaker vectors and Whittaker
functions from (Kharchev et al., 2002). The role of q-shifted factorials,
or equivalently q-gamma functions, is now taken over by Ruijsenaars'
(Ruijsenaars, 1997) hyperbolic gamma function. The hyperbolic gamma
function is directly related to Barnes' double gamma function, as well
as
to Kurokawa's double sine function, see (Ruijsenaars, 1999) and refer-
ences therein. The quantum group technique applied to this particular
set-up leads to an eigenfunction of the Askey-Wilson second order dif-

Aslcey- Wilson functions and quantum groups
413
ference operator for 141
=
1,
given explicitly
as
an Euler type integral
involving hyperbolic gamma functions.
The emphasis in this paper lies on exhibiting the similarities between
the 0
<
q
<
1
case and the 1q1
=
1
case as much as possible. Other
approaches might very well lead to eigenfunctions for the Askey-Wilson
second order difference operator for Iql
=
1
which are "more optimal," in
the sense that they satisfy
two
Askey-Wilson type difference equations
in the geometric parameter, one with respect to base q
=
exp(2nir), the
other with respect to the "modular inverted" base q
=
exp(2~i/r), cf.
(Kharchev et al., 2002) for q-Whittaker functions. Such eigenfunctions
are expected to be realized as matrix coefficients of the
modular double
of the quantum group Uq(51(2, C)) (a concept introduced by Faddeev in
(Faddeev, 2000)), and are expected to be closely related to Ruijsenaars'
(Ruijsenaars, 1999), (Ruijsenaars, 2001) R-function. The R-function is
an eigenfunction of two Askey-Wilson type second order difference oper-
ators in the geometric parameter, which is explicitly given as a Barnes'
type integral involving hyperbolic gamma functions. I hope to return to
these considerations in a future paper.
Acknowledgments
It is a pleasure to dedicate this paper to Mizan Rahman. His im-
portant contributions to the theory of basic hypergeometric series and,
more concretely, his kind help in the earlier stages of the research on the
Askey-Wilson functions in (Koelink and Stokman, 2001b), have played,
and still play, an important role in my research on Askey-Wilson func-
tions.
2.
Generalized gamma functions
In this section we discuss q-analogues of the gamma function for de-
formation parameter
q
in the regions 0
<
Iql
<
1
and IqJ
=
1.
2.1
The q-gamma function for
0
<
Iql
<
1.
Let
r
be a fixed complex number in the upper half plane
W.
The
corresponding deformation parameter q
=
q,
=
exp(2nir) has modulus
less than one. We write q"
=
exp(2~iru) for
u
E
C.
Let
b, bj
E
C and
n
E
Z>o
-
U
{oo).
The q-shifted factorial is defined by
