Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

424

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

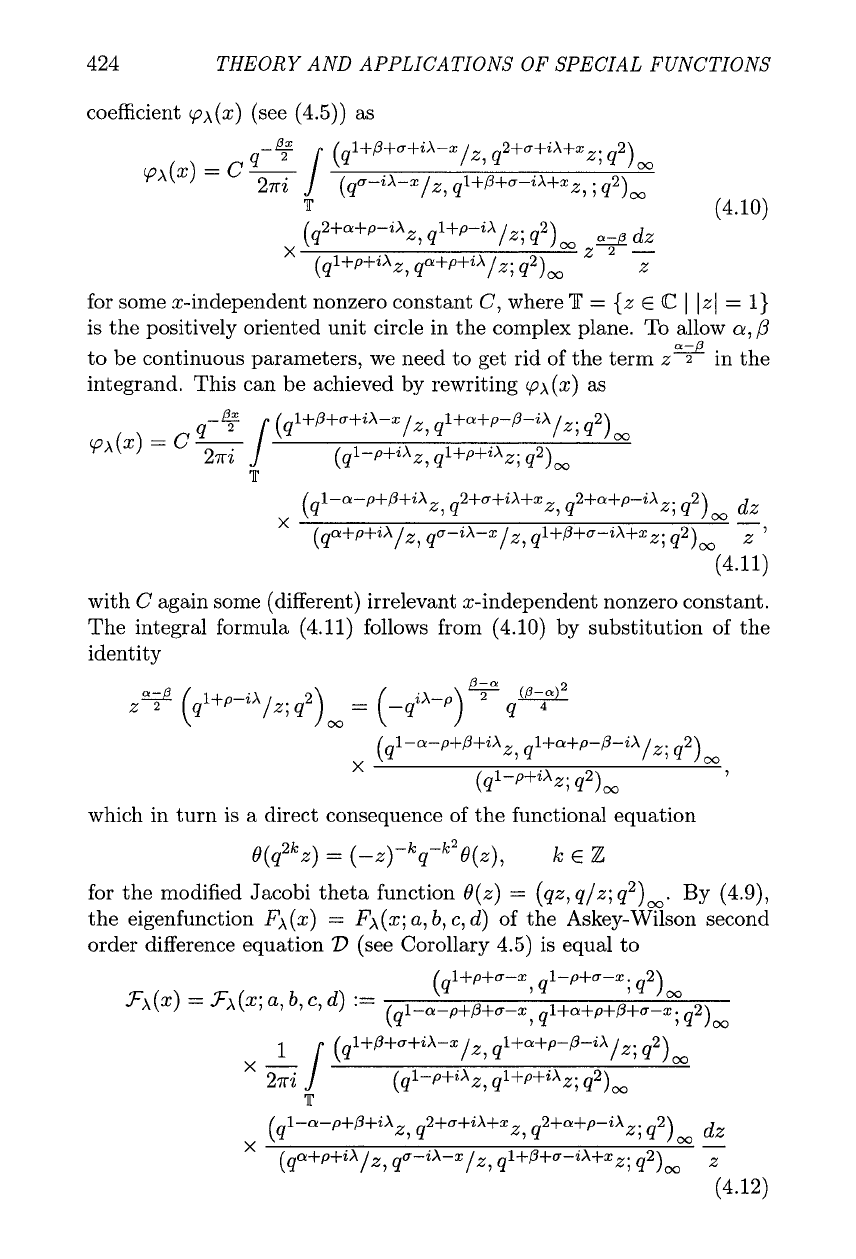
coefficient cpx(x) (see (4.5))

for some x-independent nonzero constant C, where

lzl

is the positively oriented unit circle in the complex plane. To allow

to be continuous parameters, we need to get rid of the term z%@ in the

integrand. This can be achieved

rewriting cpx(x) as

with C again some (different) irrelevant x-independent nonzero constant.

The integral formula (4.11) follows from (4.10) by substitution of the

identity

which in turn is a direct consequence of the functional equation

for the modified Jacobi theta function Q(z)

(az, q/z;

a2),.

By (4.9),

the eigenfunction FA(%)

Fx(x; a,

c, d) of the Askey-Wilson second

order difference equation

(see Corollary 4.5) is equal to

Askey- Wilson functions and quantum groups

425

up to some nonzero x-independent multiplicative constant.

Define for the Askey-Wilson parameters (a, b, c, d) given by (3.7), dual

Askey-Wilson parameters (a,

c, d) by

This notion of dual Askey-Wilson parameters coincides with the notion

of dual parameters as used in, e.g., (Koelink and Stokman, 2001a).

In the following theorem we express Fx(x) in terms of basic hyperge-

ometric series by shrinking the radius of the integration circle

to zero

while picking up residues. Recall that the very-well-poised

s(p7

series is

defined by

for lzl

see (Gasper and Rahman, 1990).

Theorem

4.6.

Let

a,P

IR>O

and p,a

R>3.

Under the

parameter correspondence (3.7), the function .FA(x)

-FA

(x; a,

given by (4.12) can be expressed in terms of basic hypergeometric series

with the (irrelevant) generically nonzero, x-independent constant

given

Furthermore, FA(x) is ~-l-~eriodic and satisfies the Aslcey- Wilson dif-

ference equation (27.F~)

(x)

E(X)FA(x) for generic x

Proof. The expression for

follows by shrinking the radius of the inte-

gration contour

to zero while picking up residues. It is actually a spe-

cial case of (Gasper and Rahman, 1990, Exerc. 4.4, p. 122), in which one

should replace the base q by q2 and the parameters

(a,

426

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

We have seen that the difference equation (DFA) (x)

E(A)FA(x) for

generic x

is valid under the extra assumption

2Z>o. For

this difference equation has been proved by Ismail and Rah-

man (Ismail and Rahman, 1991) using the explicit expression of FA(x)

as very-well-poised

8(P7

series.

Using the explicit expressions of FA(x), the conditions on x,

and the

four parameters

can be relaxed by meromorphic continuation.

The resulting function

is a meromorphic, ~-'-~eriodic eigenfunction

of the Askey-Wilson second order difference operator

D~*~*~,~

with

eigenvalue

(A)

(A;

Remark

4.7.

Some special cases of the matrix coeficients

(PA

were ex-

plicitly expressed

terms of very-well-poised

S(P7

series

(Koelink and

Stokman, 2001b) using the realization of the representation

.rrx

on the

representation space l2(2). In this approach the basic hypergeometric se-

ries manipulations are much harder, since one needs a highly nontrivial

evaluation of a non-symmetric Poisson type kernel involving nontermi-

nating 2pl-series which is due to Rahman, see the appendix of (Koelink

and Stokman, 2001 b) and (Koelink and Rosengren, 2002).

Remark

4.8.

The explicit

897

expression

(4.13)

FA(x)

and its an-

alytic continuation was named the Askey- Wilson function in (Koelink

and Stokman, 2001a). Suslov (Suslov, 1997), (Suslov, 2002) established

Fourier-Bessel type orthogonality relations for the Aslcey- Wilson func-

tion. Koelink and the author (Koelink and Stokman, 2001b), (Koelink

and Stokman, 2OOla) defined a generalized Fourier transform involv-

ing the Askey- Wilson function as the integral kernel, and established its

Plancherel and inversion formula. This transform, called the Askey-

Wilson function transform, arises as Fourier transform on the noncom-

pact quantum group

SUq

(1,l)

(see (Koelink and Stokman, ,2001 b)), and

may thus be seen as a natural analogue of the Jacobi function transform.

The expansion formula and the elliptic cosine

kernel

In this section we still assume that

ilW>o, so

exp(2ri~)

1. To keep contact with the conventions of the previous

section, we keep working in base

q2.

Askey- Wilson functions and quantum groups

427

First we recall the (normalized) Askey-Wilson polynomials (Askey and

Wilson, 1985). The Askey-Wilson polynomials Em(x)

Em(x; a, b, c, d)

Z>o)

are defined by

with

al, a2,

a3,

(~1,

a21

a31

a4; qh

I,[

4V3

(

hib21b3

;

'")

k=O (q,

bll

b3;

q)k

The Askey-Wilson polynomial E,(x) is a polynomial in qx

q-" of

degree m, normalized by Em(a)

1. They satisfy the orthogonality

relations

for m

provided that Re(a), Re@), Re(c), Re(d)

Observe that

the Askey-Wilson polynomial Em(x) is regular at the special choice

of Askey-Wilson parameters. The above orthogonality relations also

extend (by continuity) to the Askey-Wilson parameters (5.1), leading to

for m

hence we conclude that

cos(2~m~x), m

Z>o, (5.2)

which is the usual cosine kernel from the Fourier theory on the unit

circle, cf. (Askey and Wilson, 1985, (4.25)). In this section we derive an

analogous result for the Askey-Wilson function.

In the previous section we have introduced the dual Askey-Wilson pa-

rameters associated to a,

c and

They can be alternatively expressed

428

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Note that the special choice (5.1) of Askey-Wilson parameters is self

dual, (a,

c, d)

(a,

c, d). For our present purposes it is convenient

to use yet another normalization of the Askey-Wilson function, namely

for

lq2-d-pI

For fixed

the eigenfunction

.FA(-)

of the Askey-

Wilson second order difference operator

is a constant multiple of

&+(2iX,

.).

The present normalization of the Askey-Wilson function is

convenient due to the properties

and

(-a, -a)

The property (5.3) is called duality and can be

proved using a transformation formula for very-well-poised

897

series,

see (Koelink and Stokman, 2001a) for details. Furthermore,

see, e.g., (Koelink and Stokman, 2001a, (3.5)), thus the Askey-Wilson

function &+(p, x) provides a natural meromorphic continuation of the

Askey-Wilson polynomial in its degree.

The meromorphic continuation of &+(p, x) in p and x can be estab-

lished by the integral representation of the Askey-Wilson function (see

the previous section), or by the expression of the Askey-Wilson func-

tion

a sum of two balanced

493'~

(see, e.g., (Koelink and Stokman,

2001a, (3.3))). For our present purposes, it is most convenient to con-

sider the meromorphic continuation via the expansion formula of the

Askey-Wilson function in Askey-Wilson polynomials, given by

see (Stokman, 2002, Thm. 4.2). The sum converges absolutely and uni-

formly on compacta of (p, x)

due to the Gaussian

qm2.

The

Aslcey- Wilson functions and quantum groups

429

expansion formula (5.5) shows that the Askey-Wilson function @(p, x)

is well defined and regular at the special choice (5.1) of Askey-Wilson

parameters. In fact, for this special choice of parameters, the Askey-

Wilson function can be expressed in terms of the (renormalized) Jacobi

theta function

(x)

(-ql+",

-ql-" ;

q2)

follows.

Proposition

5.1.

We have the identity

Proof.

To simplify notations, we write

for the duration of the proof.

We substitute the special choice (5.1) of Askey-Wilson parameters in

the expansion formula for @(p, x). By simple q-series manipulations

and by (5.2)) we obtain the explicit formula

Using the well known Jacobi triple product identity

and the elementary identity

cos(2~mrp) cos(2nmrx)

(cos(2?rmr(p

x))

cos (2?rmr(p

x)))

we deduce that

Simplifying the multiplicative constant yields the desired result.

430

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

Remark

5.2.

The Jacobi theta function 6(x) is the natural ~-'-~eriodic

analogue of the Gaussian since

by the Jacobi triple product identity and the Jacobi inversion formula.

In fact,

(Stokman, 2002) and (Stokman, 2001) it is shown that the

function

(q2-d+x, q2-d-x;

q2)_, which reduces to 6(x) for the Askey-

Wilson parameters (5.1), plays the role of the Gaussian

the Askey-

Wilson theory. If one replaces the theta functions by Gaussians

the

right hand side of (5.6), then we obtain up to a multiplicative constant

which is essentially the classical cosine kernel. Thus the right hand side

of (5.6) is an elliptic analogue of the cosine kernel.

Remark

5.3.

Using the quasi-periodicity

of the Jacobi theta function, we obtain as a consequence of (5.6),

which is in accordance with (5.2) and (5.4).

Remark

5.4.

The orthogonality relations for the Askey- Wilson poly-

nomials with Askey- Wilson parameters (5.1) are equivalent to the L2-

theory of the classical Fourier tansform on the unit circle. On the

other hand, the L2-theory of the Askey- Wilson function transform, see

(Koelink and Stokman, 2OOla), does not reduce to the L2-theory of the

classical Fourier theory on the real line for the Askey- Wilson parame-

ters (5.1). Instead one obtains a Fourier type transform with integral

kernel given by the elliptic cosine function (5.6). For the corresponding

L2 theory, the transform is defined on a weighted L2-space consisting of

functions that are supported on a finite closed interval and an infinite,

unbounded sequence of discrete mass points. This transform, as well as

the general Askey- Wilson function transform, still has many properties

common with the classical Fourier transform on the real line, see e.g.,

(Koelink and Stokman, 2001a), (Stokman, 2002) and (Stokman, 2001).

Aslcey- Wilson functions and quantum groups

431

Cherednik's (Cherednik, 1997) Hecke algebra approach to q-special

functions leads to a direct proof that the right hand side of the expan-

sion formula (5.5) is an eigenfunction of the Askey-Wilson second or-

der difference operator V, see (Stokman, 2001). The expansion formula

(5.5) may thus be seen as the explicit link between Cherednik's approach

and Ismail's and Rahman's (Ismail and Rahman, 1991) construction of

eigenfunctions of

in terms of very-well-poised

897

series. We end this

section by sketching a proof of Proposition 5.1 using Cherednik's Hecke

algebra approach.

The affine Hecke algebra techniques for Askey-Wilson polynomials

are developed in full detail in (Noumi and Stokman, 2000), and for

Askey-Wilson functions in (Stokman, 2001). We first recall one of the

main results from (Stokman, 2001)) specialized to the present rank one

situation.

Define two difference-reflection operators by

qa+")

qb+')

(2'~~~

(x)

-qa+bf (x)

q2")

(-4

(4)

The connection with affine Hecke algebras follows from the fact that

T:~

and TI

T:'~

satisfy Hecke type quadratic relations. These

relations imply that the operators To and TI are invertible. Consider

the (invertible) operator

Remark

5.5.

The operator

Y-I, acting on even functions, is es-

sentially the Askey- Wilson second order difference operator V; see, e.g.,

(Noumi and Stokman, 2000, Prop.

5.8).

Theorem 5.17 in (Stokman, 2001) states that for generic Askey-Wilson

parameters (a,

d),

there exists a unique meromorphic function

&(.,

@(.,

c, d) on

satisfying the following six conditions:

@(p, x) is ~-'-~eriodic

p and x,

(p,

(q2-d+p,

q2-d-p

q 2-d+x,

q2-d-~

Irn

&(p, x) is analytic,

For fixed generic p

E(p,

is an eigenfunction of

~~!~l~3~

with

eigenvalue qa-p,

432

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

For fixed generic x

&(.,

x) is an eigenfunction of

~~y~,~,~

with

eigenvalue qa-"

(rpb&(p,

.))

(x)

-cia+)

(T;?"(.,x)) (p),

The existence of a kernel

satisfying the above six conditions is proved

by explicitly constructing

series expansion in nonsymmetric ana-

logues of the Askey-Wilson polynomials, see (Stokman, 2001, (6.6)).

This expansion formula for

is very similar to the expansion formula

(5.5) of the Askey-Wilson function

in Askey-Wilson polynomials. In

fact, a comparison of the formulas leads to the explicit link

see (Stokman, 2001, Thm. 6.20). These results allow us to study the

Askey-Wilson function

using the characterizing conditions

1-6

for

the underlying kernel

instead of focussing on the explicit expression

for

&+.

The kernel &(p,

is regular at the Askey-Wilson parameters (5.1).

The resulting kernel

is the unique meromorphic kernel satisfying the six conditions

1-6

for

the special Askey-Wilson parameters (5.1). Observe that the operators

To, TI and

for the special Askey-Wilson parameters (5.1) reduce to

hence Eo is the unique meromorphic kernel satisfying the six conditions

1'.

Eo(p, x) is ~-l-~eriodic in p and x,

2'.

(p, x)

6(p)6(x)

(p,

is analytic,

3'.

@o(p, x

q-%(p, x),

Askey- Wilson functions and quantum groups

We conclude that

since the right hand side satisfies

1'-6'

due to the quasi-periodicity (5.8)

of 29(x). Thus eo(p, x) is an elliptic analogue of the exponential kernel

exp(-4ni~px), cf. Remark 5.2. Using the notation (5.7)' we conclude

that

which is the desired formula

(5.6).

The

Askey-Wilson function for

Iql

In this section we take

that q

exp(2ni~) has

modulus one and q

The assignment

uniquely extends to a unital, anti-linear, anti-algebra involution on

Uq.

This particular choice of *-structure corresponds to the real form sI(2, R)

of 42,

C).

The *-unitary sesquilinear form for the representations nx

R) of

(see Section 3) is

ico

where

and

are meromorphic functions which are regular on a large

enough strip around the imaginary axes and decay sufficiently fast at

ioo. Koornwinder's twisted primitive element iYp

is *-selfadjoint

for

R. Thus in principle we are all set to extend the construction of

eigenfunctions of the Askey-Wilson second order difference operator

to the 141

case by simply replacing the role of the q-gamma function

F2,

by G2,. We need to be careful though due to the following differences

with the

case:

The analogue of the explicit eigenfunction of iYp (cf. (4.2)), given

now as quotient of hyperbolic gamma functions G2,, has more

singularities.

No ~-l-~eriodicity conditions have to be imposed. Consequently,

the parameters

and

do not need to be discretized for the Iql

case.