Mavko G., Mukerji T., Dvorkin J. The Rock Physics Handbook
Подождите немного. Документ загружается.


An effective dynamic permeability k* can be derived from the relation
k
Ps
¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
io=ðk
NÞ
p
as (Muller et al., 2007):
k
ð3DÞ
k
0
¼1
2
pp
3
þ
2
3
2
pp
k
2
Psr
Z
1
0
rBðrÞexpðrk
Psr
Þsinðrk
Psr
Þdr
k
ð1DÞ
k
0
¼1
2
pp
þ
ffiffiffi
2
p
2
pp
k
Psr
Z
1
0
BðrÞexpðrk
Psr
Þsinðrk
Psr
þ p=4Þdr
In the low-frequency limit,
k
ð3DÞ
ðo ! 0Þk
0
ð1
2
kk
=3Þ
k
ð1DÞ
ðo ! 0Þk
0
ð1
2
kk
Þ
The one-dimensional low-frequency limit corresponds to a harmonic average of the
permeability for small fluctuations. The high-frequency limit, for both one and three
dimensions, is just the arithmetic average of the permeability values. The effective
permeability is bounded by the harmonic and arithmetic average:
1
k
1
¼ k
ð1DÞ
ðo ! 0Þ < k
ð3DÞ
ðo ! 0Þ < k
ð1D;3DÞ
ðo !1Þ¼ k
hi
The one-dimensional result for the frequency-dependent effective permeability can
be expressed in a rescaled form as (Muller et al., 2007):
k
ð1DÞ
k
0
¼
1
k
1
k
hi
1
þ
2
R
ffiffiffi
2
p
k
Psr
Z
1
0
BðrÞexpðrk
Psr
Þsinðrk
Psr
þ p=4Þdr
2
R
¼ 1
1
k
k
hi
1
¼
normalized difference of the harmonic
and arithmetic averages.
An extended first-order approximation for the effective fast P-wavenumber
k
P
can
be obtained by using
k
Ps
in place of the background slow P-wavenumber k
Ps
in the
expressions for
k
P
. Numerical results by Muller et al. (2007) show that including the
permeability fluctuations (with correlation lengths of elastic moduli and permeability
fluctuations equal) has the following effects compared with the homogeneous per-
meability case: the maximum attenuation peak shifts to slightly lower frequencies;
the magnitude of maximum attenuation is slightly reduced; there is a broadening of
the attenuation peak; and phase velocities are slightly faster.
Uses
The results described in this section can be used to estimate velocity dispersion and
attenuation caused by fluid-flow effects in heterogeneous porous media.
337 6.19 Heterogeneous poroelastic media
Assumptions and limitations
The equations described in this section apply under the following conditions:
linear poroelastic media;
small fluctuations in the material properties of the heterogeneous medium. Numerical
tests in one dimension indicate that the model works for relative contrasts of at least 30%;
isotropic spatial autocorrelation function of the fluctuations; and
low-frequency approximation. The scale of the heterogeneities is smaller than the
wavelength, but larger than the pore scale.
6.20 Waves in a pure viscous fluid
Synopsis
Acoustic waves in a pure viscous fluid are dispersive and attenuate because of the
shear component in the wave-induced deformation of an elementary fluid volume.
The linearized wave equation in a viscous fluid can be derived from the Navier–
Stokes equation (Schlichting, 1951)as
]
2
u
]t
2
¼ c
2
0
]
2
u
]x
2
þ
4
3
]
3
u
]x
2
]t
c
2
0
¼
K
f
Then the wavenumber-to-angular-frequency ratio is
k
o
¼
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
c
2
0
þ igo
c
4
0
þ g
2
o
2
s
¼
e
i arctan z=2
c
0
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
4
p
¼
1
c
0
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
4
p
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
þ 1
2
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
s
þ i
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
1
2
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
s
0
@
1
A
g ¼
4
3
; z ¼
go
c
2
0
and the phase velocity, attenuation coefficient, and inverse quality factor are
V ¼ c
0
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
2ð1 þ z
2
Þ
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
þ 1
s
a ¼
o
c
0
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
1
2ð1 þ z
2
Þ
s
Q
1
¼ 2
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
1
ffiffiffiffiffiffiffiffiffiffiffiffi
1 þ z
2
p
þ 1
s
338 Fluid effects on wave propagation
If z 1 these expressions can be simplified to
V ¼ c
0
1 þ 2
4pf
3c
2
0
2
"#
a ¼
8p
2
f
2
3c
3
0
Q
1
¼
8pf
3c
2
0
where u is displacement, r is density, is viscosity, K
f
is the bulk modulus of the
fluid, x is a coordinate, t is time, k is the wavenumber, o is angular frequency, V is the
phase velocity, a is an attenuation coefficient, Q is the quality factor, and f is
frequency.
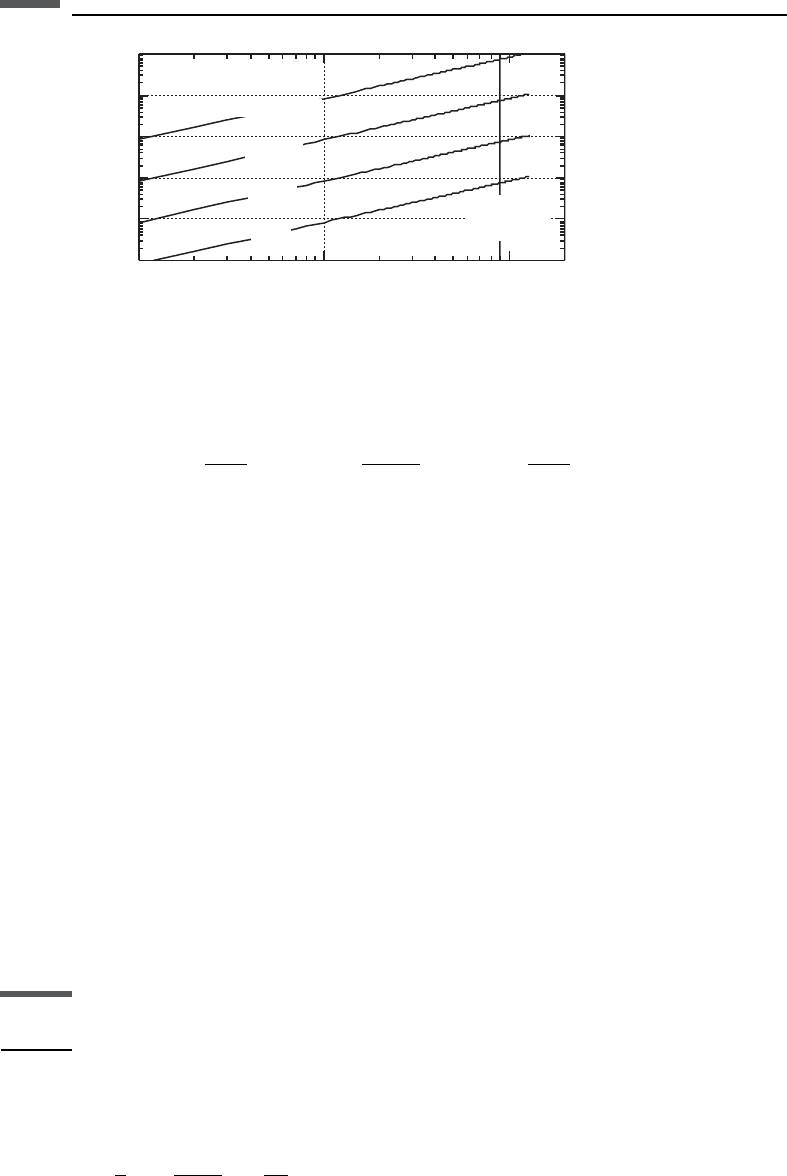
Attenuation is very small at low frequency and for a low-viscosity fluid; however,
it may become noticeable in high-viscosity fluids (Figure 6.20.1).
Uses
The equations presented in this section can be used for estimating the frequency
dependence of the acoustic velocity in viscous fluids.
Assumptions and limitations
The preceding equations assume that the fluid is Newtonian. Many high-viscosity oils
are non-Newtonian (i.e., their flow cannot be accurately described by the Navier–
Stokes equation).
6.21 Physical properties of gases and fluids
Synopsis
The bulk modulus (K) of a fluid or a gas is defined as
K ¼
1
b
¼
dP
dV=V
¼
dP
d
10
−2
10
−3
10
−4
10
−5
10
−6
10
−7
10
4
10
5
10
6
Q
−1
Frequency (Hz)
1 cPs
10 cPs
100 cPs
Viscosity = 1000 cPs
c
0
= 1 km/s
r = 1 g/cm
3
Figure 6.20.1 Inverse quality factor for acoustic waves in viscous fluid.
339 6.21 Physical properties of gases and fluids

where b is compressibility, P is pressure, and V is volume. For small pressure
variations (typical for wave propagation), the pressure variation is related to the
density variation through the acoustic velocity c
0
(which is 1500 m/s for water at
room conditions) as follows:
dP ¼ c
2
0
d:
Therefore,
K ¼ c
2
0
:
Batzle and Wang (1992) have summarized some important properties of reservoir
fluids.
Brine
The density of brine r
B
of salinity S of sodium chloride is
B
¼
w
þ Sf0:668 þ 0:44S þ 10
6
½300P 2400PS
þ Tð80 þ 3T 3300S 13P þ 47PSÞg
where the density of pure water (r
w
)is
w
¼1 þ 10
6
ð80T 3:3T
2
þ 0:00175 T
3
þ 489P 2TP þ 0:016T
2
P 1:3 10
5
T
3
P
0:333P
2
0:002TP
2
Þ
In these formulas pressure P is in MPa, temperature T is in degrees Celsius, salinity S
is in fractions of one (parts per million divided by 10
6
), and density (r
B
and r
w
)is
in g/cm
3
.
The acoustic velocity in brine V
B
in m/s is
V
B
¼V
w
þ Sð1170 9:6T þ 0:055T
2
8:5 10
5
T
3
þ 2:6P 0:0029TP 0:0476P
2
Þ
þ S
3=2
ð780 10P þ 0:16P
2
Þ1820S
2
where the acoustic velocity in pure water V
w
in m/s is
V
w
¼
X
4
i¼0
X
3
j¼0
o
ij
T
i
P
j
and coefficients o
ij
are
340 Fluid effects on wave propagation

o
00
¼ 1402.85 o
02
¼ 3.437 10
–3
o
10
¼ 4.871 o
12
¼ 1.739 10
–4
o
20
¼ –0.04783 o
22
¼ –2.135 10
–6
o
30
¼ 1.487 10
–4
o
32
¼ –1.455 10
–8
o
40
¼ –2.197 10
–7
o
42
¼ 5.230 10
–11
o
01
¼ 1.524 o
03
¼ –1.197 10
–5
o
11
¼ –0.0111 o
13
¼ –1.628 10
–6
o
21
¼ 2.747 10
–4
o
23
¼ 1.237 10
–8
o
31
¼ –6.503 10
–7
o
33
¼ 1.327 10
–10
o
41
¼ 7.987 10
–10
o
43
¼ –4.614 10
–13
We define the gas–water ratio R
G
as the ratio of the volume of dissolved gas at
standard conditions to the volume of brine. Then, for temperatures below 250
C, the
maximum amount of methane that can go into solution in brine is
log
10
ðR
G
Þ¼log
10
ð0:712PjT 76:71j
1:5
þ 3676P
0:64
Þ
4 7:786SðT þ 17:78Þ
0:306
If K
B
is the bulk modulus of the gas-free brine, and K
G
is that of brine with gas–water
ratio R
G
, then
K
B
K
G
¼ 1 þ 0:0494R
G
(i.e., the bulk modulus decreases linearly with increasing gas content). Experimental
data are sparse for the density of brine, but the consensus is that the density is almost
independent of the amount of dissolved gas.
The viscosity of brine in cPs for temperatures below 250
Cis
¼ 0:1 þ 0:333S þð1:65 þ 91:9S
3
Þexpf½0:42ðS
0:8
0:17Þ
2
þ 0:045T
0:8
g
Gas
Natural gas is characterized by its gravity G, which is the ratio of gas density to air
density at 15.6
C and atmospheric pressure. The gravity of methane is 0.56. The
gravity of heavier gases may be as large as 1.8. Algorithms for calculating the gas
density and the bulk modulus follow.
Step 1: Calculate absolute temperature T
a
as
T
a
¼ T þ 273:15
where T is in degrees Celsius.
341 6.21 Physical properties of gases and fluids

Step 2: Calculate the pseudo-pressure P
r
and the pseudo-temperature T
r
as
P
r
¼
P
4:892 0:4048G
T
r
¼
T
a
94:72 þ 170:75G
where pressure is in MPa.
Step 3: Calculate density r
G
in g/cm
3
as
G
28:8GP
ZRT
a
Z ¼ aP
r
þ b þ EE¼ cd
d ¼ exp 0:45 þ 80:56
1
T
r
2
"#
P
1:2
r
T
r
()
c ¼ 0:109ð3:85 T
r
Þ
2
b ¼ 0:642T
r
0:007T
4
r
0:52
a ¼ 0:03 þ 0:00527ð3:5 T
r
Þ
3
R ¼ 8:314 41 J=ðg mol degÞðgas constantÞ
Step 4: Calculate the adiabatic bulk modulus K
G
in MPa as
K
G
Pg
1 P
r
=ðZf Þ
; g ¼ 0:85 þ
5:6
P
r
þ 2
þ
27:1
ðP
r
þ 3:5Þ
2
8:7e
0:65ðP
r
þ1Þ
f ¼ cdm þ a; m ¼ 1:2 0:45 þ 80:56
1
T
r
2
"#
P
0:2
r
T
r
()
The preceding approximate expressions for r
G
and K
G
are valid as long as P
r
and T
r
are not both within 0.1 of unity.
Oil
Oil density under room conditions may vary from under 0.5 to 1 g/cm
3
, and most
produced oils are in the 0.7–0.8 g/cm
3
range. A reference (standard) density that can
be used to characterize an oil r
0
is measured at 15.6
C and atmospheric pressure.
A widely used classification of crude oil is the American Petroleum Institute’s oil
gravity (API gravity). It is defined as
API ¼
141:5
0
131:5
where density is in g/cm
3
. API gravity may be about 5 for very heavy oils and about
100 for light condensates.
342 Fluid effects on wave propagation

Acoustic velocity V
P
in oil may generally vary with temperature T and molecular
weight M:
V
P
ðT; MÞ¼V
0
bT a
m
1
M
1
M
0
1
b
¼ 0:306
7:6
M
In this formula, V
0
is the velocity of oil of molecular weight M
0
at temperature T
0
; a
m
is a positive function of temperature, and thus oil velocity increases with molecular
weight. When components are mixed, velocity can be approximately calculated as a
fractional average of the end components.
For dead oil (oil with no dissolved gas), the effects of pressure and temperature on
density are largely independent. The pressure dependence is
P
¼
0
þð0:00277P 1:71 10
7
P
3
Þð
0
1:15Þ
2
þ 3:49 10
4
P
where r
P
is the density in g/cm
3
at pressure P in MPa. Temperature dependence of
density at a given pressure P is
¼
P
=½0:972 þ 3:81 10
4
ðT þ 17:78Þ
1:175
where temperature is in degrees Celsius.
The acoustic velocity in dead oil depends on pressure and temperature as
V
P
ðm=sÞ¼2096
0
2:6
0
1=2
3:7T þ 4:64P
þ 0:0115½4:12ð1:08
0
1
1Þ
1:2
1TP
or, in terms of API gravity as
V
P
ðft=sÞ¼15450ð77:1 þ APIÞ
1=2
3:7T þ 4:64P
þ 0:0115½0:36API
1=2
1TP
Live oil
Large amounts of gas can be dissolved in an oil. The original fluid in situ is
usually characterized by R
G
, the volume ratio of liberated gas to remaining oil at
atmospheric pressure and 15.6
C. The maximum amount of gas that can be dissolved
in an oil is a function of pressure, temperature, and the composition of both the gas
and the oil:
R
ðmaxÞ
G
¼ 0:02123 GPexp
4:072
0
0:003 77 T
1:205
343 6.21 Physical properties of gases and fluids

or, in terms of API gravity:
R
ðmaxÞ
G
¼ 2:03G½P expð0:02878 API 0:00377 TÞ
1:205
where R
G
is in liters/liter (1 L/L ¼5.615 ft
3
/bbl) and G is the gas gravity. Temperature
is in degrees Celsius, and pressure is in MPa.
Velocities in oils with dissolved gas can still be calculated versus pressure and
temperature as follows, by using the preceding formulas with a pseudo-density r
0
used instead of r
0
:
0
¼
0
B
0
ð1 þ 0:001 R
G
Þ
1
B
0
¼0:972 þ0 :00038 2:4R
G
G
0
1=2
þ T þ 17:8
"#
1:175
The true density of oil with gas (in g/cm
3
) at saturation can be calculated as
G
¼ð
0
þ 0:0012GR
G
Þ=B
0
For temperatures and pressures that differ from those at saturation, r
G
must be
adjusted using the temperature and pressure corrections described in the section
above on dead oil.
Calculate the density and acoustic velocity of live oil of 30 API gravity at 80
C
and 20 MPa. The gas–oil ratio R
G
is 100, and the gas gravity is 0.6.
Calculate r
0
from API as
0
¼
141:5
API þ 131:5
¼
141:5
30 þ 131:5
¼ 0:876 g=cm
3
Calculate B
0
as
B
0
¼0:972 þ 0:000 38 2:4R
G
G
0
1=2
þ T þ 17:8
"#
1:175
B
0
¼0:972 þ 0:000 38 2:4 100
0:6
0:876
1=2
þ 80 þ 17:8
"#
1:175
¼1:2770
Calculate the pseudo-density r
0
as
0
¼
0
B
0
ð1 þ 0:001R
G
Þ
1
¼ 0:623 g=cm
3
344 Fluid effects on wave propagation

Calculate the density of oil with gas r
G
as
G
¼ð
0
þ 0:0012GR
G
Þ=B
0
¼ 0:7424 g=cm
3
Correct this density for pressure and find the density r
P
as
P
¼
G
þð0:002 77 P 1:71 10
7
P
3
Þð
G
1:15Þ
2
þ 3:49 10
4
P
¼0:742 þð0:002 77 20 1:71 10
7
20
3
Þð0:742 1:15Þ
2
þ 3:49 10
4
20 ¼ 0:758 g=cm
3
Finally, apply the temperature correction to obtain the actual density as
¼
P
=½0:972 þ 3:81 10
4
ðT þ 17:78Þ
1:175
¼0:758=½0:972 þ 3:81 10
4
ð80 þ 17 :78Þ
1:175
¼0:718 g =cm
3
Calculate the velocity in oil with gas V
P
as
V
P
¼2096
0
2:6
0
1=2
3:7T þ 4:64P
þ 0:0115 4:12ð1:08=
0
1Þ
1=2
1
hi
TP
¼2096
0:623
2:6 0:623
1=2
3:7 80 þ 4:64 20
þ 0:0115 4:12ð1:08=0:623 1Þ
1=2
1
hi
80 20
¼1020 m=s
The viscosity of dead oil (
h
) decreases rapidly with increasing temperature.
At room pressure for a gas-free oil we have
log
10
ð þ 1Þ¼0:505yð17:8 þ TÞ
1:163
log
10
ðyÞ¼5:693 2:863=
0
Pressure has a smaller influence on viscosity and can be estimated independently
of the temperature influence. If oil viscosity is
0
at a given temperature and room
pressure, its viscosity at pressure P and the same temperature is
¼
0
þ 0:145PI
log
10
ðIÞ¼18:6½0:1 log
10
ð
0
Þþðlog
10
ð
0
Þþ2Þ
0:1
0:985
345 6.21 Physical properties of gases and fluids

Viscosity in these formulas is in centipoise, temperature is in degrees Celsius, and
pressure is in MPa.
Uses
The equations presented in this section are used to estimate acoustic velocities and
densities of pore fluids.
Assumptions and limitations
The formulas are mostly based on empirical measurements summarized by Batzle
and Wang (1992).
346 Fluid effects on wave propagation
