Munson B.R. Fundamentals of Fluid Mechanics

Подождите немного. Документ загружается.

6.68 Water flows around a 6-ft-diameter bridge pier with a velocity

of Estimate the force (per unit length) that the water exerts on

the pier. Assume that the flow can be approximated as an ideal fluid

flow around the front half of the cylinder, but due to flow separation

(see Video V6.8), the average pressure on the rear half is constant

and approximately equal to

⁄2 the pressure at point A (see Fig. P6.68).

12 ft/s.

calculate the circulation by assuming the air sticks to the rotating

cylinders. Note: This calculated force is at right angles to the direc-

tion of the wind and it is the component of this force in the direction

of motion of the ship that gives the propulsive thrust. Also, due to

viscous effects, the actual propulsive thrust will be smaller than that

calculated from Eq. 6.124 which is based on inviscid flow theory.

6.73 A fixed circular cylinder of infinite length is placed in a

steady, uniform stream of an incompressible, nonviscous fluid. As-

sume that the flow is irrotational. Prove that the drag on the cylinder

is zero. Neglect body forces.

6.74 Repeat Problem 6.73 for a rotating cylinder for which the

stream function and velocity potential are given by Eqs. 6.119 and

6.120, respectively. Verify that the lift is not zero and can be ex-

pressed by Eq. 6.124.

6.75 At a certain point at the beach, the coast line makes a right-

angle bend, as shown in Fig. P6.75a. The flow of salt water in this

bend can be approximated by the potential flow of an incompress-

ible fluid in a right-angle corner. (a) Show that the stream function

for this flow is where A is a positive constant.

(b)A fresh-water reservoir is located in the corner. The salt water is

to be kept away from the reservoir to avoid any possible seepage of

salt water into the fresh water (Fig. P6.75b). The fresh-water source

can be approximated as a line source having a strength m, where m

is the volume rate of flow (per unit length) emanating from the

source. Determine m if the salt water is not to get closer than a dis-

tance L to the corner. Hint: Find the value of m (in terms of A and

L) so that a stagnation point occurs at (c) The streamline

passing through the stagnation point would represent the line dividing

the fresh water from the salt water. Plot this streamline.

y  L.

c  A r

sin 2u,

Problems

327

U, p

F I G U R E P6.67

U = 12 ft/s

6 ft

F I G U R E P6.68

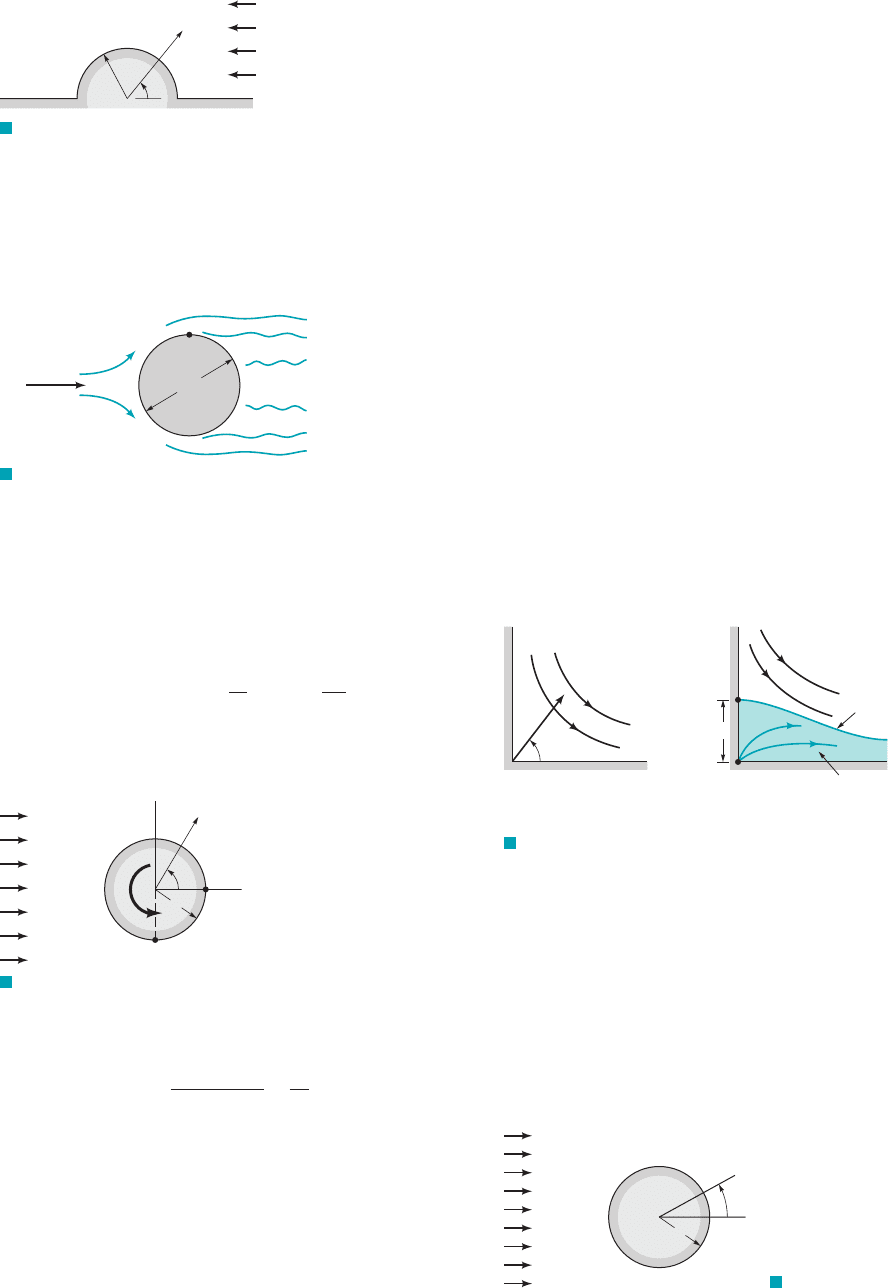
*6.69 Consider the steady potential flow around the circular cylin-

der shown in Fig. 6.26. On a plot show the variation of the magni-

tude of the dimensionless fluid velocity, along the positive

y axis. At what distance, 1along the y axis2, is the velocity within

1% of the free-stream velocity?

6.70 The velocity potential for a cylinder 1Fig. P6.702rotating in a

uniform stream of fluid is

where is the circulation. For what value of the circulation will the

stagnation point be located at: (a) point A, (b) point B?



f  Ur a1 

b cos u 





F I G U R E P6.70

6.71 Show that for a rotating cylinder in a uniform flow, the fol-

lowing pressure ratio equation is true.

Here U is the velocity of the uniform flow and qis the surface speed

of the rotating cylinder.

6.72 (See Fluids in the News article titled “A sailing ship without

sails,” Section 6.6.3.) Determine the magnitude of the total force

developed by the two rotating cylinders on the Flettner “rotor-ship”

due to the Magnus effect. Assume a wind speed relative to the ship

of (a) 10 mph and (b) 30 mph. Each cylinder has a diameter of 9 ft,

a length of 50 ft, and rotates at 750 rev/min. Use Eq. 6.124 and

top

 p

bottom

stagnation



Salt water

Dividing

streamline

Fresh water

source

(b)(a)

F I G U R E P6.75

6.76 Typical inviscid flow solutions for flow around bodies indi-

cate that the fluid flows smoothly around the body, even for blunt

bodies as shown in Video V6.10. However, experience reveals that

due to the presence of viscosity, the main flow may actually separate

from the body creating a wake behind the body. As discussed in a

later section (Section 9.2.6), whether or not separation takes place

depends on the pressure gradient along the surface of the body, as

calculated by inviscid flow theory. If the pressure decreases in the

direction of flow (a favorable pressure gradient), no separation will

occur. However, if the pressure increases in the direction of flow (an

adverse pressure gradient), separation may occur. For the circular

cylinder of Fig. P6.76 placed in a uniform stream with velocity, U,

F I G U R E P6.76

JWCL068_ch06_263-331.qxd 9/23/08 12:21 PM Page 327

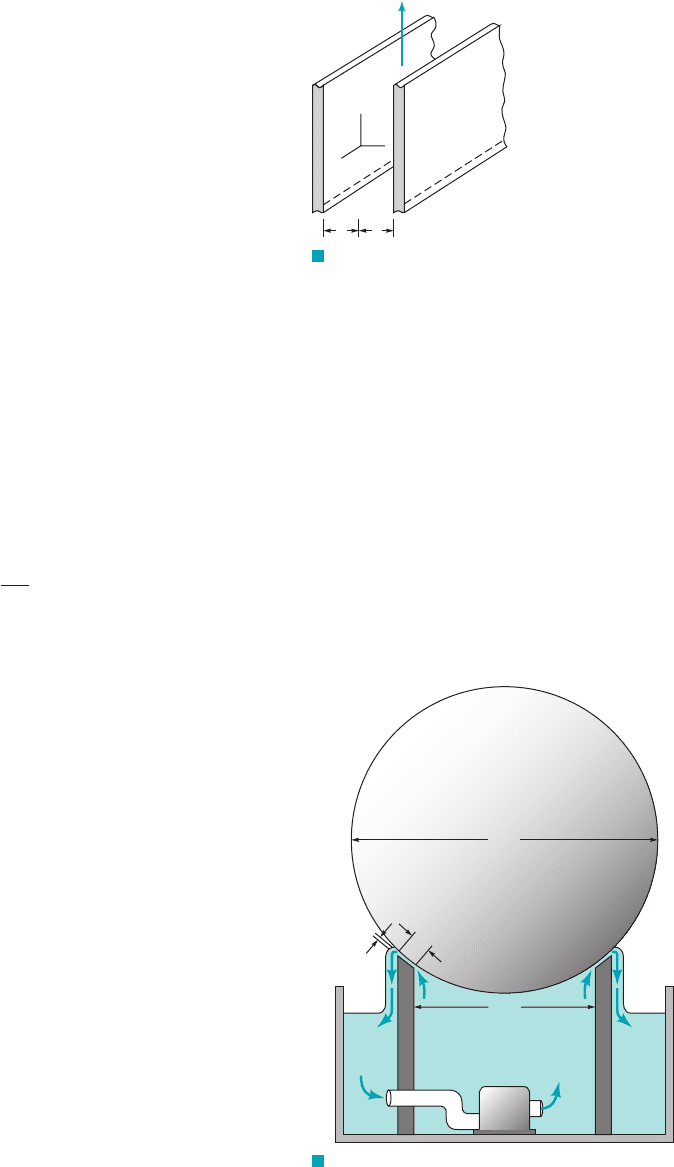
determine an expression for the pressure gradient in the direction of

flow on the surface of the cylinder. For what range of values for the

angle will an adverse pressure gradient occur?

Section 6.8 Viscous Flow

6.77 Obtain a photograph/image of a situation in which the cylin-

drical form of the Navier–Stokes equations would be appropriate

for the solution. Print this photo and write a brief paragraph that de-

scribes the situation involved.

6.78 For a steady, two-dimensional, incompressible flow, the ve-

locity is given by , where a and c

are constants. Show that this flow can be considered inviscid.

6.79 Determine the shearing stress for an incompressible Newtonian

fluid with a velocity distribution of

6.80 The two-dimensional velocity field for an incompressible

Newtonian fluid is described by the relationship

where the velocity has units of m/s when x and y are in me-

ters. Determine the stresses and at the point

if pressure at this point is 6 kPa and the fluid is

glycerin at Show these stresses on a sketch.

6.81 For a two-dimensional incompressible flow in the x  y plane

show that the z component of the vorticity, varies in accordance

with the equation

What is the physical interpretation of this equation for a nonviscous

fluid? Hint: This vorticity transport equation can be derived from

the Navier–Stokes equations by differentiating and eliminating the

pressure between Eqs. 6.127a and 6.127b.

6.82 The velocity of a fluid particle moving along a horizontal

streamline that coincides with the x axis in a plane, two-dimensional,

incompressible flow field was experimentally found to be described

by the equation Along this streamline determine an expres-

sion for (a) the rate of change of the component of velocity with re-

spect to y, (b) the acceleration of the particle, and (c) the pressure

gradient in the x direction. The fluid is Newtonian.

Section 6.9.1 Steady, Laminar Flow between Fixed

Parallel Plates

6.83 Obtain a photograph/image of a situation which can be approx-

imated by one of the simple cases covered in Sec. 6.9. Print this photo

and write a brief paragraph that describes the situation involved.

6.84 Oil flows between two fixed horizontal

infinite parallel plates with a spacing of 5 mm. The flow is laminar

and steady with a pressure gradient of per unit meter.

Determine the volume flowrate per unit width and the shear stress

on the upper plate.

6.85 Two fixed, horizontal, parallel plates are spaced 0.4 in. apart.

A viscous liquid flows be-

tween the plates with a mean velocity of . The flow is lami-

nar. Determine the pressure drop per unit length in the direction of

flow. What is the maximum velocity in the channel?

6.86 A viscous, incompressible fluid flows between the two infi-

nite, vertical, parallel plates of Fig. P6.86. Determine, by use of the

Navier–Stokes equations, an expression for the pressure gradient in

the direction of flow. Express your answer in terms of the mean ve-

locity. Assume that the flow is laminar, steady, and uniform.

0.5 ft



1m  8  10

3



, SG  0.92

900 1N



1m  0.4 N ⴢ s



u  x

 n§

20 °C.

0.5 m, y  1.0 m

x t

, s

V  112xy

 6x

 118x

y  4y

112x

y  y

V  13xy

 4x



V  1ax  cy2i

 1ay  cx2j

328 Chapter 6 ■ Differential Analysis of Fluid Flow

6.87 A fluid is initially at rest between two horizontal, infinite,

parallel plates. A constant pressure gradient in a direction parallel

to the plates is suddenly applied and the fluid starts to move. Deter-

mine the appropriate differential equation1s2, initial condition, and

boundary conditions that govern this type of flow. You need not

solve the equation1s2.

6.88 (See Fluids in the News article titled “10 tons on 8 psi,” Section

6.9.1.) A massive, precisely machined, 6-ft-diameter granite sphere

rests upon a 4-ft-diameter cylindrical pedestal as shown in Fig.

P6.88. When the pump is turned on and the water pressure within

the pedestal reaches 8 psi, the sphere rises off the pedestal, creating

a 0.005-in. gap through which the water flows. The sphere can then

be rotated about any axis with minimal friction. (a) Estimate the

pump flowrate, , required to accomplish this. Assume the flow in

the gap between the sphere and the pedestal is essentially viscous

flow between fixed, parallel plates. (b) Describe what would hap-

pen if the pump flowrate were increased to 2Q

Direction of flow

F I G U R E P6.86

F I G U R E P6.88

0.005 in.

4 in.

6 ft

4 ft

p  8 psi

Pump

Section 6.9.2 Couette Flow

6.89 Two horizontal, infinite, parallel plates are spaced a distance

b apart. A viscous liquid is contained between the plates. The bot-

tom plate is fixed, and the upper plate moves parallel to the bottom

plate with a velocity U. Because of the no-slip boundary condition

JWCL068_ch06_263-331.qxd 9/23/08 12:21 PM Page 328

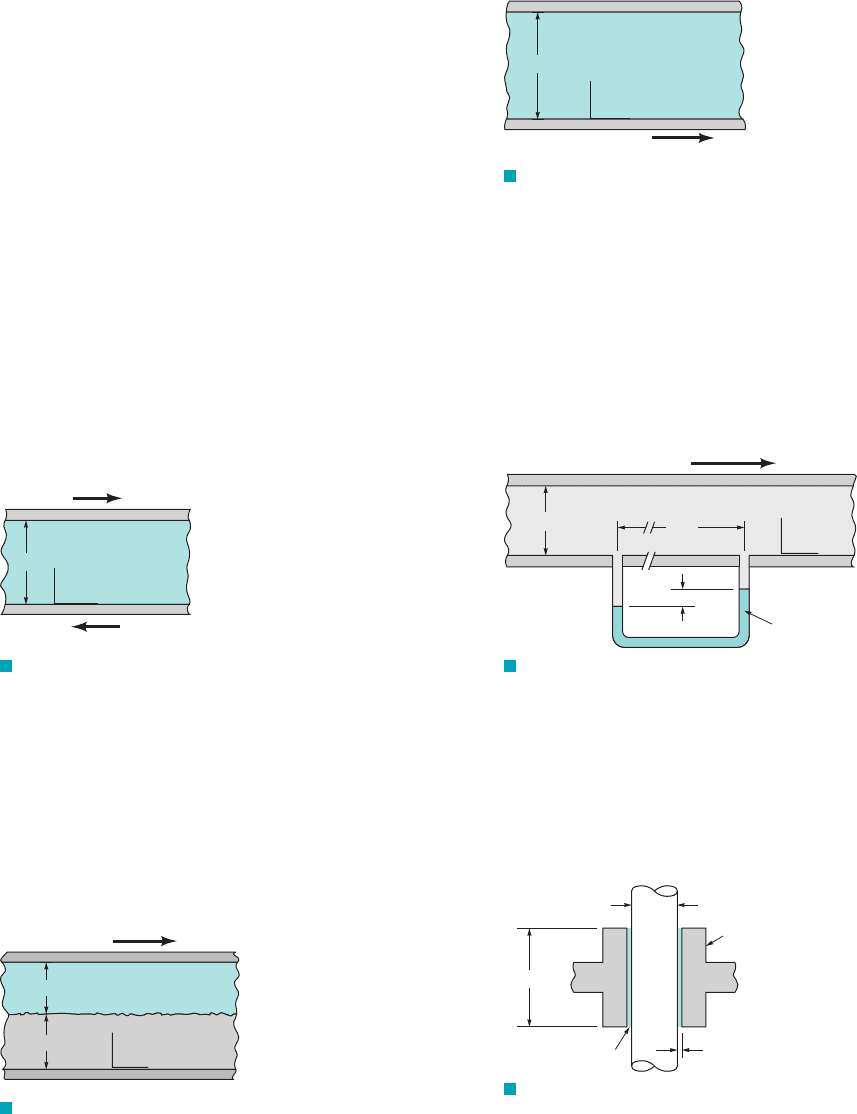
(see Video V6.11), the liquid motion is caused by the liquid being

dragged along by the moving boundary. There is no pressure gra-

dient in the direction of flow. Note that this is a so-called simple

Couette flow discussed in Section 6.9.2. (a) Start with the

Navier–Stokes equations and determine the velocity distribution

between the plates. (b) Determine an expression for the flowrate

passing between the plates (for a unit width). Express your answer

in terms of b and U.

6.90 A layer of viscous liquid of constant thickness 1no velocity

perpendicular to plate2flows steadily down an infinite, inclined

plane. Determine, by means of the Navier–Stokes equations, the re-

lationship between the thickness of the layer and the discharge per

unit width. The flow is laminar, and assume air resistance is negli-

gible so that the shearing stress at the free surface is zero.

6.91 Due to the no-slip condition, as a solid is pulled out of a vis-

cous liquid some of the liquid is also pulled along as described in

Example 6.9 and shown in Video V6.11. Based on the results given

in Example 6.9, show on a dimensionless plot the velocity distribu-

tion in the fluid film when the average film velocity,

V, is of the belt velocity,

6.92 An incompressible, viscous fluid is placed between hori-

zontal, infinite, parallel plates as is shown in Fig. P6.92. The two

plates move in opposite directions with constant velocities,

and as shown. The pressure gradient in the x direction is zero,

and the only body force is due to the fluid weight. Use the Navier–

Stokes equations to derive an expression for the velocity distribu-

tion between the plates. Assume laminar flow.

.10%

vs. x

Problems

329

F I G U R E P6.92

6.93 Two immiscible, incompressible, viscous fluids having the

same densities but different viscosities are contained between two

infinite, horizontal, parallel plates 1Fig. P6.932. The bottom plate is

fixed and the upper plate moves with a constant velocity U. Deter-

mine the velocity at the interface. Express your answer in terms of

U, and The motion of the fluid is caused entirely by the

movement of the upper plate; that is, there is no pressure gradient

in the x direction. The fluid velocity and shearing stress are contin-

uous across the interface between the two fluids. Assume laminar

flow.

ρμ

Fixed

plate

F I G U R E P6.93

Fixed

plate

F I G U R E P6.94

0.1 in.

6 in.

1.0 in.

U = 0.02 ft/s

= 100 lb/ft

Fixed

plate

F I G U R E P6.95

6.94 The viscous, incompressible flow between the parallel plates

shown in Fig. P6.94 is caused by both the motion of the bottom

plate and a pressure gradient, As noted in Section 6.9.2, an

important dimensionless parameter for this type of problem is

Ⲑ

0x.

( ) where is the fluid viscosity. Make a plot

of the dimensionless velocity distribution (similar to that shown in

Fig. 6.32b) for For this case where does the maximum ve-

locity occur?

P ⫽ 3.

m0p

Ⲑ

0xP ⫽⫺1b

/2 mU2

6.96 A vertical shaft passes through a bearing and is lubricated

with an oil having a viscosity of as shown in Fig.

P6.96. Assume that the flow characteristics in the gap between the

shaft and bearing are the same as those for laminar flow between

infinite parallel plates with zero pressure gradient in the direction

of flow. Estimate the torque required to overcome viscous resis-

tance when the shaft is turning at 80 rev兾min.

0.2 N

Ⲑ

0.25 mm

75 mm

Shaft

Oil

160 mm

Bearing

F I G U R E P6.96

6.97 A viscous fluid is contained between two long concentric

cylinders. The geometry of the system is such that the flow between

the cylinders is approximately the same as the laminar flow between

two infinite parallel plates. (a) Determine an expression for the

torque required to rotate the outer cylinder with an angular velocity v.

6.95 A viscous fluid 1specific weight viscosity

is contained between two infinite, horizontal parallel

plates as shown in Fig. P6.95. The fluid moves between the plates

under the action of a pressure gradient, and the upper plate moves

with a velocity U while the bottom plate is fixed. A U-tube

manometer connected between two points along the bottom indi-

cates a differential reading of 0.1 in. If the upper plate moves with

a velocity of 0.02 ft兾s, at what distance from the bottom plate does

the maximum velocity in the gap between the two plates occur? As-

sume laminar flow.

0.03 lb

Ⲑ

⫽⫽ 80 lb

Ⲑ

;

JWCL068_ch06_263-331.qxd 9/23/08 12:21 PM Page 329

The inner cylinder is fixed. Express your answer in terms of the

geometry of the system, the viscosity of the fluid, and the angular ve-

locity. (b) For a small, rectangular element located at the fixed wall

determine an expression for the rate of angular deformation of this

element. (See Video V6.3 and Fig. P6.9.)

*6.98 Oil 1SAE 302flows between parallel plates spaced 5 mm apart.

The bottom plate is fixed, but the upper plate moves with a velocity of

0.2 in the positive xdirection. The pressure gradient is 60

and it is negative. Compute the velocity at various points across the

channel and show the results on a plot. Assume laminar flow.

Section 6.9.3 Steady, Laminar Flow in Circular Tubes

6.99 Consider a steady, laminar flow through a straight horizontal

tube having the constant elliptical cross section given by the equation

The streamlines are all straight and parallel. Investigate the possibil-

ity of using an equation for the z component of velocity of the form

as an exact solution to this problem. With this velocity distribution,

what is the relationship between the pressure gradient along the

tube and the volume flowrate through the tube?

6.100 A simple flow system to be used for steady flow tests con-

sists of a constant head tank connected to a length of 4-mm-

diameter tubing as shown in Fig. P6.100. The liquid has a viscosity

of a density of and discharges into the

atmosphere with a mean velocity of . (a) Verify that the flow

will be laminar. (b) The flow is fully developed in the last 3 m of the

tube. What is the pressure at the pressure gage? (c) What is the mag-

nitude of the wall shearing stress, in the fully developed region?t

2 m

Ⲑ

1200 kg

Ⲑ

,0.015 N

Ⲑ

w ⫽ A a1 ⫺

⫺

⫹

⫽ 1

kPa

Ⲑ

m,m

Ⲑ

330 Chapter 6 ■ Differential Analysis of Fluid Flow

Pressure

gage

3 m

Diameter = 4 mm

F I G U R E P6.100

6.101 (a) Show that for Poiseuille flow in a tube of radius R the

magnitude of the wall shearing stress, can be obtained from the

relationship

for a Newtonian fluid of viscosity The volume rate of flow is Q.

(b) Determine the magnitude of the wall shearing stress for a fluid

having a viscosity of flowing with an average veloc-

ity of in a 2-mm-diameter tube.

6.102 An infinitely long, solid, vertical cylinder of radius R is lo-

cated in an infinite mass of an incompressible fluid. Start with the

Navier–Stokes equation in the direction and derive an expression

for the velocity distribution for the steady flow case in which the

cylinder is rotating about a fixed axis with a constant angular

velocity You need not consider body forces. Assume that the

flow is axisymmetric and the fluid is at rest at infinity.

130 mm

Ⲑ

0.004 N

Ⲑ

01t

wall

0⫽

4mQ

*6.103 As is shown by Eq. 6.150 the pressure gradient for laminar

flow through a tube of constant radius is given by the expression

For a tube whose radius is changing very gradually, such as the one

illustrated in Fig. P6.103, it is expected that this equation can be

used to approximate the pressure change along the tube if the actual

radius, R1z2, is used at each cross section. The following measure-

ments were obtained along a particular tube.

0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0

1.00 0.73 0.67 0.65 0.67 0.80 0.80 0.71 0.73 0.77 1.00

Compare the pressure drop over the length for this nonuniform

tube with one having the constant radius Hint: To solve this

problem you will need to numerically integrate the equation for the

pressure gradient given above.

R1z2

Ⲑ

⫽⫺

8mQ

(z)

ᐉ

F I G U R E P6.103

2 m

4 mm

Δh

Density of

gage fluid = 2000 kg/m

F I G U R E P6.104

6.104 A liquid (viscosity ⫽ 0.002 N⭈s/m

; density ⫽ 1000 kg/m

)

is forced through the circular tube shown in Fig. P6.104. A differ-

ential manometer is connected to the tube as shown to measure the

pressure drop along the tube. When the differential reading, ⌬h, is

9 mm, what is the mean velocity in the tube?

Section 6.9.4 Steady,Axial, Laminar Flow in an Annulus

6.105 An incompressible Newtonian fluid flows steadily between

two infinitely long, concentric cylinders as shown in Fig. P6.105.

The outer cylinder is fixed, but the inner cylinder moves with a lon-

gitudinal velocity as shown. The pressure gradient in the axial

direction is . For what value of will the drag on the inner

cylinder be zero? Assume that the flow is laminar, axisymmetric,

and fully developed.

⫺¢p

Ⲑ

Fixed wall

F I G U R E P6.105

JWCL068_ch06_263-331.qxd 9/30/08 8:21 AM Page 330

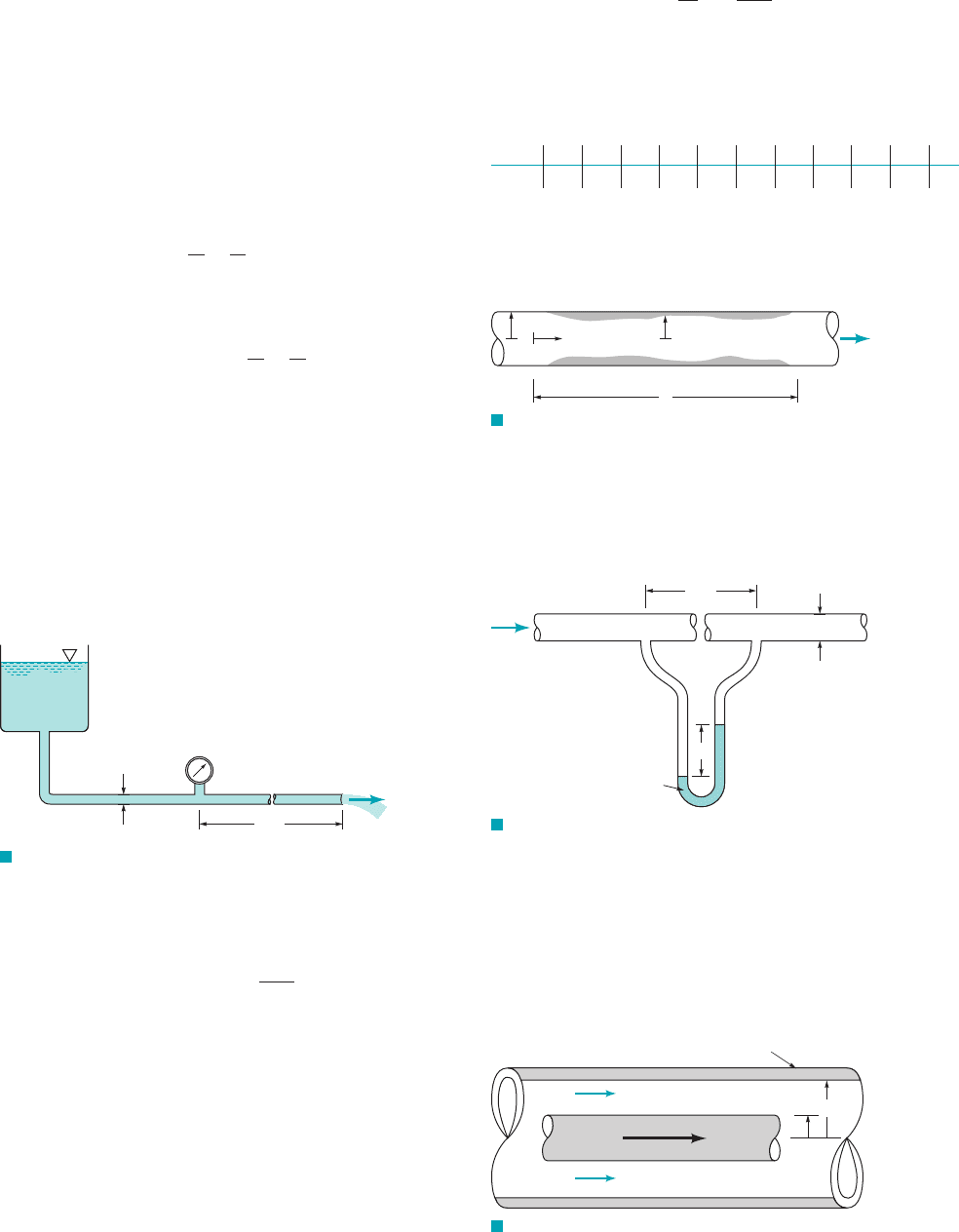
6.106 A viscous fluid is contained between two infinitely long,

vertical, concentric cylinders. The outer cylinder has a radius and

rotates with an angular velocity The inner cylinder is fixed and

has a radius Make use of the Navier–Stokes equations to obtain

an exact solution for the velocity distribution in the gap. Assume

that the flow in the gap is axisymmetric 1neither velocity nor pres-

sure are functions of angular position within the gap2and that

there are no velocity components other than the tangential compo-

nent. The only body force is the weight.

6.107 For flow between concentric cylinders, with the outer cylinder

rotating at an angular velocity and the inner cylinder fixed, it is

commonly assumed that the tangential velocity distribution in the

gap between the cylinders is linear. Based on the exact solution to this

problem 1see Problem 6.1062the velocity distribution in the gap is not

linear. For an outer cylinder with radius and an inner

cylinder with radius 1.80 in., show, with the aid of a plot, how the

dimensionless velocity distribution, varies with the dimen-

sionless radial position, for the exact and approximate solutions.

6.108 A viscous liquid

flows through the annular space between two horizontal, fixed, con-

centric cylinders. If the radius of the inner cylinderis 1.5 in. and the ra-

dius of the outer cylinder is 2.5 in., what is the pressure drop along the

axis of the annulus per foot when the volume flowrate is

6.109 Show how Eq. 6.155 is obtained.

6.110 A wire of diameter d is stretched along the centerline of a

pipe of diameter D. For a given pressure drop per unit length of

0.14 ft

Ⲑ

slugs

Ⲑ

2r ⫽ 1.791m ⫽ 0.012 lb

Ⲑ

⫽

⫽ 2.00 in.

Problems 331

pipe, by how much does the presence of the wire reduce the

flowrate if (a) (b)

Section 6.10 Other Aspects of Differential Analysis

6.111 Obtain a photograph/image of a situation in which CFD has

been used to solve a fluid flow problem. Print this photo and write

a brief paragraph that describes the situation involved.

■ Life Long Learning Problems

6.112 What sometimes appear at first glance to be simple fluid

flows can contain subtle, complex fluid mechanics. One such ex-

ample is the stirring of tea leaves in a teacup. Obtain information

about “Einstein’s tea leaves” and investigate some of the complex

fluid motions interacting with the leaves. Summarize your findings

in a brief report.

6.113 Computational fluid dynamics (CFD) has moved from a re-

search tool to a design tool for engineering. Initially, much of the

work in CFD was focused in the aerospace industry, but now has

expanded into other areas. Obtain information on what other indus-

tries (e.g., automotive) make use of CFD in their engineering de-

sign. Summarize your findings in a brief report.

■ FE Exam Problems

Sample FE (Fundamentals of Engineering) exam questions for

fluid mechanics are provided on the book’s web site, www.wiley.

com/college/munson.

Ⲑ

D ⫽ 0.01?d

Ⲑ

D ⫽ 0.1;

JWCL068_ch06_263-331.qxd 9/23/08 12:21 PM Page 331

imensional

Analysis,

Similitude,

and Modeling

imensional

Analysis,

Similitude,

and Modeling

332

CHAPTER OPENING PHOTO: Flow past a circular cylinder with The pathlines of flow

past any circular cylinder 1regardless of size, velocity, or fluid2are as shown provided that the dimension-

less parameter called the Reynolds number, Re, is equal to 2000. For other values of Re, the flow pattern

will be different 1air bubbles in water2. (Photograph courtesy of ONERA, France.)

Re ⫽ rVD

Ⲑ

m ⫽ 2000:

Although many practical engineering problems involving fluid mechanics can be solved by us-

ing the equations and analytical procedures described in the preceding chapters, there remain a

large number of problems that rely on experimentally obtained data for their solution. In fact, it

is probably fair to say that very few problems involving real fluids can be solved by analysis

alone. The solution to many problems is achieved through the use of a combination of theoret-

ical and numerical analysis and experimental data. Thus, engineers working on fluid mechanics

problems should be familiar with the experimental approach to these problems so that they can

interpret and make use of data obtained by others, such as might appear in handbooks, or be

able to plan and execute the necessary experiments in their own laboratories. In this chapter we

consider some techniques and ideas that are important in the planning and execution of experi-

ments, as well as in understanding and correlating data that may have been obtained by other

experimenters.

An obvious goal of any experiment is to make the results as widely applicable as possible.

To achieve this end, the concept of similitude is often used so that measurements made on one

system 1for example, in the laboratory2can be used to describe the behavior of other similar sys-

tems 1outside the laboratory2. The laboratory systems are usually thought of as models and are used

Learning Objectives

After completing this chapter, you should be able to:

■ apply the Buckingham pi theorem.

■ develop a set of dimensionless variables for a given flow situation.

■ discuss the use of dimensionless variables in data analysis.

■ apply the concepts of modeling and similitude to develop prediction equations.

Experimentation

and modeling are

widely used tech-

niques in fluid

mechanics.

V7.1 Real and

model flies

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 332

to study the phenomenon of interest under carefully controlled conditions. From these model studies,

empirical formulations can be developed, or specific predictions of one or more characteristics of

some other similar system can be made. To do this, it is necessary to establish the relationship be-

tween the laboratory model and the “other” system. In the following sections, we find out how this

can be accomplished in a systematic manner.

7.1 Dimensional Analysis 333

Fluids in the News

Model study of New Orleans levee breach caused by

Hurricane Katrina Much of the devastation to New Orleans from

Hurricane Katrina in 2005 was a result of flood waters that surged

through a breach of the 17th Street Outfall Canal. To better under-

stand why this occurred and to determine what can be done to pre-

vent future occurrences, the U.S. Army Engineer Research and

Development Center Coastal and Hydraulics Laboratory is con-

ducting tests on a large (1:50 length scale) 15,000 square foot hy-

draulic model that replicates 0.5 mile of the canal surrounding the

breach and more than a mile of the adjacent Lake Pontchartrain

front. The objective of the study is to obtain information regarding

the effect that waves had on the breaching of the canal and to in-

vestigate the surging water currents within the canals. The waves

are generated by computer-controlled wave generators that can

produce waves of varying heights, periods,and directions similar to

the storm conditions that occurred during the hurricane. Data from

the study will be used to calibrate and validate information that

will be fed into various numerical model studies of the disaster.

To illustrate a typical fluid mechanics problem in which experimentation is required, consider the

steady flow of an incompressible Newtonian fluid through a long, smooth-walled, horizontal, cir-

cular pipe. An important characteristic of this system, which would be of interest to an engineer

designing a pipeline, is the pressure drop per unit length that develops along the pipe as a result

of friction. Although this would appear to be a relatively simple flow problem, it cannot gener-

ally be solved analytically 1even with the aid of large computers2without the use of experimen-

tal data.

The first step in the planning of an experiment to study this problem would be to decide on

the factors, or variables, that will have an effect on the pressure drop per unit length,

We expect the list to include the pipe diameter, D, the fluid den-

sity, , fluid viscosity, , and the mean velocity, V, at which the fluid is flowing through the pipe.

Thus, we can express this relationship as

(7.1)

which simply indicates mathematically that we expect the pressure drop per unit length to be some

function of the factors contained within the parentheses. At this point the nature of the function is

unknown and the objective of the experiments to be performed is to determine the nature of this

function.

To perform the experiments in a meaningful and systematic manner, it would be necessary

to change one of the variables, such as the velocity, while holding all others constant, and mea-

sure the corresponding pressure drop. This series of tests would yield data that could be repre-

sented graphically as is illustrated in Fig. 7.1a. It is to be noted that this plot would only be valid

for the specific pipe and for the specific fluid used in the tests; this certainly does not give us the

general formulation we are looking for. We could repeat the process by varying each of the other

variables in turn, as is illustrated in Figs. 7.1b, 7.1c, and 7.1d. This approach to determining the

functional relationship between the pressure drop and the various factors that influence it, although

logical in concept, is fraught with difficulties. Some of the experiments would be hard to carry

out—for example, to obtain the data illustrated in Fig. 7.1c it would be necessary to vary fluid den-

sity while holding viscosity constant. How would you do this? Finally, once we obtained the var-

ious curves shown in Figs. 7.1a, 7.1b, 7.1c, and 7.1d, how could we combine these data to obtain

the desired general functional relationship between and V which would be valid for

any similar pipe system?

Fortunately, there is a much simpler approach to this problem that will eliminate the diffi-

culties described above. In the following sections we will show that rather than working with the

¢p

, D, r, m,

¢p

⫽ f1D, r, m, V2

¢p

31lb

Ⲑ

ft ⫽ lb

Ⲑ

or N

Ⲑ

7.1 Dimensional Analysis

It is important to

develop a meaning-

ful and systematic

way to perform an

experiment.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 333

original list of variables, as described in Eq. 7.1, we can collect these into two nondimensional

combinations of variables 1called dimensionless products or dimensionless groups2so that

(7.2)

Thus, instead of having to work with five variables, we now have only two. The necessary

experiment would simply consist of varying the dimensionless product and determining

the corresponding value of The results of the experiment could then be represented

by a single, universal curve as is illustrated in Fig. 7.2. This curve would be valid for any com-

bination of smooth-walled pipe and incompressible Newtonian fluid. To obtain this curve we

could choose a pipe of convenient size and a fluid that is easy to work with. Note that we would-

n’t have to use different pipe sizes or even different fluids. It is clear that the experiment would

be much simpler, easier to do, and less expensive 1which would certainly make an impression

on your boss2.

The basis for this simplification lies in a consideration of the dimensions of the variables

involved. As was discussed in Chapter 1, a qualitative description of physical quantities can be given

in terms of basic dimensions such as mass, M, length, L, and time, Alternatively, we could use

force, F, L, and T as basic dimensions, since from Newton’s second law

F ⬟ MLT

⫺2

D ¢p

Ⲑ

rVD

Ⲑ

D ¢p

⫽ f a

rVD

334 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

F I G U R E 7.1 Illus-

trative plots showing how the pres-

sure drop in a pipe may be affected

by several different factors.

Δp

ᐉ

Δp

ᐉ

Δp

ᐉ

Δp

ᐉ

D, , – constant

ρμ

(a)

V, , – constant

ρμ

(b)

D, V, – constant

(c)

D, , V– constant

(d)

F I G U R E 7.2 An illustrative plot of pressure drop

data using dimensionless parameters.

Δp

ᐉ

____

Dimensionless

products are impor-

tant and useful in

the planning,

execution, and

interpretation of

experiments.

As noted in Chapter 1, we will use T to represent the basic dimension of time, although T is also used for temperature in thermodynamic

relationships 1such as the ideal gas law2.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 334

1Recall from Chapter 1 that the notation is used to indicate dimensional equality.2The dimen-

sions of the variables in the pipe flow example are

and 3Note that the pressure drop per unit length has the dimensions of

A quick check of the dimensions of the two groups that appear in Eq. 7.2 shows that they are in

fact dimensionless products; that is,

and

Not only have we reduced the number of variables from five to two, but the new groups are

dimensionless combinations of variables, which means that the results presented in the form of

Fig. 7.2 will be independent of the system of units we choose to use. This type of analysis is called

dimensional analysis, and the basis for its application to a wide variety of problems is found in

the Buckingham pi theorem described in the following section.

rVD

⬟

1FL

⫺4

21LT

⫺1

21L2

1FL

⫺2

⬟ F

D ¢p

⬟

L1F

Ⲑ

1FL

⫺4

21LT

⫺1

⬟ F

Ⲑ

L ⫽ FL

⫺3

.4V ⬟ LT

⫺1

m ⬟ FL

⫺2

T,¢p

⬟ FL

⫺3

, D ⬟ L, r ⬟ FL

⫺4

⬟

7.2 Buckingham Pi Theorem 335

A fundamental question we must answer is how many dimensionless products are required to re-

place the original list of variables? The answer to this question is supplied by the basic theorem

of dimensional analysis that states the following:

If an equation involving k variables is dimensionally homogeneous, it can be reduced

to a relationship among independent dimensionless products, where r is the

minimum number of reference dimensions required to describe the variables.

The dimensionless products are frequently referred to as “pi terms,” and the theorem is called the

Buckingham pi theorem.

Edgar Buckingham used the symbol to represent a dimensionless

product, and this notation is commonly used. Although the pi theorem is a simple one, its proof is

not so simple and we will not include it here. Many entire books have been devoted to the subject

of similitude and dimensional analysis, and a number of these are listed at the end of this chapter

1Refs. 1–152. Students interested in pursuing the subject in more depth 1including the proof of the

pi theorem2can refer to one of these books.

The pi theorem is based on the idea of dimensional homogeneity which was introduced in

Chapter 1. Essentially we assume that for any physically meaningful equation involving k vari-

ables, such as

the dimensions of the variable on the left side of the equal sign must be equal to the dimensions

of any term that stands by itself on the right side of the equal sign. It then follows that we can

rearrange the equation into a set of dimensionless products 1pi terms2so that

where is a function of through

The required number of pi terms is fewer than the number of original variables by r, where

r is determined by the minimum number of reference dimensions required to describe the origi-

nal list of variables. Usually the reference dimensions required to describe the variables will be

the basic dimensions M, L, and T or F, L, and T. However, in some instances perhaps only two

dimensions, such as L and T, are required, or maybe just one, such as L. Also, in a few rare cases

k⫺r

.ß

f1ß

, ß

, . . . , ß

k⫺r

⫽ f1ß

, ß

, . . . , ß

k⫺r

⫽ f1u

, u

, . . . , u

k ⴚ r

7.2 Buckingham Pi Theorem

Although several early investigators, including Lord Rayleigh 11842–19192in the nineteenth century, contributed to the development of

dimensional analysis, Edgar Buckingham’s 11867–19402name is usually associated with the basic theorem. He stimulated interest in the sub-

ject in the United States through his publications during the early part of the twentieth century. See, for example, E. Buckingham, On Physi-

cally Similar Systems: Illustrations of the Use of Dimensional Equations, Phys. Rev., 4 119142, 345–376.

Dimensional analy-

sis is based on the

Buckingham pi

theorem.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 335

the variables may be described by some combination of basic dimensions, such as and L,

and in this case r would be equal to two rather than three. Although the use of the pi theorem

may appear to be a little mysterious and complicated, we will actually develop a simple, system-

atic procedure for developing the pi terms for a given problem.

Ⲑ

336 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

Several methods can be used to form the dimensionless products, or pi terms, that arise in a dimen-

sional analysis. Essentially we are looking for a method that will allow us to systematically form the pi

terms so that we are sure that they are dimensionless and independent, and that we have the right num-

ber. The method we will describe in detail in this section is called the method of repeating variables.

It will be helpful to break the repeating variable method down into a series of distinct steps

that can be followed for any given problem. With a little practice you will be able to readily com-

plete a dimensional analysis for your problem.

Step 1 List all the variables that are involved in the problem. This step is the most difficult

one and it is, of course, vitally important that all pertinent variables be included. Other-

wise the dimensional analysis will not be correct! We are using the term “variable” to

include any quantity, including dimensional and nondimensional constants, which play a

role in the phenomenon under investigation. All such quantities should be included in

the list of “variables” to be considered for the dimensional analysis. The determination

of the variables must be accomplished by the experimenter’s knowledge of the problem

and the physical laws that govern the phenomenon. Typically the variables will include

those that are necessary to describe the geometry of the system 1such as a pipe diame-

ter2, to define any fluid properties 1such as a fluid viscosity2, and to indicate external

effects that influence the system 1such as a driving pressure drop per unit length2. These

general classes of variables are intended as broad categories that should be helpful in

identifying variables. It is likely, however, that there will be variables that do not fit eas-

ily into one of these categories, and each problem needs to be carefully analyzed.

Since we wish to keep the number of variables to a minimum, so that we can mini-

mize the amount of laboratory work, it is important that all variables be independent. For

example, if in a certain problem the cross-sectional area of a pipe is an important variable,

either the area or the pipe diameter could be used, but not both, since they are obviously

not independent. Similarly, if both fluid density, and specific weight, are important

variables, we could list and or and g 1acceleration of gravity2, or and g. However,

it would be incorrect to use all three since that is, and g are not independent.

Note that although g would normally be constant in a given experiment, that fact is irrel-

evant as far as a dimensional analysis is concerned.

Step 2 Express each of the variables in terms of basic dimensions. For the typical fluid me-

chanics problem the basic dimensions will be either M, L, and T or F, L, and T. Dimension-

ally these two sets are related through Newton’s second law so that

For example, Thus, either set can be used. The basic dimensions

for typical variables found in fluid mechanics problems are listed in Table 1.1 in Chapter 1.

Step 3 Determine the required number of pi terms. This can be accomplished by means of the

Buckingham pi theorem, which indicates that the number of pi terms is equal to

where k is the number of variables in the problem 1which is determined from Step 12and

r is the number of reference dimensions required to describe these variables 1which is deter-

mined from Step 22. The reference dimensions usually correspond to the basic dimensions

and can be determined by an inspection of the dimensions of the variables obtained in Step

2. As previously noted, there may be occasions 1usually rare2in which the basic dimen-

sions appear in combinations so that the number of reference dimensions is less than the

number of basic dimensions. This possibility is illustrated in Example 7.2.

Step 4 Select a number of repeating variables, where the number required is equal to the

number of reference dimensions. Essentially what we are doing here is selecting from

the original list of variables several of which can be combined with each of the remaining

k ⫺ r,

r ⬟ ML

⫺3

or r ⬟ FL

⫺4

F ⬟ MLT

⫺2

.1F ⫽ ma2

r, g,g ⫽ rg;

grg,r

g,r,

7.3 Determination of Pi Terms

A dimensional

analysis can be

performed using a

series of distinct

steps.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 336