Munson B.R. Fundamentals of Fluid Mechanics

Подождите немного. Документ загружается.

where s is measured along the streamline. If we write the velocity, and length, s, in dimension-

less form, that is,

where V and represent some characteristic velocity and length, respectively, then

and

⫽

ds*

⫽

ds*

⫽

s* ⫽

7.6 Common Dimensionless Groups in Fluid Mechanics 347

F I G U R E 7.3 The force of gravity acting on a

fluid particle moving along a streamline.

Streamline

Special names

along with physical

interpretations are

given to the most

common dimen-

sionless groups.

TABLE 7.1

Some Common Variables and Dimensionless Groups in Fluid Mechanics

Variables: Acceleration of gravity, g; Bulk modulus, Characteristic length, Density, ; Frequency of

oscillating flow, ; Pressure, p (or p); Speed of sound, c; Surface tension, ; Velocity, V; Viscosity,

Dimensionless Interpretation (Index of Types of

Groups Name Force Ratio Indicated) Applications

Reynolds number, Re Generally of importance in

all types of fluid dynamics

problems

Froude number, Fr Flow with a free surface

Euler number, Eu Problems in which pressure,

or pressure differences, are

of interest

Cauchy Flows in which the

compressibility of the fluid

is important

Mach Ma Flows in which the

compressibility of the fluid

is important

Strouhal number, St Unsteady flow with a

characteristic frequency of

oscillation

Weber number, We Problems in which surface

tension is important

The Cauchy number and the Mach number are related and either can be used as an index of the relative effects of inertia and compressibil-

ity. See accompanying discussion.

number,

ms¢v

rᐍ;E

;

1g/

rV/

inertia force

surface tension force

inertia 1local2 force

inertia 1convective2 force

inertia force

compressibility force

inertia force

compressibility force

pressure force

inertia force

gravitational force

inertia force

viscous force

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 347

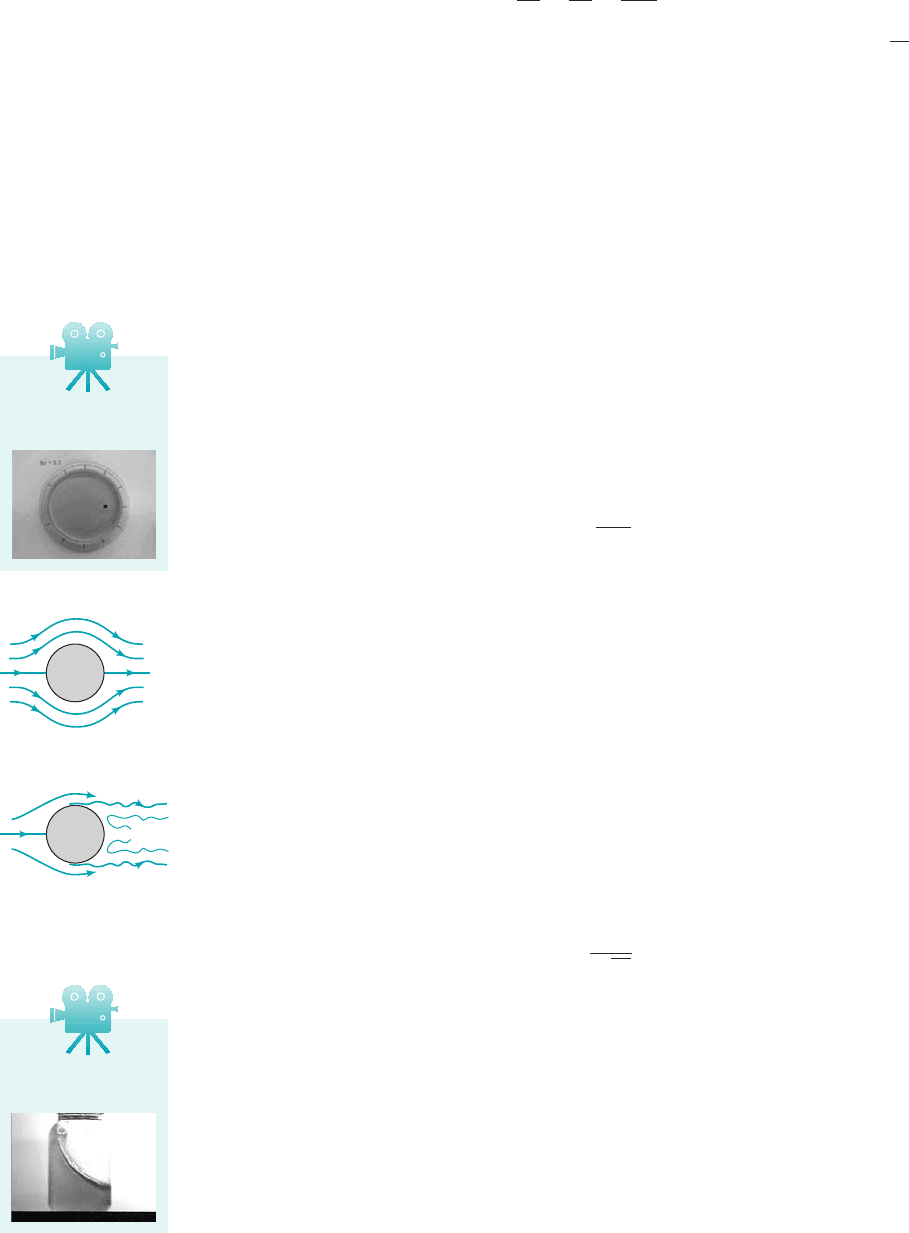
The magnitude of the weight of the particle, so the ratio of the inertia to the grav-

itational force is

Thus, the force ratio is proportional to and the square root of this ratio, is called

the Froude number. We see that a physical interpretation of the Froude number is that it is a mea-

sure of, or an index of, the relative importance of inertial forces acting on fluid particles to the weight

of the particle. Note that the Froude number is not really equal to this force ratio, but is simply

some type of average measure of the influence of these two forces. In a problem in which gravity

1or weight2is not important, the Froude number would not appear as an important pi term. A sim-

ilar interpretation in terms of indices of force ratios can be given to the other dimensionless groups,

as indicated in Table 7.1, and a further discussion of the basis for this type of interpretation is given

in the last section in this chapter. Some additional details about these important dimensionless groups

are given below, and the types of application or problem in which they arise are briefly noted in the

last column of Table 7.1.

Reynolds Number. The Reynolds number is undoubtedly the most famous dimension-

less parameter in fluid mechanics. It is named in honor of Osborne Reynolds 11842–19122,a

British engineer who first demonstrated that this combination of variables could be used as a cri-

terion to distinguish between laminar and turbulent flow. In most fluid flow problems there will

be a characteristic length, and a velocity, V, as well as the fluid properties of density, and

viscosity, which are relevant variables in the problem. Thus, with these variables the Reynolds

number

arises naturally from the dimensional analysis. The Reynolds number is a measure of the ratio of

the inertia force on an element of fluid to the viscous force on an element. When these two types

of forces are important in a given problem, the Reynolds number will play an important role. How-

ever, if the Reynolds number is very small this is an indication that the viscous forces

are dominant in the problem, and it may be possible to neglect the inertial effects; that is, the den-

sity of the fluid will not be an important variable. Flows at very small Reynolds numbers are com-

monly referred to as “creeping flows” as discussed in Section 6.10. Conversely, for large Reynolds

number flows, viscous effects are small relative to inertial effects and for these cases it may be

possible to neglect the effect of viscosity and consider the problem as one involving a “nonvis-

cous” fluid. This type of problem is considered in detail in Sections 6.4 through 6.7. An example

of the importance of the Reynolds number in determining the flow physics is shown in the figure

in the margin for flow past a circular cylinder at two different Re values. This flow is discussed

further in Chapter 9.

Froude Number. The Froude number

is distinguished from the other dimensionless groups in Table 7.1 in that it contains the acceler-

ation of gravity, g. The acceleration of gravity becomes an important variable in a fluid dynam-

ics problem in which the fluid weight is an important force. As discussed, the Froude number is

a measure of the ratio of the inertia force on an element of fluid to the weight of the element. It

will generally be important in problems involving flows with free surfaces since gravity princi-

pally affects this type of flow. Typical problems would include the study of the flow of water

around ships 1with the resulting wave action2or flow through rivers or open conduits. The Froude

number is named in honor of William Froude 11810–18792, a British civil engineer, mathemati-

cian, and naval architect who pioneered the use of towing tanks for the study of ship design. It

is to be noted that the Froude number is also commonly defined as the square of the Froude num-

ber listed in Table 7.1.

Fr 

1g/

1Re  12,

Re 

rV/

r,/,



1g/,V



g/,F





ds*

, is F

 gm,

348 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

V7.3 Reynolds

number

V7.4 Froude

number

No separation

Laminar boundary layer,

wide turbulent wake

≈ 0.2

Re ≈ 20,000

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 348

Euler Number. The Euler number

can be interpreted as a measure of the ratio of pressure forces to inertial forces, where p is some

characteristic pressure in the flow field. Very often the Euler number is written in terms of a pres-

sure difference, so that Also, this combination expressed as is called

the pressure coefficient. Some form of the Euler number would normally be used in problems in

which pressure or the pressure difference between two points is an important variable. The Euler

number is named in honor of Leonhard Euler 11707–17832, a famous Swiss mathematician who

pioneered work on the relationship between pressure and flow. For problems in which cavitation

is of concern, the dimensionless group is commonly used, where is the vapor

pressure and is some reference pressure. Although this dimensionless group has the same form

as the Euler number, it is generally referred to as the cavitation number.

Cauchy Number and Mach Number. The Cauchy number

and the Mach number

are important dimensionless groups in problems in which fluid compressibility is a significant fac-

tor. Since the speed of sound, c, in a fluid is equal to 1see Section 1.7.32, it follows

that

and the square of the Mach number

is equal to the Cauchy number. Thus, either number 1but not both2may be used in problems in

which fluid compressibility is important. Both numbers can be interpreted as representing an in-

dex of the ratio of inertial forces to compressibility forces. When the Mach number is relatively

small 1say, less than 0.32, the inertial forces induced by the fluid motion are not sufficiently large

to cause a significant change in the fluid density, and in this case the compressibility of the fluid

can be neglected. The Mach number is the more commonly used parameter in compressible flow

problems, particularly in the fields of gas dynamics and aerodynamics. The Cauchy number is

named in honor of Augustin Louis de Cauchy 11789–18572, a French engineer, mathematician, and

hydrodynamicist. The Mach number is named in honor of Ernst Mach 11838–19162, an Austrian

physicist and philosopher.

Strouhal Number. The Strouhal number

is a dimensionless parameter that is likely to be important in unsteady, oscillating flow problems

in which the frequency of the oscillation is It represents a measure of the ratio of inertial forces

due to the unsteadiness of the flow 1local acceleration2to the inertial forces due to changes in ve-

locity from point to point in the flow field 1convective acceleration2. This type of unsteady flow

may develop when a fluid flows past a solid body 1such as a wire or cable2placed in the moving

stream. For example, in a certain Reynolds number range, a periodic flow will develop downstream

from a cylinder placed in a moving fluid due to a regular pattern of vortices that are shed from the

body. 1See the photograph at the beginning of this chapter and Fig. 9.21.2This system of vortices,

called a Kármán vortex trail [named after Theodor von Kármán 11881–19632, a famous fluid

St ⫽

⫽

⫽ Ca

Ma ⫽ V

c ⫽ 1E

Ⲑ

Ma ⫽

Ca ⫽

⫺ p

Ⲑ

¢p

Ⲑ

Eu ⫽ ¢p

Ⲑ

.¢p,

Eu ⫽

7.6 Common Dimensionless Groups in Fluid Mechanics 349

The Mach number

is a commonly used

dimensionless pa-

rameter in com-

pressible flow

problems.

V7.5 Strouhal

number

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 349

mechanician], creates an oscillating flow at a discrete frequency, such that the Strouhal number

can be closely correlated with the Reynolds number. When the frequency is in the audible range,

a sound can be heard and the bodies appear to “sing.” In fact, the Strouhal number is named in

honor of Vincenz Strouhal 11850–19222, who used this parameter in his study of “singing wires.”

The most dramatic evidence of this phenomenon occurred in 1940 with the collapse of the Tacoma

Narrows bridge. The shedding frequency of the vortices coincided with the natural frequency of

the bridge, thereby setting up a resonant condition that eventually led to the collapse of the bridge.

There are, of course, other types of oscillating flows. For example, blood flow in arteries is pe-

riodic and can be analyzed by breaking up the periodic motion into a series of harmonic components

1Fourier series analysis2, with each component having a frequency that is a multiple of the fundamen-

tal frequency, 1the pulse rate2. Rather than use the Strouhal number in this type of problem, a di-

mensionless group formed by the product of St and Re is used; that is

The square root of this dimensionless group is often referred to as the frequency parameter.

Weber Number. The Weber number

may be important in problems in which there is an interface between two fluids. In this situation

the surface tension may play an important role in the phenomenon of interest. The Weber number

can be thought of as an index of the inertial force to the surface tension force acting on a fluid el-

ement. Common examples of problems in which this parameter may be important include the flow

of thin films of liquid, or the formation of droplets or bubbles. Clearly, not all problems involving

flows with an interface will require the inclusion of surface tension. The flow of water in a river

is not affected significantly by surface tension, since inertial and gravitational effects are dominant

However, as discussed in a later section, for river models 1which may have small depths2

caution is required so that surface tension does not become important in the model, whereas it is

not important in the actual river. The Weber number is named after Moritz Weber 11871–19512,a

German professor of naval mechanics who was instrumental in formalizing the general use of com-

mon dimensionless groups as a basis for similitude studies.

1We  12.

We 

St  Re 

rv/

350 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

V7.6 Weber number

Fluids in the News

Slip at the micro scale A goal in chemical and biological analy-

ses is to miniaturize the experiment, which has many advantages

including reduction in sample size. In recent years, there has been

significant work on integrating these tests on a single microchip to

form the “lab-on-a-chip” system. These devices are on the mil-

limeter scale with complex passages for fluid flow on the micron

scale (or smaller). While there are advantages to miniaturization,

care must be taken in moving to smaller and smaller flow regimes,

as you will eventually bump into the continuum assumption. To

characterize this situation, a dimensionless number termed the

Knudsen number, , is commonly employed. Here is

the mean free path and is the characteristic length of the system.

If Kn is smaller than 0.01, then the flow can be described by

the Navier–Stokes equations with no-slip at the walls. For

, the same equations can be used, but there can

be “slip” between the fluid and the wall so the boundary conditions

need to be adjusted. For , the continuum assumption

breaks down and the Navier–Stokes equations are no longer valid.

Kn 7 10

0.01 6 Kn 6 0.3

lKn  l



One of the most important uses of dimensional analysis is as an aid in the efficient handling, in-

terpretation, and correlation of experimental data. Since the field of fluid mechanics relies heav-

ily on empirical data, it is not surprising that dimensional analysis is such an important tool in

this field. As noted previously, a dimensional analysis cannot provide a complete answer to any

given problem, since the analysis only provides the dimensionless groups describing the phe-

nomenon, and not the specific relationship among the groups. To determine this relationship,

suitable experimental data must be obtained. The degree of difficulty involved in this process

depends on the number of pi terms, and the nature of the experiments 1How hard is it to obtain

7.7 Correlation of Experimental Data

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 350

the measurements?2. The simplest problems are obviously those involving the fewest pi terms,

and the following sections indicate how the complexity of the analysis increases with the in-

creasing number of pi terms.

7.7.1 Problems with One Pi Term

Application of the pi theorem indicates that if the number of variables minus the number of refer-

ence dimensions is equal to unity, then only one pi term is required to describe the phenomenon.

The functional relationship that must exist for one pi term is

where C is a constant. This is one situation in which a dimensional analysis reveals the specific

form of the relationship and, as is illustrated by the following example, shows how the individual

variables are related. The value of the constant, however, must still be determined by experiment.

 C

7.7 Correlation of Experimental Data 351

GIVEN As shown in Fig. E7.3, assume that the drag, d, act-

ing on a spherical particle that falls very slowly through a vis-

cous fluid, is a function of the particle diameter, D, the particle

velocity, V, and the fluid viscosity, ␮.

FIND Determine, with the aid of dimensional analysis, how

the drag depends on the particle velocity.

OLUTION

F I G U R E E7.3

Flow with Only One Pi Term

COMMENTS Actually, the dimensional analysis reveals that

the drag not only varies directly with the velocity, but it also

varies directly with the particle diameter and the fluid viscosity.

We could not, however, predict the value of the drag, since the

constant, C, is unknown. An experiment would have to be per-

formed in which the drag and the corresponding velocity are mea-

sured for a given particle and fluid. Although in principle we

would only have to run a single test, we would certainly want to

repeat it several times to obtain a reliable value for C. It should be

emphasized that once the value of C is determined it is not neces-

sary to run similar tests by using different spherical particles and

fluids; that is, C is a universal constant so long as the drag is a

function only of particle diameter, velocity, and fluid viscosity.

An approximate solution to this problem can also be obtained

theoretically, from which it is found that C  3␲ so that

This equation is commonly called Stokes law and is used in the

study of the settling of particles. Our experiments would reveal that

this result is only valid for small Reynolds numbers (␳VD/␮  1).

This follows, since in the original list of variables, we have

d  3␲␮VD



= f(

, )

XAMPLE 7.3

From the information given, it follows that

d  f(D, V, ␮)

and the dimensions of the variables are

We see that there are four variables and three reference dimen-

sions (F, L, and T) required to describe the variables. Thus, ac-

cording to the pi theorem, one pi term is required. This pi term

can be easily formed by inspection and can be expressed as

Because there is only one pi term, it follows that

where C is a constant. Thus,

Thus, for a given particle and fluid, the drag varies directly with

the velocity so that

(Ans)

d r V

d  C␮VD

␮VD

 C



␮VD

␮ ⬟ FL

2

V ⬟ LT

1

D ⬟ L

d ⬟ F

V7.7 Stokes flow

If only one pi term

is involved in a

problem, it must be

equal to a constant.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 351

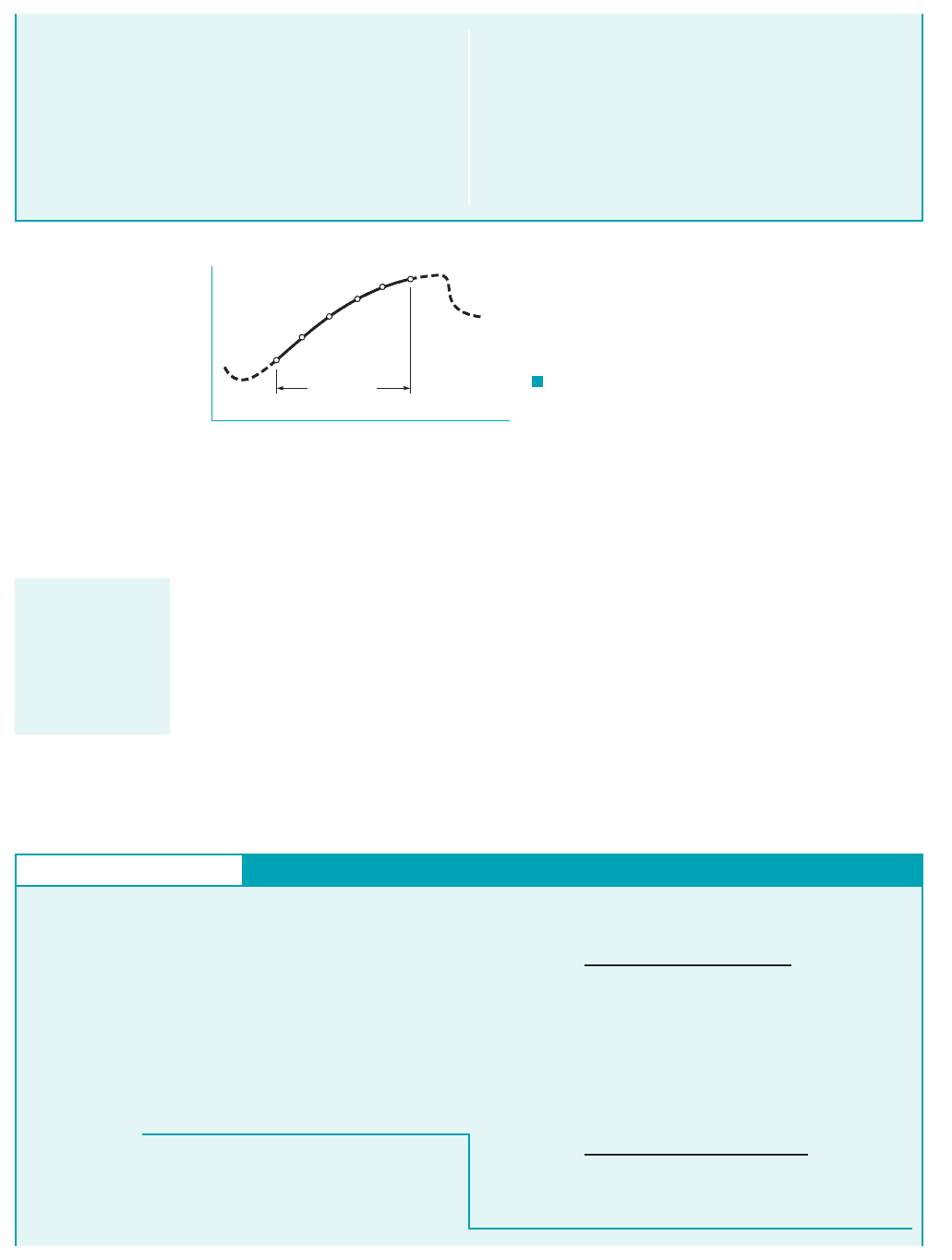
7.7.2 Problems with Two or More Pi Terms

If a given phenomenon can be described with two pi terms such that

the functional relationship among the variables can then be determined by varying and mea-

suring the corresponding values of For this case the results can be conveniently presented in

graphical form by plotting versus as is illustrated in Fig. 7.4. It should be emphasized that

the curve shown in Fig. 7.4 would be a “universal” one for the particular phenomenon studied.

This means that if the variables and the resulting dimensional analysis are correct, then there is

only a single relationship between and as illustrated in Fig. 7.4. However, since this is an

empirical relationship, we can only say that it is valid over the range of covered by the exper-

iments. It would be unwise to extrapolate beyond this range, since as illustrated with the dashed

lines in the figure, the nature of the phenomenon could dramatically change as the range of is

extended. In addition to presenting the data graphically, it may be possible 1and desirable2to ob-

tain an empirical equation relating and by using a standard curve-fitting technique.ß

,ß

 f1ß

352 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

neglected inertial effects (fluid density is not included as a vari-

able). The inclusion of an additional variable would lead to

another pi term so that there would be two pi terms rather than

one.

Consider a free body diagram of a sphere in Stokes flow; there

would be a buoyant force in the same direction as the drag in Fig.

E7.3, as well as a weight force in the opposite direction. As shown

above, the drag force is proportional to the product of the diame-

ter and fall velocity, . The weight and buoyant force ared r VD

proportional to the diameter cubed, W and . Given equi-

librium conditions, the force balance can be written as

Based on the scaling laws for these terms, it follows that

Hence, the fall velocity will be proportional to the square of the di-

ameter, . Therefore, for two spheres, one having twice the

diameter of the other, and falling through the same fluid, the sphere

with the larger diameter will fall four times faster (see Video V7.7).

V r D

VD r D

d  W  F

r D

For problems in-

volving only two pi

terms, results of an

experiment can be

conveniently pre-

sented in a simple

graph.

Valid range

k – r = 2

F I G U R E 7.4 The graphical presen-

tation of data for problems involving two pi terms,

with an illustration of the potential danger of extrap-

olation of data.

GIVEN The relationship between the pressure drop per unit

length along a smooth-walled, horizontal pipe and the variables

that affect the pressure drop is to be determined experimentally. In

the laboratory the pressure drop was measured over a 5-ft length of

smooth-walled pipe having an inside diameter of 0.496 in. The

fluid used was water at

Tests were run in which the velocity was varied

and the corresponding pressure drop measured. The results of

these tests are shown below:

1.94 slugs



60 °F 1m  2.34  10

5



, r 

OLUTION

Dimensionless Correlation of Experimental Data

XAMPLE 7.4

The first step is to perform a dimensional analysis during the

planning stage before the experiments are actually run. As was

discussed in Section 7.3, we will assume that the pressure drop

per unit length, is a function of the pipe diameter, D, fluid¢p

Pressure

Velocity drop for 5-ft

(ft/s) length (lb/ft

)

1.17 6.26

1.95 15.6

2.91 30.9

5.84 106

11.13 329

16.92 681

23.34 1200

28.73 1730

FIND Make use of these data to obtain a general relationship

between the pressure drop per unit length and the other variables.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 352

As the number of required pi terms increases, it becomes more difficult to display the results

in a convenient graphical form and to determine a specific empirical equation that describes the

phenomenon. For problems involving three pi terms

it is still possible to show data correlations on simple graphs by plotting families of curves as il-

lustrated in Fig. 7.5. This is an informative and useful way of representing the data in a general

 f1ß

, ß

7.7 Correlation of Experimental Data 353

density, fluid viscosity, and the velocity, V. Thus,

and application of the pi theorem yields two pi terms

Hence,

To determine the form of the relationship, we need to vary the

Reynolds number, and to measure the correspond-

ing values of The Reynolds number could be varied

by changing any one of the variables, V, D, or or any combi-

nation of them. However, the simplest way to do this is to vary the

velocity, since this will allow us to use the same fluid and pipe.

Based on the data given, values for the two pi terms can be com-

puted, with the result:

0.0195

0.0175

0.0155

0.0132

0.0113

0.0101

0.00939

0.00893

These are dimensionless groups so that their values are independent

of the system of units used so long as a consistent system is used.

For example, if the velocity is in ft兾s, then the diameter should be in

feet, not inches or meters. Note that since the Reynolds numbers

are all greater than 2100, the flow in the pipe is turbulent 1see Sec-

tion 8.1.12.

A plot of these two pi terms can now be made with the results

shown in Fig. E7.4a. The correlation appears to be quite good, and

if it was not, this would suggest that either we had large experimen-

tal measurement errors or that we had perhaps omitted an important

variable. The curve shown in Fig. E7.4a represents the general rela-

tionship between the pressure drop and the other factors in the range

of Reynolds numbers between and Thus,

for this range of Reynolds numbers it is not necessary to repeat the

tests for other pipe sizes or other fluids provided the assumed inde-

pendent variables are the only important ones.

Since the relationship between and is nonlinear, it is not

immediately obvious what form of empirical equation might be

used to describe the relationship. If, however, the same data are

1D, r, m, V2

9.85  10

.4.01  10

9.85  10

8.00  10

5.80  10

3.81  10

2.00  10

9.97  10

6.68  10

4.01  10

RVD



MD¢p

ᐍ



m,r,

D ¢p



Re  rVD



D ¢p

 f a

rVD



D ¢p

and ß



rVD

¢p

 f 1D, r, m, V2

m,r,

plotted on logarithmic graph paper, as is shown in Fig. E7.4b, the

data form a straight line, suggesting that a suitable equation is of

the form where A and n are empirical constants to be

determined from the data by using a suitable curve-fitting tech-

nique, such as a nonlinear regression program. For the data given

in this example, a good fit of the data is obtained with the equation

(Ans)

COMMENT

In 1911, H. Blasius 11883–19702, a German

fluid mechanician, established a similar empirical equation that is

used widely for predicting the pressure drop in smooth pipes in

the range 1Ref. 162. This equation can be

expressed in the form

The so-called Blasius formula is based on numerous experimen-

tal results of the type used in this example. Flow in pipes is dis-

cussed in more detail in the next chapter, where it is shown how

pipe roughness 1which introduces another variable2may affect the

results given in this example 1which is for smooth-walled pipes2.

D ¢p

 0.1582 a

rVD

1



4  10

6 Re 6 10

 0.150 ß

0.25

 Aß

For problems in-

volving more than

two or three pi

terms, it is often

necessary to use

a model to predict

specific character-

istics.

0.022

0.020

0.018

0.016

0.014

0.012

0.010

0.008

0 20,000 40,000 60,000 80,000 100,000

(

D Δp

ᐉ

______

–2

4 × 10

–3

2 4 6 8 10

D Δp

ᐉ

______

(b)

_____

Re =

_____

Re =

F I G U R E E7.4

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 353

way. It may also be possible to determine a suitable empirical equation relating the three pi terms.

However, as the number of pi terms continues to increase, corresponding to an increase in the gen-

eral complexity of the problem of interest, both the graphical presentation and the determination

of a suitable empirical equation become intractable. For these more complicated problems, it is of-

ten more feasible to use models to predict specific characteristics of the system rather than to try

to develop general correlations.

354 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

= C

(constant)

= C

k – r = 3

F I G U R E 7.5 The graphi-

cal presentation of data for problems

involving three pi terms.

Models are widely used in fluid mechanics. Major engineering projects involving structures, air-

craft, ships, rivers, harbors, dams, air and water pollution, and so on, frequently involve the use of

models. Although the term “model” is used in many different contexts, the “engineering model”

generally conforms to the following definition. A model is a representation of a physical system

that may be used to predict the behavior of the system in some desired respect. The physical sys-

tem for which the predictions are to be made is called the prototype. Although mathematical or

computer models may also conform to this definition, our interest will be in physical models, that

is, models that resemble the prototype but are generally of a different size, may involve different

fluids, and often operate under different conditions 1pressures, velocities, etc.2. As shown by the

figure in the margin, usually a model is smaller than the prototype. Therefore, it is more easily

handled in the laboratory and less expensive to construct and operate than a large prototype (it

should be noted that variables or pi terms without a subscript will refer to the prototype, whereas

the subscript m will be used to designate the model variables or pi terms). Occasionally, if the pro-

totype is very small, it may be advantageous to have a model that is larger than the prototype so

that it can be more easily studied. For example, large models have been used to study the motion

of red blood cells, which are approximately in diameter. With the successful development of

a valid model, it is possible to predict the behavior of the prototype under a certain set of condi-

tions. We may also wish to examine a priori the effect of possible design changes that are pro-

posed for a hydraulic structure or fluid-flow system. There is, of course, an inherent danger in the

use of models in that predictions can be made that are in error and the error not detected until the

prototype is found not to perform as predicted. It is, therefore, imperative that the model be prop-

erly designed and tested and that the results be interpreted correctly. In the following sections we

will develop the procedures for designing models so that the model and prototype will behave in

a similar fashion.

7.8.1 Theory of Models

The theory of models can be readily developed by using the principles of dimensional analysis. It

has been shown that any given problem can be described in terms of a set of pi terms as

(7.7)

In formulating this relationship, only a knowledge of the general nature of the physical phenome-

non, and the variables involved, is required. Specific values for variables 1size of components, fluid

properties, and so on2are not needed to perform the dimensional analysis. Thus, Eq. 7.7 applies

⫽ f1ß

, ß

, . . . , ß

8 mm

7.8 Modeling and Similitude

V7.8 Model

airplane

Prototype

ᐉ

Model

ᐉ

JWCL068_ch07_332-382.qxd 9/25/08 6:56 PM Page 354

to any system that is governed by the same variables. If Eq. 7.7 describes the behavior of a par-

ticular prototype, a similar relationship can be written for a model of this prototype; that is,

(7.8)

where the form of the function will be the same as long as the same phenomenon is involved in

both the prototype and the model. Variables, or pi terms, without a subscript will refer to the pro-

totype, whereas the subscript m will be used to designate the model variables or pi terms.

The pi terms can be developed so that contains the variable that is to be predicted from

observations made on the model. Therefore, if the model is designed and operated under the fol-

lowing conditions,

(7.9)

then with the presumption that the form of is the same for model and prototype, it follows that

(7.10)

Equation 7.10 is the desired prediction equation and indicates that the measured value of ob-

tained with the model will be equal to the corresponding for the prototype as long as the other

pi terms are equal. The conditions specified by Eqs. 7.9 provide the model design conditions, also

called similarity requirements or modeling laws.

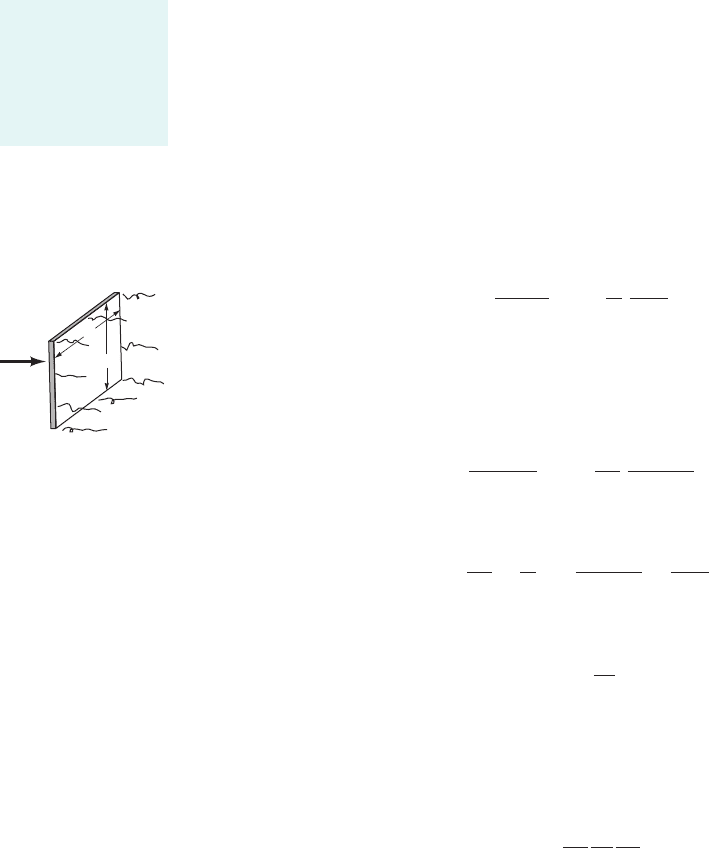
As an example of the procedure, consider the problem of determining the drag, on a thin

rectangular plate placed normal to a fluid with velocity, V, as shown by the figure

in the margin. The dimensional analysis of this problem was performed in Example 7.1, where it

was assumed that

Application of the pi theorem yielded

(7.11)

We are now concerned with designing a model that could be used to predict the drag on a certain

prototype 1which presumably has a different size than the model2. Since the relationship expressed

by Eq. 7.11 applies to both prototype and model, Eq. 7.11 is assumed to govern the prototype, with

a similar relationship

(7.12)

for the model. The model design conditions, or similarity requirements, are therefore

The size of the model is obtained from the first requirement which indicates that

(7.13)

We are free to establish the height ratio but then the model plate width, is fixed in ac-

cordance with Eq. 7.13.

The second similarity requirement indicates that the model and prototype must be operated at

the same Reynolds number. Thus, the required velocity for the model is obtained from the relationship

(7.14)V







rVw

 f a

rVw

d  f1w, h, m, r, V2

1w  h in size2

 ß

 f1ß

, ß

, . . . , ß

7.8 Modeling and Similitude 355

The similarity re-

quirements for a

model can be read-

ily obtained with

the aid of dimen-

sional analysis.

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 355

Note that this model design requires not only geometric scaling, as specified by Eq. 7.13, but also

the correct scaling of the velocity in accordance with Eq. 7.14. This result is typical of most model

designs—there is more to the design than simply scaling the geometry!

With the foregoing similarity requirements satisfied, the prediction equation for the drag is

Thus, a measured drag on the model, must be multiplied by the ratio of the square of the plate

widths, the ratio of the fluid densities, and the ratio of the square of the velocities to obtain the

predicted value of the prototype drag,

Generally, as is illustrated in this example, to achieve similarity between model and pro-

totype behavior, all the corresponding pi terms must be equated between model and prototype.

Usually, one or more of these pi terms will involve ratios of important lengths 1such as in

the foregoing example2; that is, they are purely geometrical. Thus, when we equate the pi terms

involving length ratios, we are requiring that there be complete geometric similarity between

the model and prototype. This means that the model must be a scaled version of the prototype.

Geometric scaling may extend to the finest features of the system, such as surface roughness,

or small protuberances on a structure, since these kinds of geometric features may significantly

influence the flow. Any deviation from complete geometric similarity for a model must be

carefully considered. Sometimes complete geometric scaling may be difficult to achieve, par-

ticularly when dealing with surface roughness, since roughness is difficult to characterize and

control.

Another group of typical pi terms 1such as the Reynolds number in the foregoing exam-

ple2involves force ratios as noted in Table 7.1. The equality of these pi terms requires the ratio

of like forces in model and prototype to be the same. Thus, for flows in which the Reynolds

numbers are equal, the ratio of viscous forces in model and prototype is equal to the ratio of in-

ertia forces. If other pi terms are involved, such as the Froude number or Weber number, a sim-

ilar conclusion can be drawn; that is, the equality of these pi terms requires the ratio of like

forces in model and prototype to be the same. Thus, when these types of pi terms are equal in

model and prototype, we have dynamic similarity between model and prototype. It follows that

with both geometric and dynamic similarity the streamline patterns will be the same and corre-

sponding velocity ratios and acceleration ratios are constant throughout the flow

field. Thus, kinematic similarity exists between model and prototype. To have complete similar-

ity between model and prototype, we must maintain geometric, kinematic, and dynamic similar-

ity between the two systems. This will automatically follow if all the important variables are in-

cluded in the dimensional analysis, and if all the similarity requirements based on the resulting

pi terms are satisfied.

Ⲑ

a21V

Ⲑ

d ⫽ a

b a

⫽

356 Chapter 7 ■ Dimensional Analysis, Similitude, and Modeling

Fluids in the News

Modeling parachutes in a water tunnel The first use of a para-

chute with a free-fall jump from an aircraft occurred in 1914, al-

though parachute jumps from hot air balloons had occurred since the

late 1700s. In more modern times parachutes are commonly used by

the military, and for safety and sport. It is not surprising that there re-

mains interest in the design and characteristics of parachutes, and re-

searchers at the Worcester Polytechnic Institute have been studying

various aspects of the aerodynamics associated with parachutes. An

unusual part of their study is that they are using small-scale para-

chutes tested in a water tunnel. The model parachutes are reduced in

size by a factor of 30 to 60 times. Various types of tests can be per-

formed, ranging from the study of the velocity fields in the wake of

the canopy with a steady free-stream velocity to the study of condi-

tions during rapid deployment of the canopy. According to the re-

searchers, the advantage of using water as the working fluid, rather

than air, is that the velocities and deployment dynamics are slower

than in the atmosphere, thus providing more time to collect detailed

experimental data. (See Problem 7.47.)

Similarity between

a model and a pro-

totype is achieved

by equating pi

terms.

V7.9 Environmental

models

JWCL068_ch07_332-382.qxd 9/23/08 10:46 AM Page 356