Blank B.E., Krantz S.G. Calculus: Single Variable
Подождите немного. Документ загружается.

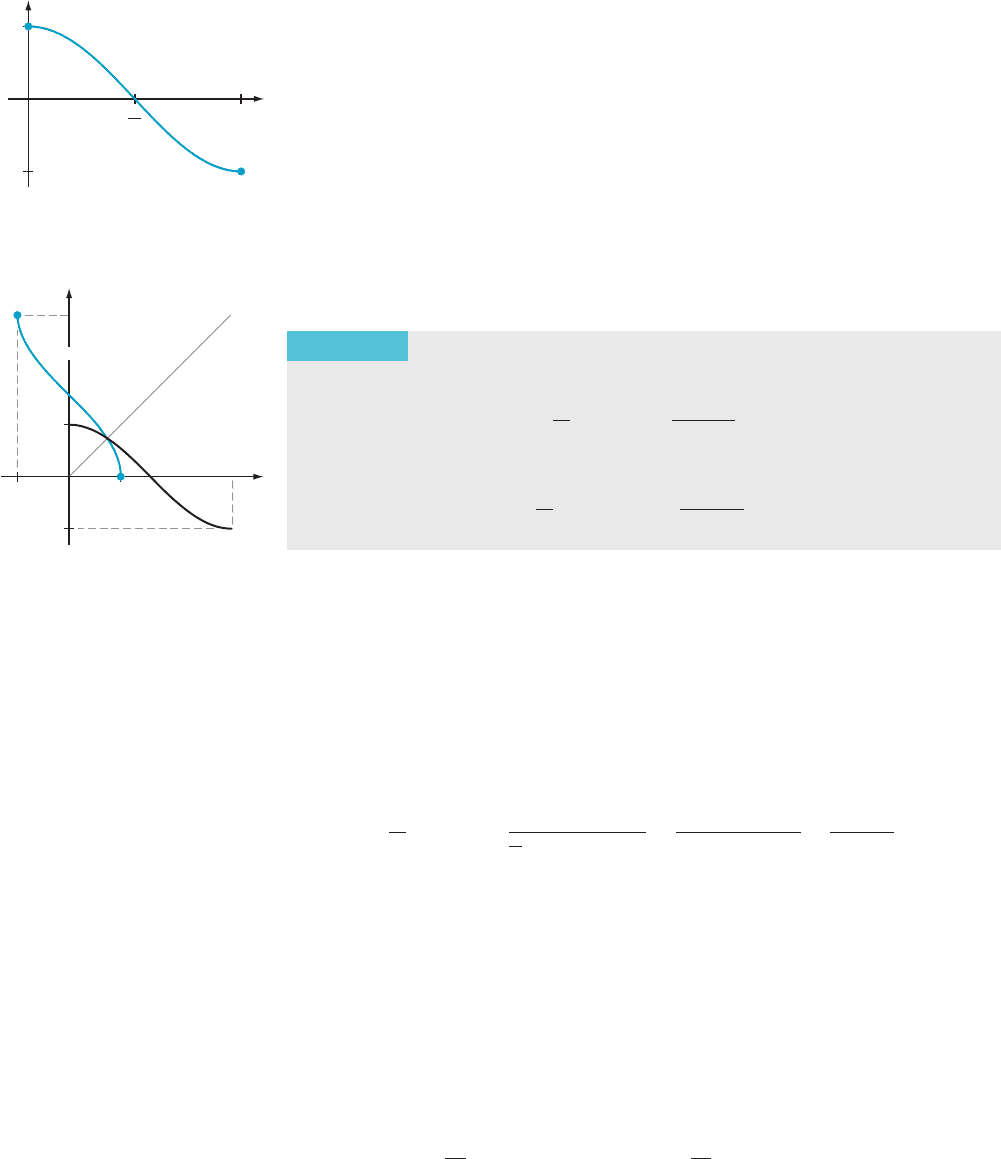
function restricted to the interval [0, π]. Then, as Figure 5 shows, x / Cos(x)isa
one-to-one function. It takes on all the values in the interval [21, 1]. Thus Cos : [0,
π] - [21, 1] is both one-to-one and onto, hence invertible. Its inverse function,
Cos
21
:[21, 1] - [0, π], is decreasing, one-to-one, and onto. The inverse cosine
x / Cos
21
(x) is also called the arccosine and denoted by arccos (x ). We reflect the
graph of y 5 Cos(x ) in the line y 5 x to obtain the graph of y 5 Cos
21
(x). The result
is shown in Figure 6.
⁄ EX
AMPLE 2 Calculate arccosð2
ffiffiffi
2
p
=2Þ, arccos (0), and arccosð
ffiffiffi
3
p
=2Þ.
Solution We
calculate as in Example 1 except that here we are looking for values
in the interval [0, π]. We have arccosð2
ffiffiffi
2
p
=2Þ5 3π=4; arccosð0Þ5 π=2, and arccos
ð
ffiffiffi
3
p
=2Þ5 π=6.
¥
THEOREM 1
The functions t / arcsin ( t) and t / arccos (t) are differentiable
on the open interval (21, 1) and
d
dt
arcsinðtÞ5
1
ffiffiffiffiffiffiffiffiffiffiffiffi
1 2 t
2
p
ð3:10:1Þ
and
d
dt
arccosðtÞ52
1
ffiffiffiffiffiffiffiffiffiffiffiffi
1 2 t
2
p
: ð3:10:2Þ
Proof. For t in
the open interval (21, 1), let s 5 arcsin (t). Then s is in the open
interval (2π/2, π/2) and cos (s) is positive. Using this observation together with the
identity cos
2
(s) 5 1 2 sin
2
(s), we calculate that
cosðsÞ5
ffiffiffiffiffiffiffiffiffiffiffiffiffi
ffiffi
cos
2
ðsÞ
q
5
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 sin
2
ðsÞ
q
5
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2
sin
arcsinðtÞ
2
r
5
ffiffiffiffiffiffiffiffiffiffiffiffi
1 2 t
2
p
:
We may now obtain the formula for the derivative of arcsin (t) by using the
Inverse Function Deri vative Rule that we learned in Section 3.6:
d
dt
arcsinðtÞ5
1
d
ds
sinðsÞj
s5arcsinðtÞ
5
1
cosðsÞj
s5arcsinðtÞ
5
1
ffiffiffiffiffiffiffiffiffiffiffiffi
1 2 t
2
p
:
The derivative of arccos (t) is calculated in the same way. ’
From formula (3.10.1), we see that arcsin
0
(t) . 0. In Chapter 4, we will learn
that this inequality is the analytic characterization of a graphical property that we
have already observed: The inverse sine is an increasing function. Similarly, for-
mula (3.10.2) shows that arccos
0
(t) , 0 for all t A (21, 1). In Chapter 4, we will learn
that this inequality implies that the inverse cosine is a decreasing function, which
we have already observed from its graph.
⁄ EX
AMPLE 3 Calculate
d
dx
arcsinðxÞ
x50
and
d
dx
arccosðxÞ
x51=2
:
1
1
y
x
y Cos(x)
2
m Figure 5
y
x
1
1
1
1
y Cos(x)
y x
y arccos(x)
m Figure 6
3.10 Other Transcendental Functions 255

Solution From (3.10.1) and (3.10.2), we obtain
d
dx
arcsinðxÞ
x50
5
1
ffiffiffiffiffiffiffiffiffiffiffi
12x
2
p
x50
5 1
and
d
dx
arccosðxÞj
x51=2
52
1
ffiffiffiffiffiffiffiffiffiffiffi
12x
2
p
x51=2
52
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 ð1=2 Þ
2
q
52
2
ffiffiffi
3
p
: ¥
In Chain Rule form, the derivative formulas of Theorem 1 become
d
dx
arcsinðuÞ5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 u
2
p
du
dx
ð3:10:3Þ
and
d
dx
arccosðuÞ52
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 u
2
p
du
dx
: ð3:10:4Þ
⁄ EX
AMPLE 4 Calculate the following derivatives:
d
dx
arcsin
1
x
x522=
ffiffi
3
p
and
d
dx
arccosð
ffiffiffi
x
p
Þ
x51=4
:
Solution Using
(3.10.3) with u 5 1/x and du/dx 521/x
2
, we obtain
d
dx
arcsin
1
x
x522=
ffiffi
3
p
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
12ð1=xÞ
2
q
2
1
x
2
x522=
ffiffi
3
p
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 3=4
p
2
1
4=3
52
1
1=2
3
4
52
3
2
:
Using (3.10.4) with u 5
ffiffiffi
x
p
and du=dx 5 ð1=2Þx
21=2
, we have
d
dx
arccosð
ffiffiffi
x
p
Þ
x51=4
52
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
12ð
ffiffiffi
x
p
Þ
2
q
1
2
x
21=2
x51=4
52
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 ð1=2 Þ
2
q
1
2
ffiffiffiffiffiffiffiffi
1=4
p
52
2
ffiffiffi
3
p
: ¥
⁄ EX
AMPLE 5 Let a be
a positive constant. Show that
d
dx
arcsin
x
a
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
a
2
2 x
2
p
: ð3:10:5Þ
Solution Setting u 5 x/a in
formula (3.10.3), we calculate
d
dx
arcsin
x
a
5
d
dx
arcsinðuÞ5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 u
2
p
du
dx
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 ðx=aÞ
2
q
1
a
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
a
2
2 x
2
p
: ¥
256 Chapter 3 The Derivative
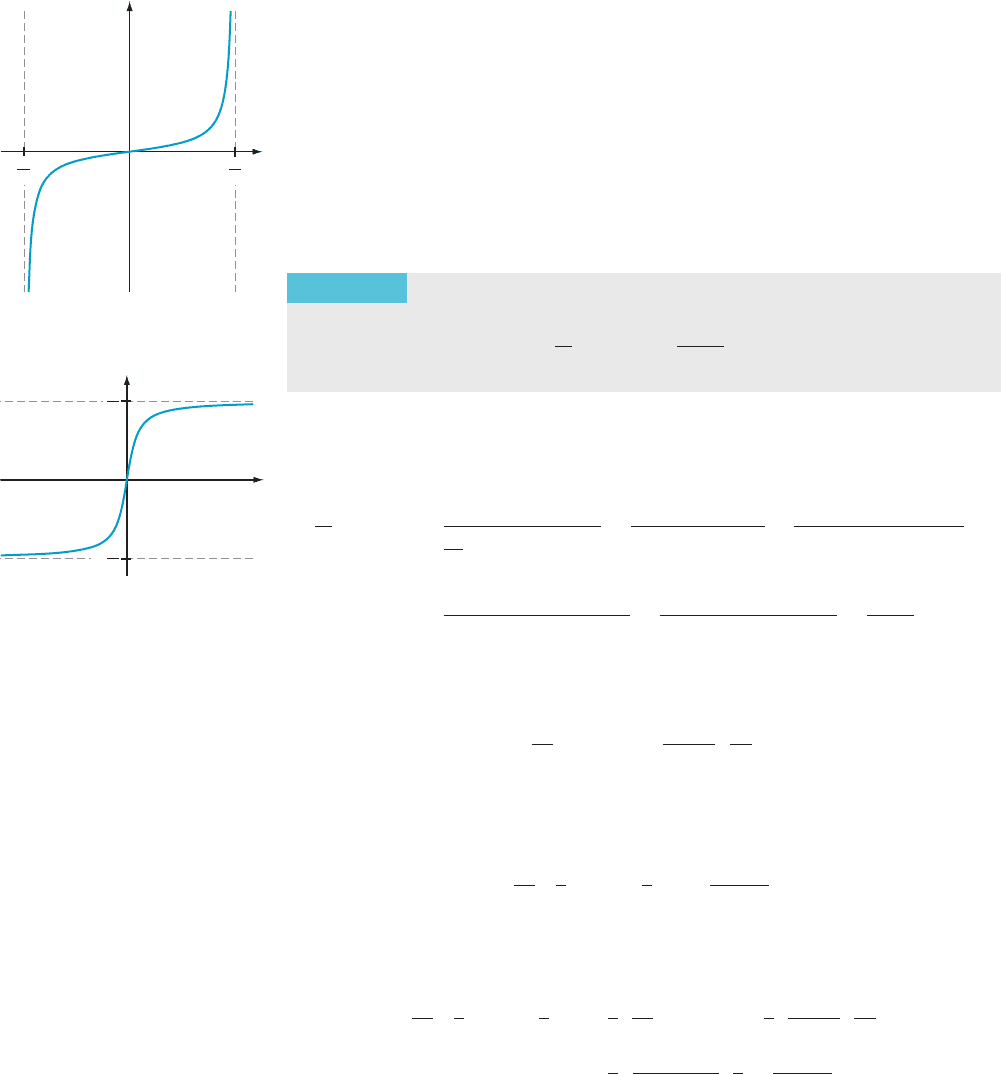
The Inverse Tangent
Function
Define the function x / Tan(x) to be the restriction of x / tan (x) to the interval
(2π/2, π/2). See Figure 7 for the graph of y 5 Tan(x). Because the function Tan is a
one-to-one, onto function with domain (2π/2, π/2) and range (2N,N), the inverse
function Tan
21
exists. We read Tan
21
(x) as “the inverse tangent of x” or “the
arctangent of x.” The notation arctan (x) is often used instead of Tan
21
(x).
The domain of Tan
21
(x)is(2N,N), and its image is (2π/2, π/2). The graph of the
arctangent is shown in Figure 8.
⁄ EX
AMPLE 6 Calculate arctan (1), arctanð
ffiffiffi
3
p
Þ, and arctanð2 1=
ffiffiffi
3
p
Þ:
Solution We
calculate arctan (t) by finding the unique s between 2π/2 and π/2 for
which tan (s) 5 t. Thus arctan ð1Þ5 π=4; arctanð
ffiffiffi
3
p
Þ5 π=3; and arctanð2 1=
ffiffiffi
3
p
Þ5
2 π=6.
¥
THEOREM 2
The function t / arctan (t) is differentiable for every t and
d
dt
arctanðt Þ5
1
1 1 t
2
: ð 3 :10:6Þ
Proof. As
usual, we calculate the derivative of a new inverse funct ion by using the
method of Section 3.6:
d
dt
arctanðtÞ 5
1
d
ds
TanðsÞ
s5arctanðtÞ
5
1
sec
2
ðsÞj
s5arctanðtÞ
5
1
1 1 tan
2
ðsÞj
s5arctanðtÞ
5
1
1 1 ðtanðsÞÞ
2
s5arctanðtÞ
5
1
1 1 ðtanðarctanðtÞÞÞ
2
5
1
1 1 t
2
:
’
The Chain Rule form of the inverse tangent derivative formula is
d
dx
arctanðuÞ5
1
1 1 u
2
du
dx
: ð3:10:7Þ
⁄ EX
AMPLE 7 Let a be a positive constant. Show that
d
dx
1
a
arctan
x
a
5
1
a
2
1 x
2
: ð3:10:8Þ
Solution Using
equation (3.10.7) with u 5 x/a, we calculate
d
dx
1
a
arctan
x
a
5
1
a
d
dx
arctanðuÞ5
1
a
1
1 1 u
2
du
dx
5
1
a
1
1 1 ðx=aÞ
2
1
a
5
1
a
2
1 x
2
: ¥
y
x
y Tan(x)
2
2
m Figure 7
y
x
y Tan
1
(x)
2
2
m Figure 8
3.10 Other Transcendental Functions 257
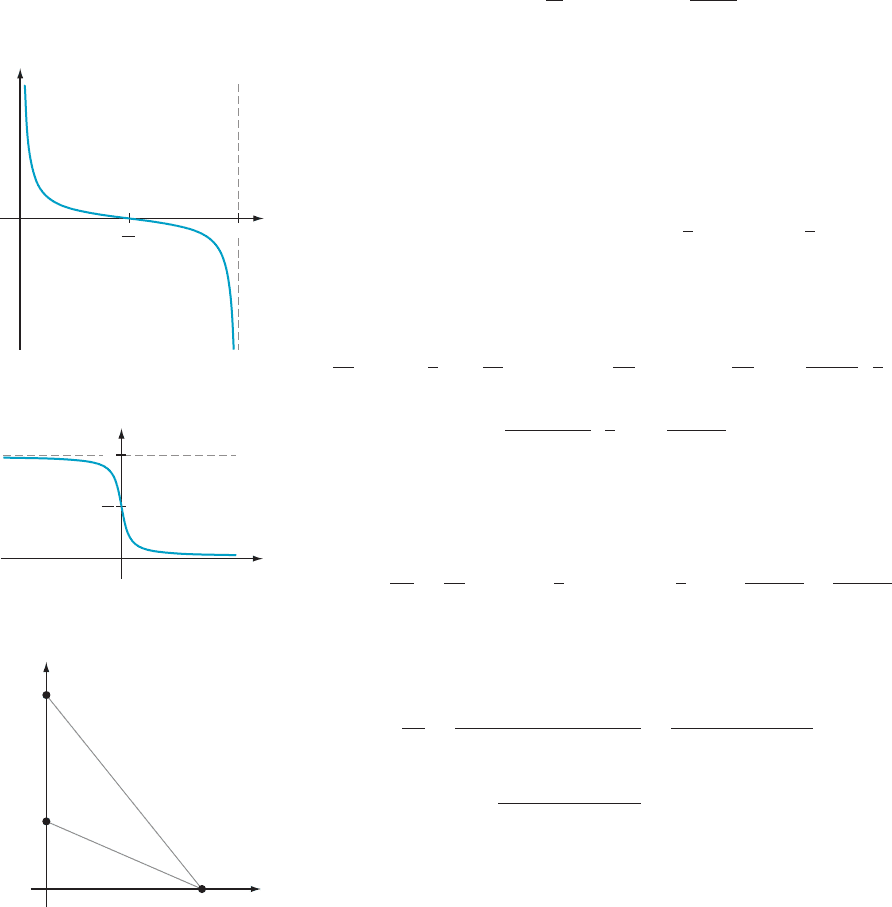
Other Inverse
Trigonometric
Functions
We will say just a few words about the inverse cotangent, the inverse secant, and
the inverse cosecant (because they do not arise frequently). We use x / Cot(x)to
denote the restriction of the cotangent function to the interval (0, π). From the
graph of y 5 Cot(x) in Figure 9, we see that x / Cot(x) is a decreasing function
that takes on all real values. Consequently, the inverse function Cot
21
:(2N,N) -
(0, π) exists. Refer to Figure 10 for its graph. We sometimes write Cot
21
(x)as
arccot (x). A computation similar to that of Theorem 2 yields the derivative of the
inverse cotangent:
d
dt
arccotðtÞ52
1
1 1 t
2
: ð3:10:9Þ
⁄ EX
AMPLE 8 Figure 11 shows two fixed points T 5(0, 8) and B 5 (0, 2) on
the y-axis and a variable point W 5 (x, 0) on the x-axis. The radian measure α of
+TWB is a function of x. Calculate dα/dx.
Solution From
Figure 11, we see that cot (α 1 ψ) 5 x/8, and cot (ψ) 5 x/2. It follows
that
α 5 ðα 1 ψÞ2 ψ 5 arccot
x
8
2 arccot
x
2
:
Both inverse cotangents in this equation have the form arccot (x/a). Letting u 5 x/a,
we use equation (3.10.9) and the Chain Rule to calculate
d
dx
arccot
x
a
5
d
dx
arccotðuÞ5
d
du
arccotðuÞ
du
dx
52
1
1 1 u
2
1
a
52
1
1 1 ðx=aÞ
2
1
a
52
a
a
2
1 x
2
:
ð3:10:10Þ
We may replace a with 2 and 8 in (3.10.10). If we subtract the resulting formulas,
then we obtain
dα
dx
5
d
dx
arccot
x
8
2 arccot
x
2
5
2
2
2
1 x
2
2
8
8
2
1 x
2
:
We complete the calculation by expressing the right side of this last equation in
terms of a common denominator. We have
dα
dx
5
2ð64 1 x
2
Þ2 8ð4 1 x
2
Þ
ð4 1 x
2
Þð64 1 x
2
Þ
5
96 2 6x
2
ð4 1 x
2
Þð64 1 x
2
Þ
526
ðx
2
2 16Þ
ð4 1 x
2
Þð64 1 x
2
Þ
: ¥
ð3:10:11Þ
For
the inverse secant, we define x / Sec(x) to be the restriction of the secant to
the set [0, π/2) , (π/2, π]. From Figure 12, we see that x / Sec(x) is one-to-one
with image consisting of all numbers greater than or equal to 1 and all numbers less
than or equal to 21. The inverse function
x
y
y Cot(x)
x
2
m Figure 9
x
y Cot
1
(x)
2
y
m Figure 10
B
W
T
(0, 8)
(0, 2)
(
x, 0
)
y
x
m Figure 11
258 Chapter
3 The Derivative

Sec
21
: ð2 N; 2 1,½1; NÞ-½0; π=2Þ,ðπ=2; π
is plotted in Figure 13. We sometimes write arcse c (x) for Sec
21
(x) and read
“arcsecant” instead of “inverse secant .” The derivative of the arcsecant is given by
d
dt
arcsecðtÞ5
1
jtj
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
; jtj. 1: ð3:10:12Þ
⁄ EX
AMPLE 9 Derive formula (3.10.12).
Solution The
derivation is quite similar to the ones we have already carried out,
but the absolute value in formula (3.10.12) alerts us to the need for paying careful
attention to the signs of the terms that arise. Applying the Inverse Function
Derivative Rule, we have
d
dt
arcsecðtÞ5
1
d
ds
SecðsÞ
s5arcsecðtÞ
5
1
secðsÞtanðsÞj
s5arcsecðtÞ
5
1
sec
arcsecðtÞ
tan
arcsecðtÞ
5
1
t tan
arcsecðtÞ
:
ð3:10:13Þ
Next, trigonometric identity (1.6.2) with θ 5 arcsec (t) gives us tan
2
(arcsec (t)) 5
sec
2
(arcsec (t))21 5 t
2
2 1, or tanðarcsecðtÞÞ56
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
.Ift . 1, then 0 ,
arcsec(t) , π/2 and tan (arcsec (t)) . 0. Therefore tan
arcsecðtÞ
51
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
for
t . 1. In this case, t 5 jtj and (3.10.13) becomes
d
dt
arcsecðtÞ5
1
t tan
arcsecðtÞ
5
1
jtjð1
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
Þ
5
1
jtj
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
:
If t ,21, then π/2 , arcsec (t) , π and tan (arcsec (t)) , 0. Therefore
tanðarcsecðtÞÞ52
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
for t ,21. In this case, t 52|t| and (3.10.13) becomes
d
dt
arcsecðtÞ5
1
t tan
arcsecðtÞ
5
1
t ð2
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
Þ
5
1
ð2 tÞ
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
5
1
jtj
ffiffiffiffiffiffiffiffiffiffiffiffi
t
2
2 1
p
: ¥
We obtain the final inverse trigonometric function by defining x / Csc(x)t
o
be the restriction of the cosecant to the set [2π/2, 0) , (0, π/2]. The graph is
exhibited in Figure 14. We see that x / Csc(x) is one-to-one and therefore has an
inverse function Csc
21
that maps the image of Csc to the domain of Csc. As x
ranges over the values in the domain of Csc, sin (x) takes on all values in the
interval [21, 1] except for 0. Therefore the cosecant takes on all values greater than
or equal to 1 and all values less than or equal to 21. Thus Csc
21
has domain (2N,
21] , [1,N) and range [2π/2, 0) , (0,π/2]. Its graph is shown in Figure 15. The
derivative of the inverse cosecant is given by
1
(0, 1)
(, 1)
y Sec(x)
x
y
y Sec(x)
1
2
m Figure 12
(1, )
(1, 0)
11
y
Sec
1
(x)
y
y Sec
1
(x)
x
2
m Figure 13
y
x
, 1
y Csc(x)
y Csc(x)
2
, 1
2
m Figure 14
y
x
y Csc
1
(x)
y Csc
1
(x)
11
1,
2
1,
2
m Figure 15
3.10 Other Transcendental Functions 259
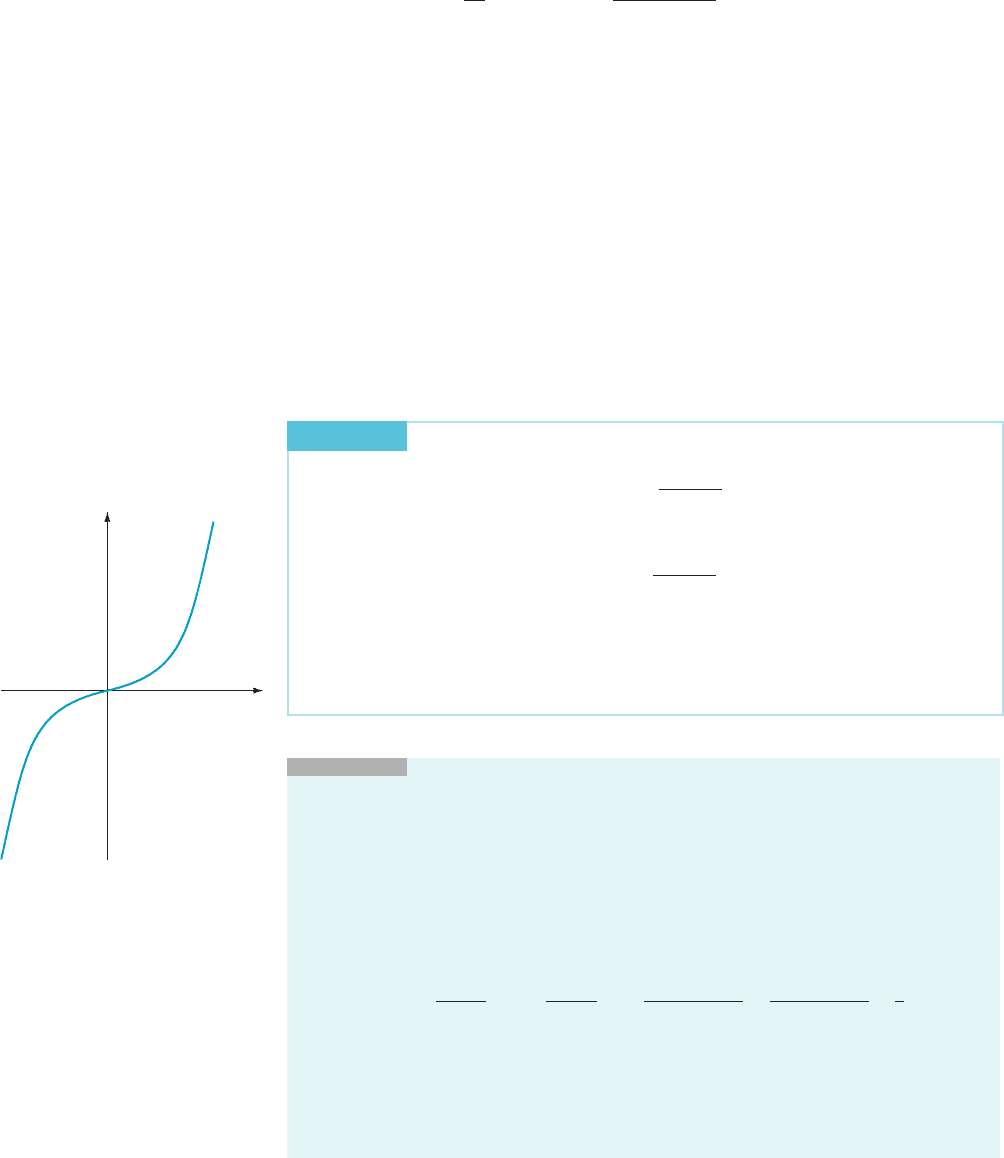
d
dx
Csc
21
ðxÞ52
1
jxj
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
2 1
p
; jxj. 1: ð3:10:14Þ
Notation In this section we have carefully distinguished between the trigonometric functions
and their restrictions to domains on which they are invertible. This has led us to
use the notations Sin
21
, Cos
21
, Tan
21
, and Sec
21
. It is standard practice among
mathematicians and scientists to simply write sin
21
, cos
21
, tan
21
, and sec
21
. When
you see an expression such as tan
21
(x), remember that it refers to the inverse of a
restriction of the tangent. It is especially important not to confuse an inverse tri-
gonometric function (such as Tan
21
(x)) with the reciprocal of the corresponding
trigonometric function (such as (Tan(x))
21
5 1/Tan(x)). The “arc” notation elim-
inates this danger.
The Hyperbolic
Functions
In eng ineering, physics, and other applications, certain special funct ions called the
hyperbolic functions arise frequently. Here we shall define and briefly discuss them.
DEFINITION
The hyperbolic cosine of t is defined to be
cosh ðtÞ5
e
t
1 e
2t
2
for all real numbers t. The hyperbolic sine of t is defined to be
sinh ðtÞ5
e
t
2 e
2t
2
:
The graphs of the hyperbolic sine and cosine are given in Figures 16 and 17.
Notice that the hyperbolic cosine, like the ordinary cosine, is an even function.
That is, cosh (2t) 5 cosh (t). The hyperbolic sine, like the ordinary sine, is an
odd function: sinh (2t) 52sinh (t).
INSIGHT
Why is the term “hyperbolic” used? Recall that, for classical trigonometry
based on the unit circle, the numbers
x 5 cos ðtÞ and y 5 sin ðtÞ
satisfy x
2
1y
2
5 1. That is, the point (cos (t), sin (t)) lies on the unit circle.
By contrast, the numbers
x 5 cosh ðtÞ and y 5 sinh ðtÞ
satisfy
x
2
2 y
2
5
e
t
1e
2t
2
2
2
e
t
2e
2t
2
2
5
e
2t
1 2 1 e
22t
4
2
e
2t
2 2 1 e
22t
4
5
4
4
5 1:
The hyperbolic cosine and hyperbolic sine therefore satisfy the identity
cosh
2
ðtÞ2 sinh
2
ðtÞ5 1: ð3:10:15Þ
t
m Figure 16 y 5 sinh(t)
260 Chapter 3 The Derivative
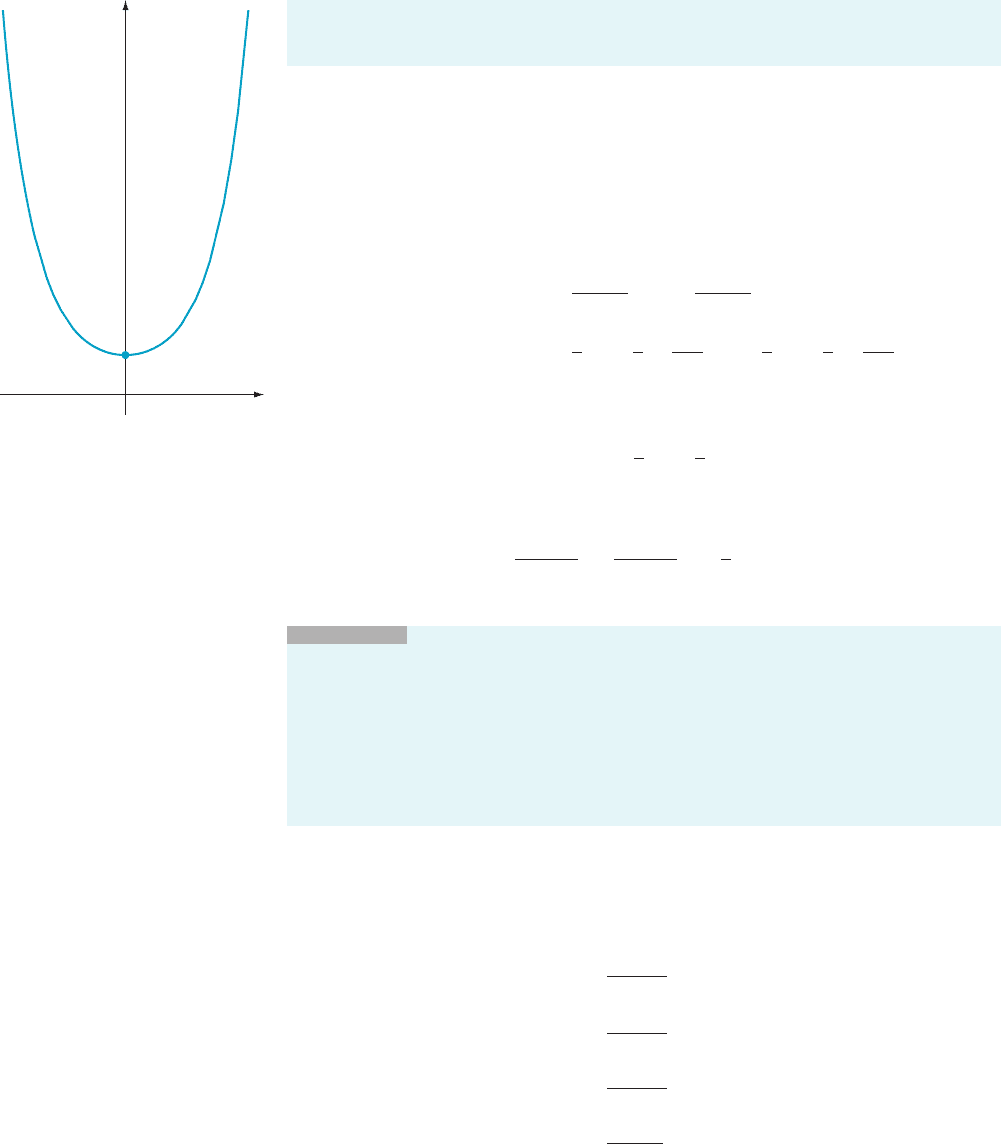
In other words, the point (cosh (t), sinh (t)) lies on the “unit hyperbola” whose
equation is x
2
2y
2
5 1.
⁄ EXAMPLE 10 Show that
coshð2tÞ5 cosh
2
ðtÞ1 sinh
2
ðtÞ and sinhð2tÞ5 2sinhðtÞcoshðtÞ:
Solution The
basic method of establi shing identities among the hyperbolic
trigonometric functions is to convert them to exponential functions. Thus
cosh
2
ðtÞ1 sinh
2
ðtÞ5
e
t
1e
2t
2
2
1
e
t
2e
2t
2
2
5
1
4
e
2t
1
1
2
1
1
4e
2t
1
1
4
e
2t
2
1
2
1
1
4e
2t
:
On simplification, we obtain
cosh
2
ðtÞ1 sinh
2
ðtÞ5
1
2
e
2t
1
1
2
e
22t
5 coshð2tÞ:
Similarly
2 sinh ðtÞcosh ðtÞ5 2
e
t
2 e
2t
2
e
t
1 e
2t
2
5
1
2
ðe
2t
2 e
22t
Þ5 sinh ð2tÞ: ¥
INSIGHT
There are many analogies between the standard trigonometric functions
and the hyperbolic trigonometric functions. Compare the identities in Example 10 with
the circular trigonometric identities
cos ð2tÞ5 cos
2
ðtÞ2 sin
2
ðtÞ and sin ð2tÞ5 2 sinðtÞcosðtÞ:
As a rule of thumb, there is a hyperbolic trigonometric formula for every circular tri-
gonometric formula. But you must exercise some caution because there are some dif-
ferences in the occurrence of minus signs.
Just as the tangent, the cotangent, the secant, and the cosecant can be defined as
quotients involving the sine and cosine, so can the hyperbolic tangent (tanh), hyper-
bolic cotangent (coth), hyperbolic secant (sech), and hyperbolic cosecant (csch):
tanhðtÞ 5
sinhðtÞ
coshðtÞ
; all real t;
cothðtÞ 5
coshðtÞ
sinhðtÞ
; t 6¼ 0;
sech ðtÞ 5
1
coshðtÞ
; all real t;
csch ðtÞ 5
1
sinhðtÞ
; t 6¼ 0:
t
(0, 1)
m Figure 17 y 5 cosh(t)
3.10 Other Transcendental Functions 261
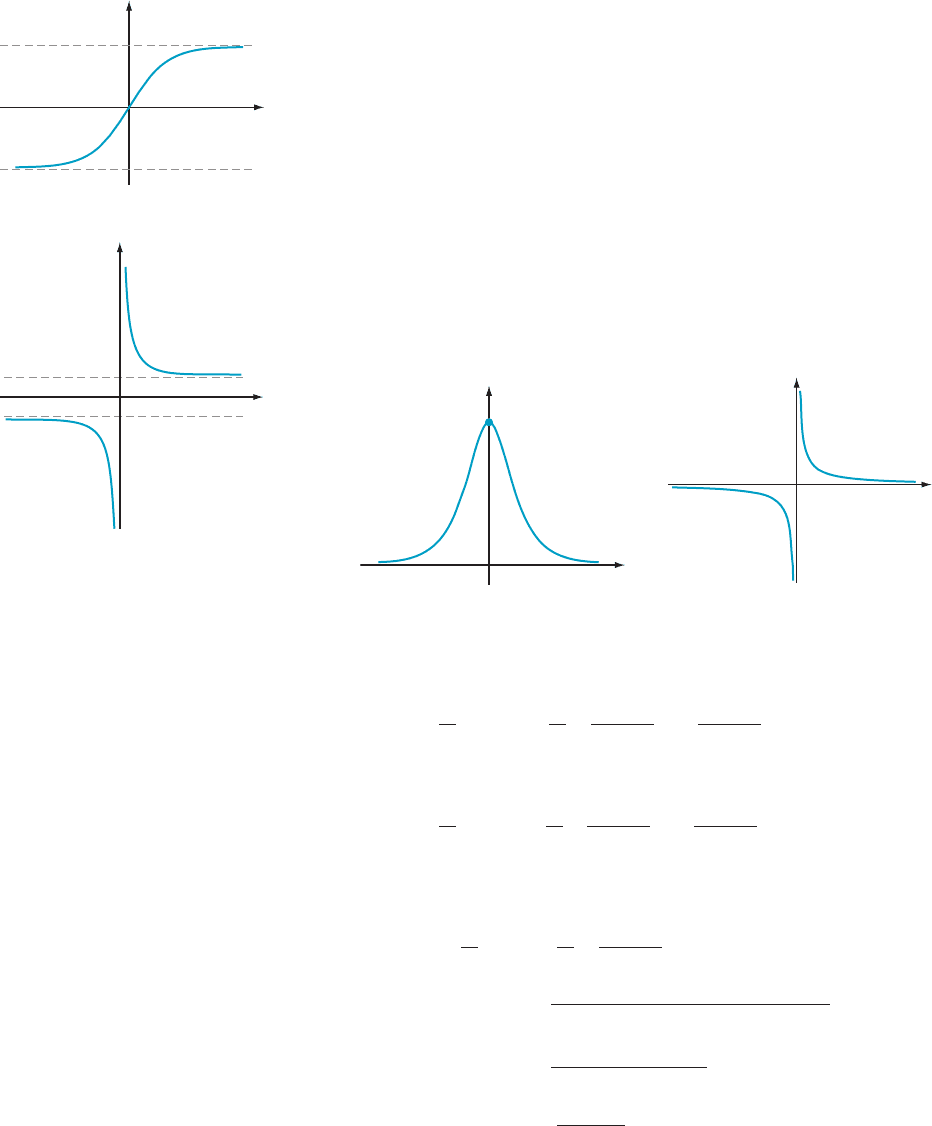
The graphs of these hyperbolic functions are given in Figures 18, 19, 20, and 21. Notice
that the graphs of y 5 tanh (x) and y 5 coth (x) have the lines y 5 1 and y 521as
horizontal asymptotes:
lim
x-N
tanhðxÞ5 lim
x-N
coth ðxÞ5 1 and lim
x- 2 N
tanhðxÞ5 lim
x- 2 N
cothðxÞ521:
The graphs of sech and csch have the line y 5 0 as a horizontal asymptote:
lim
x-N
sech ðxÞ5 lim
x- 2 N
sech ðxÞ5 0 and lim
x-N
csch ðxÞ5 lim
x- 2 N
csch ðxÞ5 0:
The graphs of coth and csch have the line x 5 0 as a vertical asymptote:
lim
x-0
1
cschðxÞ5 lim
x-0
1
cothðxÞ5 N an d lim
x-0
2
cschðxÞ5 lim
x-0
2
coth 52N:
Derivatives of the
Hyperbolic Functions
It is easy to calculate that
d
dt
cosh ðtÞ5
d
dt
e
t
1 e
2t
2
5
e
t
2 e
2t
2
5 sinh ðtÞ:
Similarly,
d
dt
sinh ðtÞ5
d
dt
e
t
2 e
2t
2
5
e
t
1 e
2t
2
5 cosh ðtÞ:
The calculation of the derivatives of the remaining four hyperbolic functions
follow from these formulas and the Quotient Rule. For example,
d
dt
tanh 5
d
dt
sinh ðtÞ
cosh ðtÞ
5
cosh ðtÞsinh
0
ðtÞ2 sinh ðtÞcosh
0
ðtÞ
cosh
2
ðtÞ
5
cosh
2
ðtÞ2 sinh
2
ðtÞ
cosh
2
ðtÞ
5
1
cosh
2
ðtÞ
5 sech
2
ðtÞ:
y 1
y
x
y 1
y coth(x)
m Figure 19
x
y
y tanh(x)
y 1
y 1
m Figure 18
(0, 1)
y sech(x)
x
y
m Figure 20
x
y
y csch(x)
m Figure 21
262 Chapter
3 The Derivative
The six differentiation formulas for the hyperbolic trigonometric functions are
listed in the following table.
d
dt
sinh ðtÞ 5 coshðtÞ
d
dt
cothðtÞ 52csch
2
ðtÞ
d
dt
coshðtÞ 5 sinhðtÞ
d
dt
sech ðtÞ 52sech ðtÞtanhðtÞ
d
dt
tanhðt Þ 5 sech
2
ðtÞ
d
dt
csch ðtÞ 52csch ðtÞcothðtÞ
The Inverse Hyperbolic
Functions
Because the hyperbolic sine is increasing on the entire real line, it is one-to-on e.
We may therefore discuss its inverse, sinh
21
(x) (or arcsinh(x)). The other hyper-
bolic functions are either not one-to one, or not onto R. But if their domains and
ranges are suitably restricted, then their inverses may be defined. These inverses
may be calculated explicitly in terms of funct ions that we already understand.
⁄ EX
AMPLE 11 Show that
sinh
21
ðxÞ5 ln
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
:
Solution Let y 5 sinh
21
(x). Then sinh (y) 5 x,ore
y
2 e
2y
5 2x. If we let u 5 e
y
,
then our last equation becomes u 2 u
21
5 2x,oru
2
2 2x u 2 1 5 0. We may solve
this quadratic equation in u by using the Quadratic Formula:
u 5
2x 6
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
ð2 2 x Þ
2
2 4ð1Þð2 1Þ
q
2
5 x 6
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
:
Because u 5 e
y
cannot be negative, the positive sign is used. Thus
e
y
5 u 5 x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
. From this we conclude that y 5 lnðx 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
Þ: ¥
For reference, the following list provides the formulas for the inverses of the
hyperbolic functions.
sinh
21
ðxÞ 5 ln ðx 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
Þ; all real x;
cosh
21
ðxÞ 5 ln ðx 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
2 1
p
Þ; x $ 1;
tanh
21
ðxÞ 5
1
2
ln
1 1 x
1 2 x
; 2 1 , x , 1;
coth
21
ðxÞ 5
1
2
ln
x 1 1
x 2 1
; jxj. 1;
sech
21
ðxÞ 5 ln
1 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 x
2
p
x
; 0 , x # 1;
csch
21
ðxÞ 5 ln
1
x
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 1 x
2
p
jxj
; x 6¼ 0:
3.10 Other Transcendental Functions 263

The following list shows the basic derivative rules for the inverse hyperbolic
functions,
d
dx
sinh
21
ðxÞ 5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 1 x
2
p
all real x;
d
dx
cosh
21
ðxÞ 52
1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
2 1
p
jxj. 1;
d
dx
tanh
21
ðxÞ 5
1
1 2 x
2
2 1 , x , 1;
d
dx
sech
21
ðxÞ 52
1
x
ffiffiffiffiffiffiffiffiffiffiffiffiffi
1 2 x
2
p
0 , x , 1
The next example will derive these equations in a somewh at more general form.
⁄ EX
AMPLE 12 Let a be a positive constant. Show that
d
dx
sinh
21
x
a
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
a
2
1 x
2
p
; ð3:10:16Þ
d
dx
1
a
tanh
21
x
a
5
1
a
2
2 x
2
; ð3:10:17Þ
and
d
dx
1
a
sech
21
x
a
52
1
x
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
a
2
2 x
2
p
: ð3:10:18Þ
Solution We
can use the explicit formulas for the inverse hyperbolic functions. For
example, because sinh
21
ðxÞ5 lnðx 1
ffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 1
p
Þ; we have
sinh
21
x
a
5 ln
x
a
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
a
2
1 1
s
5 ln
x
a
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
a
5 ln
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
a
5 ln
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
2 lnðaÞ:
Therefore for u 5 x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
,wehave
d
dx
sinh
21
x
a
5
d
dx
lnðx 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
Þ2 lnðaÞ
5
d
dx
lnðuÞ2
d
dx
lnðaÞ5
d
dx
lnðuÞ:
We apply the Chain Rule to obtain
d
dx
sinh
21
x
a
5
d
du
lnðuÞ
du
dx
5
1
u
du
dx
5
1
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
d
dx
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
5
1
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
1 1
x
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
:
The rest of the calculation consists of algebraic simplification:
d
dx
sinh
21
ðxÞ5
1
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
1 1
x
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
5
1
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
x 1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
5
1
ffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
x
2
1 a
2
p
:
264 Chapter 3 The Derivative
