Blank B.E., Krantz S.G. Calculus: Single Variable

Подождите немного. Документ загружается.

Proof. Let fa 5 x

, x

, ...,x

5 bg be the uniform partition of order N.By

applying the Mean Value Theorem to F on each subinterval I

, we obtain a point s

in I

such that

Fðx

Þ2 Fðx

j21

2 x

j21

5 F

ðs

Þ:

Therefore

Fðx

Þ2 Fðx

j21

Þ5 F

ðs

Þðx

2 x

j21

Þ5 f ðs

ÞΔx:

The Riemann sum corresponding to the choice of points S

5 fs

, s

, ...,s

g is

given by

Rðf ; S

Þ¼

j¼1

f ðs

ÞΔx

j¼1



Fðx

Þ2 Fðx

j21





Fðx

Þ2 Fðx





Fðx

Þ2 Fðx





Fðx

Þ2 Fðx



1 þ



Fðx

N21

Þ2 Fðx

N22





Fðx

Þ2 Fðx

N21



This sort of telescoping sum has been considered in Example 4 from Section 5.1.

Observe that the terms 2F (x

) and F (x

) appear once in this sum. The other

terms, F (x

) with 0 , j , N, all appear twice: once with a positive sign and once with

a negative sign. Therefore after the ensuing cancellations, only the terms 2F (x

)

and F(x

) remain. We obtain

Rðf ; S

Þ5 Fðx

Þ2 Fðx

Þ5 FðbÞ2 FðaÞ:

From this equation and Theorem 2, we deduce that

f ðxÞdx 5 lim

N-N

Rðf ; S

Þ5 lim

N-N

ðFðbÞ2 FðaÞÞ

|ﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ}

independent of N

5 FðbÞ2 FðaÞ: ’

INSIGHT

Formula (5.2.4) holds for every antiderivative of f. Thus if F and G are

both antiderivatives of f, then F (b) 2 F (a) must equal G (b) 2 G (a) because, according

to Theorem 3, each difference is equal to

f ðtÞdt . We can also deduce this fact from our

work in Section 4.2: Because F

5 G

(5 f ), Theorem 5 from Section 4.2 tells us that there

is a constant C such that G 5 F 1 C. It follows that

GðbÞ2 GðaÞ5 ðFðbÞ1 CÞ2 ðFðaÞ1 CÞ5 FðbÞ2 FðaÞ:

The signiﬁcance for us is that we do not have to choose a particular antiderivative when

we apply Theorem 3.

Theorem 3 provides us with a very powerful tool for calculating deﬁnite

integrals. The right side of equation (5.2.4) occurs so frequently in calculus that a

special notation has been introduced: Fj

and FðxÞj

x5 b

x5 a

are both used to symbolize

the difference F (b) 2 F (a). That is,

5 FðbÞ2 FðaÞ and FðxÞj

x5 b

x5 a

5 FðbÞ2 FðaÞ:

5.2 The Riemann Integral 395

Theorem 3 states the ﬁrst of two important relationships between the two main

branches, differentiation and integration, of calculus. The second of these rela-

tionships will be discussed in Section 5.4. Together, they make up the Fundamental

Theorem of Calcul us.

⁄ EX

AMPLE 3 Evaluate

ﬃﬃﬃ

dx.

Solution We

calculate the antiderivative FðxÞ5

2=3

of the integrand f (x) 5 x

21/3

From Theorem 3, it follows that

ﬃﬃﬃ

dx 5 Fð27Þ2 Fð1 Þ5

ð27

2=3

2 1

2=3

ð9 2 1Þ5 12: ¥

⁄ EX

AMPLE 4 Evaluate

sinðxÞdx.

Solution Because F (x) 52cos( x )

is an antiderivative of f (x) 5 sin (x), it follows

that

sinðxÞdx 5 FðπÞ2 Fð0Þ52cosðπÞ2 ð2cosð0ÞÞ52ð21Þ2 ð21Þ5 2: ¥

Using the Fundamental

Theorem of Calculus to

Compute Areas

Let f be a positive continuous function deﬁned on an interval [a, b]. In Section 5.1,

we deﬁned the area A of the region under the graph of f to be a limit of

Riemann sums (as we now call them ). Theorem 2 tells us that this limit exists. If

we can ﬁnd an antiderivative F of f, then we can use Theorem 3 to calculate

A 5

f ðxÞdx 5 FðbÞ2 FðaÞ:

⁄ EX

AMPLE 5 Calculate the area A of the region that lies under the graph

of f (x) 5 x

, above the x-axis, and between the vertical lines x 5 2andx 5 6.

Solution In

Example 7 from Section 5.1, we found that A 5 208/3 by means of a

somewhat tedious computation. We can now use Theorem 3 to calculate A much

more easily. Because F (x) 5 x

/3 is an antiderivative of f (x) 5 x

, as we know from

formula (4.13), we have

A 5

dx 5 Fð6Þ2 Fð2Þ5

208

: ¥

⁄ EX

AMPLE 6 Calculate the area A of

the shaded region in Figure 11.

Solution Because 2 ln(x) 2 x is an antiderivative of 2/x 2 1, we have

A 5



2 1



dx 5



2lnðxÞ2 x





x5 2

x5 1



2lnð2Þ2 2





2lnð1Þ2 1



|ﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄﬄﬄ}

Note parentheses

Therefore

A 5 ð2lnð2Þ2 2Þ2 ð0 2 1Þ5 2lnð2Þ2 2 1 1 5 2lnð2Þ2 1: ¥

y   1

m Figure 11

396 Chapter

5 The Integral

INSIGHT

The minus sign in the expression FðxÞj

x5 b

x5 a

5 FðbÞ2 FðaÞ is the source of

many algebraic errors. It is a good idea to enclose F (a) in parentheses when it is negative or

when it involves a sum of terms. Doing so will help you distribute the minus sign correctly.

QUICK QUIZ

1. If fs

, s

g is a choice of points associated with the order 4 uniform partition

of the interval [2 1, 2], what is the smallest possible value that can be chosen

for s

? The largest?

2. What are the lower and upper Riemann sums for f ( x ) 5 x

when the order 4

uniform partition of [21, 2] is used?

3. Evaluate

ð3x

2 2xÞdx.

4. True or false:

f ðxÞdx 5

f ðuÞdu.

5. True or false:

f ðuÞdx 5

f ðxÞdu.

Answers

1. Smallest: 1/2; Largest: 5/4 2. Lower: 45/32; Upper: 327/64 3. 6

True 5. False

EXERCISES

Problems for Practice

c In Exercises 124, for the given function f, interval I, and

uniform partition of order N:

a. Evaluate

the Riemann sum R( f, S) using the choice of

points S that consists of the midpoints of the subintervals

of I.

b. Evaluate the deﬁnite integral that R( f, S) approximates. b

1. f (x) 5 1/x S5 f4,

8, 12g, I 5 [2, 14], N 5 3

2. f (x) 5 3 2 x

/2 S5 f24, 22, 0g, I 5 [25, 1], N 5 3

3. f (x) 5 x

S5 f21, 1, 3, 5g, I 5 [22, 6], N 5 4

4. f (x) 5 x

1 x S5 f23, 1, 5, 9g, I 5 [25, 11], N 5 4

c In Exercises 528, for the given function f,

interval I, and

uniform partition of order N:

a. Evaluate

the Riemann sum R( f, S) using the choice of

points S that consists of the left endpoints of the sub-

intervals of I.

b. Evaluate the deﬁnite integral that R( f, S) approximates. b

5. f (x) 5 x

1 4x S 5 f1, 3, 5g, I 5 [1, 7], N 5 3

6. f (x) 5 sin (x) S 5 f0, π/3, π2/3, πg, N 5 4

7. f (x) 5 jxjS5 f27, 24, 21, 2g, I 5 [27, 5], N 5 4

8. f (x) 5 1/x S5 f1, 5/4. 3/2, 7/4, 2g, I 5 [1, 9/4], N 5 5

c In Exercises 9212, for the given function f,

interval I, and

uniform partition of order N:

a. Evaluate

the Riemann sum R( f, S) using the choice of

points S that consists of the right endpoints of the sub-

intervals of I.

b. Evaluate the deﬁnite integral that R( f, S) approximates. b

9. f (x) 5 e

S5 f22, 0, 2g, I 5 [24, 2], N 5 3

10. f (x) 5 1/x S5 f1, 5/4, 3/2, 7/4, 2g, I 5 [3/4, 2], N 5 5

11. f (x) 5 cos (x) S5 f2π/3, π/3, π,5π/3g, I 5 [2π,5π/3],

N 5 4

12. f (x) 5 x

2 x S 5 f22, 21, 0, 1g, I 5 [23, 1], N 5 4

c In Exercises 13216, calculate the lower and upper Rie-

mann

sums for the given function f, interval I, and uniform

partition of order 2. b

13. f (x) 5 x

I 5 [21/2, 2]

14. f (x) 5 x

2 2x 1 2 I 5 [0, 3]

15. f (x) 5 cos (x) I 5 [0, 4π/3]

16. f (x) 5 x 1 1/xI5 [1/2, 2]

c In each of Exercises 17223, sketch the integrand of the

given

deﬁnite integral over the interval of integration. Eval-

uate the integral by calculating the area it represents. b

17.

ﬃﬃﬃ

18.

ðcos

ðxÞ1 sin

ðxÞÞdx

19.

jxjdx

20.

ð2 1 signumðxÞÞdx

21.

ð1 1 xÞdx

22.

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 2 x

23.

jx 2 1jdx

c In each of Exercises 24244, evaluate the given deﬁnite

integral

by ﬁnding an antiderivative of the integrand and

applying Theorem 3. b

5.2 The Riemann Integral 397

24.

3π

π dx

25.

ð6x

2 2xÞdx

26.

27.

ﬃﬃﬃ

28.

ðx 2 1=

ﬃﬃﬃ

Þdx

29.

π=4

sec

ðxÞdx

30.

3π

π=3

sinðxÞdx

31.

π=3

secðxÞtanðxÞdx

32.

23=4

33.

1=3

34.

ð6=x

Þdx

35.

36.

ð1=xÞdx

37.

ð1=xÞdx

38.

11x

39.

40.

ð1=10

Þdx

41.

π=3

π=4

csc

ðxÞdx

42.

π=4

π=6

cscðxÞcotðxÞdx

43.

þ 5x þ 6

x þ 2

44.

þ 2x þ 1

Further Theory and Practice

c In each of Exercises 45250, a function f, an interval [a, b],

and a positive integer N are given. Calculate R( f, L

), the

Riemann sum R( f, S) using the midpoint of each subinterval

for the choice of points, and R( f, U

). (You will notice that

the inequalities of line (5.2.2) hold.) b

45. f (x) 5 e

I 5 [0, 1], N 5 1

46. f (x) 5 log

(x) I 5 [10, 100], N 5 1

47. f (x) 5 1/(1 1 x) I 5 [1, 3], N 5 2

48. f (x) 5 sin (x) I 5 [0, π/2], N 5 2

49. f (x) 5 ln(1 1 x) I 5 [0, 3], N 5 3

50. f (x) 5 1 1 sin (x) I 5 [2π/2, π/2], N 5 4

c In each of Exercises 51256, evaluate the given integral. b

51.

π=3

sinð3xÞdx

52.

π=4

π=6

cosð2xÞdx

53.

π=8

sec

ð2xÞdx

54.

1=2

1=4

cscðπxÞcotðπxÞ dx

55.

56.



1=2



57. Using f0, 1, 2, 3g for the partition, compute the upper and

lower Riemann sums for

f ðxÞ5 2x

2 9x

1 12x 1 1

and the interval [0, 3]. Verify that

f ðxÞdx is between

these two Riemann sums.

c In each of Exercises 58261, a function f and

an interval

[a, b] are given. Let Δx 5 (b 2 a)/2. Use the Mean Value

Theorem to ﬁnd points s

in [a,(a 1 b)/2] and s

in [(a 1 b)/2, b]

such that the Riemann sum

Rðf ; fs

; s

gÞ5 f ðs

ÞΔx 1 f ðs

ÞΔx

is equal to the Riemann integral of f over [a, b]. (Hint: The

method for ﬁnding the required points can be found in the proof

of Theorem 3.) b

58. f (x) 5 x

[0, 2]

59. f (x) 5 4x

[1, 5]

60. f (x) 5 4x 1 3x

[0, 2]

61. f (x) 5 1/x [1, 2]

62. Suppose that a , b. Obtain a formula for

dx that is

valid for every k . 0. Now suppose that k , 0. What

assumptions on a and b must you make in this case to apply

Theorem 3? With these assumptions, evaluate

dx for

k , 0, making sure to treat k 521asaspecialcase.

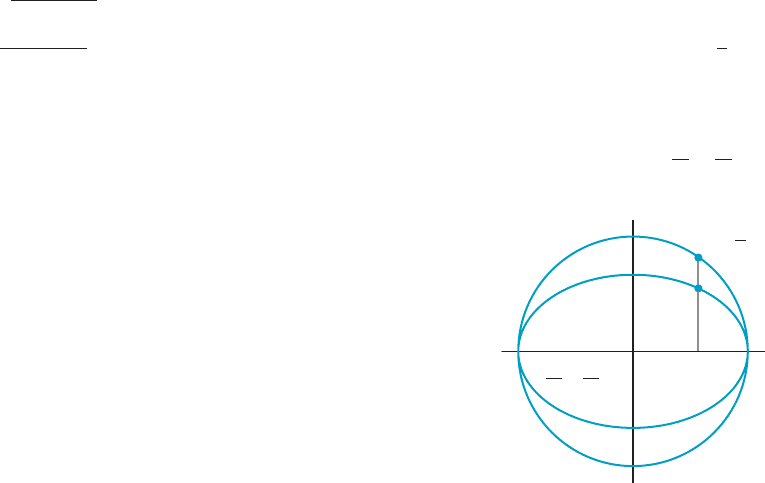
63. Suppose 0 , b , a. With respect to the ellipse and cir-

cumscribed circle plotted in Figure 12, show that

 y

By comparing Riemann sums for the ellipse with Riemann

sums for the circle, deduce that the area enclosed by the

ellipse

5 1

is πab.

64. Let x

5 1 1 j/N for 0 # j # N. Let S

5 fs

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

j21

 x

1 # j # Ng.

a. Verify that S

is a valid choice of points for the order

N uniform partition of interval [1, 2].

 y



 1

m Figure 12

398 Chapter

5 The Integral

b. Let f (x) 5 1/x

. Show that

Rðf ; S

Þ5 N

j5 1



N 1 j 2 1

N 1 j



c. For the same f, prove that R( f, S

) 5 1/2 for every N.

(Use part b and your result from Exercise 37 from

Section 5.1.)

d. Use part c to evaluate

1=x

dx.

65. Let c be the midpoint of [a, b]. Let m and h be any con-

stants. Show that



mðx 2 cÞ1 h



dx 5 hðb 2 aÞ:

66. Suppose that f is a continuous, positive function on [a, b].

Let c be the midpoint of [a, b]. Let L denote the line that

is tangent to y 5 f (x) at the point (c, f (c)). Suppose that

the graph of f lies above L. Use the preceding exercise to

deduce that



a 1 b



ðb 2 aÞ#

f ðxÞdx:

67. Suppose that f is a positive function whose graph over the

interval [a, b] lies under the chord joining (a, f (a)) to (b,

f (b)). By calculating the area of the trapezoid with ver-

tices (a, 0), (b, 0), (b, f (b)), and (a, f (a)), deduce that

f ðxÞdx # ðb 2 aÞ



f ðaÞ1 f ðbÞ



68. Suppose f is continuous and increasing on [a, b]. Show that

Rðf ; U

Þ2 Rðf ; L

Þ5 ðf ðbÞ2 f ðaÞÞ

b 2 a

69. Suppose that f is continuous on [a, b], differentiable on

(a, b), and that jf

(x)j # M

for x in (a, b). Let f‘

, ...,‘

and fu

,...,u

g be the points that give rise to the lower

and upper Riemann sums of order N. For each j 5 1 ... N,

apply the Mean Value Theorem to f on the interval with

endpoints ‘

and u

. Deduce that f (u

) 2 f (‘

) # M

Δx.

Use this inequality to prove that

Rðf ; U

Þ2 Rðf ; L

Þ# M

ðb2aÞ

Calculator/Computer Exercises

c In each of Exercises 7073, a Riemann sum R( f, fs

,...,

g) is to be calculated as an approximation to the given

deﬁnite integral. Use the given value of N and, for each j

between 1 and N, choose s

to be the midpoint of the j

subinterval of the uniform partition of order N. Figure 13

shows how Maple can be used to do this for

π=2

lnð1 1 sinðxÞÞdx and N 5 100. b

70.

ﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx N 5 80

71.

cos

ðπxÞdx N 5 100

72.

expð2x

Þdx N 5 40

73.

x sinðxÞ dx N 5 60

c In Exercises 7477, plot the function f over

the interval

[a, b]. Calculate the lower and upper Riemann sums R( f, L

)

and R( f, U

). (The exact value of

f ðxÞdx lies between

these two estimates.) b

74. f ðxÞ5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ﬃ

1 1

ﬃﬃﬃ

I 5 ½0; 9

75. f ðxÞ5



2 1 sinðxÞ



4=3

I 5 ½2π=2; π=2

76. f ðxÞ5 1=ð3 2 x

1=3

Þ I 5 ½1; 8

77. f ðxÞ5 secðx

=4Þ I 5 ½0;

ﬃﬃﬃ



5.3 Rules for Integration

The calculation of integrals can be simpliﬁed if we take note of certain rules.

THEOREM 1

If f and g are integrable functions on the interval [a, b] and if α is

a constant, then f 1 g, f 2 g, and α f are integrable, and

m Figure 13

5.3 Rules for Integration 399

ðf ðxÞ1 gðxÞÞdx 5

f ðxÞdx 1

gðxÞdx and

ðf ðxÞ2 gðxÞÞdx 5

f ðxÞdx 2

gðxÞdx;

α  f ðxÞdx 5 α 

f ðxÞdx;

αdx 5 α ðb 2 aÞ;

f ðxÞdx 5 0; and

e. If a # c # b; then

f ðxÞdx 1

f ðxÞdx 5

f ðxÞdx:

Notice that Theorem 1a says that f 1 g and f 2 g are integrable, provided that f

and g can be integrated separately. It also tells how to calculate the integrals of

f 1 g and f 2 g. Likewise, Theorem 1b says that αf is integrable, provided that f is. It

tells how to calculate the integral of αf. Rules a and b are consequences of ana-

logous limit rules that we learned in Section 2.2 of Chapter 2.

Theorem 1c states that integrating a constant α over an interval I results in α

times the length of I. In a sense, this rule simply states that the area of a rectangle is

the length of the base times the height. This may be proved either by referring to

the deﬁnition of the integral as a limit or by applyi ng Theorem 3 from Section 5.2

after noting that αx is an antiderivative of α.

Theorem 1d says that the integral over an interval of zero length is zero,

whereas rule 1e says that contiguous intervals of integration may be added. If we

think of the integral as representing area, for instance, then rules 1d and 1e are

geometrically plausi ble (see Figure 1).

⁄ EX

AMPLE 1 Calculate the integral

3 1 5t

dt:

Solution We

use Theorem 1a and 1b to rewrite the integral as

3 1 5t

dt 5

dt 1

dt 5 3 

dt 1 5 

tdt:

Because 2t

is an antiderivative of t

and t

/2 is an antiderivative of t, we can

evaluate each integral using the Fundamental Theorem of Calculus (Theorem 3,

Section 5.2):

Theorem 1d

acb

Theorem 1e

m Figure 1 Theorem 1d: Area 5 base 3 height 5 0 3 f (a) 5 0.

Theorem 1e: Area (abRP) 5 area (acQP) 1 area (cbRQ)

400 Chapter 5 The Integral

3 1 5t

dt 5 3 

dt 1 5 

tdt5 3 





t5 2

t5 1



1 5





t5 2

t5 1



5 3



2 ð21Þ



1 5





5 9: ¥

⁄ EX

AMPLE 2 Can we use the equations

xdx5 1=2 and

dx 5 1=3to

evaluate the integral

dx by observing that its integrand x

is the product of x

and x

Solution No.

It is tempting, but wrong, to think that we can somehow factor this

integral into two simpler integrals that we already know. Using the Fundamental

Theorem of Calculus, we obtain

dx 5



x5 1

x5 0

2 0 5

;

which is certainly not (1/2) 3 (1/3). Thus

f ðxÞgðxÞdx 6¼

f ðxÞdx 

gðxÞdx

in general. In other words, the integral of a product is not the product of the

integrals. In Chapt er 7, we will learn techniques for handling integrals of

products.

⁄ EXAMPLE 3 Let f be a continuous function on the interval [22, 5] such that

f ðxÞdx 5 6and

f ðxÞdx 522:

Use this information to evaluate

f ðxÞdx.

Solution Using

Theorem 1e, we ha ve

f ðxÞdx 5

f ðxÞdx 1

f ðxÞdx 5 6 1 ð22Þ5 4: ¥

⁄ EXAMPLE 4 Suppose that g is

a continuous function on the interval [4, 9]

that satisﬁes

gðxÞdx 5 8and

gðxÞdx 527:

Calculate

gðxÞdx.

Solution We

use Theorem 1e to see that

8 5

gðxÞdx 5

gðxÞdx 1

gðxÞdx 527 1

gðxÞdx:

Therefore

gðxÞdx 5 15. ¥

5.3 Rules for Integration 401

⁄ EXAMPLE 5 Evaluate

π=2

5=2

sinðt

Þdt.

Solution Theorem

1d tells us that

f ðxÞdx 5 0, no matter what the integrand is.

Therefore

π=2

5=2

sinðt

Þdt 5 0: ¥

Reversing the Direction

of Integration

In the deﬁnition of the integral, the upper limit of integration is taken to be greater

than or equal to the lower limit. However, it is sometimes convenient to allow

the upper limit to be less than the lower limit. If a is greater than b, then we deﬁne

f ðxÞdx for a function f integrable on [b, a]by

f ðxÞdx 52

f ðxÞdx: ð5:3:1Þ

⁄ EX

AMPLE 6 Evaluate

dt.

Solution Notice

that F (t) 5 t

/3 is an antiderivative of t

. Thus

dt 52

dt 52ðFð1Þ2 Fð24ÞÞ 52



264



: ¥

INSIGHT

Remember that if f is nonnegative and a , b, then the integral of f

over [a, b] represents area and is positive. In Example 6, f (t) 5 t

is nonnegative, but

dt is negative. This is because the lower limit of integration is greater than the

upper limit.

Let us verify that the Fundamental Theorem of Calculus (Theorem 3 from

Section 5.2) remains true regardless of the order of the limits of integration.

THEOREM 2

If f is continuous on an interval that contains the points a and b

(in any order) and if F is an antiderivative of f, then

f ðxÞdx 5 FðbÞ2 FðaÞ: ð5:3: 2 Þ

Proof. If a , b,

then equation (5.3.2) is precisely formula (5.2.4). If a 5 b, then

the right side of equation (5.3.2) is 0 and so, by Theorem 1d, is the left side. In the

remaining case, a . b, we have

f ðxÞdx 5

ð5:3:1Þ

f ðxÞdx 5

ð5:2:4Þ



FðaÞ2 FðbÞ



5 FðbÞ2 FðaÞ: ’

Now that we have deﬁned the deﬁnite integral even when the lower limit of

inte

gration is greater than the upper limit of integration, we may ask if Theorem 1e

continues to hold no matter what the order of a, b,andc may be. The answer is

“yes,” and we state the result as a theorem.

402 Chapter 5 The Integral

THEOREM 3

If f is integrable on an interval that contains the three points a, b,

and c (in any order), then

f ðxÞdx 1

f ðxÞdx 5

f ðxÞdx: ð5:3:3Þ

Rather than give a general proof of Theorem 3, we will work out one example.

⁄ EX

AMPLE 7 Suppose that f is integrable on [1, 5]. Show that equation

(5.3.3) is valid for a 5 5, b 5 3, and c 5 1.

Solution We

have

f ðxÞdx 1

f ðxÞdx 52

f ðxÞdx 2

f ðxÞdx By formula ð5:3: 1 Þ



f ðxÞdx 1

f ðxÞdx



Reorder the terms

f ðxÞdx By Theorem 1e

f ðxÞdx: By formula ð5:3:1Þ ¥

Order Properties

of Integrals

In this subsection, we collect a number of facts concerning integrals and order

relations.

THEOREM 4

If f, g, and h are integrable on [a, b] and

gðxÞ# f ðxÞ# hðxÞ for x in ½a; b

then

gðxÞdx #

f ðxÞdx #

hðxÞdx: ð5:3:4Þ

In particular, if f is integrable on [a, b]andifm and M are constants such that

m # f ðxÞ# M

for all x A [a, b], then

m ðb 2 aÞ#

f ðxÞdx # M ðb 2 aÞ: ð5:3:5Þ

Proof. If S

5 fs

g is a choice of points associated with the order N uniform

partition of [a, b ], then

j5 1

gðs

ÞΔx #

j5 1

f ðs

ÞΔx #

j5 1

hðs

ÞΔx;

or R(g, S

) # R( f, S

) # R(h, S

). Line (5.3.4) results by letting N tend to

inﬁnity.

In the particular case when g(x) 5 m and h(x) 5 M for all x, line (5.3.4) sim-

pliﬁes to become line (5.3.5). ’

5.3 Rules for Integration 403

⁄ EXAMPLE 8 Use the inequalities of line (5.3.5) to estimate

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx.

Solution Because

ﬃﬃﬃﬃﬃﬃﬃﬃ

ﬃﬃﬃﬃﬃ

1 1 x

is increasing on [0, 2], we have

1 5

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ﬃ

1 1 0

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 2

5 3 for 0 # x # 2:

We use the estimates of line (5.3.5) with a 5 0, b 5 2, m 5 1, and M 5 3 to obtain

2 5 1 ð2 2 0Þ#

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 1 x

dx # 3 ð2 2 0 Þ5 6:

The value of the given integral is between 2 and 6.

INSIGHT

The integrand of Example 8 does not have an elementary antiderivative.

Therefore we cannot use the Fundamental Theorem of Calculus to evaluate the

integral exactly. In such cases, we must rely on an approximation. As the estimates

obtained in Example 8 suggest, Theorem 4 usually gives us only an order of magnitude

estimate. In Section 5.8, we study techniques for achieving accurate approximations of

deﬁnite integrals.

The following theorem, which is used often, is obtained by taking m 5 0 in the

ﬁrst inequality of line (5.3.5).

THEOREM 5

If f is integrable on [a, b]andiff (x) $ 0, then

f ðxÞdx $ 0.

The next theorem may be deduced from line (5.3.4) by taking g (x) 52jf (x)j

and h(x) 5 jf(x)j.

THEOREM 6

If f is integrable on [a, b], then



f ðxÞdx



jf ðxÞjdx: ð5:3:6Þ

INSIGHT

Thinking of the deﬁnite integral as a limit of Riemann sums, we may

regard inequality (5.3.6) as a continuous analogue of the (discrete) triangle inequality

1 1 A

j # j A

j1 1 j A

j. In fact, if we set A

5 f (s

) Δx for 1 # j # N and

let N -N, then we obtain an alternative proof of inequality (5.3.6).

⁄ EXAMPLE 9 Verify inequality (5.3.6) for f (x) 5 x

1 x and the interval

[21, 2].

Solution Because x

/3 1 x

/2 is an antiderivative of f (x), Theorem 3 from Section

5.2 gives us

f ðxÞdx 5















404 Chapter 5 The Integral