Ismail M., Koelink E. (editors) Theory and Applications of Special Functions
Подождите немного. Документ загружается.

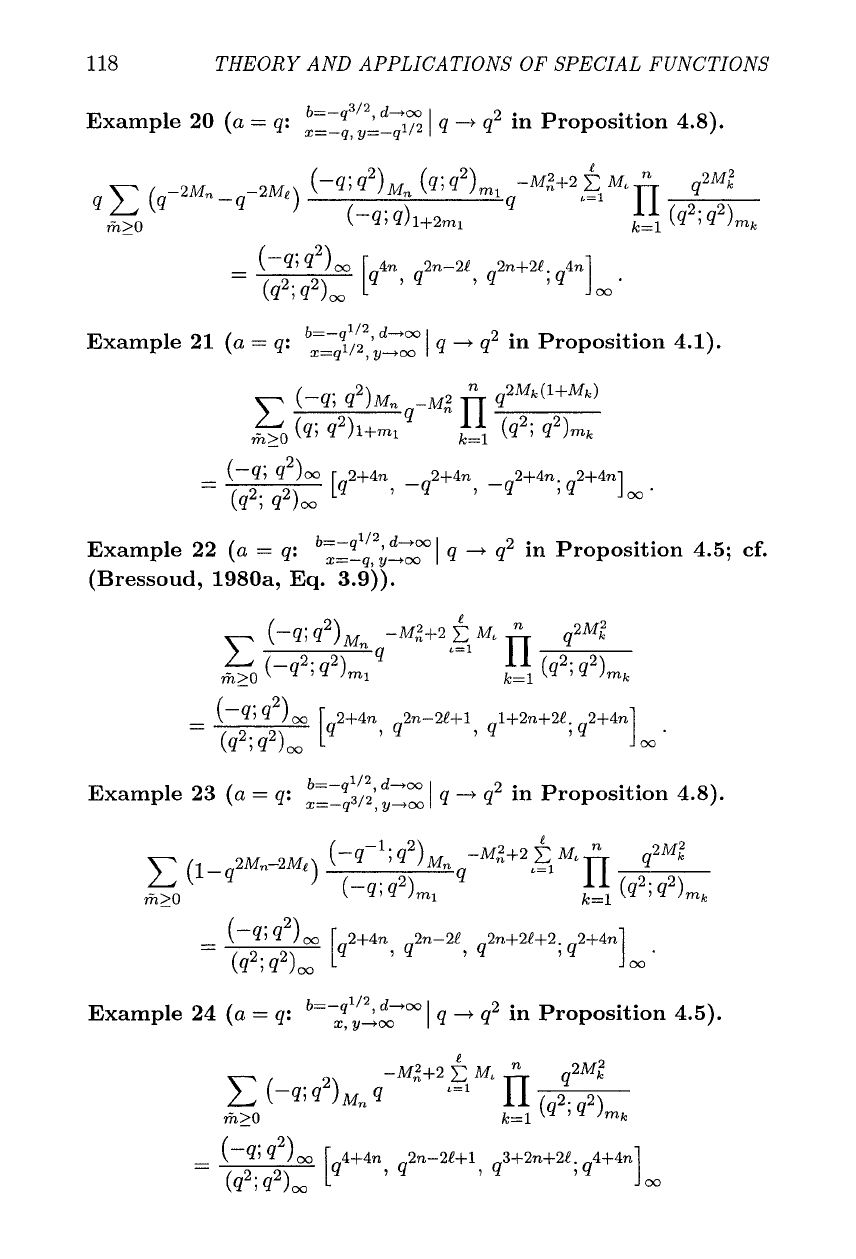
118
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
b=-q3/2, d-00
Example 20 (a
=
q:
x=-q,
y=-q1/2
1
q
+
q2
in Proposition 4.8).
b=-q'/2, d+m
Example 21 (a
=
q:
x=ql,2,
+m
I
q
+
q2
in Proposition 4.1).
Example 22 (a
=
q:
b=-q
d400
x=-q
,
Y--+OO
I
q
-+
q2
in Proposition 4.5;
cf.
(Bressoud, 1980a, Eq. 3.9)).
Example 23 (a
=
q:
b=-q
112, d+,
=-
I
q
+
q2
in Proposition 4.8).
r
Y-'m
Example 24 (a
=
q:
b=-q
1/29d^m
I
q
+
q2
in Proposition 4.5).
x,
Y+00
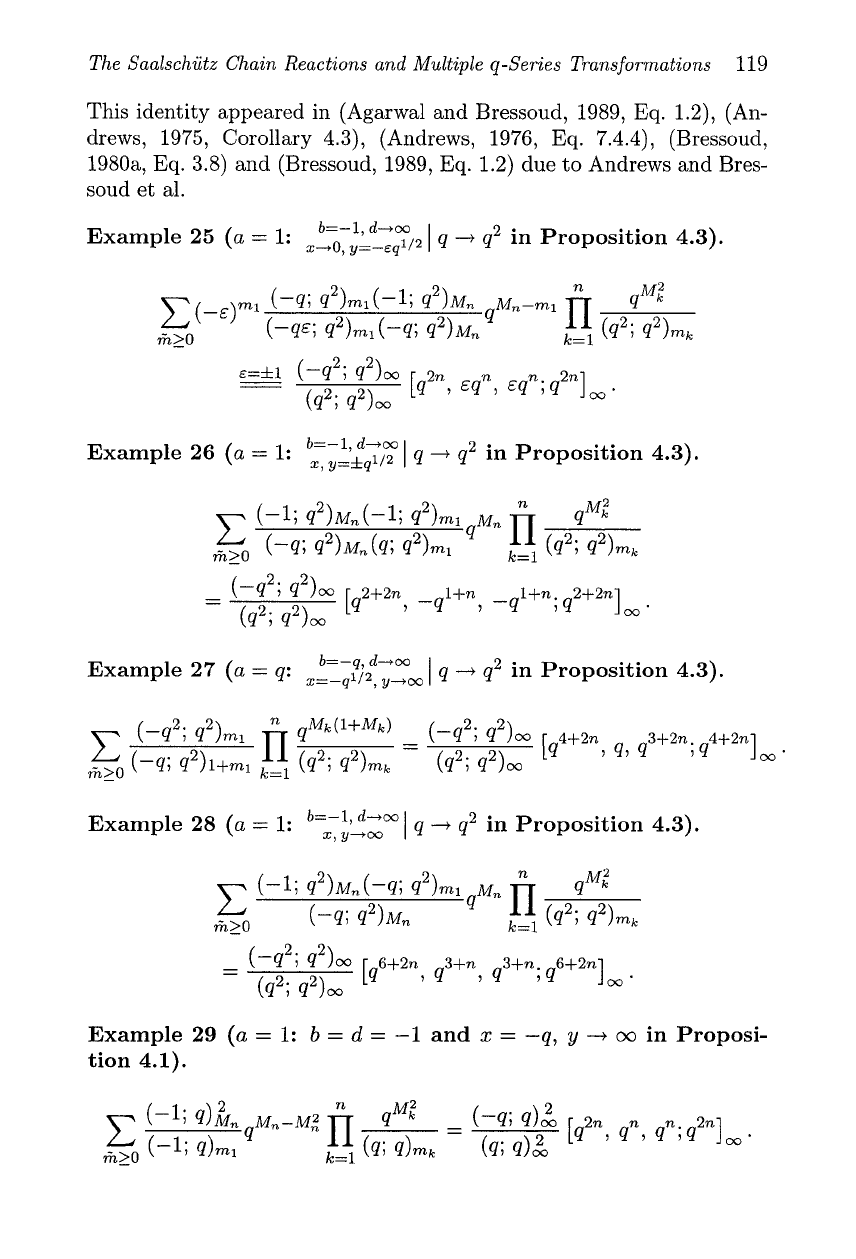
The Saalschiitz Chain Reactions and Multiple q-Series Transformations 119
This identity appeared in (Agarwal and Bressoud, 1989,
Eq.
1.2), (An-
drew~, 1975, Corollary 4.3), (Andrews, 1976,
Eq.
7.4.4), (Bressoud,
1980a,
Eq.
3.8) and (Bressoud, 1989,
Eq.
1.2) due to Andrews and Bres-
soud et al.
b=-1,
d-tm
Example
25
(a
=
1:
x+o,
y=-Eq1/2
1
t
q2
in Proposition 4.3).
b=-1,
d4m
Example
26
(a
=
1:
%,
y=+ql,2
I
q
t
q2
in Proposition 4.3).
Example
27
(a
=
q:
b=-q
,
d
-to3
z=-ql,,,
+,
1
q
+
q2
in Proposition 4.3).
Example
28
(a
=
1:
b=x~j"m
I
q
+
q2
in Proposition 4.3).
Example
29
(a
=
1:
b
=
d
=
-1
and
x
=
-q,
y
t
oo
in Proposi-
tion
4.1).

120
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
b=d=-1
Example
30
(a
=
1:
z=-
9,
Y+m
I
q
-4
q2
in
Proposition
4.3).
These examples are selected from about two hundreds multiple Rogers-
Ramanujan identities derived from the propositions displayed in the last
section. More identities of such kind may be found in (Agarwal and
Bressoud, 1989)) (Andrews, 1984), (Bressoud, 1980a; Bressoud, 1989)
and (Stembridge, 1990)) mainly due to Andrews and Bressoud. For the
most recent development, we refer to (Bressoud et al., 2000)) (Garrett
et al., 1999), (Schilling and Warnaar, 1997), (Stanton, 2001) and (War-
naar, 2001).
Acknowledgments
The author thanks to the anonymous referee for the careful corrections
and useful suggestions.
References
Agarwal, A.
K.,
Andrews,
G.
E.,
and Bressoud,
D.
M.
(1987).
The Bailey lattice.
J.
Indian Math. Soc. (N.S.),
51:57-73.
Agarwal, A.
K.
and Bressoud, D. M.
(1989).
Lattice paths and multiple basic hyper-
geometric series. Pacific
J.
Math.,
136:209-228.
Andrews,
G.
E.
(1974).
An analytic generalization of the Rogers-Ramanujan identities
for odd moduli. Proc. Nat. Acad. Sci. U.S.A.,
71:4082-4085.
Andrews,
G.
E.
(1975).
Problems and prospects for basic hypergeometric functions.
In Askey,
R.,
editor, The Theory and Applications of Special Functions, pages
191-224.
Academic Press, New York.
Andrews,
G.
E.
(1976).
The Theory of Partitions, volume
2
of Encyclopedia of Math.
and Appl. Cambridge University Press, Cambridge.
Andrews,
G.
E.
(1979a).
Connection coefficient problems and partitions. In Relations
between combinatorics and other parts of mathematics (Proc. Sympos. Pure Math.,
Ohio State Univ., Columbus, Ohio,
1978),
Proc. Sympos. Pure Math., XXXIV,
pages
1-24.
Amer. Math. Soc., Providence, RI.
Andrews,
G.
E.
(l979b).
Partitions and Durfee dissection. Amer.
J.
Math.,
101(3):735-
742.
Andrews,
G.
E.
(1981).
Multiple q-series identities. Houston
J.
Math.,
7(1):11-22.
Andrews,
G.
E.
(1984).
Multiple series Rogers-Ramanujan type identities. Pacific
J.
Math.,
114(2):267-283.
Andrews,
G.
E.
(1986).
q-Series: Their Development and Application in Analysis,
Number Theory, Combinatorics, Physics, and Computer Algebra, volume
66
of

The Saalschutz Chain Reactions and Multiple q-Series Transformations
121
CBMS Regional Conference Series in Mathematics. Published for the Conference
Board of the Mathematical Sciences, Washington, DC.
Bailey,
W.
N.
(1935).
Generalized Hypergeometric Series. Cambridge University Press,
Cambridge.
Bailey,
W.
N.
(1947).
Some identities in combinatory analysis. Proc. London Math.
SOC. (2),
49:421-435.
Bailey,
W.
N.
(1948).
Identities of the Rogers-Ramanujan type. Proc. London Math.
SOC. (2),
5O:l-10.
Bressoud, D. M.
(1980a).
Analytic and combinatorial generalizations of the Rogers-
Ramanujan identities. Mem. Amer. Math. Soc.,
24(227):54.
Bressoud, D. M.
(1980b).
An analytic generalization of the Rogers-Ramanujan iden-
tities with interpretation. Quart. J. Math. Oxford Ser. 8,
31:385-399.
Bressoud, D. M.
(1981).
On partitions, orthogonal polynomials and the expansion of
certain infinite products. Proc. London Math. Soc.
(3),
42:478-500.
Bressoud, D. M.
(1988).
The Bailey lattice: an introduction. In Ramanujan Revisited
(Urbana-Champaign, Ill., 1987), pages
57-67.
Academic Press, Boston, MA.
Bressoud, D. M.
(1989).
Lattice paths and the Rogers-Ramanujan identities. In Num-
ber theory, Madras 1987, volume
1395
of Lecture Notes in Mathematics, pages
140-172.
Springer-Verlag, Berlin-New York.
Bressoud, D. M., Ismail, M., and Stanton, D.
(2000).
Change of base in Bailey pairs.
Ramanujan J.,
4(4):435-453.
Chu,
W.
(2002).
The Saalschiitz chain reactions and bilateral basic hypergeometric
series. Constructive Approx.,
18:579-598.
Garrett,
T.,
Ismail, M., and Stanton,
D.
(1999).
Variants of the Rogers-Ramanujan
identities. Adv. Appl. Math.,
23(3):274-299.
Gessel,
I.
and Stanton, D.
(1983).
Applications of q-Lagrange inversion to basic hy-
pergeometric series. Trans. Amer. Math. Soc.,
277:173-201.
Milne, S. C.
(1980).
A multiple series transformation of the very well poised
Pacific J. Math.,
91:419-430.
Schilling, A. and Warnaar, S.
0.
(1997).
A
higher-level Bailey lemma: Proof and
application. Preprint.
Slater, L.
J.
(1951).
A new proof of Rogers' transformations of infinite series. Proc.
London Math. Soc. (8),
53:460-475.
Slater,
L.
J.
(1952).
Further identities of the Rogers-Ramanujan type. Proc. London
Math. Soc. (.),
54:147-167.
Slater, L.
J.
(1966).
Generalized Hypergeometric Functions. Cambridge University
Press, Cambridge.
Stanton,
D.
(2001).
The Bailey-Rogers-Ramanujan group, volume
291
of Contempo-
rary Mathematics, pages
55-70.
Amer. Math. Soc., Providence,
RI.
Stembridge,
J.
R.
(1990).
Hall-Littlewood functions, plane partitions, and the Rogers-
Ramanujan identities. Trans. Amer. Math. Soc.,
319:469-498.
Warnaar, S.
0.
(2001).
The generalized Borwein conjecture
I:
The Burge transform,
volume
291
of Contemporary Mathematics, pages
243-267.
Amer. Math. Soc., Prov-
idence,
RI.
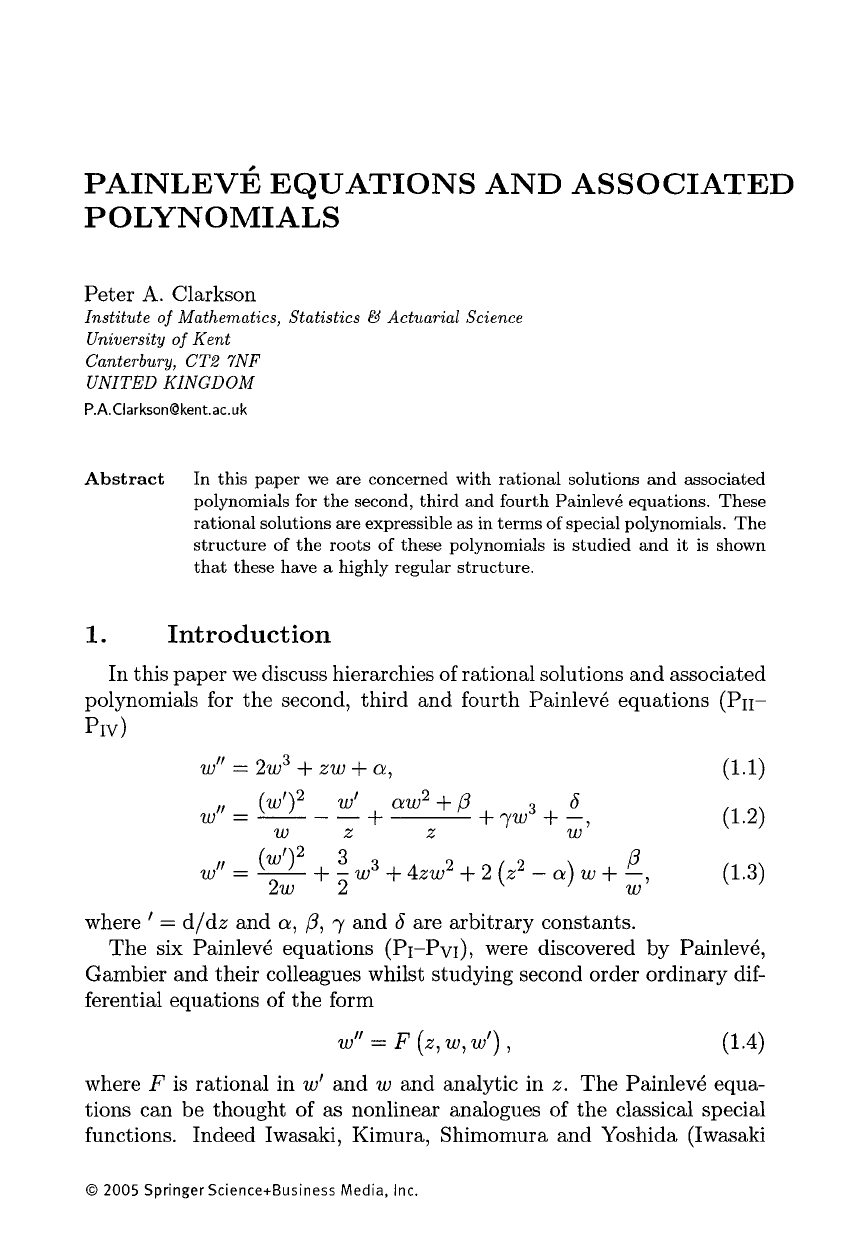
PAINLEVE
EQUATIONS
AND
ASSOCIATED
POLYNOMIALS
Peter
A.
Clarkson
Institute
of
Mathematics, Statistics
&
Actuarial Science
University
of
Kent
Canterbury, CT2 7NF
UNITED KINGDOM
P.A.Clarkson@kent.ac.uk
Abstract
In this paper we are concerned with rational solutions and associated
polynomials for the second, third and fourth Painlev6 equations. These
rational solutions are expressible as in terms of special polynomials. The
structure of the roots of these polynomials is studied and it is shown
that these have a highly regular structure.
1.
Introduction
In this paper we discuss hierarchies of rational solutions and associated
polynomials for the second, third and fourth Painlev6 equations (PII-
PIV)
where
'
=
d/dz and
a,
P,
y
and
6
are arbitrary constants.
The six Painlev6 equations (PI-PVI), were discovered by Painlev6,
Gambier and their colleagues whilst studying second order ordinary dif-
ferential equations of the form
where
F
is rational in
w'
and
w
and analytic in
Z.
The Painlev6 equa-
tions can be thought of as nonlinear analogues of the classical special
functions. Indeed Iwasaki, Kimura, Shimomura and Yoshida (Iwasaki
O
2005
Springer Science+Business Media, Inc.

124
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
et al., 1991) characterize the six Painlev6 equations as "the most im-
portant nonlinear ordinary differential equations" and state that ('many
specialists believe that during the twenty-first century the Painlev6 func-
tions will become new members of the community of special functions."
The general solutions of the Painlev6 equations are transcendental in
the sense that they cannot be expressed in terms of (known) classical
functions and so require the introduction of a new transcendental func-
tion to describe their solution. However it is well-known that PII-PVI,
possess hierarchies of rational solutions for special values of the param-
eters (see, for example, (Airault, 1979; Albrecht et al., 1996; Bassom
et al., 1995; Fokas and Ablowitz, 1982; F'ukutani et al., 2000; Gromak,
1999; Gromak et al., 2002; Okamoto, 1987a; Okamoto, 1987b; Okamoto,
1986; Okamoto, 1987c; Umemura and Watanabe, 1997; Umemura and
Watanabe, 1998; Vorob'ev, 1965; Watanabe, 1995; Yablonskii, 1959;
Yuan and Li, 2002) and the references therein). These hierarchies are
usually generated from "seed solutions" using the associated Backlund
transformations and frequently can be expressed in the form of determi-
nants through '%-functions".
Vorob'ev (Vorob'ev, 1965) and Yablonskii (Yablonskii, 1959) expressed
the rational solutions of PII in terms of the logarithmic derivative of
certain polynomials which are now known
as
the Yablonskii-Vorob'ev
polynomials. Okamoto (Okamoto, 1986) obtained analogous polynomi-
als related to some of the rational solutions of PIv, these polynomials
are now known as the Okamoto polynomials. Further Okamoto noted
that they arise from special points in parameter space from the point-
of-view of symmetry, which is associated to the affine Weyl group of
type
A?).
Umemura (Umemura, 2003) associated analogous special
polynomials with certain rational and algebraic solutions of PIII, PV
and PvI which have similar properties to the Yablonskii-Vorob'ev poly-
nomials and the Okamoto polynomials; see also (Noumi M. and H.,
1998; Umemura, 1998; Umemura, 2001; Yamada, 2000). Subsequently
there have been several studies of special polynomials associated with the
rational solutions of PII (F'ukutani et al., 2000; Kajiwara and Masuda,
1999a; Kajiwara and Ohta, 1996; Taneda, 2000), the rational and alge-
braic solutions of PIII (Kajiwara and Masuda, 199913; Ohyama, 2001))
the rational solutions of
PIv
(F'ukutani et al., 2000; Kajiwara and Ohta,
1998; Noumi and Yamada, 1999), the rational solutions of Pv (Masuda
et al., 2002; Noumi and Yamada, 199813) and the algebraic solutions
of
PvI
(Kirillov and Taneda, 2002b; Kirillov and Taneda, 2002a; Ma-
suda, 2002; Taneda, 2001a; Taneda, 2001b). However the majority of
these papers are concerned with the combinatorial structure and deter-
minant representation of the polynomials, often related to the Hamil-

Painlevk Equations and Associated Polynomials
125
tonian structure and affine Weyl symmetries of the Painlev6 equations.
Typically these polynomials arise as the "T-functions" for special so-
lutions of the Painlev6 equations and are generated through nonlinear,
three-term recurrence relations which are Toda equations that arise from
the associated Backlund transformations of the Painlev6 equations. The
coefficients of these special polynomials have some interesting, indeed
somewhat mysterious, combinatorial properties (see (Noumi M. and H.,
1998; Umemura, 1998; Umemura, 2001; Umemura, 2003)). Addition-
ally these polynomials have been expressed as special cases of Schur
polynomials, which are irreducible polynomial representations of the
general linear group
GL(n)
and arise
as
T-functions of the Kadomtsev-
Petviashvili (KP) hierarchy (Jimbo and Miwa, 1983). The Yablonskii-
Vorob'ev polynomials associated with PII are expressible in terms of
2-reduced Schur functions (Kajiwara and Masuda, 1999a; Kajiwara and
Ohta, 1996), and are related to the T-function for the rational solution
of the modified Korteweg de Vries (mKdV) equation since PII arises as a
similarity reduction of the mKdV equation. The Okamoto polynomials
associated with PIv are expressible in terms of 3-reduced Schur functions
(Kajiwara and Ohta, 1998; Noumi and Yamada, 1999) since PIv arises
as
a similarity reduction of the Boussinesq equation (cf. (Clarkson and
Kruskal, 1989)), which belongs to the so-called 3-reduction of the KP
hierarchy (Jimbo and Miwa, 1983).
It is also well-known that PII-PVI possess solutions which are express-
ible in terms of the classical special functions; these are often referred
to as "one-parameter families of solutions". For PII these special func-
tion solutions are expressed in terms of Airy functions Ai(z) (Airault,
1979; Flaschka and Newell, 1980; Gambier, 1910; Okamoto, 1986), for
PIII they are expressed in terms of Bessel functions Jv(z) (Lukashevich,
1967a; Milne et al., 1997; Murata, 1995; Okamoto, 1987c), for PIv they
are expressed in terms of Weber-Hermite (parabolic cylinder) functions
Dv(z) (Bassom et al., 1995; Gromak, 1987; Lukashevich, 196713; Mu-
rata, 1985; Okamoto, 1986), for Pv they are expressed in terms of
Whittaker functions M,,p(z), or equivalently confluent hypergeomet-
ric functions 1Fl(a; c; z) (Lukashevich, 1968; Gromak, 1976; Okamoto,
198713; Watanabe, 1995), and for PVI they are expressed in terms of
hypergeometric functions 2F1(a,
b;
c;
z)
(Fokas and Yortsos, 1981; Luka-
shevich and Yablonskii, 1967; Okamoto, 1987a); see also (Ablowitz and
Clarkson, 1991; Gromak, 197813; Gromak, 1999; Gromak and Lukashe-
vich, 1982; Tamizhmani et al., 2001). Some classical orthogonal poly-
nomials arise as particular cases of these special function solutions and
thus yield rational solutions of the associated Painlev6 equations, espe-
cially in the representation of rational solutions through determinants.

126
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
For PIII and Pv these are in terms of associated Laguerre polynomials
~~'(z) (Charles, 2002; Kajiwara and Masuda, 1999b; Masuda et al.,
2002; Noumi and Yamada, 1998b), for PIv in terms of Hermite polyno-
mials Hn(z) (Bassom et al., 1995; Kajiwara and Ohta, 1998; Murata,
1985; Okamoto, 1986), and for for PvI in terms of Jacobi polynomials
~p")(z) (Masuda, 2002; Taneda, 2001b). In fact all rational solutions
of PvI arise as particular cases of the special solutions given in terms of
hypergeometric functions (Mazzocco, 2001).
This paper is organised as follows. The Yablonskii-Vorob'ev poly-
nomials and rational solutions for PII are studied in 92. We compare
the properties of these special polynomials with properties of classical
orthogonal polynomials. The analogous special polynomials associated
with rational solutions of PIII, which occur in the generic case when
76
#
0, are studied in $3. Further, in 93 we study the special polynomi-
als associated with algebraic solutions of
PIII,
which occur in the cases
when either
y
=
0 and
a6
#
0, or
6
=
0 and
py
#
0. In 94 the special
polynomials associated with rational solutions for PIv. Here there are
four types of special polynomials, two classes of Okamoto polynomials,
which were introduced by Okamoto (Okamoto, 1986), generalized Her-
mite polynomials and generalized Okamoto polynomials, both of which
were introduced by Noumo and Yamada (Noumi and Yamada, 1999).
Finally in 95 we discuss our results and pose some open questions.
2.
Second Painlev6 equation
2.1
Rational solutions of PII
The rational solutions of PII (1.1) are summarized in the following
Theorem due to Vorob'ev (Vorob'ev, 1965) and Yablonskii (Yablonskii,
1959); see also (Fukutani et al., 2000; Umemura, 1998; Umemura and
Watanabe, 1997; Taneda, 2000).
Theorem
2.1.
Rational solutions of
PII
exist
if
and only
if
a
=
n
E
Z,
which are unique, and have the form
for
n
2
1,
where the polynomials
Qn(z)
satisfy the d.i,terentiaGdifference
equation
Qn+~Qn-l=
ZQ~
-
4
[Q~Q:
-
(~b)~]
9
(2.2)
with
Qo(z)
=
1
and
Ql(z)
=
z.
The other rational solutions are given
by
W(Z;
0)
=
0, w(z; -n)
=
-w(z;
n).
(2.3)

Painleve' Equations and Associated Polynomials
127
The polynomials Q, (z) are monic polynomials of degree $n(n+
1)
with
integer coefficients, and are called the
Yablonskii- Vorob'ev polynomials.
The first few polynomials Q,(z) are
Remarks
2.2.
1. The hierarchy of rational solutions for
PII
given by (2.1) can also
be derived using the Backlund transformation of
PII
(Lukashevich, 1971), with "seed solution" wo
=
w(z; 0)
=
0.
2. It is clear from the recurrence relation (2.2) that the Q,(z) are
rational functions, though it is not obvious that in fact they are
polynomials since one is dividing by Q,-l(z) at every iteration.
Indeed it is somewhat remarkable that the Qn(z) defined by (2.2)
are polynomials.
3.
Letting Q, (z)
=
C~T,
(z) exp(z3/24), with
c,
=
(2i),(,+l), in (2.2)
yields the Toda equation
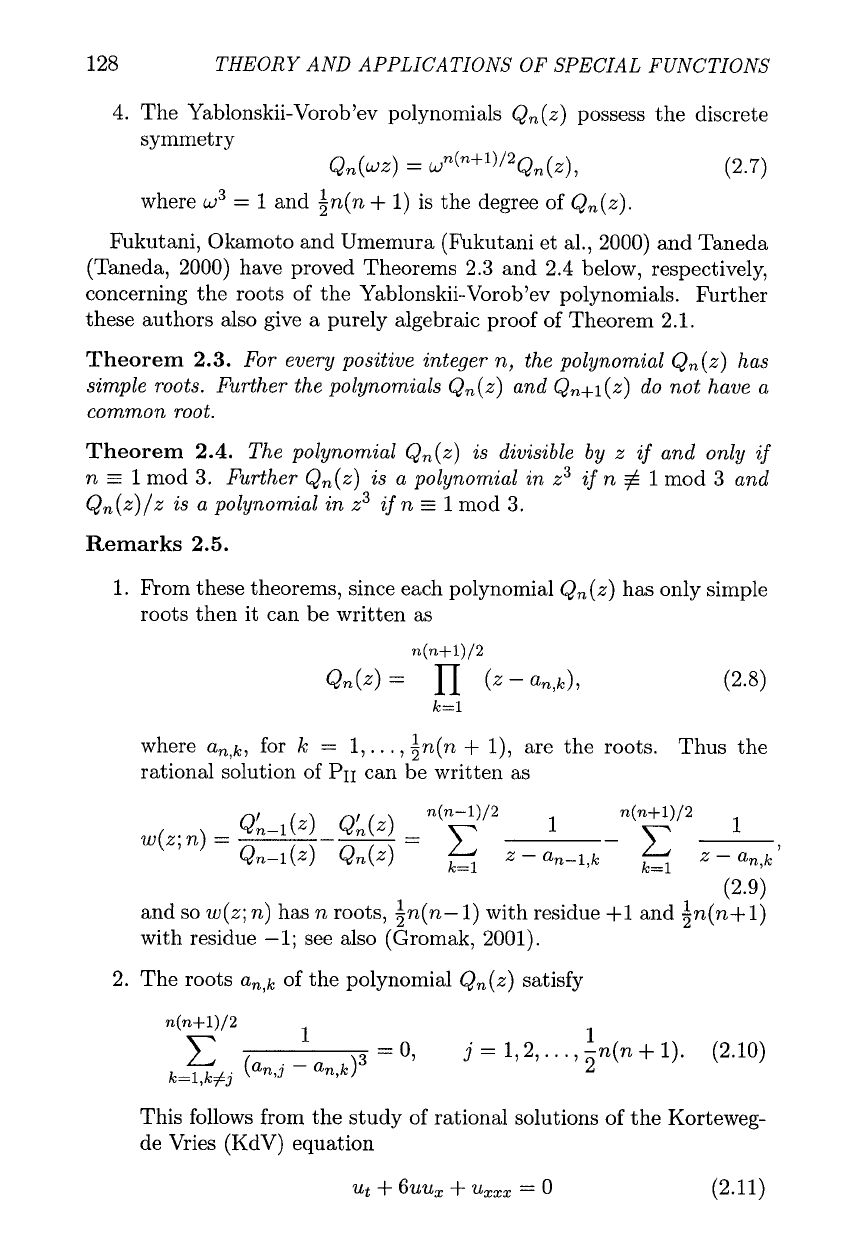
128
THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS
4.
The Yablonskii-Vorob'ev polynomials Qn(z) possess the discrete
symmetry
Qn(wz)
=
w
n(n+1)/2
Qn
(2)
7
(2-7)
where
u3
=
1
and fn(n
+
1)
is the degree of Qn(z).
Fukutani, Okamoto and Umemura (Fukutani et al., 2000) and Taneda
(Taneda, 2000) have proved Theorems 2.3 and 2.4 below, respectively,
concerning the roots of the Yablonskii-Vorob'ev polynomials. Further
these authors also give a purely algebraic proof of Theorem 2.1.
Theorem
2.3.
For every positive integer
n,
the polynomial
Qn(z)
has
simple roots. Further the polynomials
Qn(z)
and
Qn+l(z)
do not have a
common root.
Theorem
2.4.
The polynomial
Qn(z)
is divisible by
z
if
and only
if
n
-
1
mod 3.
Further
Qn(z)
is a polynomial
in
z3
if
n
$
1
mod
3
and
Qn(z)/z
is a polynomial
in
z3
if
n
-
1
mod 3.
Remarks
2.5.
1.
From these theorems, since each polynomial Qn(z) has only simple
roots then it can be written
as
where an,k, for
k
=
1,
.
. .
,
&n(n
+
I), are the roots.
Thus the
rational solution of
PII
can be written
as
(2.9)
and so
w
(z; n) has
n
roots, $n(n- 1) with residue
+1
and
f
n(n+
1)
with residue
-1;
see also (Gromak, 2001).
2. The roots an,k of the polynomial Qn(z) satisfy
This follows from the study of rational solutions of the Korteweg-
de Vries (KdV) equation
