Ismail M., Koelink E. (editors) Theory and Applications of Special Functions

Подождите немного. Документ загружается.

Painleve' Equations and Associated Polynomials

139

the affine Weyl group

21,

which also is the transformation group for

PII

(Okamoto, 1986; Umemura, 2000; Umemura and Watanabe, 1997).

Ohyama (Ohyama, 2001) derived special polynomials associated with

the rational solutions of (3.12). These are essentially described in The-

orem

3.5

below, though here the variables have been scaled and the

expression of the rational solutions of (3.12) in terms of these special

polynomials is explicitly given.

Theorem

3.5.

Suppose that Rn(<) satisfies the recursion relation (Toda

equation)

with Ro(<)

and R1

(<)

c2. Then

un(O

&+I

(C)

&-I(<)

R%)

satisfies (3.12) with

Additionally u-,(<)

-iu,(i<)

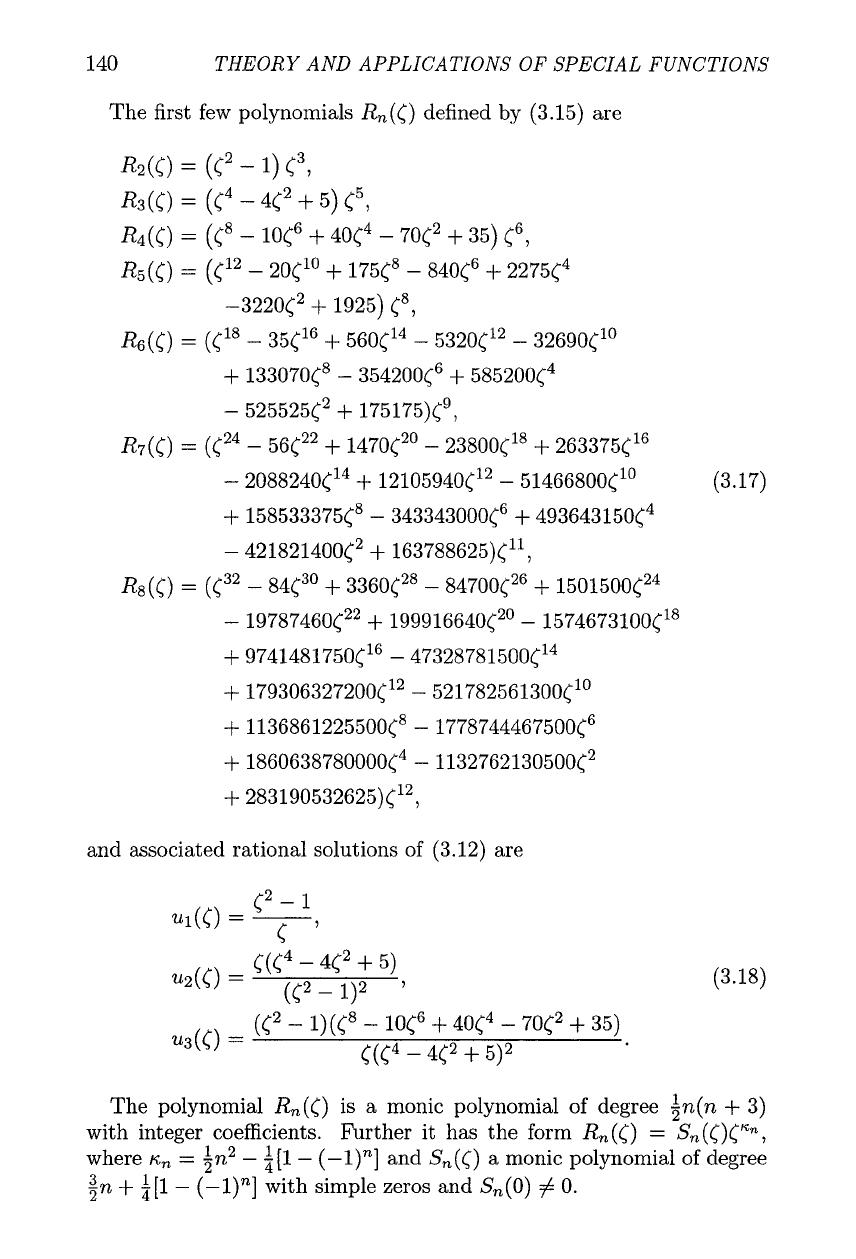
140

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

The first few polynomials Rn(c) defined by (3.15) are

and associated rational solutions of (3.12) are

The polynomial

&(c)

is a monic polynomial of degree in(n

with integer coefficients. Further it has the form Rn(<)

Sn(c)CKn,

where

ten

in2

[l-

(-l)n]

and

Sn(C)

a monic polynomial of degree

$[l

(-l)n] with simple zeros and S,(O)

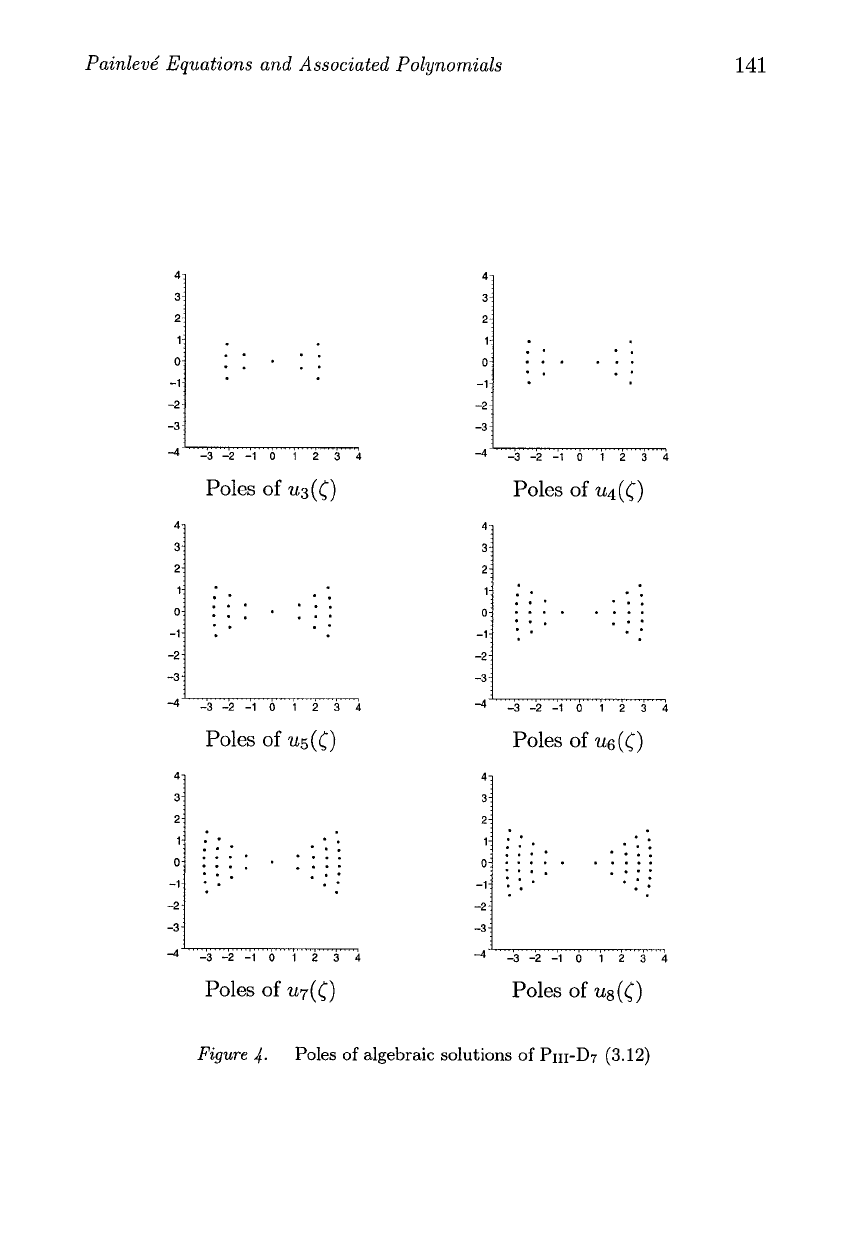
Painleve' Equations and Associated Polynomials

Poles of

us(<)

Poles of

us(<)

Poles of

245)

Poles of

u4(<)

Poles of

us(<)

-4

-3-2-10

Poles of

u8(<)

Figure

Poles of algebraic solutions of

PIII-D7

(3.12)

142

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

In Figure 4 plots of the locations of the poles of the algebraic solutions

of PIII-D7 (3.12) given

un(z), for

3,4,

,8, as defined in (3.18)

are given. These take the form of two "triangles" in a "bow-tie" shape.

Fourth Painlev6 equation

4.1

Rational solutions of PIv

Lukashevich (Lukashevich, 196713)) Gromak (Gromak, 1987) and Mu-

rata (Murata, 1985) (see also (Bassom et al., 1995; Gromak et al.,

2002; Umemura and Watanabe, 1997))) have proved the following theo-

rem

Theorem

4.1.

PIv

has rational solutions

and only

with

Further the rational solutions for these parameter values

are unique.

Three simple rational solutions of PIv are

w1(2;

2, -2)

l/z, w2(2; 0, -2)

-22, w3

(2;o,

-;)

32.

(4.3)

It is known that there are three families of unique rational solutions of

PIv, which have the solutions (4.3) as the simplest members. These are

summarized in the following theorem (see (Bassom et al., 1995; Murata,

1985; Umemura and Watanabe, 1997) for further details).

Theorem

4.2.

There are three families of rational solutions of

PIv,

which have the forms

Painleve' Equations and Associated Polynomials

143

where

Pj,n(z)

and

Qj,n(2),

1,2,3,

are polynomials of degree

and

The three hierarchies given in this theorem are known as the "-l/z

hierarchy", the "-22 hierarchy" and the

"-$2

hierarchy", respectively

(see (Bassom et al., 1995) where the terminology was introduced). The

"-l/z hierarchy" and the "-22 hierarchy" form the set of rational so-

lutions of

PIv

with parameter values given by (4.1) and the "-$2 hier-

archy" forms the set with parameter values given by (4.2). The rational

solutions of

PIv

with parameter values given by (4.1) lie at the vertexes

of the "Weyl chambers" and those with parameter values given by (4.2)

lie at the vertexes of the "Weyl chamber" (Umemura and Watanabe,

1997).

4.2

Okamoto polynomials

In a comprehensive study of the fourth Painlev6 equation

PIv,

Okamoto

(Okamoto, 1986), see also (Kajiwara and Ohta, 1998; Noumi and Ya-

mada, 1999; Umemura, 1998) defined two sets of polynomials analogous

to the Yablonskii-Vorob'ev polynomials associated with

PII.

These poly-

nomials are defined in Theorems 4.3 and 4.5 below, where they have been

scaled compared to Okamoto's original definition, where the polynomials

were monic, so that they are for the standard version of PIv.

Theorem

4.3.

Suppose that

Qn(z)

satisfies the recursion relation

with

Qo(z)

Ql (2)

Then

w(2;a,,Pn)

-z+

{ln

[Q;;;;;)]

)

(4.7)

3 dz

satisfies

PIv

with

(an,

,&)

(271,

-2).

144

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

The first few polynomials Q,(z), which are referred to as the Okamoto

polynomials, are

Remarks

4.4.

The polynomials Q, (z) are polynomials of degree

n(n

I),

in fact

they are monic polynomials in

fiz with integer coefficients,

which is the form in which Okamoto (Okamoto, 1986) originally

defined these polynomials.

2. The hierarchy of rational solutions of

PIv

defined by (4.7) can be

derived using the following Backlund transformation of

PIv

(w'

2zw)

z;&,p

( -)

2w[w1-w2-2zw+2(a+l)]' (4.8)

&=a++,

p=p,

where w

w(z;

p), which is the Backlund transformation

I+

derived by Murata (Murata, 1985) and the Schlesinger transfor-

mation

7d51

derived by Fokas, Mugan and Ablowitz (Fokas et al.,

Painleve' Equations and Associated Polynomials

1988). Specifically

where w,

(2;

2n,

-a),

with "seed solution"

w (z; 0,

-$)

-";.

The solutions w, are members of the so-called

"-$2"

hierarchy of

rational solutions of PIv, recall Theorem 4.2, which is one of three

hierarchies of rational solutions of PIv (see, for example, (Bassom

et al., 1995; Murata, 1985) for further details).

The second set of polynomials introduced by Okamoto (Okamoto,

1986) are defined in the following theorem.

Theorem

4.5.

Suppose that

S,(z)

satisfies the recursion relation

with

So(z)

and

Sl(z)

fiz.

Then

(z;

a,,

p,)

--z

(4.11)

for

satisfies

PIv

with

(a,,

(2n

-9).

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

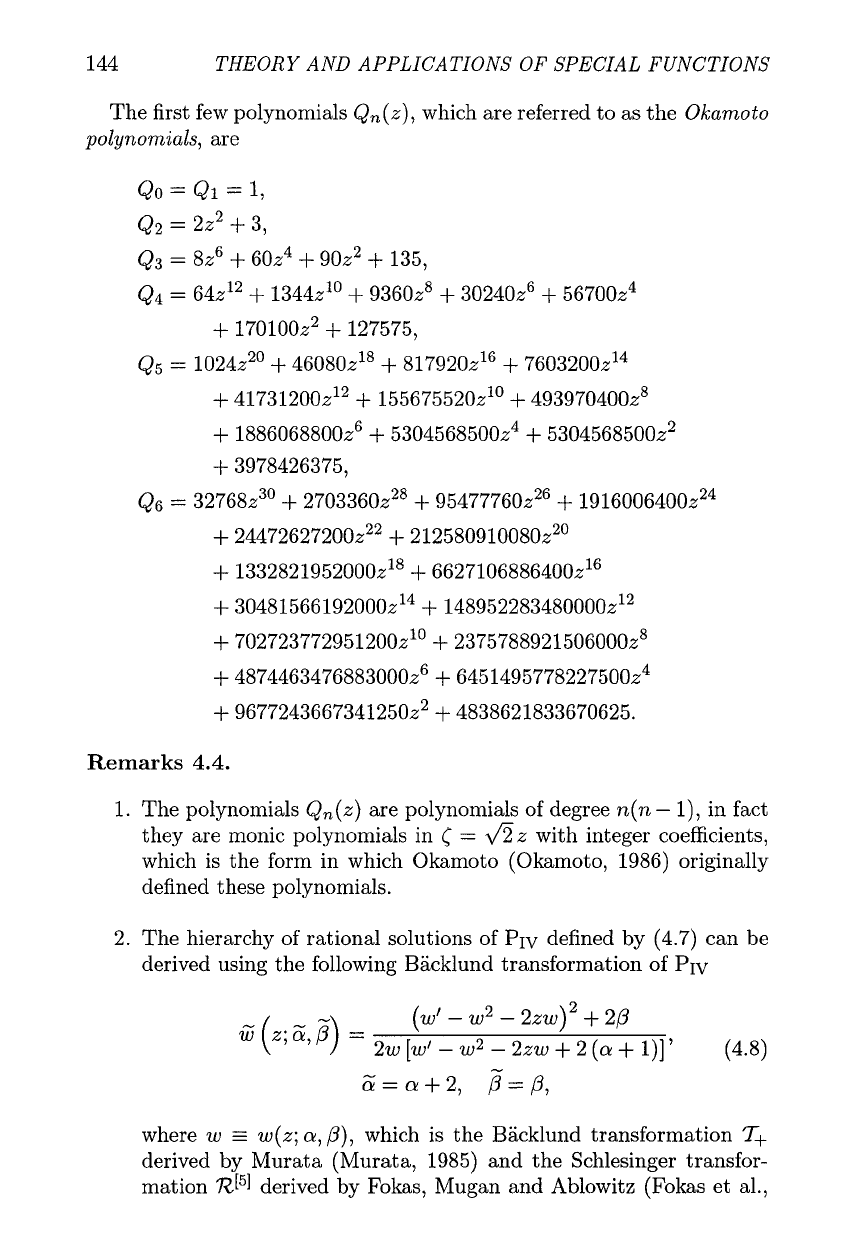
Roots of

Qg (z)

Roots of

Q6(z)

Roots of

Q4(z)

ahii

2-8

Roots of

Q8(z)

Figure

bots of the Okamoto polynomials

defined by

(4.6)

Painleve' Equations and Associated Polynomials

The first few polynomials Sn(z), are

Remarks

4.6.

1. The polynomials Sn(z) are polynomials of degree n2, in fact they

are monic polynomials in

22 with integer coefficients, which

is the form in which Okamoto (Okamoto, 1986) originally defined

these polynomials.

2. The hierarchy of rational solutions of

PIv

defined by (4.11) can be

derived using the Backlund transformation (4.8) of PIv, derived

by Murata (Murata, 1985) and Fokas, Mugan and Ablowitz (Fokas

et al., 1988). Hence

where Gn

(z; 2n

1,2n

g),

with "seed solution"

(z;

-!)

-3.2

l/z.

148

THEORY AND APPLICATIONS OF SPECIAL FUNCTIONS

The solutions

67,

are also members of the so-called "-Zz" hierar-

chy of rational solutions of PIv, recall Theorem 4.2.

4. The two hierarchies of rational solutions of

PIv

given by (4.7) and

(4.11) are linked by the Schlesinger transformations and

%d31

for PIv given by Fokas, Mugan and Ablowitz (Fokas et al., 1988).

where w

w(z;

p),

w[jl

w (z; abl, pb]). Specifically, for n

(wk

a)2

w: [8n

(wn

2~)~]

(4.15)

2wn (wi

2zwn

w:,

5. The Schlesinger transformations

R['],

R[~]

and

7?f51

are related by

R[~]R[~]

R[~]R[']

~1~1,

from the definition given by Fokas,

Mugan and Ablowitz (Fokas et al., 1988).

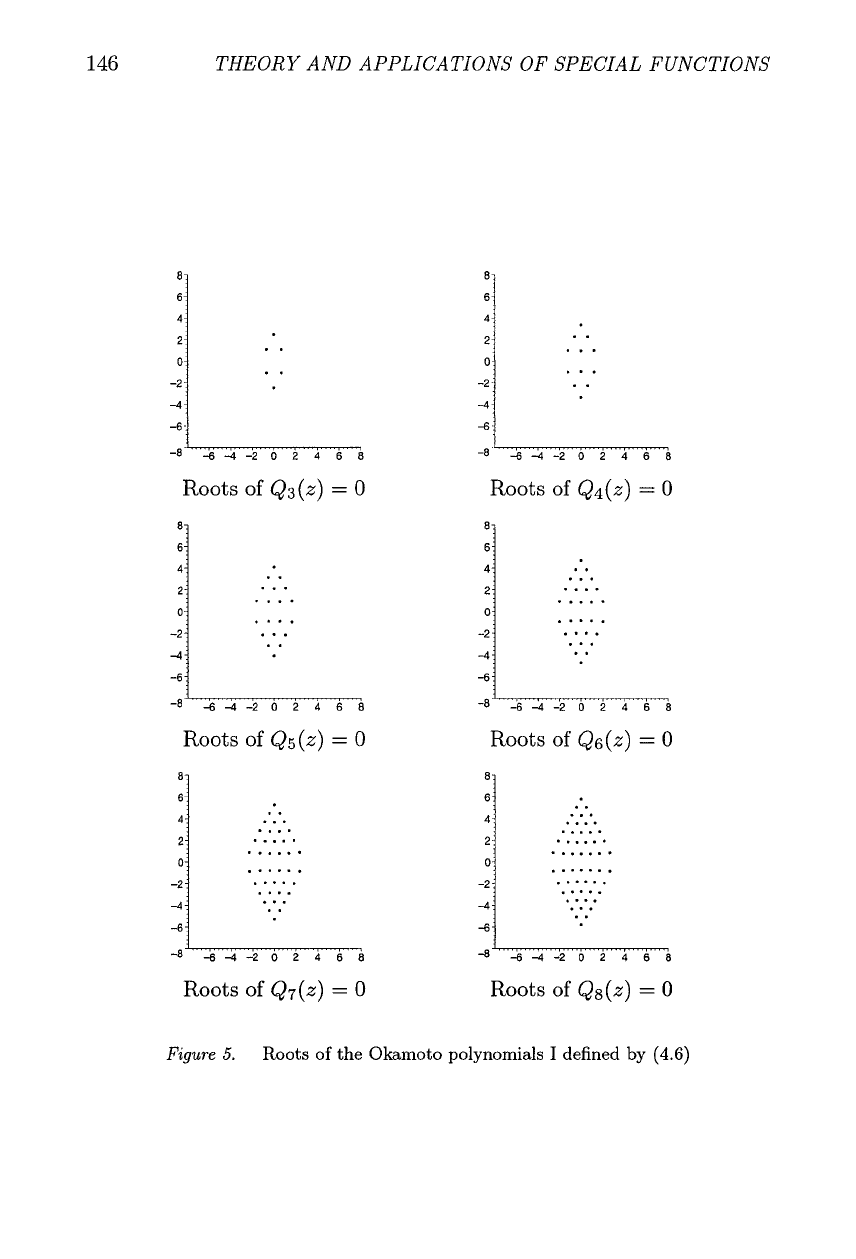
In Figures 5 and 6 plots of the locations of the roots for the Okamoto

polynomials Qn(z)

0, defined by (4.6)) and Sn(z)

0, defined by

(4.10)) for

3,4,.

. .

,8, respectively, are given. These both take the

form of two "triangles" with the polynomials

h(z)

having an additional

row of roots on

straight line between the two "triangles."

4.3

Generalized Hermite polynomials

Noumi and Yamada (Noumi and Yamada, 1999) generalized the re-

sults of Okamoto (Okamoto, 1986) and introduced the generalized Her-

mite polynomials Hm,,(z)) which are defined in Theorem 4.7 and dis-

cussed below in this section, and generalized Okamoto polynomials Qm,,(z),