Jones M., Fleming S.A. Organic Chemistry

Подождите немного. Документ загружается.

8.10 Summary 359

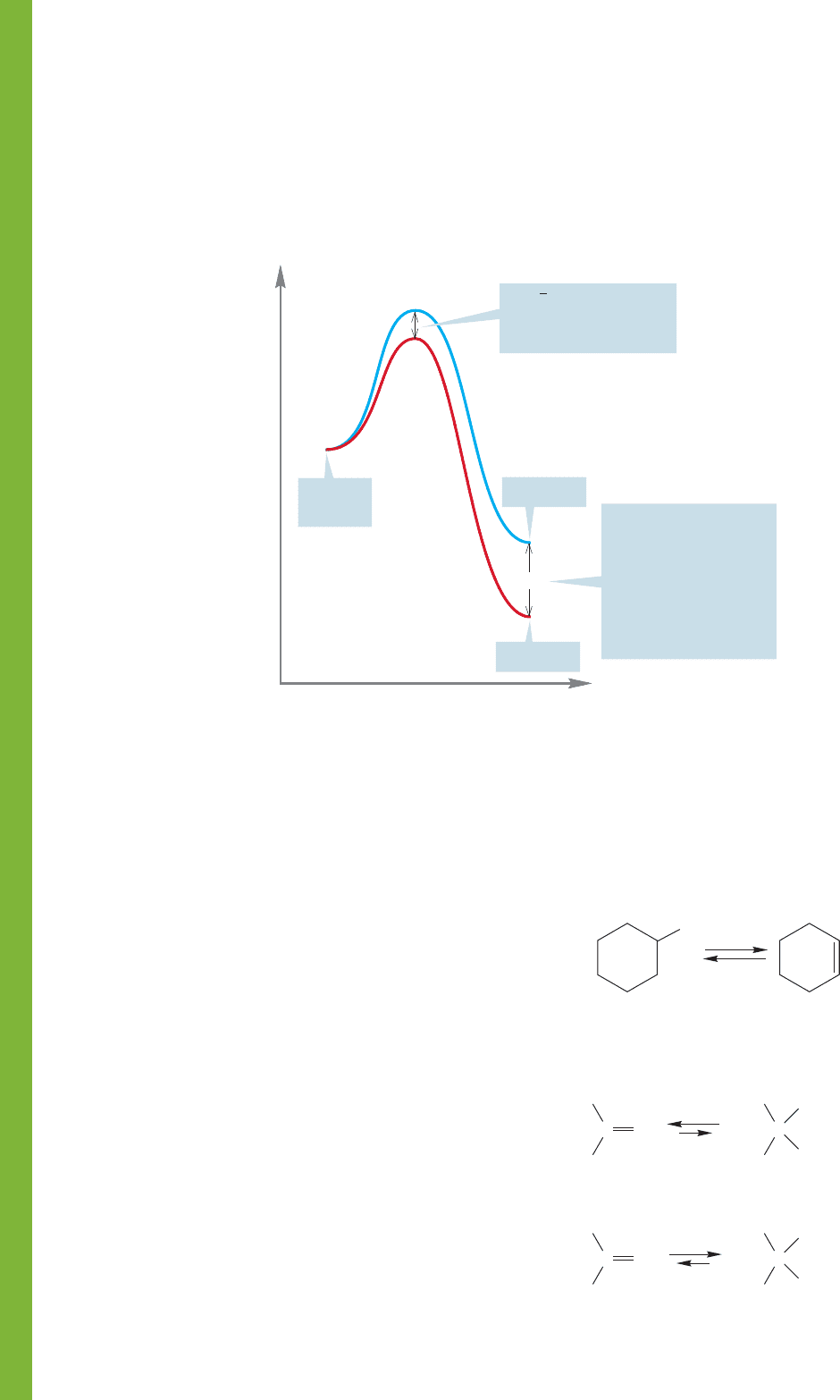
FIGURE 8.36 Kinetic control of product distribution.

Key Terms

Boltzmann distribution (p. 340)

endergonic (p. 335)

entropy change (ΔS°) (p. 335)

equilibrium constant (K ) (p. 334)

exergonic (p. 335)

first-order reaction (p. 340)

Gibbs free energy change (ΔG°) (p. 335)

Hammond postulate (p. 355)

kinetic control (p. 353)

kinetics (p. 351)

Le Châtelier’s principle (p. 336)

microscopic reversibility (p. 346)

pseudo-first-order reaction (p. 340)

rate constant (k) (p. 341)

second-order reaction (p. 340)

thermodynamic control (p. 353)

thermodynamics (p. 351)

Energy

Reaction

ress

Starting

material

Product X

Product Y

⌬G⬚

ΔΔG

, the energy difference between

the two transition states; if X and Y

cannot revert to starting material, it is

this difference that solely determines

the relative amounts of products

ΔG⬚ is the energy difference

between the products; this

energy can have no effect

on the product distribution

unless X and Y can return to

starting material; if not, it will

be the difference between the

two activation energies that

solely influences the product

distribution

†

Therefore, a small amount of energy difference (ΔG°) has a

great influence on the equilibrium constant K.

The ratios of products formed in a reaction depend either

on the energy difference between the products ΔG° (thermo-

dynamic control) or on the relative heights of the transition

states leading to products (kinetic control).

Differences in G°, the Gibbs free energy, whether they

are ΔG°, the difference in energy between starting material

and product, or ΔG

‡

, the difference in energy between

starting material and the transition state (activation energy),

are made up of entropy (ΔS°) and enthalpy (ΔH°) terms

(ΔG°  ΔH°  T ΔS° or ΔG

‡

 ΔH

‡

 T ΔS

‡

The rate of a reaction depends on temperature, the

concentration of the reactant(s), and the rate constant k for

the reaction. The rate constant is a property of the reaction

and depends on temperature, pressure, and solvent, but not

on the concentrations of the reactants.

The rate-determining step of a reaction is the step with the

highest-energy transition state. It may or may not be the same

as the product-determining step. For example, in the S

reaction it is the same, but in the S

1 reaction it is not.

The principle of microscopic reversibility says that the

lowest-energy path in one direction of an equilibrium reaction

is the lowest-energy path for the reverse reaction.

The Hammond postulate says that the transition state for

an endothermic reaction resembles the product, or, equivalently,

that the transition state for an exothermic reaction resembles

the starting material.

The most common problem experienced with the material in this

chapter is the confusion between thermodynamics and kinetics.

Rates of reactions (kinetics) are determined by the relative

heights of the available transition states leading to products.

If there is insufficient energy available for the products to

re-form starting materials, it will be the relative heights of the

transition states that determine the mixture of products, no mat-

ter what the energy differences among those products (Fig. 8.36).

Common Errors

360 CHAPTER 8 Equilibria

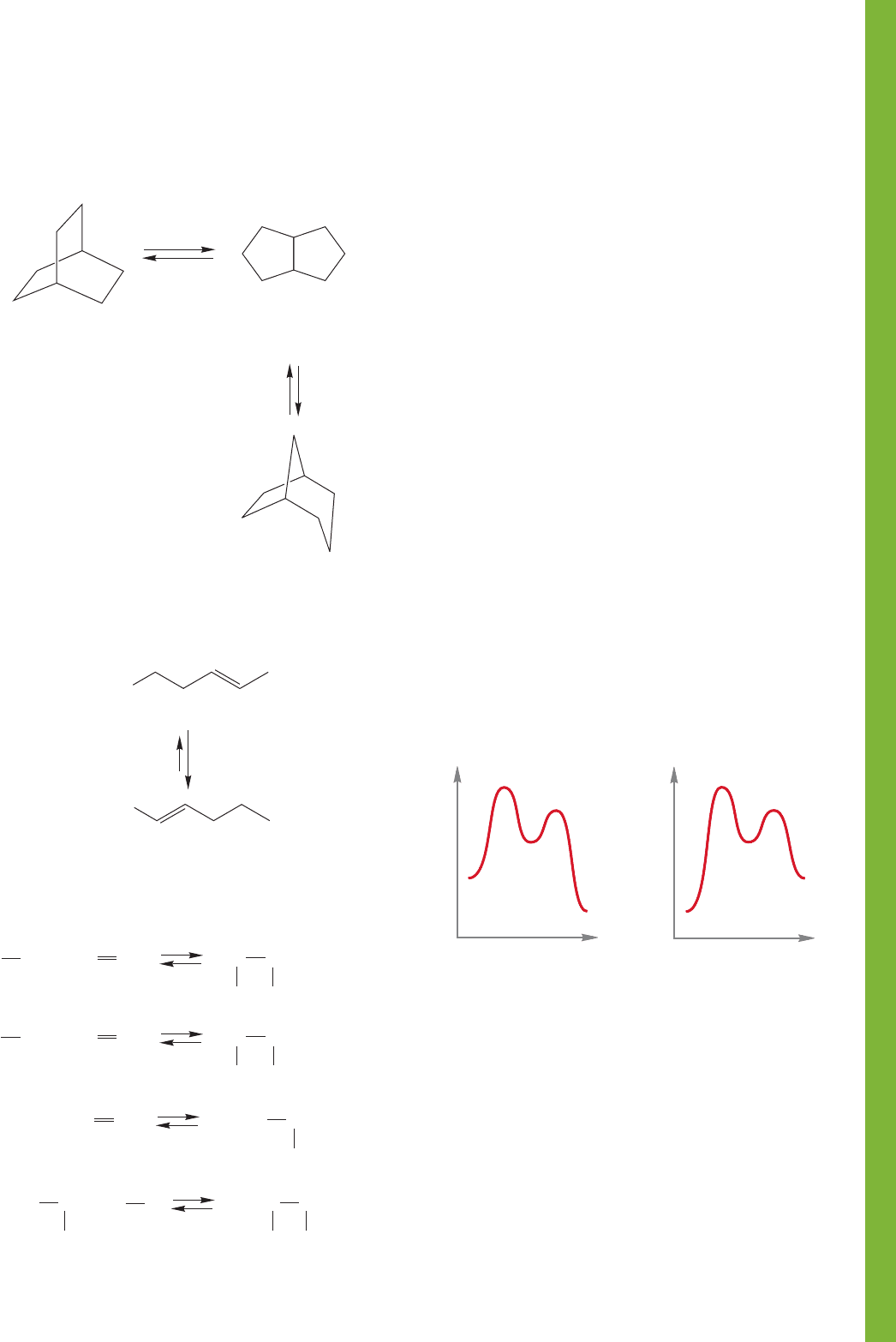
Energy

Reaction

ress

Starting

material

Product X

Product Y

⌬G⬚

ΔΔG

, the energy

difference between the

two transition states

The energy difference

between the products;

if X and Y can

equilibrate by returning

to starting material, it

will be this ⌬G⬚

that

determines the relative

amounts of X and Y

†

FIGURE 8.37 Thermodynamic control of product distribution.

PROBLEM 8.11 The Finkelstein reaction:

X  Cl or Br

is often used to prepare alkyl iodides from alkyl chlorides or

bromides through an S

2 reaction.

(a) Suggest a simple way to drive this equilibrium toward the

desired iodide.

(b) In practice, the Finkelstein reaction is usually run in acetone

solvent, because sodium iodide, but not sodium chloride or

sodium bromide, is soluble in acetone. Explain carefully how

this difference in solubilities drives the equilibrium toward

the desired iodide.

PROBLEM 8.12 The alcohol dehydration and alkene hydration

shown below is an equilibrium process.

(a) Suggest experimental conditions that will favor cyclohexene.

X + NaI

I + NaX

(b) Suggest experimental conditions that will favor cyclohexanol.

PROBLEM 8.13 Calculate the energy differences between the

carbonyl ( ) compounds and their hydrates at 25 °C.

K = 2.8 ⳯10

(b)

(a)

K = 1.4 ⳯10

–

8.11 Additional Problems

On the other hand, if equilibrium is established, if the

products can revert to starting materials, it will be the relative

energies of the product molecules that determine how much of

each is formed (Fig. 8.37).

Remember also to be careful to distinguish between the rate of

a reaction and the rate constant for that reaction.The rate depends

on the concentration(s) of the reacting molecules; the rate con-

stant does not, and is a fundamental property of the reaction.

8.11 Additional Problems 361

PROBLEM 8.14

Under certain highly acidic conditions, the

three bicyclooctanes shown below can be isomerized. Given the

ratios at equilibrium (3.66 32.95 63.35), calculate the relative

energies of the three isomers at 25 °C.

PROBLEM 8.15 It is possible to equilibrate the following two

ethers. Compound B is favored over A by 1.51 kcal/mol. What

is the equilibrium constant for this interconversion at 25 °C?

PROBLEM 8.16 Use the BDE of Table 8.2 (p. 337) to estimate

ΔH° for the following reactions. Reaction mechanisms are not

necessary!

(a)

CCH

(b)

CCH

(c)

(CH

)

CI CH

(CH

)

CCH

(d)

HCl ClCH

(CH

)

C (CH

)

CCH

Fe(CO)

Bicyclo[2.2.2]octane

(3.66%)

Bicyclo[3.3.0]octane

(32.95%)

Bicyclo[3.2.1]octane

(63.35%)

AlBr

25 ⬚C

AlBr

25 ⬚C

PROBLEM 8.17 Use the data in Table 8.2 (p. 337) to design an

endothermic reaction. Don’t worry about mechanism! This

problem does not ask you to design a reasonable reaction—it

merely asks you to find reactions that would be endothermic if

they occurred.

PROBLEM 8.18 For the equilibrium , calculate:

(a) ΔG° at 25 and 200 °C given ΔH° 14.0 kcal/mol and

ΔS° 15.8 cal/deg

mol.

(b) ΔG° at 25 and 200 °C given ΔH° 6.0 kcal/mol and

ΔS° 15.8 cal/deg

mol.

PROBLEM 8.19 Draw the complete reaction mechanism for

the E1 and S

1 reactions of tert-butyl alcohol with a catalytic

amount of H

and solvent ethanol.

PROBLEM 8.20 Because the E1 and S

1 reactions are

reversible, the selection between the two can often be driven

by increasing the temperature. In this fashion the more stable

product is favored. Use data in Table 8.2 to choose the more

stable products in the reaction of tert-butyl alcohol in catalytic

and ethanol.

PROBLEM 8.21 Draw Energy versus Reaction progress dia-

grams for E1 and E2 reactions that are overall (a) exothermic,

(b) endothermic. Use a tertiary iodide as a sample substrate

molecule.

PROBLEM 8.22 For the following two reactions, described

by the two Energy versus Reaction progress diagrams,

(1) and (2):

(a) In each case, which compounds will be present at the end

of the reaction?

(b) In each case, which compound will be present in the largest

amount? Which will be present in the smallest amount?

reaction in each case?

(d) Use the diagrams to point out in each case the activation

energy for the following reactions:

AB, AC, CB, and CA

(e) In each case, what is the rate-determining step for the

reaction AC?

UUUU

Energy

Reaction progress

(1)

(2)

Energy

Reaction progress

A + B

362 CHAPTER 8 Equilibria

PROBLEM 8.23 Explain in painstaking detail why one is

justified in saying, “The reaction of 2-methyl-2-butene

with HCl leads to the tertiary chloride because a tertiary

carbocation is more stable than a secondary carbocation.”

You might start with the construction of Energy versus

Reaction progress diagrams and good drawings for the

transition states.

PROBLEM 8.24 Find the errors in each diagram.

Energy

Reaction progress

(a)

Energy

Reaction progress

(b)

Use Organic Reaction Animations (ORA) to answer the

following questions:

PROBLEM 8.25 Select the animation titled “Unimolecular elimi-

nation: E1” and observe the energy diagram. Do you suppose this

reaction is reversible? Use the energy diagram to answer this ques-

tion. Use the nature of the products to answer the same question.

PROBLEM 8.26 Compare the E1 reaction with the “Bimolecular

elimination: E2” in terms of the energy diagram. Do you sup-

pose the E2 reaction is more or less reversible than the E1

reaction? Use the energy diagram to answer this question. Use

the nature of the products to answer the same question.

PROBLEM 8.27 If you needed to make an alkene, would you

prefer to use the E1 reaction or the E2 reaction? Why?

PROBLEM 8.28 Observe the “Hofmann elimination” reaction.

Draw the energy diagram shown for the reaction. Draw a new

reaction curve for the same reaction run in a more polar solvent.

Draw the curve for a reaction run in a less polar solvent. Which

solvent would likely give the faster Hofmann elimination?

Additions to Alkenes 1

363

9.1 Preview

9.2 Mechanism of the Addition of

Hydrogen Halides to Alkenes

9.3 Effects of Resonance on

Regiochemistry

9.4 Brief Review of Resonance

9.5 Resonance and the Stability of

Carbocations

9.6 Inductive Effects on Addition

Reactions

9.7 Addition Reactions:

Hydration

9.8 Dimerization and

Polymerization of Alkenes

9.9 Rearrangements during

Addition to Alkenes

9.10 Hydroboration

9.11 Hydroboration in Synthesis:

Alcohol Formation

9.12 Special Topic: Rearrangements

in Biological Processes

9.13 Summary

9.14 Additional Problems

TWO PATHS Depending on the nature of the alkene, addition of an electrophile to

the alkene can follow more than one path.The path taken and the final product

depend on the nature of the starting alkene and the reagents encountered along

the way.

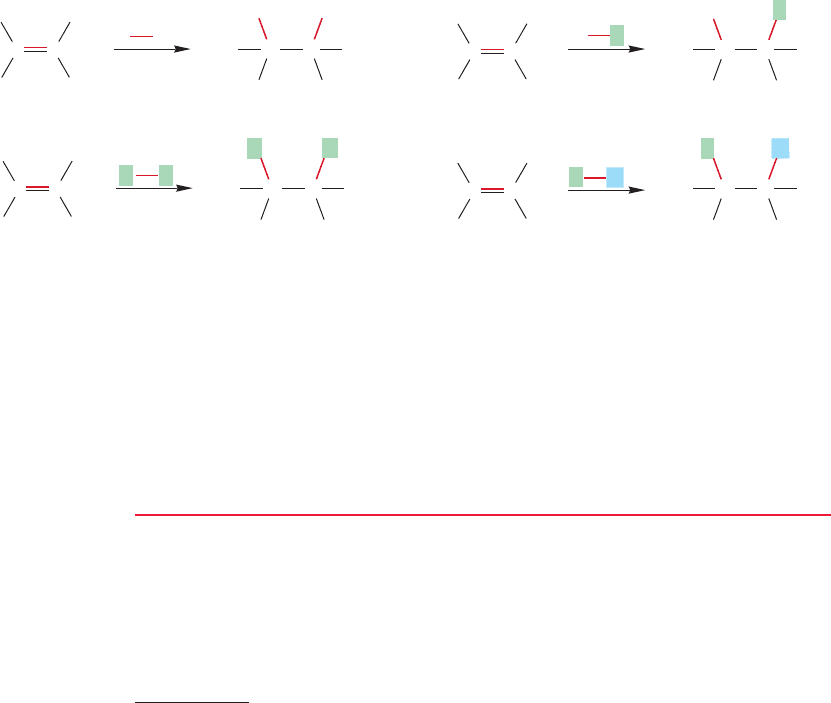
364 CHAPTER 9 Additions to Alkenes 1

HBr

C C

H X

C C

FIGURE 9.1 A variety of additions to alkenes. Many , X

, and reagents undergo the addition reaction.XYHX

In the last third of his life, there came over Laszlo Jamf—so it seemed to

those who from out in the wood lecture halls watched his eyes slowly

granulate, spots and wrinkles grow across his image, disintegrating it

towards old age—a hostility, a strangely

personal

hatred, for the covalent

bond.

—THOMAS PYNCHON,

GRAVITY’S RAINBOW

9.1 Preview

In this chapter, we revisit one of the building-block reactions of organic chemistry—

addition to alkenes. We first saw this useful process in Chapter 3, where we used

it to introduce reactivity. Here and in Chapter 10, we review very briefly the

material from Chapter 3 and then expand on the addition process, using the style of

theme and variations. By all means, go back and reread the material in Chapter 3

(pp. 132–139) if this introduction is not completely obvious to you.

In Chapter 3, we restricted ourselves to a few examples of the addition of HX

reagents to alkenes. Now we expand to a variety of old and new HX reagents, and

in Chapter 10, we add X

and XY reagents (Fig. 9.1). Our growing catalog of reac-

tions will continue to add to your expertise in synthesis.

ESSENTIAL SKILLS AND DETAILS

1. Many addition reactions, especially HX additions, proceed through formation of a

carbocation that is captured in a second step by X



. You must be able to analyze the

possibilities for cation formation and pick out the most stable one possible. In practice,

this task is not especially difficult.

Thomas Pynchon is an American author born in 1937.

The kinds of molecules we know how to make will increase sharply as a result

of material in Chapters 9 and 10. Addition of hydrogen bromide and hydrogen

chloride to alkenes provides access to alkyl halides. We already have the S

1 and

2 reactions available for transforming the alkyl halides into other compounds

(Fig. 7.94, p. 312).

9.2 Mechanism of the Addition of Hydrogen Halides to Alkenes 365

One of the important factors influencing cation stability is delocalization of

charge through the phenomenon we call resonance. Writing resonance forms

well is an essential skill, and this chapter provides a review of how to do it

properly.

3. Hydration and hydroboration/oxidation give you the ability to add water across an

unsymmetrical double bond in either a Markovnikov or an anti-Markovnikov

fashion, respectively. Although the mechanistic details of the hydroboration process

are complex, the synthetic outcome is decidedly not. Be sure you know how to use

these reactions to good effect in making alcohols.

4. Less stable carbocations rearrange to more stable ones through hydride (H:



) shifts.

Stay alert—from now on, every time you see a carbocation, you have to consider

whether it will rearrange into a lower-energy carbocation. Once again, you have to

be able to judge and predict stability.

2,3-Dimethyl-2-butene 2-Chloro-2,3-dimethylbutane

C C

–

Protonation

Addition of Cl

–

WEB 3D

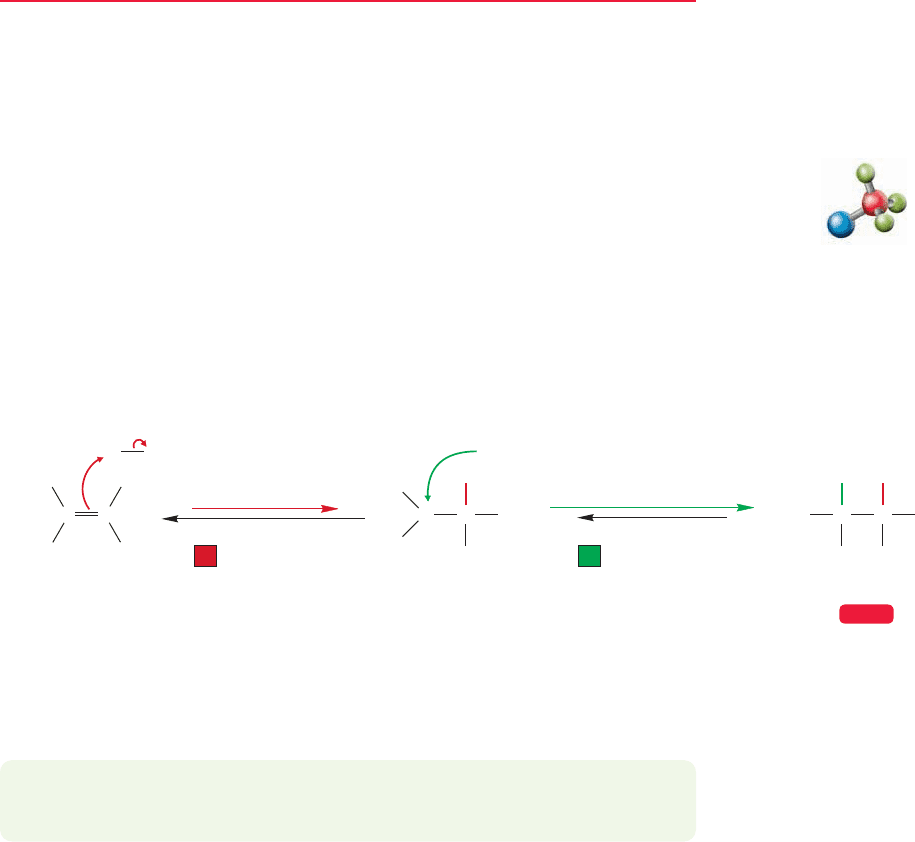
FIGURE 9.2 The first step in this two-step reaction is the protonation of the alkene to give a carbocation. In the

second step, a chloride ion adds to the cation to give the final product.

PROBLEM 9.1 Use the bond dissociation energies of Table 8.2 (p. 337) to estimate

the exothermicity or endothermicity of the reaction in Figure 9.2.

Alkene hydrohalogenation

9.2 Mechanism of the Addition of Hydrogen

Halides to Alkenes

Consider once again the reaction of 2,3-dimethyl-2-butene with hydrogen chlo-

ride to give 2-chloro-2,3-dimethylbutane (Fig. 9.2). This process is called an

alkene hydrohalogenation because it is an addition of hydrogen and halogen

across a π bond. The arrow formalism maps out the process for us and develops

the picture of a two-step mechanism. In the first step, the alkene, with the filled

π orbital acting as base, is protonated by hydrogen chloride to give a carbocation

and a chloride ion. In the second step, chloride acts as a nucleophile and adds to

the strongly Lewis acidic cation to give the product.

The protonation of the alkene to give a carbocation is the slow step in the

reaction. The rate-determining step, the one with the highest-energy transition

state, is this addition of a proton. Because this step is endothermic, the transition

366 CHAPTER 9 Additions to Alkenes 1

Reaction

ress

Energy

⌬G

–

The activation energy for

the first, and rate-determining,

step of the addition

Transition state for

the second step

Transition state

for the first step

–

†

FIGURE 9.3 In the addition of

hydrogen chloride to an alkene, the

first step, the endothermic formation

of a carbocation, is the slow, rate-

determining step. In the transition

state for this step, the positive charge

accumulates on one of the carbons of

the starting alkene.

state will resemble the carbocation intermediate, and it will have substantial

positive charge developed on carbon (Fig. 9.3). That notion leads directly to the

next topic.

C C

–

ClH

FIGURE 9.4 For an unsymmetrical

alkene, there are two possible

intermediate cations, and therefore

two possible chloride products.

9.3 Effects of Resonance on Regiochemistry

The reaction of hydrogen chloride with a symmetrically substituted alkene such as

2,3-dimethyl-2-butene is relatively simple.Protonation is followed by chloride addi-

tion to give the alkyl chloride. There are no choices to be made about the position

of protonation, and once the carbocation is made, chloride formation is easy to

rationalize. What happens if the ends of the alkene are differently substituted? In

such an instance, two products are possible (Fig. 9.4). We discussed this question

briefly in Chapter 3 (p. 137), and the answer is that it depends on how the ends of

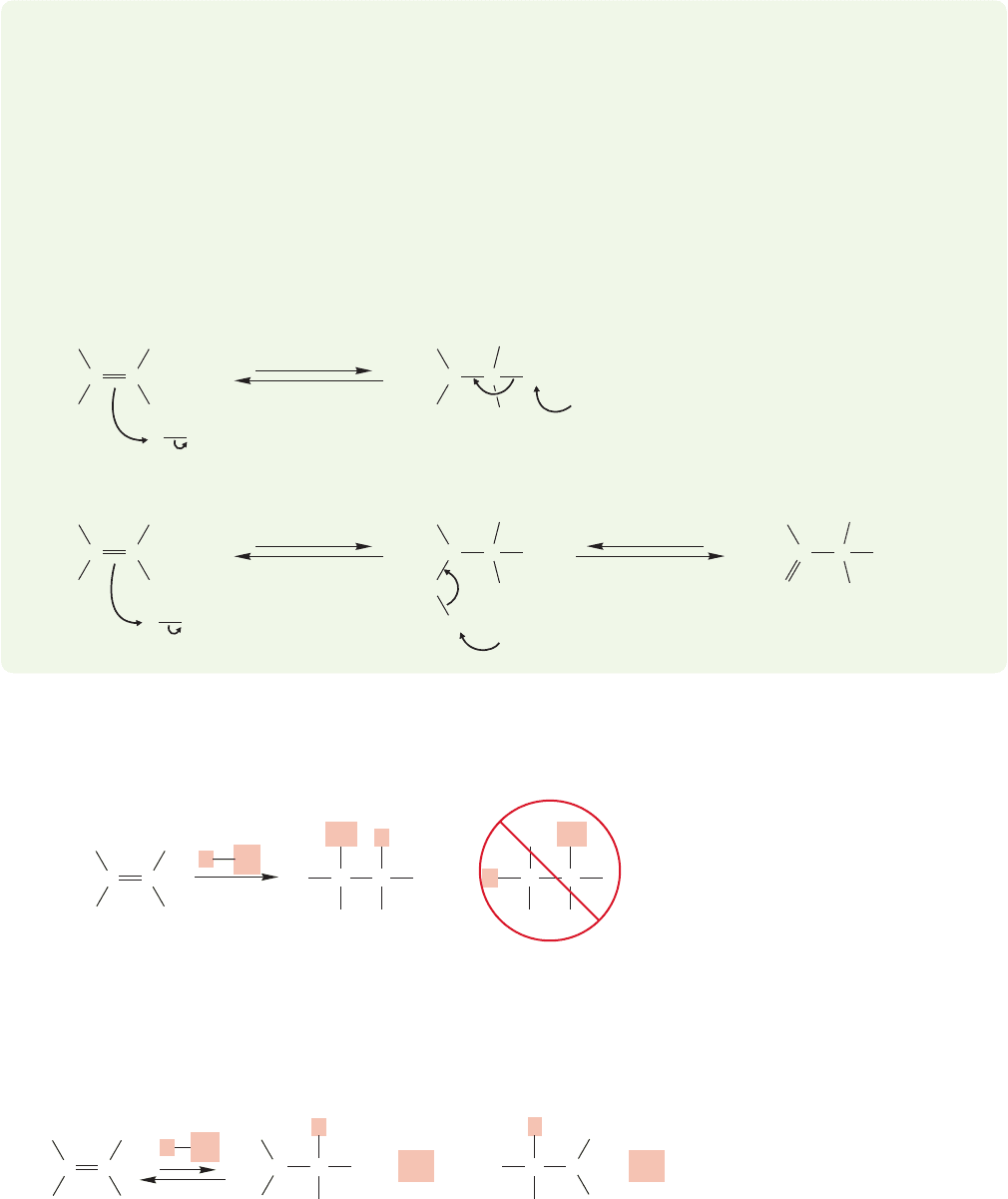
9.3 Effects of Resonance on Regiochemistry 367

WORKED PROBLEM 9.2 Chloride formation isn’t the only result possible in the

reaction of Figure 9.2. In the early stages of this reaction, a small amount of an

alkene isomeric with the 2,3-dimethyl-2-butene is produced. What is this other

alkene, and what is the mechanism of its formation?

ANSWER Remember the E1 reaction! Just because we are starting a new subject

does not mean that old material disappears. Cations will undergo the E1 and S

reactions as well as the addition reactions encountered in this chapter. The cation

formed on protonation of 2,3-dimethyl-2-butene can undergo an E1 elimination

in two ways. Path a simply reverses to give starting compounds back, whereas

path b leads to the isomeric alkene, 2,3-dimethyl-1-butene.

1,1-Dichloroethane not 1,2-DichloroethaneVinyl chloride

(chloroethylene)

ClH

FIGURE 9.5 Addition of hydrogen

chloride to vinyl chloride gives

1,1-dichloroethane as the major

product, not 1,2-dichloroethane.

Hor

–

FIGURE 9.6 The two cations that

could be formed by protonation of

vinyl chloride.

the alkene differ from each other.The preferential formation of one isomer in those

cases where a choice is possible is called regioselectivity. The next few sections dis-

cuss factors influencing regiochemistry.

C H

(a)

protonation

elimination

–

C H

(b)

protonation

elimination

protonation

elimination

path a

path b

–

CCH

When hydrogen chloride adds to vinyl chloride (chloroethylene), the major product

is 1,1-dichloroethane, not 1,2-dichloroethane (Fig. 9.5).The best way to rationalize the

regiospecificity of this reaction is to examine the two possible intermediate carbocations

(Fig. 9.6) and see which is more stable. Remember: The molecule has an empty p orbital

on the carbon bearing the positive charge. In the first carbocation shown in Figure 9.6,

this empty orbital overlaps with a p orbital on chlorine, which contains two electrons.

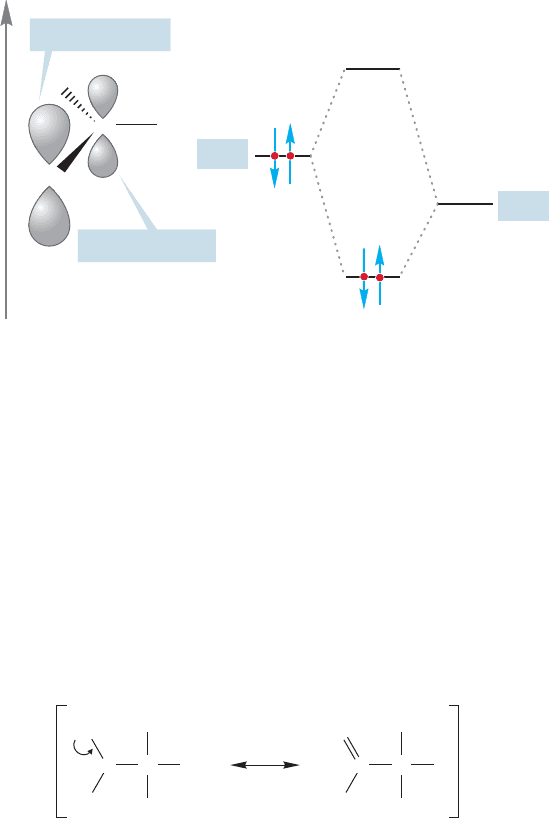
368 CHAPTER 9 Additions to Alkenes 1

This overlap of filled and empty orbitals produces strong stabilization (Fig. 9.7).

In this ion, the chlorine atom helps bear the positive charge. Only when the charge

is adjacent to the chlorine is there stabilization through overlap of the empty 2p

orbital on carbon with the filled 3p orbital on chlorine. Because this stabilization

is not available to the second carbocation shown in Figure 9.6, it is much harder

to form.

Energy

C 2p

Cl 3p

Empty 2p orbital

Filled 3p orbital

FIGURE 9.7 Stabilization of a cation

through overlap of a filled and empty

orbital.

In this resonance

form, the carbon bears

the

ositive char

In this resonance

form, the chlorine bears

the

ositive char

FIGURE 9.8 Resonance stabilization

of a carbocation.

Another way to represent this stabilization is through a resonance formulation.

(For an earlier treatment of resonance see Section 1.4, p. 22.) There are two rea-

sonable ways to draw a Lewis structure for the cation next to chlorine (Fig. 9.8).

In one, the carbon is positively charged, and in the other it is the chlorine that

bears the positive charge.The two representations differ only in their distribution

of electrons,which means they are resonance forms of the same structure. By itself

neither is exactly right, as you learned in Section 1.4. Neither by itself shows that

two atoms share the charge. Taken together they do. The real structure of this

cation is not well represented by either form alone, but the two combined do an

excellent job.

The molecular orbital picture we developed in Figure 9.7 does a good job of

showing the stabilization, but it is not particularly well suited for bookkeeping

purposes. In practice, the ease of bookkeeping—of easily drawing the next chemi-

cal reaction—makes the resonance picture simpler to use.The price is that you have

to either draw two structures (or more in other cases) or adopt some other code that

indicates the presence of more than one representation for the molecule. We will

see many examples in the next pages and chapters.